ABSTRACT

The asymmetric parabolic double quantum wells (DQWs) with resonant levels (the ground state energy in one well coincides with the first excited state energy in another well) is analyzed. The splitting of these levels and the tunneling times are calculated. If the typical life time of the excited state is much smaller than the tunneling time between wells, the charged particle can radiate as a result of the quantum transition from the excited state to the ground state. In the opposite case, the asymmetric DQWs can be treated as a metastable excited nanosystem regardless of that the dipole transition from the excited state to ground state is permitted. The lifetime of this metastable state can be considerably reduced by putting it into a resonant cavity. The possibility of coherent radiation of an ensemble of asymmetric DQWS is discussed.

1. Introduction

The tera hertz range of the electromagnetic spectrum spans the frequency range between microwave and mid infrared (100 GHz - 10 THz). This frequency range is still the least exploited region of the spectrum owing to the very limited availability of suitable sources and detectors [1-4]. The double quantum wells can be considered as possible sources of the terra hertz radiation [5,6]. Recently, an efficient THz generation within multiple quantum wells has been reported in [7].

The quantum mechanical tunneling in symmetric double quantum wells is accompanied by quantum transitions between the split levels, which are used in masers [8,9]. The solution of the Schrodinger equation for the asymmetric DQWs and finding the energy level splitting to such systems are more complex than the symmetric ones. It seems that the reliable analytic results can be obtained only for the parabolic DQWs. For arbitrary wells the problem may be solved only numerically. Usage of the WKB approximation [10,11] for calculation of the level splitting can give good accuracy only for the high levels.

In this paper, we consider the tunneling of a charged particle in asymmetric DQWs that is formed by applying the gate voltage to the symmetrical parabolic DQWs. By tuning the electric field, it is possible to obtain coincidence of the ground state energy in one well and the first excited state energy in another well. The quantum tunneling takes place between these levels. It differs from the tunneling between ground state levels in symmetric DQWs. In our case, the tunneling particle may execute a quantum transition from the excited state to the ground state in the same well, radiating the quantum of the electromagnetic energy, with the frequency much larger than the frequency of transitions between the doublet levels that appear as a result of splitting. Spontaneous emission from a two level atom and tunneling in a double quantum well potential was studied recently [12].

The paper is organized as follows. In the second section, the splitting between resonant levels in the asymmetric DQWs is calculated with the help of the known eigenfunctions of the individual wells. To check this method, we calculated the splitting of the ground state energy levels of symmetric parabolic DQWs and compared it with known results obtained by other methods. In the third section, the tunneling time between the resonant levels-the ground state in one well and the first excited state in another one is calculated and compared with the lifetime of the excited state. The parameters of the metastable excited state of the asymmetric DQWs and organization of the dipole radiating state are discussed. In the conclusion, we summarize the results obtained in the paper.

2. Splitting of Resonant Levels of Asymmetric Parabolic DQWs

We consider a particle of mass and charge that moves in the 1D potential.

The first term describes the potential of the symmetric parabolic DQWs (is the frequency, is a coordinate of the particle, are the positions of minima of the left and right parabolas) and the second one is the potential energy of the particle in the electric field. The Schrodinger equation of this problem can be written in the form

(1)

Here we introduced the potential energies of the left and right wells (See Figure 1).

(2)

with the following denotations,

, and.

The electric field makes the QDWs asymmetric with new positions of minima and, but the distance between minima remains the same. At the potential energy of the wells coincides

as for the symmetric DQWs.

For the electric field in the positive direction of the x-axis, the right well, as a whole, goes down by

and the left well is lifted by.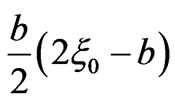

For “far” separate wells the low lying levels practically coincide with those ones of the simple harmonic oscillator:

(3)

Here the integers cannot be large and are restricted by the inequality

(4)

Energy levels (3) relate to the separate left and right wells and do not coincide for an arbitrary electric field. It is worth noting that energy splitting of the ground state levels in a symmetric DQWs is exponentially small Therefore, even small applied electric field violates the tunneling in symmetrical DQWs. However, the tunneling between wells can be restored by tuning the electric field in such a way that some energy levels coincide, (see Figure 2). This resonance condition specifies the electric field, at which the tunneling between two wells is possible. In particular the tunneling, between the ground state energy level of the left well and the first excited state energy level of the right well, is possible at. The corresponding electric field and gate voltage are given by the formulas

(5)

Now we calculate splitting of the coinciding levels in the case of. We consider the case when the ground state energy of the left well coincides with the first excited state energy. The normalized wave functions related to them are given by the following expressions

(6)

The functions (6) describe the ground state in the left well and the first excited state in the right well provided that the electric field is given by (5). The solution of the Schrodinger equation (1) we seek as the linear combination of functions (6)

, (7)

with unknown coefficients and. It is convenient to write down the Hamiltonian of the Schrodinger equations (1) in the form

(8)

Multiplying (8) by and according to (6) and integrating over, we obtain a system of two linear homogeneous algebraic equations for land.

(9)

were the matrix elements of the Hamiltonian (8) and the overlapping integral are given by

(10)

In matrix elements (10), we keep only the leading terms (with account of the exponentially small parameter and use the “tuned” electric field. The nontrivial solution of these systems is obtained from the condition of vanishing of its determinant. It gives the energies of two levels appearing from the resonant levels of the asymmetric DQWs

(11)

At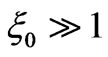, these levels are very close and we will call them a resonant doublet. The wave functions related to these levels are

(12)

While obtaining the relation between coefficients, we neglect a small factor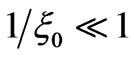. The splitting or the distance between these levels is

(13)

This result shows that splitting of the resonant levels is exponentially small. It is interesting to compare this result with the splitting of the ground state level of the symmetric harmonic DQWs obtained by the different method in [13,14]

(14)

A comparison gives

We note that the splitting (14) can be obtained acting in same manner as above with the Hamiltonian of the

symmetric double quantum wells (no external electric field). This can be considered as a confirmation of the result (13) obtained with the help of the known wave functions of “far” separated wells.

3. Tunneling and Radiation in the Asymmetric Parabolic DQWs

The time dependent wave functions, which describe the above obtained doublet (13), may be written in the form

, (15)

where and are the lower and upper energies of the doublet, and are the wave functions of these energy levels. It is known that wave function (15) can be rewritten as [15]

, (16)

where is the splitting given by (13). This shows that the charged particle in the asymmetric DQWs oscillates back and forth between the resonant states and with a frequency in the left and right wells, respectively. This oscillatory “motion” can also be considered as a quantum mechanical tunneling. Now it would be relevant to discuss how to realize the state of the asymmetric DQWs with the tunneling electron. Let us consider the symmetric DQWs that could be obtained if the electric field in (1) is set to be zero. The ground state of this problem is the energy doublet (14) with the electron tunneling between the wells. If we switch on a very weak but finite electric field, the resonance of the ground state levels will be violated. The level in the left well will be slightly higher and the level in the right well will be slightly lower comparing with the ground state energy at. The electron is captured by the left or the right well with a probability 1/2. Increasing the electric field up to the value (5), we meet the resonance condition of the ground state level in the left well and the first excited state in the right well (see Figure 2). If the electron was in the left well, it will be in the tunneling regime. If it was captured in the right well, it will stay on the ground state level. The probability of realization of these states is 1/2. If we have an ensemble of asymmetric DQWs, half of them will be in the tunneling regime and can be treated as excited states. Below we deal with these states.

The typical time of the tunneling motion between wells is. With the help of (13), we get

. (17)

Relation (17) allows one to evaluate how much time the particle spends in one of the wells. The particle “sitting” in the right well may return back to the left well but it may also perform a spontaneous quantum transition to the ground state of the right quantum well with radiation of a quantum. Such a transition occurs if the average life time of the first state of harmonic oscillator is much less than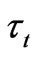. The average life time of the first excited state of a harmonic oscillator is known [15]

, (18)

where is the matrix element of dipole transition of the harmonic oscillator. Keeping in mind that and are the probabilities per unit time of tunneling and spontaneous radiation, respectively, we introduce the probability of tunneling

, (19)

and the probability of spontaneous radiation

, (20)

The spontaneous radiation of the asymmetric parabolic DQWs takes place if or the following inequality holds true. It is convenient to present the last inequality in the form

(21)

To solve the above inequality, it would be convenient to introduce Hz and cm. This means that we measure the frequency in 10 THz and the length in 10 nm. Substituting the numerical values of the physical constants and value of the typical effective mass of electron (is the mass of a free electron) as for GaAs, we obtain the simplified inequality

, (22)

where. It satisfies with and allows us to find the frequency for a given or vice versa. For example, exploiting the terra Hz frequency range, we obtain that for 10 THz, nm and for 1 THz, nm. We can see that realization of the above proposed mechanism of radiation of the resonant levels requires the nanoscale DQWs.

With the help of (5) and above obtaine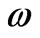d and one can estimate the gate voltage and corresponding strength of the electric field 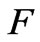required for formation of the resonant levels in the DQWs. The results read: for 10 THz, nm, V and V/cm; for 1THz, nm, V and V/cm. These fields can be easily obtained in laboratory. It is necessary to remember that formation of the resonant levels requires the fine tuning of the gate voltage.

On the basis of the above report, we can claim that the asymmetric DQWs with resonant levels: the ground state in one well and the excited one in another well may be a source of spontaneous radiation in a THz frequency range. After emission of the quantum from the right well, the electron stays in the ground state of the right well. If we change the direction of the electric field, the system will be again in the excited state during the time.

The lateral current pumped GaAs/AlGaAs quantum wells were used as a source of incoherent THz radiation in [16].

Now we consider the case when inequality (22) is reversed. In the THz frequency range for the accepted model it requires that a distance between the wells be smaller than (45 nm for THz and 140 nm for THz). In this case, the electron “jumps” between the wells so fast that it has no time to make a quantum transition in the right well from first excited state to the ground state. In other words, the system will be in the excited state with the electron tunneling between the wells. We consider this state as a metastable nanosystem.

It is possible to force the system to emit the quantum if we put the asymmetric DQWs into a resonator with the electromagnetic radiation. In this case the life time of the excited state radiation decreases and may be written in the form [15]

(23)

where is a typical time of the stimulated emission, and is a density of the external radiation of a frequency. It is seen from (23) that at, the life time of the system with respect to stimulated radiation can be made much smaller than the tunneling time (17).

It is necessary to note that low lying energy levels of a harmonic oscillator are equidistant with spacing. The equidistance between levels is violated by the splitting of resonant levels, which depend on the number of levels. However, the other models of asymmetrical DQWs (for example, rectangular DQWs with a gate voltage) do not possess the equidistant resonant levels and the problem of re absorption will be removed.

The possibility of the generation of the THz radiation in resonant tunneling structures with several quantum wells was discussed in [17]. Moreover, it is claimed in [18] that the combined effects of the static electric field and the THz coherent radiation field can be useful in designing new optoelectronic devices.

4. Conclusions

In this paper, we obtained the condition of formation of the resonant levels for a model a charged particle in parabolic DQWs with an applied electric field. The tuned electric field provides the coincidence of the energies of the ground state in one well with the energy of the first excited state in another well.

The account of degeneracy of the quantum states in the wells results in the splitting of the two-fold degenerate level and in the tunneling of the particle between the wells. The typical time of tunneling or the time staying of the electron in one of the wells is calculated. 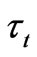But unlike the tunneling of the particle in the ground state of a symmetric parabolic DQWs now the electron may execute also a spontaneous quantum transition from the excited state of the well with radiation of a quantum provided that is much larger than the typical life time of the excited state. In the opposite case, the asymmetric DQWs under consideration may be treated as a metastable nanosystem.

The radiation time of the asymmetric DQWs with parameters placed into a resonator of the frequency may be made considerably smaller than. At large enough density of the stimulated radiation it is possible to get. This means that the metastabl state of the nanosystem can be transformed in the dipole radiating state.

5. Acknowledgements

The authors are thankful to Professor Singh P. and Dr. Mulugeta B. for useful discussions of the results obtained in the paper. Authors are thankful to the School of Graduate Studies of Addis Ababa University for a financial support.

REFERENCES