Journal of Modern Physics
Vol.06 No.01(2015), Article ID:53222,3 pages
10.4236/jmp.2015.61004
Neutron-Nucleus Total Elastic Scattering
Cvavb Chandra Raju
Department of Physics, Osmania University, Hyderabad, India
Email: cvavbc@gmail.com
Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 11 December 2014; accepted 24 December 2014; published 14 January 2015
ABSTRACT
Using an exact and complete Yukawa Potential Energy (YPE), the neutron-triton and neutron-he- lium elastic total scattering cross section is estimated and extrapolated to zero energy. The estimated value agrees pretty well with the experimental value and the procedure can be extended to any neutron-nucleus total scattering. The results are extended to the case of 4He, 7Li, 9Be and 27Al nuclei also.
Keywords:
Totalelastic Scattering Cross-Section, Complete Yukawa Potential Energy, Born Approximation, One Pion Exchange Potential

1. Introduction
The theoretical description of A = 4 systems still constitutes a challenging problem from the stand-point of nuclear few body theory. Only recently the study of alpha particle bound state reached a satisfactory level of accuracy because of new numerical methods. The 4N bound state has been calculated to a few tenths of KeV [1] -[3] . But these numerical methods are very involved. The total elastic scattering cross section 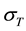 of a neutron by a Triton nucleus is discussed and compared to the experiment in [1] . Here we use a mass dependent Yukawa Potential Energy to estimate the total elastic scattering cross section of a neutron by a nucleus.
of a neutron by a Triton nucleus is discussed and compared to the experiment in [1] . Here we use a mass dependent Yukawa Potential Energy to estimate the total elastic scattering cross section of a neutron by a nucleus.
Instead of the P-3He elastic scattering at low energies, the situation is simpler for the n-3H elastic scattering. There is no Coulomb complication with the latter. Experimentally, only the total cross section  has been measured with high precision for large range of energies. The extrapolation of the measured
has been measured with high precision for large range of energies. The extrapolation of the measured 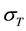 to zero energy is straightforward. The value obtained is [1] ,
to zero energy is straightforward. The value obtained is [1] ,
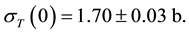 (1.1)
(1.1)
The above value is a very precisely measured value for the neutron-triton elastic scattering. There is another parameter called the coherent scattering length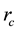 . The coherent scattering length has been measured by neutron-interferometry techniques. The most recent value reported in the literature is [1] ,
. The coherent scattering length has been measured by neutron-interferometry techniques. The most recent value reported in the literature is [1] ,
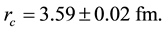 (1.2)
(1.2)
Estimation is also obtained from P-3He scattering data for the coherent scattering length through Coulomb- corrected R-matrix theory. This gives [1] ,
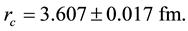 (1.3)
(1.3)
The above value is also an approximate experimental value. The theoretically obtained values are [1] - [3] ,
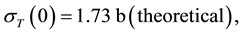 (1.4)
(1.4)
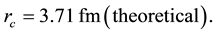 (1.5)
(1.5)
In this paper we estimate the above experimental values with an exact Yukawa Potential Energy function and by using the methods of ordinary quantum mechanics. In Section 2, we present the relevant theory and apply it to the nucleus triton. In Section 3 the estimations are extended to many more nuclei with ease. We present our conclusions in Section 4.
2. Complete Yukawa Potential Energy
The complete Yukawa Potential Energy function operative for any nuclear process is given by,
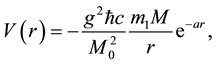 (2.1)
(2.1)
where, 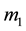 and M are the masses of the interacting parts of the nuclear matter. For example for the neutron- nucleus scattering
and M are the masses of the interacting parts of the nuclear matter. For example for the neutron- nucleus scattering 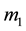 is the rest mass of the neutron and M is the rest mass of the scattering nucleus. There are two unknown parameters in the above YPE. The parameter
is the rest mass of the neutron and M is the rest mass of the scattering nucleus. There are two unknown parameters in the above YPE. The parameter 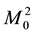 is specific to each nucleus [4] - [7] . We have shown in [4] - [7] whenever the Yukawa factor
is specific to each nucleus [4] - [7] . We have shown in [4] - [7] whenever the Yukawa factor 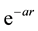 is set equal to one the resulting central potential yields the energy spectrum of the nucleus. By comparing the ground state energy with the known binding energy of the nucleus we can determine the parameter
is set equal to one the resulting central potential yields the energy spectrum of the nucleus. By comparing the ground state energy with the known binding energy of the nucleus we can determine the parameter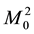 . In our computations we will use this data from the references [4] - [7] .
. In our computations we will use this data from the references [4] - [7] .
The other unknown is a of the Yukawa factor. For One Pion Exchange Potential (OPEP),
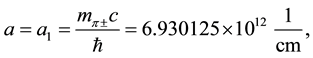 (2.2)
(2.2)
and,

The parameter 

We now have the entire YPE for any nucleus. For example for the nucleus 3H, the parameter 

We can now calculate the scattering amplitude for the scattering of a neutron of mass 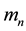

where, the reduced mass of the neutron is given by,

Here, the wave number, 
scattering cross section can be obtained by squaring Equation (2.6). The total elastic scattering cross-section can then be obtained by integrating the differential cross-section. The Yukawa potential has the advantage relative to the Coulomb potential that certain integrals converge to finite numbers. The total elastic scattering-cross section is given by,

The above expression gives the total elastic scattering-cross section for a neutron by any nucleus. It can be used for any neutron-nucleus scattering provided there are no resonances and there is no absorption. The total elastic scattering cross-section extrapolated to zero energy is given by,

where,

The above Equations (2.9) and (2.10) can be used to compute the total scattering cross section at zero energy and the coherent scattering length. The required masses and other data are given in the table given in the next section. For 3H,
When


And when, 


The above values obtained should be compared with the experimental value quoted in Equations (1.1) and (1.2). The average value of (2.11) and Equation (2.13) is 1.7285 barn. This should be compared with the value given by Equation (1.1). Similarly the average coherent radius is given by, 3.70846 fm.
3. Theoretical Results for Some Nuclei
Below we tabulate the estimated results for different nuclei. Table 1 is self-explanatory.
Table 1. The estimated results for different nuclei.
For completeness, we have also included the estimation of the Triton nucleus as well. The ease with which the bulk nuclear properties can be obtained with the YPE can be seen from Table 1. The mass M is obtained from Ref. [8] .
The scattering of a neutron by Helium-3 or Helium-4 is quite difficult to measure. But scattering of neutrons of different energy by an Aluminum foil must not be very difficult to conduct. If measurements are made for different neutron energies for the elastic total scattering off Aluminum target our results can be put to test. This will pin down the non-relativistic YPE for any nucleus. Once it is confirmed, many bulk properties of a nucleus can be predicted with ease.
4. Conclusion
In this paper an exact YPE function is used to predict the total elastic scattering-cross section of a neutron by any nucleus. For all nuclei for which the parameter 
other interactions. The interaction constant 

Acknowledgements
The author is very grateful to V. G. Krishnan, Prudhvi R. Chintalapati and G. Prasad.
References
- Fisher, B.M., Brune, C.R., Karwowski, H.J., Leonard, D.S., Ludwig, E.J., et al. (2006) Physical Review C, C74, Article ID: 034001. http://dx.doi.org/10.1103/PhysRevC.74.034001
- Viviani, M., Girlanda, L., Kievsky, A. and Marcucci, L.E. (2013) Physical Review Letters, 111, Article ID: 172302. http://dx.doi.org/10.1103/PhysRevLett.111.172302
- Lazauskas, R. and Carbonell, J. (2011) Physical Review C, 84, Article ID: 034002. http://dx.doi.org/10.1103/PhysRevC.84.034002
- Raju, C.C. (2013) Journal of Nuclear and Particle physics, 3, 25-28.
- Raju, C.C. (2013) Journal of Modern Physics, 4, 459-462. http://dx.doi.org/10.4236/jmp.2013.44064
- Raju, C.C. (2013) Journal of Modern Physics, 4, 1180-1184.
- Raju, C.C. (2014) Modern Physics Letters A, 29, Article ID: 1450027. http://dx.doi.org/10.1142/S0217732314500278
- Wong, S.S.M. (1996) Introduction to Nuclear Physics. Prentice-Hall, 457.



