Communications and Network, 2013, 5, 176-180
http://dx.doi.org/10.4236/cn.2013.53B2034 Published Online September 2013 (http://www.scirp.org/journal/cn)
A New Family of Optical Codes Based on Complementary
Theory for OCDMA System*
Xing Chen, Weixiao Meng
School of Electronics and Information Engineering, Harbin Institute of Technology, Harbin, China
Received June, 2013
ABSTRACT
A new family of optical codes for Optical Code-division Multiple Access (OCDMA) systems, named as Optical Com-
plementary Codes (OCCs), is proposed in this paper. The constructions of these codes consist of multiple sub-codes,
and the codes have an auto-correlation interference constraint as 0 and a cross-correlation interference constraint as 1.
Compared with conventional optical codes such as OPCs, OOCs and 2-D OOCs, the OCC has a shorter code length and
higher code efficiency with better correlation property.
Keywords: Optical Code-division Multiple Access (OCDMA); Complementary Code
1. Introduction
Optical Code Division Multiple Access (OCDMA) in-
troduces CDMA to optical communication system and
combines the flexibility of CDMA system and the
broadband of fibre communication system. Compared
with Wavelength-division Multiplexing (WDM) and
Time-division Multiplexing (TDM), OCDMA shares the
frequency resources and time resou rces with all the users
by signature codes. It is the only way to achieve an
asynchronous and decentralized network with the whole
bandwidth utilized by each user [1]. Besides, the
OCDMA system shares the same security potential with
the radio frequency CDMA system because they have a
similar spectrum spreading method.
Optical CDMA is a suitable access method to achieve
random access, high capacity and contention free espe-
cially for busty traffic. Besides, OCDMA can be effi-
ciently used with WDM and TDM in practical system to
satisfy different traffic requirements and multiple ser-
vices. For now, OCDMA is an important method for
achieving all-optical networks.
Optical codes for OCDMA are totally different from
codes for the CDMA systems in wireless communica-
tions, and the most important difference is th at there is no
negative signal in optical transmission. Although some
bipolar OCDMA systems have been proposed, they are
not well received by the majority because of the high
cost and complexity in implementation [2]. Hence, the
optical codes for OCDMA are unipolar codes mainly,
and in this article, we will discuss the unipolar optical
codes only. Since finding a proper optical code set is a
very important issue in OCDMA, this paper will propose
a new family of optical codes achieving optimum corre-
lation properties, named as optical complementary codes
(OCCs).
The rest of this paper is organized as follows. In the
next section, the theory of complementary codes is in-
troduced first, and then the characteristics of optical
codes which have ideal correlation properties are de-
duced. In section III, the construction method of optical
complementary coding is proposed with examples. Sec-
tion IV discusses the correlation properties and capacities
of OCCs. Section V concludes this paper.
2. Fundamental Theories Involved in OCCs
2.1. Complementary Codes
The concept of complementary codes is introduced firstly
in 1949 by Golay with the name as Complementary Se-
ries [3]. In 1961, he proposed the theory and a construc-
tion method for Complementary Series [4]. The series
proposed by Colay is constructed with a pair of binary
sequences in the same length, and he calls each series
sub-code. The auto-correlation and cross-correlation are
different from those of traditional CDMA signature
codes. Let a and b denote two complementary sequences
where 12
{, }

aaa and 12 {, }

bbb, . The operation
of complementary correlations is a little complex than
the conventional one. In complementary correlations,
each sub-codes correlate with each other correspondingly
and then the results will be summed tog ether. Th is opera-
ab
*This Article is sponsored by National Science and Technology Major
Project (2012ZX03004003)

X. CHEN, W. X. MENG 177
tion is described as follows:
the complementary auto-correlation is deno ted as
112 2

aa=a aaa  (1)
the complementary cross-correlation is deno ted as
112 2

ab=a bab  (2)
where the symbol means the inner product. 
In 2001, Hsiao-Hwa Chen evolved the complementary
pairs into complementary codes with multiple sub-codes
which could achieve better orthogonality. These codes,
called orthogonal complete complementary codes [5],
decreased the MAI (Multi Access Interference) of CDMA
systems with simple code constructions. In this paper, we
will construct OCCs using a similar method as the work
of [5]
2.2. Characteristics of Optical Codes with Ideal
Complementary Propertie
Even though the OCDMA is introduced from CDMA
system in wireless communication and share a similar
construction with it, the codes for OCDMA is quite dif-
ferent from those in CDMA. In fibre communication
system, the data are encoded with binary codes, which
are 0s and 1s, while the codes in CDMA are bipolar code
described as -1s and +1s. So, it is impossible to achiev e a
perfect orthogonal property. Though some of the re-
searchers have proposed some systems with bipolar
codes, they are not well received because of their com-
plexity and skyscraping cost. With the discussion of bi-
nary codes in optical CDMA systems.
Since the code length and code weight will influence
the properties of optical codes, however, to the best of
our knowledge, th ere is no research on the characteristics
of optical codes with ideal auto- and cross- correlation
has been published. Meanwhile, it is very important for
this paper to introduce the optical complementary codes,
so it is reasonable to deduce the characteristics. Besides,
the optical complementary codes are based on these
derivations.
The codes in OCDMA systems are presented with
their parameters. To a specific set of optical codes, it is
described as (, ,,)
ac
nw

 , where n is the length of the
codes, w is the hamming weight of a code, a

and c

are the constrains of auto-correlation and cross-correla-
tion respectively. Let code set denote (,C, ,
a) c
nw


}
n
b, and 12 n, 12 ,
. Then, the code set must satisfy the follows:
a,b C
ab {,aaa, ,}a{,bb,,b
1
1
where0
where 0
n
n
n
aiir
in
ciir
i
aa r
ab r






 
 


(3)
In these equations r is any integer which denotes the
shift of code chips and n

means the addition with
modulo n.
MAI is the most important interference in OCDMA
system [6]. In order to minimize the MAI, the best way is
to design a new family of codes with better correlation
properties. Furthermore, the OCDMA systems are in-
herently chip-asynchronous because the users access the
systems randomly without coordination [7], so it is im-
portant for codes to achieve a lower auto-correlation
constrains.
Let denote a one dimensional optical code set
C
, )
ac
(, ,nw

 ,

a,bC , 12 n, 12 n,
{,,, }aa aa{,,, }bbbb

ab.

a is the shifted sequence with a

shifts,
and ()

bis the shifted sequence with b

shifts. ,a
S

is the auto- correla tion value of and a()

ac, S
,

is the
cross-correlation value of a and ()

b. a and c
S are the
sum of S
,a
S

and c,
S

respectively. Hence, we co uld get
(
,
,
a
c
S
S





aa
ab


）
（）
(4)
It can be also described as
,0112211
,1122311
,21 324112
,1 121121
ann
ann
an
annnnn n
Saaaa aaaa
Saaaa aaaa
Saaaaaaaa
Saaaa aaaa



nn
n
n

 

 
 
 
 





(5)
Then, we can get
111 11
2
,
00000
nnn nn
aaiij ij
iijij
SS aaaaw
 







 (6)
When the shift is 0, the auto-correlation reaches its
peek, and this condition dose not consist interference.
Then, we can get the sum of auto-correlation interference
as
2(1)wwww


In a similar way, we can get
11111
2
,
00000
nnnnn
cciij ij
iijij
SS ababw








 (7)
and the sum of cross-interference is .
2
w
It could be concluded that, with the increase of code
weight w, the sum of auto-correlation and the sum cross-
correlation both increase sharply. In order to achieve the
minimum of interference and the best of correlation
properties, the code weight should be 1. Then the sum of
auto-correlation is 0 and , and we could get each
auto-correlation interference is 0 and the maximum of
cross-correlation is 1, i.e. correlation constraints are
1
c
S
0
a


and 1
c


.
But the auto-correlation peek of this kind of one di-
mension codes is 1, which could not be applied into
practical OCDMA system. In order to achieve a lager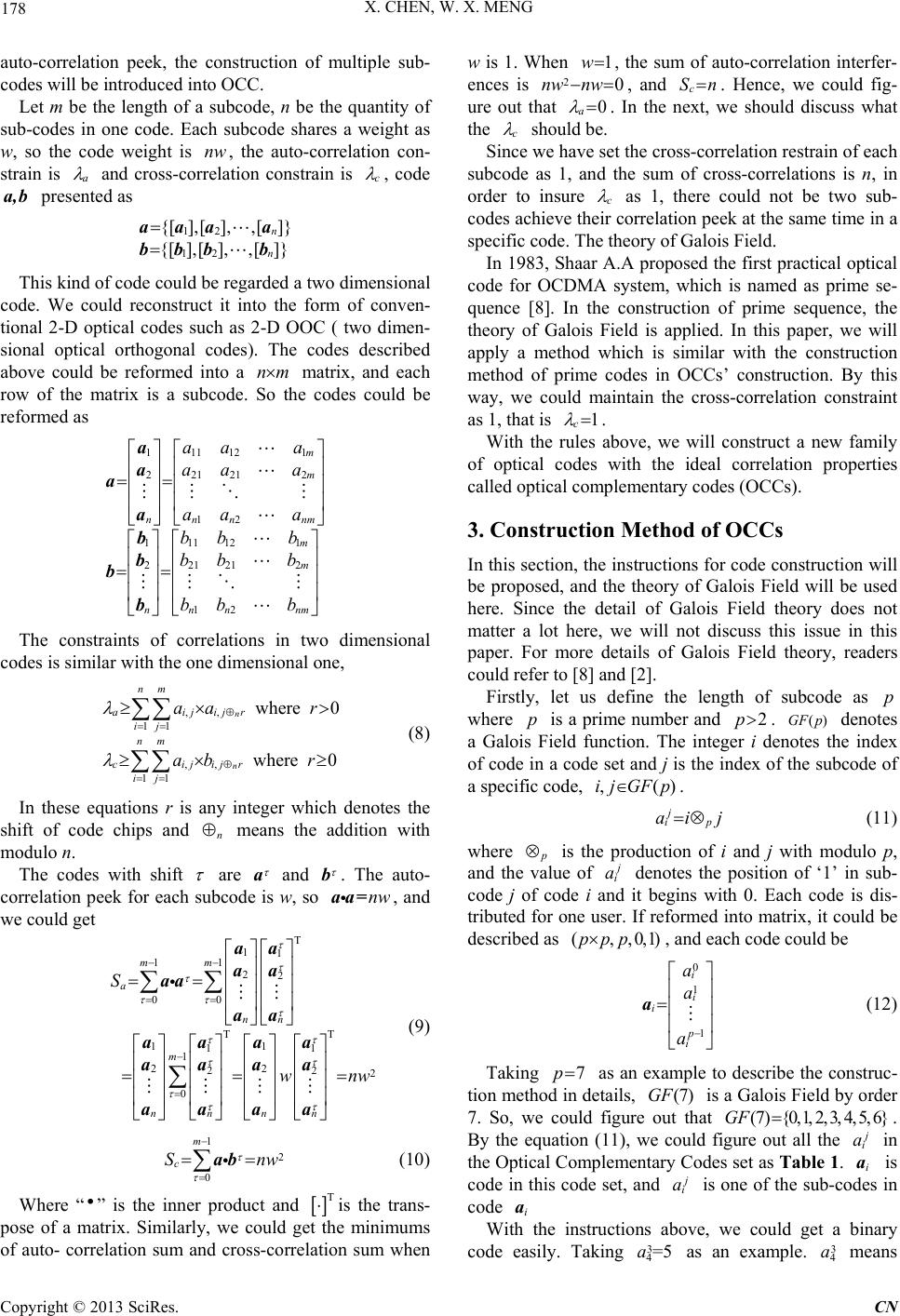

X. CHEN, W. X. MENG
178
auto-correlation peek, the construction of multiple sub-
codes will be introduced into OCC.
Let m be the length of a subcode, n be the quantity of
sub-codes in one code. Each subcode shares a weight as
w, so the code weight is , the auto-correlation con-
strain is nw
a

and cross-correlation constrain is c

, code
presented as
a,b
12
12
{[ ],[],,[]}
{[ ],[],,[]}
n
n


aaa a
bbb b


This kind of code could be regarded a two dimensional
code. We could reconstruct it into the form of conven-
tional 2-D optical codes such as 2-D OOC ( two dimen-
sional optical orthogonal codes). The codes described
above could be reformed into a matrix, and each
row of the matrix is a subcode. So the codes could be
reformed as
nm
11112 1
22121 2
12
11112 1
22121 2
12
m
m
nnn nm
m
m
nnn nm
aa a
aa a
aa a
bb b
bb b
bb b
 
 

 
 
 
 
 

 
 
 
a
a
a
a
b
b
b
b


 



 

The constraints of correlations in two dimensional
codes is similar with the one dimensional one,
,,
11
,,
11
where 0
where 0
n
n
nm
aijijr
ij
nm
cijijr
ij
aa r
ab r












(8)
In these equations r is any integer which denotes the
shift of code chips and means the addition with
modulo n. n

The codes with shift

are

a and

baa . The auto-
correlation peek for each subcode is w, so , and
we could get nw=
T
11
11
22
00
TT
11
11
1
22
22
2
0
mm
a
nn
m
nnnn
S
wn



















 
 

 
 
 


aa
aa
aa
aa
aaaa
aaaa
aaaa


w

nw

(9)
1
2
0
m
c
S





ab (10)
Where “” is the inner product and is the trans-
pose of a matrix. Similarly, we could get the minimums
of auto- correlation sum and cross-correlation sum when
w is 1. When

T

1w

, the sum of auto-correlation interfer-
ences is 2 nw 0nw


0
a
, and . Hence, we could fig-
ure out that c
Sn


. In the next, we should discuss what
the c

should be.
Since we have set the cross-correlation restrain of each
subcode as 1, and the sum of cross-correlations is n, in
order to insure c

as 1, there could not be two sub-
codes achieve their correlation peek at the same time in a
specific code. The theory of Galois Field.
In 1983, Shaar A.A proposed th e first practical optical
code for OCDMA system, which is named as prime se-
quence [8]. In the construction of prime sequence, the
theory of Galois Field is applied. In this paper, we will
apply a method which is similar with the construction
method of prime codes in OCCs’ construction. By this
way, we could maintain the cross-correlation constraint
as 1, that is 1
c


.
With the rules above, we will construct a new family
of optical codes with the ideal correlation properties
called optical complementary codes (OCCs).
3. Construction Method of OCCs
In this section, the instructions for code construction will
be proposed, and the theory of Galois Field will be used
here. Since the detail of Galois Field theory does not
matter a lot here, we will not discuss this issue in this
paper. For more details of Galois Field theory, readers
could refer to [8] and [2].
Firstly, let us define the length of subcode as
where is a prime number and . denotes
a Galois Field function. The integer i denotes the index
of code in a code set and j is the index of the subcode of
a specific code,
p
p2p()GF p
,(GF)pij

.
j
p
ijai

 (11)
where
p

is the production of i and j with modulo p,
and the value of
j
i
a
(,pp
denotes the position of ‘1’ in sub-
code j of code i and it begins with 0. Each code is dis-
tributed for one user. If reformed into matrix, it could be
described as ,0,1)p

, and each code could be
0
1
1
i
i
p
i
a
a
a
i












a (12)
Taking 7p

as an example to describe the construc-
tion method in details, is a Galois Field by order
7. So, we could figure out that .
By the equation (11), we could figure out all the
(7)GF(7) {0,1,2,3,4,5,6}GF 
j
i
a
a in the Optical Complementary Codes set as Table 1. i is
code in this code set, and
j
i
a is one of the sub-codes in
code
i
With the instructions above, we could get a binary
code easily. Taking as an example. means
a
3
4=5a3 4
a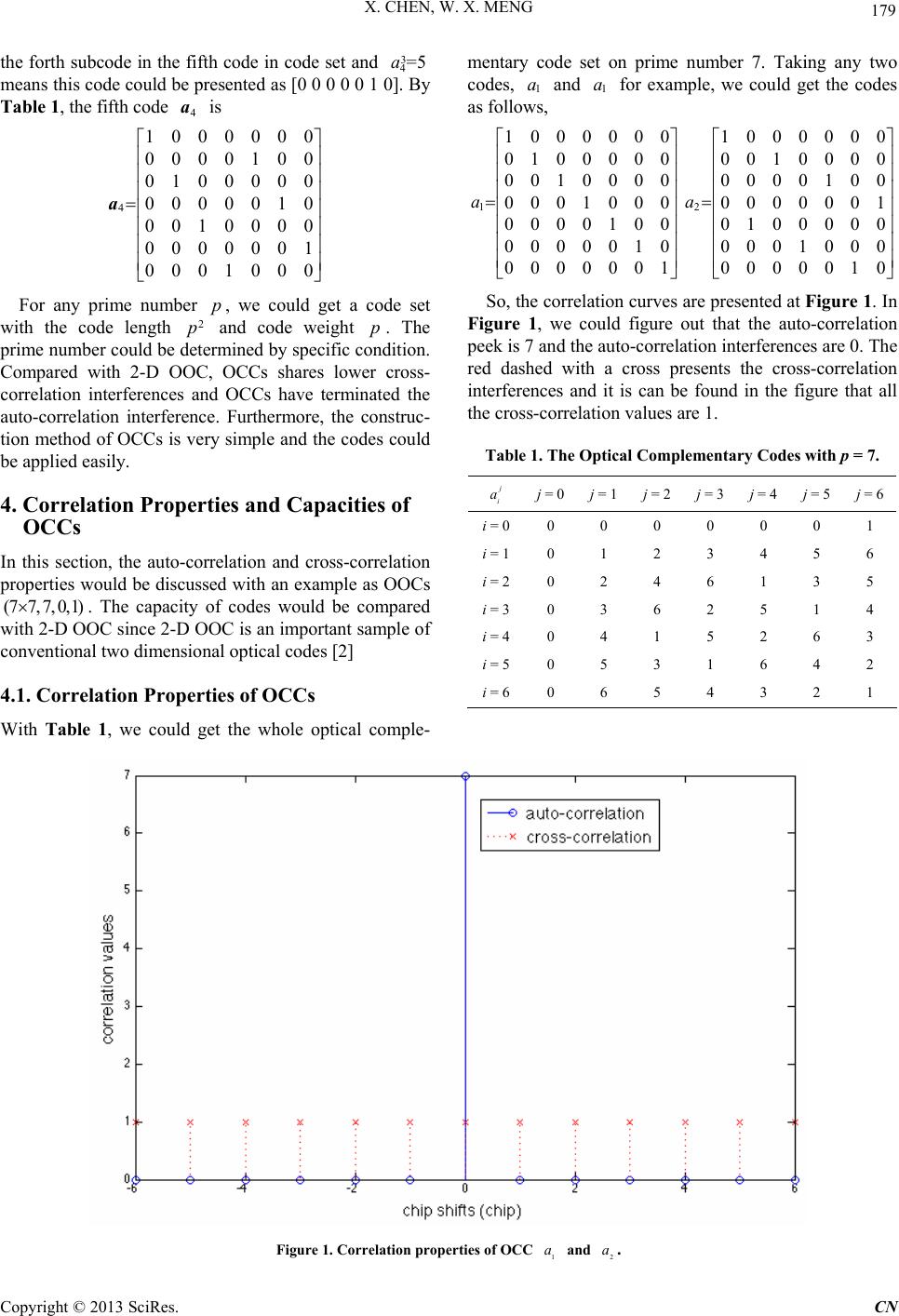

X. CHEN, W. X. MENG
179
the forth subcode in the fifth code in code set and
means this code could be presented as [0 0 0 0 0 1 0]. By
Table 1, the fifth code is
3
4=5a
4
a
1
0
0
2
p
4
1000000
0000100
0 00000
0000010
0 10000
0000001
0 01000









a
For any prime number , we could get a code set
with the code length and code weight . The
prime number could be determined by specific condition.
Compared with 2-D OOC, OCCs shares lower cross-
correlation interferences and OCCs have terminated the
auto-correlation interference. Furthermore, the construc-
tion method of OCCs is very simple and the codes could
be applied easily.
pp
4. Correlation Properties and Capacities of
OCCs
In this section, the auto-correlation and cross-correlation
properties would be discussed with an example as OOCs
. The capacity of codes would be compared
with 2-D OOC since 2-D OOC is an important sample of
conventional two dimensional optical codes [2]
(7 7,7,0,1)
4.1. Correlation Properties of OCCs
With Table 1, we could get the whole optical comple-
mentary code set on prime number 7. Taking any two
codes, and for example, we could get the codes
as follows,
1
a1 a
12
1000000 1000000
0100000 0010000
0010000 0000100
0001000 0000001
0000100 0100000
0000010 0001000
0000001 0000010
aa

 

 

 





 

 

 

 

 
So, the correlation curves are presented at Figure 1. In
Figure 1, we could figure out that the auto-correlation
peek is 7 and the auto-correlation interferences are 0. The
red dashed with a cross presents the cross-correlation
interferences and it is can be found in the figure that all
the cross-correlation values are 1.
Table 1. The Optical Complementary Codes with p = 7.
j
i
a j = 0j = 1j = 2j = 3 j = 4 j = 5j = 6
i = 00 0 0 0 0 0 1
i = 10 1 2 3 4 5 6
i = 20 2 4 6 1 3 5
i = 30 3 6 2 5 1 4
i = 40 4 1 5 2 6 3
i = 50 5 3 1 6 4 2
i = 60 6 5 4 3 2 1
Figure 1. Correlaies of OCC and
1
a2 a.tion propert

X. CHEN, W. X. MENG
180
For 2-D OOCs, whose auto-corre
o
odes are described with the
lation side lobes are
nt zeros, will have to deal with the interference in
asynchronous conditions. The cross-correlation of OCCs
is the same with 2-D OOCs but more predictable.
4.2. Capacity of OCCs
The capacity of optical c
equation (,,,)
ac
nmw

  .
According to [2]
2D-OO

C(1)
(,,)(1)
nnm
nmw ww 



 

where denotes the round down operati it is
When it comes to OCCS, for any p
w
s a new family of optical signature
ost equal to that of 2-D OCCs al-
REFERENCES
[1] K. Fouli and d Optical Coding:
(13) Principles, Applications, and Challenges,” IEEE Commu-
nications Magazine, Vol. 45, No. 8, 2007, pp. 27-34.



he 2-on. For tD OOC (,,1)ppp, the capacity of
2D ,1)1p ( -OOC(,ppp 14)
rime number p,
ith the instructions proposed in section III, we cou
find p codes for the OCC code set and each code is
distributed to one user. Basically, the capacity of OCCs
is p, which is OC C (,,1)ppp p . Hence, the capacity
of OCCs is similar with OOCs, especially when the
prime code is large enough.
Besides, the code length of 2-D OOCs is longer than
tha
ld
t of OCCs generally, which requires higher frequency
encoders and reduces the spectrum efficiency.
5. Conclusions
This paper propose
codes for OCDMA system named as Optical Comple-
mentary Codes (OCC). The auto-correlation constraints
of these codes is 0 and the cross-correlation constrains is
1, and this optical correlation property is the best that a
binary optical code set could reach. Meanwhile, the ca-
most. The construction of these codes adopted the ideas
of complementary codes and the construction of each
subcode is similar with prime sequence. With the simple
instructions, the codes could be built easily, which makes
the application of these codes very simple and practical.
pacity of OCCs isalm
M. Maier, “OCDMA an
doi:10.1109/MCOM.2007.4290311
[2] H. Ghafouri-Shiraz and M. M. Karbassian, “Optical
CDMA Networks: Principles, Analysis and Applica-
tions,” John Wiley & Sons, 2012.
doi:10.1002/9781119941330
[3] Marcel J. E. Golay, “Multislit spectroscop
the Optical Society of Amey,” Journal of rica, Vol. 39, 1949, pp.
437-444. doi:10.1364/JOSA.39.000437
[4] Marcel. J. E. Golay, “Complementary Series,” IRE Trans-
actions on Information Theory, 1961(Aprial), pp. 82-87.
[5] H. H. Chen, J.-F. Yeh and N. Suehiro, “A Multicarrier
CDMA Architecture Based on Orthogonal Complemen-
tary Codes for New Generations of Wideband Wireless
Communications,” IEEE Communications Magazine, Vol.
39, No. 10, 2001, pp. 126-135. doi:10.1109/35.956124
[6] L. Xu, I. Glesk, V. Baby, etc., “Multiple Access Interfer-
ence (MAI) Noise Reduction in a 2D Optical CDMA Sys-
tem Using Ultrafast Optical Thresholding,” Lasers and
Electro-Optics Society, 2004, Vol. 2, pp. 591-592.
[7] S. A. Khan and J. Bajcsy, “Chip-Asynchronous Binary
Optical CDMA: An Optimum Signaling Scheme for
Random Delays,” IEEE Photonics Journal, 2013, Vol. 5,
No. 2, Aricle# 7200408.
[8] A. A. Shaar and P. A. Davids, “Prime Sequences: Quasi-
optimal Sequences for or Channel Code Division Multi-
plexing,” Electronics Letters, Vol. 9, No. 21, 1983, pp.
888-889. doi:10.1049/el:19830605