Advances in Pure Mathematics
Vol.05 No.13(2015), Article ID:60982,3 pages
10.4236/apm.2015.513071
Integer Part of Cube Root and Its Combination
Zhongguo Zhou
College of Science, Hohai University, Nanjing, China

Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).


Received 6 July 2015; accepted 8 November 2015; published 11 November 2015

ABSTRACT
For the cube root of a positive integer, a direct method to determine the floor of integer combination of the cube root and its square is given.
Keywords:
Cube Root, Integer Part, Continued Fraction

1. Introduction
The continued fraction expansion of 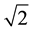 can be calculated as follow(cf. [1] -[3] ).
can be calculated as follow(cf. [1] -[3] ).

Because

hence
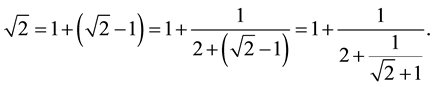
When one calculates the continued fraction expansion of  it is important to determine the integer part of
it is important to determine the integer part of 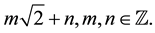 For square root, its continued fraction expansion can be obtained easily because it is circled while there is no obvious method to do so for cube root. In this note, we will determine the integer part of cube root and its combination. So we can achieve the continued fraction expansion of cube root according to the integer part of the cube root.
For square root, its continued fraction expansion can be obtained easily because it is circled while there is no obvious method to do so for cube root. In this note, we will determine the integer part of cube root and its combination. So we can achieve the continued fraction expansion of cube root according to the integer part of the cube root.
2. Main Results
Let N be a positive integer and not a cube. Denote  as its cube root. For
as its cube root. For  set
set
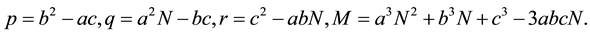
Then these numbers are satisfied the identity:
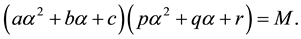 (1)
(1)
We achieve two interesting properties on 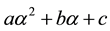 and
and .
.
Theorem 1. If , then
, then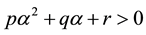 . Therefore the number
. Therefore the number 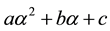 has the same sign as M.
has the same sign as M.
We consider two cases respectively.
1) If
a) If


since every term in the last expression is nonnegative.
b) If
The above inequality holds because
2) If
a) If 
We also have
Hence by the identity (1)
b) If 


So we show that 

Remark 1. The result is very amazing. Because the quotient ring 




But it is surprising that the number 
Theorem 2. If 

That is to say, 
According to Theorem 1,
Hence
So
The proof is completed.
Remark 2. Applying the Theorem 2, we can design an algorithm to calculate the continued fraction expansion of the cube root
Acknowledgements
The authors wish to thank Prof. Xiangqin Meng for her some helpful advices.
Cite this paper
ZhongguoZhou, (2015) Integer Part of Cube Root and Its Combination. Advances in Pure Mathematics,05,774-776. doi: 10.4236/apm.2015.513071
References
















