ABSTRACT

The paper investigates the variation of the spectrum of operators in infinite dimensional Banach spaces. Consider the space of bounded operators on a separable Banach space when equipped with the strong operator topology, and the Polish space of compact subsets of the closed unit disc of the complex plane when equipped with the Hausdorff topology. Then, it is shown that the unit spectrum function is Borel from the space of bounded operators into the Polish space of compact subsets of the closed unit disc. Alternative results are given when other topologies are used.

1. Introduction

Let be an infinite dimensional Banach space. We denote by an arbitrary bounded operator on and by the identity operator on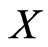. Let be the closed unit disc of the complex plane. The restriction on of the spectrum of an operator, denoted by, is the unit spectrum defined as follows:

Essential spectra of some matrix operators on Banach spaces (see [1]) and spectra of some block operator matrices (see [2]) were investigated, with applications to differential and transport operators. This paper investigates the variations of the unit spectrum as varies over the space of all bounded operator on the Banach space. First, we introduce the sets and the topologies required for this study.

Definition 1

• the set of all compact subsets of the closed unit disc of the complex plane;

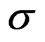• the spectrum function defined from into that maps an operator to its unit spectrum.

The set is endowed with the Hausdorff topology generated by the families of all subsets in one of the following forms

and

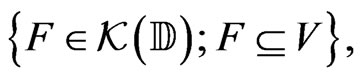
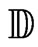for an open subset of. Therefore, 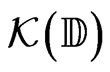is a Polish space, i.e., a separable metrizable complete space, since is Polish (see [3-5]). It is shown below that we can reduce the families that generate the above Hausdorff topology.

Proposition 1 Let be the set of compact subsets of the closed unit disc. Then equipped with the Hausdorff topology is a Polish space; where the Borel structure is generated by one of the following two families

and

Proof 1 Let be an open subset of. There exists a decreasing sequence of open subsets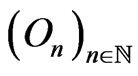; for example

such that

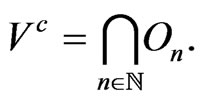
We have

On the other hand,

Indeed, if for all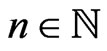, there exists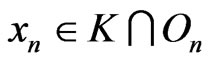, then there exists a subsequence of that converges to, and since is decreasing, we have

2. Norm Operator Topology and the Spectrum Function

We equip 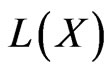with the canonical norm of operators defined by

Note that the map is not continuous when 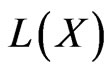is endowed with its canonical norm.

Indeed, the operators 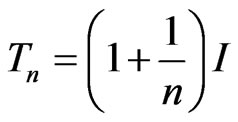converge to the identity while and. However, we have the following result.

Proposition 2 Let X be a Banach space, the space of bounded operators equipped with the norm of operators, and the set of compact subsets of the unit disc 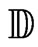equipped with the Hausdorff topology. Then the spectrum map

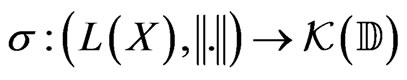
is upper-semi continuous.

Proof 2 Let be an open subset of. By proposition 1, it is only needed to show that the set

is -open in. Let be fixed in. Since, then for all

• The operator is invertible;

• And the map is continuous (see [6]).

It follows that

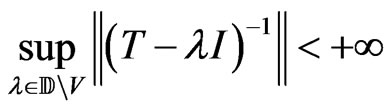
since is compact. Put

Let such that.

For any we have

Thus, is invertible and hence. In other terms, for all with

. Therefore is an open subset of.

3. Strong Operator Topology and the Spectrum Function

Consider now equipped with the strong operator topology (see [6]). In general, equipped with the strong operator topology is not a polish space (since it is not a Baire space). However, if is separable, then is a standard Borel space. Indeed, it is Borel-isomorph to a Borel subset of the Polish space equipped with the norm product topology via the map

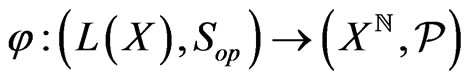
where is a dense -vector space in.

The next result shows how this topology on 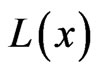affects the spectrum function.

Theorem 1 For any separable infinite dimensional Banach, the map

which maps a bounded operator to its unit spectrum, is Borel when is endowed with the strong operator topology and with the Hausddorf topology.

Proof 3 As is equipped with the Hausdorff topology, it follows from the proposition 1, that it is enough to show that for any open subset of the disc, the following subset is Borel in

Let be a fixed open subset of. We have

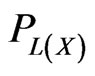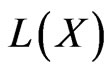where stands for the canonical projection of onto, and

By a descriptive set theory result from ([7]), to show that is a Borel set it suffices to show that is a Borel set with vertical sections.

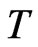For, the vertical section of the set along the direction is given by

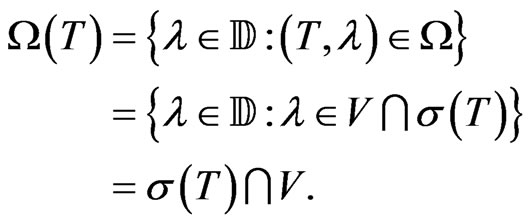
Thus, it is indeed a of.

Now, we need to prove that is a Borel set. Put

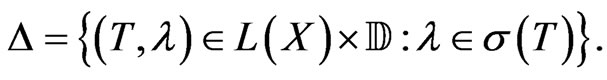
Therefore

Hence, to finish the proof, it is enough to prove the following claim.

Claim: is a Borel set of.

First, note that with

Indeed, if is an isomorphism onto its range, then is a closed subspace that will be strict if, and thus not dense in. On the other hand, since is separable, there exists a countable and dense subset 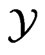in the sphere of, and there exists a dense sequence in.

Now, we will show that and are Borel sets. Let. From the definition of, We have if and only if

In other terms, this is equivalent to

By choosing the subsequence instead of, the previous statement is equivalent to

or again,

Therefore,

with

Since is equipped with the the strong operator convergence, it follows that the sets are open. Hence, is a Borel set.

On the other hand, “is not dense in” is equivalent to

or again,

Therefore

with

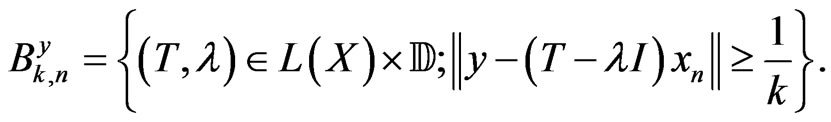
Similarly to, it is not difficult to see that the sets 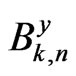are Borel sets. Hence is also a Borel set. This proves the claim and ends the proof of the theorem 1.

4. Conclusions

The variation of the unit spectrum of operators in infinite dimensional Banach spaces is investigated. The unit spectrum of an operator, denoted by, is defined as the restriction on the closed unit disc of the complex plane of the spectrum of given by.

First, the paper presents a simplified characterization of the Borel structure making the set of compact subsets of the closed unit disc a Polish space. It is also shown that for a Banach space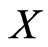, the map 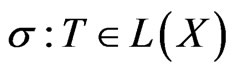that for an operator associates its unit spectrum is upper-semi continuous when is endowed with the norm of operators. On the other hand, when is endowed with the strong operator topology, it is shown that first needed to be a separable infinite dimensional Banach to guarantee a standard Borel structure on, then it is shown that the that the map is Borel in this case. Therefore, this topology is making the spectrum function more rigourous, and as a consequence the variations of the spectrum following changes in an operator or a sequence of operators.

REFERENCES