International Journal of Modern Nonlinear Theory and Application Vol.04 No.01(2015),
Article ID:54274,15 pages
10.4236/ijmnta.2015.41003
Equal Ratio Gain Technique and Its Application in Linear General Integral Control
Baishun Liu
Academy of Naval Submarine, Qingdao, China
Email: baishunliu@163.com
Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 30 September 2014; accepted 13 October 2014; published 27 February 2015
ABSTRACT
In conjunction with linear general integral control, this paper proposes a fire-new control design technique, named Equal ratio gain technique, and then develops two kinds of control design methods, that is, Decomposition and Synthetic methods, for a class of uncertain nonlinear system. By Routh’s stability criterion, we demonstrate that a canonical system matrix can be designed to be always Hurwitz as any row controller gains, or controller and its integrator gains increase with the same ratio. By solving Lyapunov equation, we demonstrate that as any row controller gains, or controller and its integrator gains of a canonical system matrix tend to infinity with the same ratio, if it is always Hurwitz, and then the same row solutions of Lyapunov equation all tend to zero. By Equal ratio gain technique and Lyapunov method, theorems to ensure semi-globally asymptotic stability are established in terms of some bounded information. Moreover, the striking robustness of linear general integral control and PID control is clearly illustrated by Equal ratio gain technique. Theoretical analysis, design example and simulation results showed that Equal ratio gain technique is a powerful tool to solve the control design problem of uncertain nonlinear system.
Keywords:
Equal Ratio Gain Technique, General Integral Control, Nonlinear Control, Robust Control, Output Regulation

1. Introduction
The complexity of nonlinear system challenges us to come up with systematic design methods to meet control objectives and specifications. Faced with such challenge, it is clear that we can not expect a particular method to apply to all nonlinear systems [1] . Therefore, although there were Linearization techniques, Gain scheduling technique, Singular perturbation technique, feedback linearization technique, sliding mode technique and so on, nonlinear design tools, this paper still develops a new control design technique, named Equal ratio gain technique, in conjunction with linear general integral control since integral control plays an irreplaceable role in the control domain.
For general integral control design, there were various design methods, such as general integral control design based on linear system theory, sliding mode technique, Feedback linearization technique and Singular perturbation technique and so on, presented by [2] - [5] , respectively. In addition, general concave integral control [6] , general convex integral control [7] , constructive general bounded integral control [8] and the generalization of the integrator and integral control action [9] were all developed by Lyapunov method. For illustrating the practicability and validity of Equal ratio gain technique and the good robustness of linear general integral control, this paper addresses general integral control design again.
Based on Equal ratio gain technique, this paper develops two kinds of systematic methods to design linear general integral control for a class of uncertain nonlinear system, that is, one is Decomposition method and another is Synthetic method. The main contributions are as follows: 1) a canonical system matrix can be designed to be always Hurwitz as any row controller gains, or controller and its integrator gains increase with the same ratio; 2) as any row controller gains, or controller and its integrator gains of a canonical system matrix tend to infinity with the same ratio, if it is always Hurwitz, and then the same row solutions of Lyapunov equation all tend to zero; 3) theorems to ensure semi-globally asymptotic stability are established in terms of some bounded information. Moreover, the striking robustness of linear general integral control and PID control is clearly illustrated by Equal ratio gain technique. All these mean that Equal ratio gain technique is a powerful tool to solve the control design problem of uncertain nonlinear system, and then makes the engineers more easily design a stable controller. Consequently, Equal ratio gain technique has not only the important theoretical significance but also the broad application prospects.
Throughout this paper, we use the notation
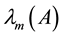 and
and
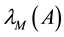 to indicate the smallest and largest eigenvalues, respectively, of a symmetric positive
define bounded matrix
to indicate the smallest and largest eigenvalues, respectively, of a symmetric positive
define bounded matrix ,
for any
,
for any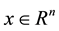 . The
norm of vector
. The
norm of vector
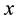 is defined as
is defined as ,
and that of matrix
,
and that of matrix
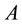 is defined as the corresponding induced norm
is defined as the corresponding induced norm
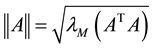 .
.
The remainder of the paper is organized as follows: Section 2 demonstrates Equal ratio gain technique. Section 3 addresses the control design. Example and simulation are provided in Section 4. Conclusions are given in Section 5.
2. Equal Ratio Gain Technique
Consider the following
 system matrix
system matrix ,
,
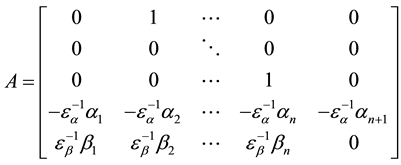
where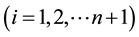
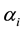 ,
,
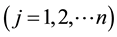
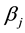 ,
,
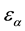 and
and






For developing Equal ration gain technique, firstly, we must ensure that the system
matrix






2.1. Hurwitz Stability
For



Step 1: the polynomial of the system matrix



By Routh’s stability criterion, the gains




Step 2: based on the gains















Step 3: by










The demonstration above is only a basic idea to ensure that the system matrix



Case 1: for

By Routh’s stability criterion, if





holds, and then the polynomial (2) is Hurwitz.
Sub-class 1:




By the inequality (4), obtain,
Sub-class 2:






For this sub-class, there are two kinds of cases:
1) if
2) if
Case 2: for

By Routh’s stability criterion, if







holds, and then the polynomial (6) is Hurwitz.
Sub-class 1:





By the inequality (8), obtain,
Sub-class 2:







For this sub-class, although the situation is complex, a moderate solution can still be obtained, that is,
From the demonstration above, it is obvious that for













Theorem 1: There exist






Discussion 1: From the system matrix




Discussion 2: From the polynomial (1), it is not hard to see that that Hurwitz stability
of the system matrix





Discussion 3: From the statements above, Hurwitz stability condition is more and
more complex as the order of the system matrix



Proposition 1: A canonical system matrix can be designed to be always Hurwitz as any row controller gains, or controller and its integrator gains increase with the same ratio.
2.2. Solution of Lyapunov Equation
By Hurwitz stability condition given by Subsection 2.1, the system matrix







where

The inversion of the system matrix



where the elements


It is well known that the solution







where
and then we have,
Case 1:



It is obvious that



where

Case 2:





It is obvious that



where


From the statements above, it is easy to see that for










by the inversion matrix















Theorem 2: If there exist the gains


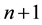



1)


2)


where
Discussion 4: Theorem 1 and 2 are all obtained by multiplying the controller gains






Discussion 5: From the statements above, the solution of the matrix







Proposition 2: As any row controller gains, or controller and its integrator gains of a canonical system matrix tend to infinity with the same ratio, if it is always Hurwitz, and then the same row solutions of Lyapunov equation all tend to zero.
2.3. Example
For testifying the justification of Theorems 1 and 2, and Propositions 1 and 2,
we consider a 6-order two variable system matrix

The polynomial of the system matrix


where
The inversion of the system matrix

By the equation

Thus, taking

and then by Routh’s stability criterion, the array of the coefficients of polynomial is,
where
Now, with the help of computer, we have: 1) if




































Table 1. Numerical Solutions
of




Table 2. Numerical Solutions
of




Table 3. Numerical Solutions
of






Table 4. Numerical Solutions
of





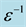
From the example above, it is obvious that: 1) for all the six cases, there all
exists







3. Control Design
Consider the following controllable nonlinear system,

where














Assumption 1: There are two unique control inputs




so that




Assumption 2: No loss of generality, suppose that the functions











for all














For the system (10), we develop two kinds of methods to design linear general integral controllers, respectively, that is, Decomposition method and Synthetic method.
3.1. Decomposition Method
The control laws




where











Thus, substituting (21) and (22) into (10), obtain two augmented systems,


By Assumption 1 and choosing




In the same way, we have,

Thus, we ensure that there are two unique solutions




Substituting (25) into (23) and (26) into (24), and then the whole closed-loop system can be rewritten as,

where






and

Moreover, it is worthy to note that the functions






The matrices








can be obtained. Where



with any given positive define symmetric matrices


Thus, using


where


Now, using the inequalities (15)-(20), obtain,


where



Substituting (31) and (32) into (30), and using



where



By Theorems 1 and 2, and Propositions 1 and 2, obtain,

Therefore, there exist






Using the fact that Lyapunov function






Theorem 3: Under Assumptions 1 and 2, if there exist the gains
















In the same way, for the case of


Theorem 4: Under Assumptions 1 and 2, if there exist the gains












3.2. Synthetic Method
The control laws



where








In the same way as Subsection 3.1, the closed-loop system can be rewritten as,

where
and




Moreover, by the same way as Subsection 3.1, the functions




The matrix






can be obtained. Where



Thus, using
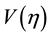

where
and

Now, using the inequalities (15)-(20), obtain,


where



Substituting (38) and (39) into (37), and using



where


By Theorems 1 and 2, and Propositions 1 and 2, obtain,


Thus, there exist



holds for all



Using the fact that Lyapunov function





Theorem 5: Under Assumptions 1 and 2, if there exist the gains











for all for all



In the same way, for the case of


Theorem 6: Under Assumptions 1 and 2, if there exist the gains











Discussion 6: From Decomposition and Synthetic methods above, it is obvious that: 1) although they are developed with two variable systems, it is not hard to extend them to the multiple variable systems; 2) as the subsystems increase, Decomposition method is simpler and more practical than Synthetic method since we can design the controllers for every subsystems, respectively, and then combine them such that the whole closed-loop system is asymptotically stable; 3) for designing a high performance controller, Synthetic method is more excellent than Decomposition method since we can use all the state variables to design the controller and integrator.
Discussion 7: From the procedure of stability analysis above, it is obvious that
so long as the bounded conditions (13)-(20) are satisfied, the asymptotically stable
control can be achieved. This shows that the striking feature of linear general
integral control, that is, its robustness with respect to



Discussion 8: Form all the statements of Sections 3 and 4, it is not hard to see that although Equal ratio gain technique is demonstrated by a class of special system and linear general integral control, its application is not limited in them and can be extend to solve the other relevant problem since Routh’s stability criterion, Lyapunov equation and Lyapunov method are all universal. For examples: 1) if the system is not given in the form (10), one can find a transformation matrix that takes the given system to this form if the system is controllable; 2) by combining Equal ratio gain technique with Feedback linearization technique, we can achieve the design of nonlinear integral controller; 3) as the integrator gains are equal to zero, the control is reduced to proportional control, and the similar conclusions can still be obtained.
Discussion 9: Although the design procedure above looks quite complicated, there need not abstruse theory since Routh’s stability criterion, Lyapunov equation and Lyapunov method are all simple enough to be presented in the text book and practical enough to have been used in the real-word problem. Therefore, Equal ratio gain technique has not only the important theoretical significance but also the broad application prospects.
4. Example and Simulation
Consider the pendulum system [1] described by,
where







and then it can be verified that

Thus, the closed-loop system can be written as,
where


The normal parameters are





Now, if the gains are taken as







holds for all



By solving the Lyapunov equation




Thus, the asymptotical stability of the closed-loop system can be ensured for all


Figure 1 showed the simulation results under normal (solid line) and perturbed (dashed line) cases. The following observations can be made: the system responses under the normal and perturbed cases are almost identical before the additive impulse-like disturbance appears. From the simulation results and design procedure, it is obvious that by Equal ratio gain technique, we can tune a linear general integral controller with good robustness. This demonstrates that not only linear general integral control can effectively deal with the uncertain nonlinear- ity but also Equal ratio gain technique is a powerful tool to solve the control design problem of uncertain nonli-
Figure 1. System output under normal (solid line) and perturbed (dashed line) cases.
near system, and then makes the engineers more easily design a stable controller. Consequently, Equal ratio gain technique has not only the important theoretical significance but also the broad application prospects.
5. Conclusions
In conjunction with linear general integral control, this paper proposes a fire-new control design technique, named Equal ratio gain technique, and then develops two kinds of control design methods, that is, Decomposition and Synthetic methods, for a class of uncertain nonlinear system. The main conclusions are as follows: 1) a canonical system matrix can be designed to be always Hurwitz as any row controller gains, or controller and its integrator gains increase with the same ratio; 2) as any row controller gains, or controller and its integrator gains of a canonical system matrix tend to infinity with the same ratio, if it is always Hurwitz, and then the same row solutions of Lyapunov equation all tend to zero; 3) theorems to ensure semi-globally asymptotic stability are established in terms of some bounded information. Moreover, the striking robustness of linear general integral control and PID control is clearly illustrated by Equal ratio gain technique. All these mean that Equal ratio gain technique is a powerful tool to solve the control design problem of uncertain nonlinear system, and then makes the engineers more easily design a stable controller. Consequently, Equal ratio gain technique has not only the important theoretical significance but also the broad application prospects.
These conclusions above are further confirmed by the design example and simulation results.
References
- Khalil, H.K. (2007) Nonlinear Systems. 3rd Edition, Electronics Industry Publishing, Beijing, 449-453, 551.
- Liu, B.S. and Tian, B.L. (2012) General Integral Control Design Based on Linear System Theory. Proceedings of the 3rd International Conference on Mechanic Automation and Control Engineering, 5, 3174-3177.
- Liu, B.S. and Tian, B.L. (2012) General Integral Control Design Based on Sliding Mode Technique. Proceedings of the 3rd International Conference on Mechanic Automation and Control Engineering, 5, 3178-3181.
- Liu, B.S., Li, J.H. and Luo, X.Q. (2014) General Integral Control Design via Feedback Linearization. Intelligent Control and Automation, 5, 19-23. http://dx.doi.org/10.4236/ica.2014.51003
- Liu, B.S., Luo, X.Q. and Li, J.H. (2014) General Integral Control Design via Singular Perturbation Technique. International Journal of Modern Nonlinear Theory and Application, 3, 173-181. http://dx.doi.org/10.4236/ijmnta.2014.34019
- Liu, B.S., Luo, X.Q. and Li, J.H. (2013) General Concave Integral Control. Intelligent Control and Automation, 4, 356- 361. http://dx.doi.org/10.4236/ica.2013.44042
- Liu, B.S., Luo, X.Q. and Li, J.H. (2014) General Convex Integral Control. International Journal of Automation and Computing, 11, 565-570. http://dx.doi.org/10.1007/s11633-014-0813-6
- Liu, B.S. (2014) Constructive General Bounded Integral Control. Intelligent Control and Automation, 5, 146-155. http://dx.doi.org/10.4236/ica.2014.53017
- Liu, B.S. (2014) On the Generalization of Integrator and Integral Control Action. International Journal of Modern Nonlinear Theory and Application, 3, 44-52. http://dx.doi.org/10.4236/ijmnta.2014.32007
- Gajic, Z. (1995) Lyapunov Matrix Equation in System Stability and Control. Mathematics in Science and Engineering, 195, 30-31.