Journal of Software Engineering and Applications, 2013, 6, 42-47
doi:10.4236/jsea.2013.63b010 Published Online March 2013 (http://www.scirp.org/journal/jsea)
A Novel Model Calculated Distribution Systems Planning
Intergrated Distribution Generators for Competitive
Electricity Markets
V. V. Thang1, N. T. D. Thuy1, D. Q. Thong2, B. Q. Khanh2
1Department of Electric Power Systems, Thainguyen University of Technology (TNUT), Thainguyen, Vietnam; 2Department of
Electric Power Systems, Hanoi University of Science and Technology (HUST), Hanoi, Vietnam.
Received 2013
ABSTRACT
Recently, the distributed generator (DG) has been successfully studied and applied in distribution system at many coun-
tries around the world. Many planning models of the DG integrated distribution system have been proposed. These
models can choose the optimization locations, capacities and technologies of DG with the objective function minimiz-
ing power loss, investment costs or total life cycle costs of the investment project. However, capacity of DG that uses
renewable energy resources is natural variability according to primary energy. This study proposed a planning model of
optimized distribution system that integrates DG in the competitive electricity market. Model can determine equipment
sizing and timeframe requiring for upgrading equipment of distribution system as well as select DG technologies with
power variable constraints of DG. The objective function is minimizing total life cycle cost of the investment project.
The proposed model is calculated and tested for a 48-bus radial distribution system in the GAMS programming lan-
guage.
Keywords: Competitive Electricity Markets (CEM); Distributed Generators (DG); Distribution Systems (DS) Planning
1. Introduction
In the past decade, distribution system planning had ma-
jor changed due to the impact of competitive electricity
market, DG technological development and environ-
mental pollutions. In particular, DG connecting directly
to DS or directly supplying to customers is used as a
popular planning approach. These sources normally use
electric generating technologies such as gas turbines,
combined heat and power, Fuel Cells, solar energy and
wind energies. Therefore, the benefits of DG including
reduction of transmission and distribution cost, power
loss and enhancement of flexibility and reliability of DS,
improvement of differential voltage at nodes as well as
reduction of environmental pollution [1]. However, DG
requires high investment, makes increasing the complex-
ity in measurement and relay protection as well as opera-
tion of DS [2]. Besides, DG using renewable energy re-
sources has the naturally variable power according to
primary energy.
Many planning models of the DG integrated distribu-
tion system are already been researched and proposed.
The authors in [3] presented a long-term DS planning
model in order to determine capacity, location and a new
building investment process or to upgrade current
equipments by using popular mathematical programming.
The objectives of model are the minimum total of in-
vestment and operation costs of DG, the investing cost
for feeder and substation transformers during planning
period. The details of DG technology is not mentioned
because of the assumption that the costing functions and
effects of DG in DS planning are the same, but these are
impossible in reality. Another model in [4] was proposed
with the objective function including the total investing
and operating costs of DG, feeders and substation trans-
formers upgrading costs, energy expenses and minimum
interruptible load costs. In this research, effects of DG
technology are also not mentioned in selecting variables.
The objective function of the two-stage DS planning
model in [5] includes the minimum of total costs for up-
grading feeders, substation transformers and DG con-
struction, energy expenses purchased from market and
environmental pollution costs. Similarly, [6] introduced a
DS planning model determining optimized equipment
sizing and timeframe required for DS upgrading. The
selection issues optimal displacement, sizing, installation
period and technology of DG to meet the demand growth
are presented in [6]. In previous studies, the power of DG

A Novel Model Calculated Distribution Systems Planning Intergrated
Distribution Generators for Competitive Electricity Markets
43
is always assumed to be constant without regarding to the
natural variability of DG capacity which depends on the
primary energy, this is not practical. Therefore, this paper
proposes an optimized DS planning model that integrates
output power characteristics of DG in the CEM.
The next parts of this paper are organized as follows.
Section II introduces a mathematical model with objec-
tive function and constraints. Section III shows calcula-
tion results from the 48-bus DS. Conclusion is presented
in Section IV
2. The Mathematical Model
In competitive electricity market, DS are managed by
distribution companies. These companies can buy elec-
trical energy completely from electricity market or com-
bine with investing DG in order to meet load demands in
future. So, economic and technical indices of planning
project are changed which affects considerably to time,
upgrading capacity of feeders and substations when DG
are chosen in DS planning.
2.1. Objective Function
The objective function of proposed model is to minimize
total life cycle cost of the investment project during cal-
culation period as shown in Equation (1).
,, ,
11
,,,
111
,,, ,,,
1
, ,,,,,,,, ,
1.( .)
(1 )
(.) .
(. ...)
(. .
TNN
FFFC Fijtij
t
tij
NSNDG KDG
SFSC SDGDG
itk ikt
iik
NS SSHQS
PS SS
sPh isthQisth h
ish
QDG PDG DGDG
skh iksthiksth kh
JCCSL
r
CCS CS
kkP kQ
kP Q










 





①
②③
④
11
)
,,, ,,
NDG KDG SSH
iksh
Min
ijNkKDGsSShHt T





 

⑤
(1)
where: Components in  are upgrading costs of feeders
for year t with fixed capital cost (CFF) and variable capi-
tal cost (CFC); Substation transformers upgrading costs in
year t with fixed capital cost (CSF) and variable capital
cost (CSC) in ;  are new investment costs in year t
with technologies k of DG; Electrical energy purchased
cost from electricity market in  and  are fuel, opera-
tion and maintenance costs of DG depending per tech-
nology k, operation season s and time h; 1/(1 )t r cal-
culated total cost at base year with discount rate r.
2.2. The Constraints
1) Contraints for nodal power balance
The output power characteristics of each DG technol-
ogy using renewable energy resources fluctuate by time
of day and season in year so the power of DG is also de-
termined by each hour, season and specially, each tech-
nology k of DG. Therefore, constraint of nodal power
balance of the model proposed in the new conditions is
expressed as shown in(2).
,,,,,,,,,,
1
, ,,,,,,,,,,,,,
1
, ,,,,,,,,,
1
, ,,,,,,,,,,,,,
1
.. .cos()
.. .sin()
KNG DG S
iksth isthisth
k
N
ijti sthjst hijtj st hi sth
j
KNGDG S
iksth isthisth
k
N
ijtisthjsthijtjsth isth
j
PPPD
YU U
QQQD
YU U
 
 







 



,,, ,,ijNkKDGsSSh HtT 

(2)
2) Constraints of DG capacity limit
These constraints allow computed DG capacity at
nodes in limit of DG technology, and it ensures annually
upgrading power corresponding to equipment parameters
as shown in (3).
,, max,,,max,
,,,, 1,,,, 1
0,0
,
1, ,
DG DGDGDG
ikt kiktk
DG DGDGDG
ikt iktiktikt
PP QQ
P
PP QQQ
tiNDG kKDG

 

 
 
(3)
3) Constraints of feeders capacity limits
The feeders upgrading constraints and upgrading
power satisfying equipment parameters are shown in(4).
,,0ij,ij,,,, ,0
, (), ()
1, ,,,,
F
FFFF FF
ij tijts thij tij
SSSmaxSS SS
tijNtTsSShH
 
  (4)
4) Constraints of substation transformers capacity lim-
its
These constraints allow to maximize the use of exist-
ing substation transformers capacity and to satisfy up-
grading power corresponding to equipment parameters
as(5).
,,0, ,,,,,1
., (),
1,, ,,
SSS SSSS
i tptiiti s thi ti t
SkSSmaxS SSS
tiNStTsSShH

 
  (5)
5) Constraints of limited nodal voltage
Technical requirement constraints of limited nodal
voltage are given in equation(6). Voltages at substation
nodes are assumed constantly.
min,,,max
,,,
,,,
,,,
isth
isth
UU UiNLsSStThH
UconstaniNSsSS tThH



(6)
The proposed planning model is a Nonlinear Pro-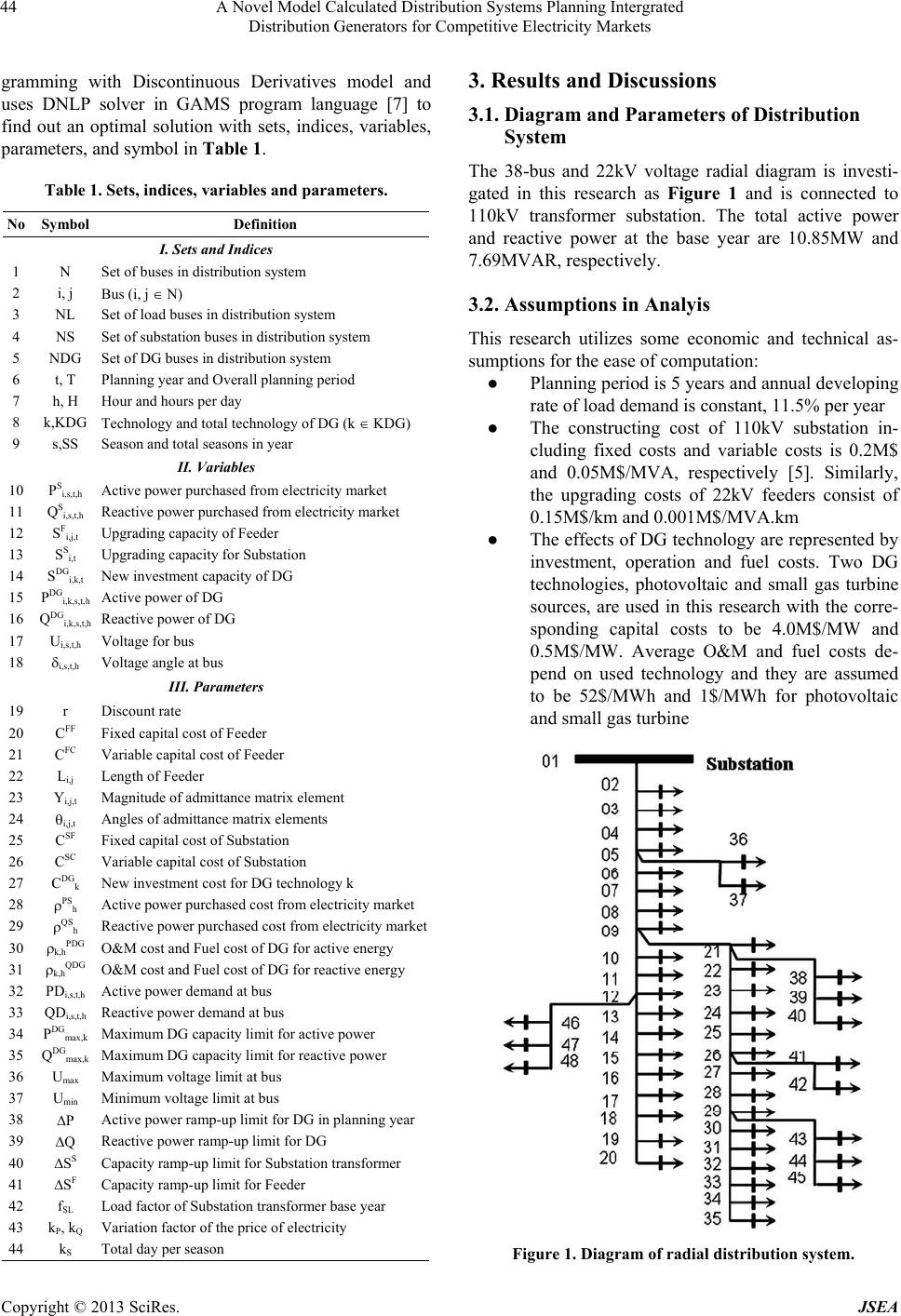

A Novel Model Calculated Distribution Systems Planning Intergrated
Distribution Generators for Competitive Electricity Markets
44
gramming with Discontinuous Derivatives model and
uses DNLP solver in GAMS program language [7] to
find out an optimal solution with sets, indices, variables,
parameters, and symbol in Table 1.
Table 1. Sets, indices, variables and parameter s.
No Symbol Definition
I. Sets and Indices
1 N Set of buses in distribution system
2 i, j Bus (i, j  N)
3 NL Set of load buses in distribution system
4 NS Set of substation buses in distribution system
5 NDG Set of DG buses in distribution system
6 t, T Planning year and Overall planning period
7 h, H Hour and hours per day
8 k,KDG Technology and total technology of DG (k  KDG)
9 s,SS Season and total seasons in year
II. Variables
10 PSi,s,t,h Active power purchased from electricity market
11 QSi,s,t,h Reactive power purchased from electricity market
12 SFi,j,t Upgrading capacity of Feeder
13 SSi,t Upgrading capacity for Substation
14 SDGi,k,t New investment capacity of DG
15 PDGi,k,s,t,h Active power of DG
16 QDGi,k,s,t,h Reactive power of DG
17 Ui,s,t,h Voltage for bus
18 i,s,t,h Voltage angle at bus
III. Parameters
19 r Discount rate
20 CFF Fixed capital cost of Feeder
21 CFC Variable capital cost of Feeder
22 Li,j Length of Feeder
23 Yi,j,t Magnitude of admittance matrix element
24 i,j,t Angles of admittance matrix elements
25 CSF Fixed capital cost of Substation
26 CSC Variable capital cost of Substation
27 CDGk New investment cost for DG technology k
28 PSh Active power purchased cost from electricity market
29 QSh Reactive power purchased cost from electricity market
30 k,hPDG O&M cost and Fuel cost of DG for active energy
31 k,hQDG O&M cost and Fuel cost of DG for reactive energy
32 PDi,s,t,h Active power demand at bus
33 QDi,s,t,h Reactive power demand at bus
34 PDGmax,k Maximum DG capacity limit for active power
35 QDGmax,k Maximum DG capacity limit for reactive power
36 Umax Maximum voltage limit at bus
37 Umin Minimum voltage limit at bus
38 P Active power ramp-up limit for DG in planning year
39 Q Reactive power ramp-up limit for DG
40 SS Capacity ramp-up limit for Substation transformer
41 SF Capacity ramp-up limit for Feeder
42 fSL Load factor of Substation transformer base year
43 kP, kQ Variation factor of the price of electricity
44 kS Total day per season
3. Results and Discussions
3.1. Diagram and Parameters of Distribution
System
The 38-bus and 22kV voltage radial diagram is investi-
gated in this research as Figure 1 and is connected to
110kV transformer substation. The total active power
and reactive power at the base year are 10.85MW and
7.69MVAR, respectively.
3.2. Assumptions in Analyis
This research utilizes some economic and technical as-
sumptions for the ease of computation:
● Planning period is 5 years and annual developing
rate of load demand is constant, 11.5% per year
● The constructing cost of 110kV substation in-
cluding fixed costs and variable costs is 0.2M$
and 0.05M$/MVA, respectively [5]. Similarly,
the upgrading costs of 22kV feeders consist of
0.15M$/km and 0.001M$/MVA.km
● The effects of DG technology are represented by
investment, operation and fuel costs. Two DG
technologies, photovoltaic and small gas turbine
sources, are used in this research with the corre-
sponding capital costs to be 4.0M$/MW and
0.5M$/MW. Average O&M and fuel costs de-
pend on used technology and they are assumed
to be 52$/MWh and 1$/MWh for photovoltaic
and small gas turbine
Figure 1. Diagram of radial distribution system.

A Novel Model Calculated Distribution Systems Planning Intergrated
Distribution Generators for Competitive Electricity Markets
45
● The life of the electrical equipment is usually
large and depends on manufacturing technolo-
gies such as Table 2
 Planning period is 5 years and annual developing
rate of load demand is constant, 11.5% per year
 The constructing cost of 110kV substation in-
cluding fixed costs and variable costs is 0.2M$
and 0.05M$/MVA, respectively [5]. Similarly,
the upgrading costs of 22kV feeders consist of
0.15M$/km and 0.001M$/MVA.km
 The effects of DG technology are represented by
investment, operation and fuel costs. Two DG
technologies, photovoltaic and small gas turbine
sources, are used in this research with the corre-
sponding capital costs to be 4.0M$/MW and
0.5M$/MW. Average O&M and fuel costs de-
pend on used technology and they are assumed to
be 52$/MWh and 1$/MWh for photovoltaic and
small gas turbine
 The life of the electrical equipment is usually
large and depends on manufacturing technologies
such as Table 3.
 DG is manufactured in compact modules occu-
pying small spaces and time to install is short.
Hence, installing areas at load locations are not
limited
 Areas of upgrading of substation transformers
and feeders are not limited
 Constraint of limited load nodes voltage changes
from 0.9pu to 1.1pu, and it should be 1.05pu at
substation node
 Decided variables in the model are continuous in
order to reduce the complexity of the model.
Hence, they should be rounded to match real
equipments.
3.3. The Output Power Characteristics of DG
The output power of PV depends on the intensity of solar
radiation and its performance. The power of 1MWp PV
with 25% performance calculated basing on the given
solar radiation intensity is presented as Figure 2.
Small gas turbines using fuel don’t depend on the na-
ture uncertainty of the primary energy source. Therefore,
the output power characteristics of the DG are not re-
stricted and can be operated at the requirements of load.
3.4. Analysis Results and Disscussions
The feasibility of the proposed model and efficiency of
DG are investigated in two cases. Case A: DG is not
considered when calculating DS planning. Case B: DG is
integrated in the researching model.
The results of calculating showed that case A need to
upgrade substation transformers with a 16MVA capacity.
In contrast, investment to upgrade substation transform-
ers in case B is deferred because of the load demand in-
creasing in the future is provided by DG. Similarly, the
case B’s feeders are not also upgraded during the plan-
ning period. In the case A, 12 feeders need to upgrade in
the time from 3rd year to 5th year as represented in Table 4.
Table 2. Lifespan of equipment.
NoTechnology Lifespan (years)
1 DG (Small gas turbine) 20
2 DG (Photovoltaic - PV) 30
3 Substation and Feeder 20
Table 3. Energy prices p urchase from electricity market.
Time block Base Intermediate Peak
Energy price ($/MWh) 36.35 58.20 105.95
0
0.05
0.1
0.15
0.2
0.25
0.3
0.35
123456789101112131415161718192021222324
hour
The output power of PV (MW)
Winter Summer
Figure 2. The output power characteristics of PV.
Table 4. Feeders Upgrading Decisions.
Feeder section upgrading in each year (mm2)Feeder capacity upgrading in each year (MVA)
Feeder
1 2 3 4 5
Feeder
1 2 3 4 5
Case A
1-2 - - - 23.24- 9-21 - - 10.10 - -
2-3 - - - 23.24- 21-22 - - - 10.10 -
3-4 - - - - 23.2422-23 - - - - 10.10
4-5 - - - - 23.2424-25 - - - - 8.00
5-6 - - - - 19.4326-27 - - - 6.67 -
6-7 - - - - 19.4327-28 - - - - 6.67
7-8 - - - - 19.43
Case B
ij - - - - - ij - - - - -

A Novel Model Calculated Distribution Systems Planning Intergrated
Distribution Generators for Competitive Electricity Markets
46
Table 5. DG Investment Decided.
DG capacity invested in each year (MW)DG capacity invested in each year (MW)
DG technology Bus
1 2 3 4 5
Bus
1 2 3 4 5
Solar PV 34 1.0 - - - - 35 1.0 - - - -
19 - - - - 0.2 33 - - 0.3 - -
20 - - 0.2 0.3 - 34 - 0.1 0.5 - -
31 - - - - 0.2 35 0.1 0.6 - - -
Gas turbine
32 - - - 0.3 - 45 - - 0.3 - -
Total 5.1MW
Table 6. Economic Indices Comparison.
Total life cycle cost (M$)
No Cost
Case A Case B
Comparison cost between Case B and Case A Note
1 Substation Transformer upgrading 0.19 0.00 -0.19
2 Feeder upgrading 0.07 0.00 -0.07
3 O&M and Electrical energy 18.44 17.09 -1.35
4 Investment DG 0.00 1.54 1.54
Total 18.66 18.63 -0.07
Total life
cycle cots is
reduced
-0.37%
Table 5 presents optimal investment decisions of pro-
posed planning model for DG. The total of investment
capacity during planning time is 5.1MW of base year’s
load demands. DG investment focuses mainly on the first
years of planning period and selected location of DG is
far from substation so high economic and technical effi-
ciencies are gained.
Economic indices are compared between case B and
case A as in table VI. As can be seen from the Table 6,
case B holds a better economic index. Cost of DG in-
vestment and equipment upgrading (feeders and substa-
tion) are more expensive than those of case A about
8.23M$ due to a very high cost of DG investment (PV
capital cost is 4.0M$/MW). However, O&M and electric
energy expenses have been decreased by 1.35M$ be-
cause of very low O&M and fuel expenses of DG (PV
has zero cost of fuel). Therefore, the efficiency gets
higher at final years of planning period. Total life cycle
cost of case B is cheaper than these of case A by 0.07M$,
equal to 0.37%.
The technical indicators of DS are also improved when
DG is integrated on DS planning. The power loss at
maximizing load demand times is reduced 4.35% in 5th
planning years so electric energy loss decreased 6,657.6
MWh during planning period. Total of electric energy
purchased from market is also decreased 71,704.25MWh
corresponding to 18,635.93tons are CO2 emission, which
contributes to the decrease of environmental pollution.
The voltage loss on the feeders reduces because of DG
has reduced the transmission capacity from the substation
to the load. Therefore, voltage profiles at the all bus are
also improved during calculation time. In particular, load
node having the biggest support is 35-bus. This bus
voltage profile increased from 0.81pu (case A) to 0.9pu
(case B) at 18th hour in 5th planning year.
4. Conclusion
Recently, the DS planning has been changed signifi-
cantly by the impacts of CEM, DG and environmental
policies. DG has many benefits for DS as enhancement
of flexibility and reliability, bus voltage improvement,
reduction of transmission cost and power loss as well as
reduction of environmental pollution. However, the in-
vestment cost of DG is expensive and DG power that
uses renewable energy resources is natural variability
according to primary energy so the planning and opera-
tion calculation of DS will be more difficult. Therefore,
this study proposed a new optimized DS planning model
that is integrated DG in the CEM. In this model, equip-
ment sizing and timeframe required for upgrading
equipment for DS well as select DG technologies with
power variable constraints of DG can be determined. The
objective function is minimizing total life cycle cost of
the investment project. Calculation results showed that
the proposed model is suitable in large DS planning cal-
culations and the planning together with using DG pro-

A Novel Model Calculated Distribution Systems Planning Intergrated
Distribution Generators for Competitive Electricity Markets
47
vided better economic and technical indicators.
REFERENCES
[1] Thomas Ackermann, Go¨ran Andersson, Lennart So¨der,
“Distributed generation: a definition”, Electric Power
Systems Research 57, 2001
[2] S. Wong, K. Bhattacharya and J.D.Fuller, “Comprehen-
sive framework for long-term distribution system plan-
ning”, Proc. IEEE PES Annual General Meeting, Tampa,
USA, 2007
[3] Algarni, A.A.S.; Bhattacharya, K., “A Novel Approach to
Disco Planning in Electricity Markets: Mathematical
Model”, Power Systems Conference and Exposition,
2009. PSCE '09. IEEE/PES
[4] El-Khattam, W.; Hegazy, Y.; Salama, M., “An integrated
distributed generation optimization model for distribution
system planning”, Power Engineering Society General
Meeting, IEEE, 2005
[5] S. Wong, K. Bhattacharya1and J.D. Fuller, “Electric
power distribution system design and planning in a de-
regulated environment”, IET Generation, Transmission &
Distribution, 2009
[6] V.V.Thang, D.Q.Thong, B.Q.Khanh, “A New Model
Applied to the Planning of Distribution Systems for Com-
petitive Electricity Markets”, The Fourth International
Conference on Electric Utility Deregulation and Restruc-
turing and Power Technologies (DRPT) 2011, Shandong,
China, 2011
[7] Richard E. Rosenthal, “GAMS - A User's Guide”, GAMS
Development Corporation, Washington, USA, 2010.