Journal of Modern Physics, 2012, 3, 1530-1536
http://dx.doi.org/10.4236/jmp.2012.310189 Published Online October 2012 (http://www.SciRP.org/journal/jmp)
Study of Heavy Quarkonium with Energy
Dependent Potential
Pramila Gupta, Indira Mehrotra
Nuclear and Particle Physics Group, Department of Physics, University of Allahabad, Allahabad, India
Received August 10, 2012; revised September 9, 2012; accepted September 17, 2012
ABSTRACT
Heavy quark systems (cc and bb ) have been studied in the nonrelativistic framework using energy dependent inter-
quark potential of the form harmonic oscillator with a small linear term as energy dependent as perturbation plus a in-
verse square potential. This potential admits exact analytical solution of the Schrodinger equation. Mass spectra, lep-
tonic decay width, root mean square radii

2
r, the expectation value of the radius


rand 1r have been
estimated for different quantum mechanical states for cc and bb systems. It is observed that energy dependent term
in the potential leads to saturation of the mass spectra and degree of saturation is governed by the magnitude of pertur-
bation. The calculated values of leptonic decay widths for 1s state are in very good agreement with the experimental
data both for cc and bb systems.
Keywords: Heavy Quark; Mass Spectrum; Energy Dependent Potential
1. Introduction
Energy spectrum of heavy quarkonium are a rich source
of information on the nature of the interquark force at
distances <0.1 fm and >1.0 fm. Many features such as
mass spectra and decay properties of heavy quarkonia
could be described by applying the ordinary nonrelativis-
tic Schrödinger wave equation to the two body quark-
antiquark system. It is well known that the non-relativis-
tic approach is justified when large quark masses are
involved and level spacing between the energy levels is
less than the constituent masses.
A variety of forms for the interquark potential have
been used in heavy quarkonia mass spectroscopy. These
can be broadly classified as 1) QCD motivated potential
[1-5] and 2) purely phenomenological potential [6-9]. A
comprehensive list of potential models is described in the
work of Lichtenberg [10]. All the potentials have almost
similar behavior in the range of 0.1 fm r


0.1 fm,
the characteristic interval of cc and bb systems but
differ from each other outside this range. At present it is
not possible to obtain exact interquark potential in the
entire range of distances from the first principal of
Quantum Chromodynamics. Moreover major shortcom-
ing of the existing potentials is that they have not been
able to account for the observed saturation pattern in the
experimental spectra.
A different category of potentials, which are energy
dependent, have been known in physics for a long time.
They occur in relativistic quantum mechanics at various
places like with Pauli Schrodinger equation [11], in the
Hamiltonian formulation of relativistic many body prob-
lem in covariant formulation with constraints in nonlin-
ear Hamiltonian evolution equation, and also in soliton
propagation. In the non-relativistic physics energy de-
pendent potentials offer the possibility of studying non-
linear effects in the framework of Schrodinger equation.
Lombard [12] have for the first time used an energy
dependent potential to study the bound state properties of
cc and bb systems. Initially they had used one di-
mensional harmonic oscillator potential with a linear
energy dependent term as perturbation to study the effect
of energy dependence on the energy eigenvalues. Later
they solved the problem for three dimensions with dif-
ferent power law potentials (harmonic, linear and Cou-
lomb) with energy dependence. Their main conclusion
has been that energy dependence saturates the mass
spectra. It is well known that any realistic interquark po-
tential has two components: asymptotic and confinement.
However Lombard’s potential has only one component
either confinement (harmonic and linear) or asymptotic
(Coulomb). In view of this in the present work we have
used a more realistic interquark potential of the form
harmonic plus inverse square with small linear energy
C
opyright © 2012 SciRes. JMP

P. GUPTA, I. MEHROTRA 1531
dependence on the confining harmonic oscillator poten-
tial. The choice of linear dependence is motivated by the
fact that it leads to a coherent theory [13]. The combina-
tion of harmonic oscillator and inverse square potential
was first of all adopted by Joshi and Mitra [14] in a the-
ory based on the Schrodinger equation for studying the
heavy meson spectroscopy. Later it has been used by Iyer
et al. [15] and Ryes et al. [16] in the study of hadron
spectroscopy. In all these studies a better fit was obtained
for the potential with a term proportional to 1/r2 because
it has singularity for r  0 that improves the behavior in
this region. Also one great advantage is that our potential
admits exact analytical solution for the radial part of the
Schrodinger equation. This is a great advantage in view
of the high nonlinearity of the differential equation to be
solved. Using this potential we have calculated the mass
spectrum, the root mean square radii, average radii, lep-
tonic decay width and 1r for cc and bb systems.
The latter two properties are sensitive to the asymptotic
part of the potential. The aim is to study the effect of
energy dependence on the low as well as high excitation
states of the system.
2. Details of Calculation
In the present work, energy spectrum of heavy quark-
onium systems (cc and bb ) have been studied in the
framework of non-relativistic Schrödinger equation using
interquark potential as spin independent harmonic oscil-
lator with a small linear term energy dependent plus in-
verse square potential given by
 
22
,,
2
1
,1
2
nl nl
g
VrEm rEr   , (1)
where ω, g and γ are constants.
The three dimensional Schrodinger equation in the
center-of-mass system is

 
2
2
,,, ,,,
,,,
2nlnlmnl nlm
VrEr Er

,,

 


 


,
(2)
where the reduced mass µ in terms of quark mass
and antiquark mass
q
m
q
m is
qq
qq
mm
mm
 .
In natural units is considered. 1c
The wave function is written as
 
,
,, ,
,, ,
nl
nlm lm
ur
rY
r

  
Now putting

22
1E



, 2 tmr


, 2E




and (1ll )2g

  , Equation (2) reduces to


 10
24
ut
tuttu t
t






 

 (3)


ut s are the solution of the radial equation, which are
bounded at infinity and are zero at the origin. As t tends
to , the bounded solution behaves like

exp 2t

and
since t = 0 is a singularity of Equation (3) we seek for a
solution in the form,
 
exp 2l
t
uttRt




 (4)
in which, on account of boundary condition,
 has to be
positive. Substituting Equation (4) into Equation (3) and
taking



2
11218
4lg

   (5)
This leads to the equation
  
11
20
244
ll
tR ttRtR t




 l

 

 (6)
Apart from the constant factor the nonsingular solution
of Equation (6) is the Confluent Hypergeometric series
[17]

11
,2 ;
44 2
l
Rt Ft




 



. (7)


ut increases without bound as t   unless the series
F reduces to a polynomial. This occurs only if
1;0,1,2
44 rr
nn

  , (8)
which implies that the energy eigenvalue
22 222
,
116
88
nl
a
Ea a

 

  (9)
where

2
4221 8an lg

 .
,nl is the classified eigen energy by principal quan-
tum number and angular quantum number ℓ ≤ (n − 1) and
the quark mass is connected to the physical mass as
E
qq() 1q s
2
M
mE

.
The parameters

, g and

are obtained from fit to
the experimental energies of triplet states of 2s and 3s
and center of gravity of 1p with respect to 1S in Equa-
tion (9). In the case of energy independent potential (
E

=
0) the two parameters

and g are obtained from fitting
to the theoretically estimated values of 2s and 1p to the
corresponding experimental data. These in turn are used
to predict eigenvalues of higher excited levels from the
energy eigenvalue Equation (9). All the experimental
data for cc and bb are taken from recent compilation
of Particle Data Group 2008 [18]. In the literature, the
charm quark mass is chosen between 1.2 < mc <1.8 GeV

P. GUPTA, I. MEHROTRA
1532
whereas that of the bottom quark is between 4.5 < mb <
5.4 GeV. In the present work we have chosen the mass to
be 1.5 GeV for the charm quark and 5.0 GeV for the
bottom quark, which is almost at the mid values of above
ranges. While solving non linear energy eigenvalue Equ-
ation (9) only negative roots of

are accepted because
negative values of

can compress the spectrum which
is experimentally observed. Once the parameters of the
potential are fixed it is substituted in the reduced radial
Schrodinger equation given by
of s wave at the origin. Mns is the mass of bound triplet
(vector) state,

is the electromagnetic fine structure
constant and eq the charge of quark in units of the elec-
tron charge


 


,,,
2
1
,
2
nlnlnl nl
ur rE Eur


 


2,Vll
r 
 (10)
This equation is solved numerically in MATHE-
MATICA 8.0 by software program obtained by Lucha et
al. [19] for each quantum state separately. The exact nu-
merical solution of wave functions so obtained are used
to calculate the leptonic decay width, r, 1r and
2
r.
Vector state with spin one, negative parity (3S1) of
quark antiquark pair can annihilate into lepton pair
through single virtual photon. Leptonic decay width of
3S1 states of cc and bb quarkonia is the physical
quantity which is very sensitive to the form of potential.
Leptonic decay widths are calculated according to Van
Royen-Weisskoph formula [20]. This formula is true for
energy dependent potential also.

 


2
22 2
3
1, ns
2
16
π
Γ01 3π
s
q
ns
mq
e
nS eeMqq


 

 


16
(11)
where

0
nS
 is the bound state radial wave function

23 ,13eee e
cb
In the present work we have taken strong coupling
constants as
.


20.37
sc
am  and .

20.26
sb
am 
Expectation values of r, 1/r and bound state root mean
square radii (rms) are obtained from


2
0
2
0
d
d
x
urr
x
urr




, (12)
where x stands for r, 1/r and r2.
3. Results and Discussion
The potential parameters obtained from fit to experimen-
tal energy levels are given for cc and bb systems in
Tables 1 and 2 respectively. These exhibit flavor de-
pendence. Parameters of set A correspond to energy in-
dependent potential and are compared with the parame-
ters obtained by Ryes et al. [16] for harmonic oscillator
plus inverse square potential. Parameters of set B are for
energy dependent potential used in present work. These
are compared with the values obtained by Lombard et al.
[12] for an energy dependent harmonic oscillator poten-
tial. The quark masses used are slightly different in each
work.
The corresponding potentials as a function of distance
are plotted in Figures 1 and 2. The energy dependent per-
turbation leads to slight variation of confinement poten-
tial for different states. When the energy of the state in-
creases with radial excitation, the classically allowed
region for harmonic oscillator potential is greater.
Table 1. Spectroscopic parameters obtained from fit to experimental data for cc system.
Parameters Present work (A) Ref [16] Present work (B) Ref [12]
mq (GeV) 1.50 3.812 1.50 1.207
γ (GeV−1) 0 0 −0.117 −0.433

(GeV) 0.174 0.285 0.203 0.55
g (GeV−1) −0.073 −0.0655 −0.155 0
Table 2. Spectroscopic parameters obtained from fit to experimental data for bb system.
Parameters Present work (A) Ref [16] Present work (B) Ref [12]
mq (GeV) 5.00 7.093 5.00 4.401
γ (GeV−1) 0 0 −0.102 −0.455

(GeV) 0.176 0.2090 0.187 0.530
g (GeV−1) −0.0425 −0.0352 −0.044 0

P. GUPTA, I. MEHROTRA 1533
10
0
10
1
-1
-0.5
0
0.5
1
1.5
2
r (GeV
-1
)
V(r) (GeV)
 = 0
1s
4s
Figure 1. Quark interquark potential curves as a function
of r for energy dependent (1s and 4s states) and energy in-
dependent case (γ = 0) for cc .
10
-1
10
0
10
1
-1
-0.5
0
0.5
1
1.5
2
r (GeV
-1
)
V(r) (GeV)
 = 0
1s
4s
Figure 2. Quark interquark potential curves as a function
of r for energy dependent (1s and 4s states) and energy in-
dependent case (γ = 0) for bb .
The energy spectra of charmonium and bottonium
system with respect to E1s are shown in Figures 3 and 4
respectively. We compare our plotted mass spectra results
with and without energy dependence with experimental
data and also with the work of Lombard et al. [12].
Position of different ns states with respect to 1s is
shown in Table 3. It is observed that the ,1, in-
creases slowly with principal quantum number. This in-
crease is less rapid for energy dependent potential,
showing saturation effect as compared to energy inde-
pendent case (
–
ns s
EE

= 0). The eigenvalues E1ℓ are displayed
up to ℓ = 20 for different values of

in Figure 5 for
the cc system. The interesting feature of the result is
that

determines the level of saturation. On decreasing

, maximum value of eigenvalue E1ℓ decreases and
reaches an upper limit. In contrast for energy independ-
ent case E1ℓ increases regularly towards with increasing ℓ.
Similar plot up to ℓ = 30 is shown for the bb system in
400
600
800
1000
1200
1400
Mass (MeV)
1p 1p 1p
2s 2s 2s 2s
1d
1d 1d 1d
2p 2p 2p 2p
3s 3s 3s
3s
4s
4s
4s
4s
(a) (b) (c) (d)
1p
Figure 3. Mass spectrum of charmonium system with re-
spect to ground state (E1s) with (a) experimental data (b) γ =
0 (c) γ ≠ 0 (d) Lombard’s work. The levels with asterisk are
used as input data in the parameter fitting.
400
600
800
1000
1200
1400
Ma ss (M eV)
(a) (b) (c) (d)
2s 2s 2s
1p 1p
1d
1d 1d
2p 2p 2p
3s 3s 3s
4s
4s 4s
4s
2s
1d
3s
1p
2p
1p
Figure 4. Mass spectrum of bottonium system with respect
to ground state (E1s) with (a) experimental data (b) γ = 0 (c)
γ ≠ 0 (c) Lombard’s work. The levels with asterisk are used
as input data in the parameters fitting.
Table 3. Spacing between the various radial excitations for ℓ
= 0.
cc bb
γ = 0 γ ≠ 0 γ = 0 γ ≠ 0
E4S-E1S (GeV)1.25 1.20 1.26 1.21
E5S-E1S (GeV)1.65 1.61 1.61 1.57
E6S-E1S (GeV)1.98 1.96 1.97 1.95
Figure 6. The saturation in bb is reached more slowly
compared to cc system. We have studied the effect of
varying quark mass on the value of the parameters for
cc and bb systems. It turns out that all the parameters
vary with quark mass. The variation of g with mc and mb
is given in Table 4 keeping
 and ω constant.
Leptonic decay width of vector meson for 1s state and
its ratio with those of other states are listed and also
compared with experimental data in Table 5. Leptonic

P. GUPTA, I. MEHROTRA
1534
05 10 15 20
0
2
4
6
8
10
l
E1l
 = 0
 = -.117
 = -.4
 = -.8
γ = 0
γ = -0.117
γ = -0.4
γ = -0.8
Figure 5. Behavior of spectrum for different values of γ in
cc system.
05 10 15 20 25 30
0
1
2
3
4
5
6
l
E
1l
 = 0
 = -.102
 = -.25
 = -.6
γ = 0
γ = -0.102
γ = -0.25
γ = -0.6
Figure 6. Behavior of spectrum for different values of γ in
bb
system.
Table 4. Values of parameter g for different masses of cc
and bb system.
cc bb
Mass (GeV) g (GeV−1) Mass (GeV) g (GeV−1)
1.2 −0.194 4.4 −0.045
1.5 −0.155 5.0 −0.040
1.7 −0.130 5.5 −0.036
decay width for 1s state obtained in the present work are
very close to experimental data and almost double of the
value of obtained by Lombard. This shows the impor-
tance of using asymptotic term in the potential.
Results for r, 1r and 2
r computed for
different quantum states of cc and bb systems are
listed in Tables 6 and 7. Also given for comparison are
the results of the calculation of Boroum et al. [21] for
r and 1r with global potential and of Chen Hong
et al [22] for root mean square radius with QCD based
potential. Root mean square radii for different 1s state
have slightly smaller values as compared with Lombard
potential reported as 0.49 (for cc ) and 0.26 (for bb ).
Our estimated values fall well in the range of the results
of other calculation.
It is observed that bb system has smaller radii than
the cc system. Root mean square radii of various states
of cc and bb fall within the interval 0.1 to 1 fm be-
cause all the potentials are similar in this range. It means
that average size (radius) of the cc system is greater
Table 5. Ratio of Leptonic decay width of different states with that of 1s state.
cc bb
Calculated value Experimental value [18] Calculated value Experimental value [18]
 (1s) (kev) 5.15 (2.65) 5.55 ± 0.14 1.05 (0.47) 1.32 ± 0.018
 
2s 1s
ee ee
 
 0.61 0.45 ± 0.08 0.68 0.46 ± 0.03
 
3s 1s
ee ee

 0.50 0.16 ± 0.04 0.63 0.33 ± 0.03
 
4s 1s
ee ee
 
 0.43 0.11 ± 0.04 0.57 0.23 ± 0.02
Table 6. r, 1r and 2 r for cc .
r (GeV−1) 1r (GeV) 2 r (fm)
Present work [21] Present work [21] Present work [22]
1s 2.790 2.618 0.507 0.491 0.456 0.401
2s 4.612 4.761 0.396 0.325 0.898 0.801
3s 5.9 0.343 1.252 1.242
1p 4.266 3.751 0.316 0.307 0.698 0.639
2p 5.588 0.239 1.113 1.101

P. GUPTA, I. MEHROTRA 1535
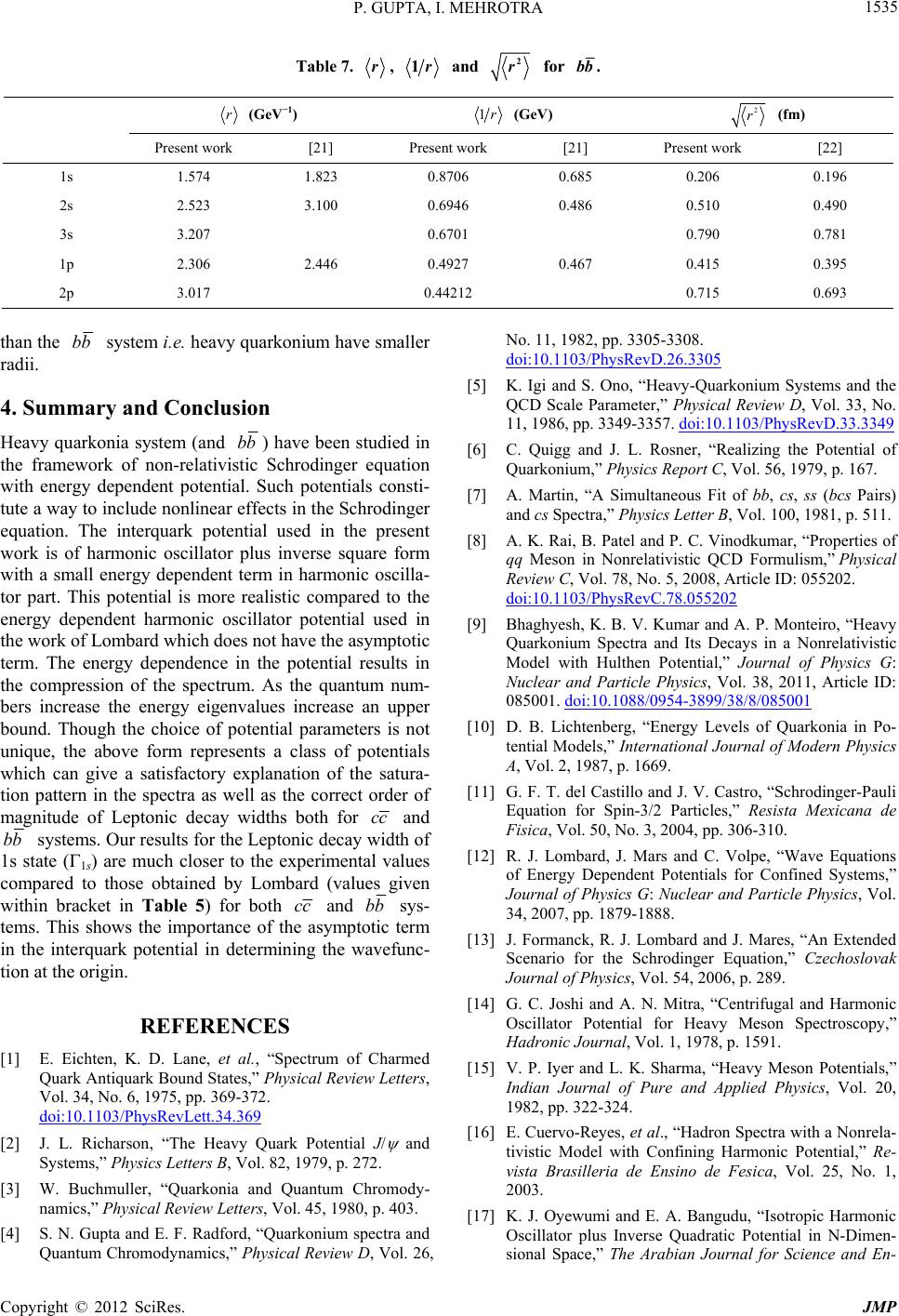
Table 7. r, 1r and 2 r for bb .
r (GeV−1) 1r (GeV) 2
r (fm)
Present work [21] Present work [21] Present work [22]
1s 1.574 1.823 0.8706 0.685 0.206 0.196
2s 2.523 3.100 0.6946 0.486 0.510 0.490
3s 3.207 0.6701 0.790 0.781
1p 2.306 2.446 0.4927 0.467 0.415 0.395
2p 3.017 0.44212 0.715 0.693
than the bb system i.e. heavy quarkonium have smaller
radii.
4. Summary and Conclusion
Heavy quarkonia system (and bb) have been studied in
the framework of non-relativistic Schrodinger equation
with energy dependent potential. Such potentials consti-
tute a way to include nonlinear effects in the Schrodinger
equation. The interquark potential used in the present
work is of harmonic oscillator plus inverse square form
with a small energy dependent term in harmonic oscilla-
tor part. This potential is more realistic compared to the
energy dependent harmonic oscillator potential used in
the work of Lombard which does not have the asymptotic
term. The energy dependence in the potential results in
the compression of the spectrum. As the quantum num-
bers increase the energy eigenvalues increase an upper
bound. Though the choice of potential parameters is not
unique, the above form represents a class of potentials
which can give a satisfactory explanation of the satura-
tion pattern in the spectra as well as the correct order of
magnitude of Leptonic decay widths both for cc and
bb systems. Our results for the Leptonic decay width of
1s state (1s) are much closer to the experimental values
compared to those obtained by Lombard (values given
within bracket in Table 5) for both cc and bb sys-
tems. This shows the importance of the asymptotic term
in the interquark potential in determining the wavefunc-
tion at the origin.
REFERENCES
[1] E. Eichten, K. D. Lane, et al., “Spectrum of Charmed
Quark Antiquark Bound States,” Physical Review Letters,
Vol. 34, No. 6, 1975, pp. 369-372.
doi:10.1103/PhysRevLett.34.369
[2] J. L. Richarson, “The Heavy Quark Potential J/  and
Systems,” Physics Letters B, Vol. 82, 1979, p. 272.
[3] W. Buchmuller, “Quarkonia and Quantum Chromody-
namics,” Physical Review Letters, Vol. 45, 1980, p. 403.
[4] S. N. Gupta and E. F. Radford, “Quarkonium spectra and
Quantum Chromodynamics,” Physical Review D, Vol. 26,
No. 11, 1982, pp. 3305-3308.
doi:10.1103/PhysRevD.26.3305
[5] K. Igi and S. Ono, “Heavy-Quarkonium Systems and the
QCD Scale Parameter,” Physical Review D, Vol. 33, No.
11, 1986, pp. 3349-3357. doi:10.1103/PhysRevD.33.3349
[6] C. Quigg and J. L. Rosner, “Realizing the Potential of
Quarkonium,” Physics Report C, Vol. 56, 1979, p. 167.
[7] A. Martin, “A Simultaneous Fit of bb, cs, ss (bcs Pairs)
and cs Spectra,” Physics Letter B, Vol. 100, 1981, p. 511.
[8] A. K. Rai, B. Patel and P. C. Vinodkumar, “Properties of
qq Meson in Nonrelativistic QCD Formulism,” Physical
Review C, Vol. 78, No. 5, 2008, Article ID: 055202.
doi:10.1103/PhysRevC.78.055202
[9] Bhaghyesh, K. B. V. Kumar and A. P. Monteiro, “Heavy
Quarkonium Spectra and Its Decays in a Nonrelativistic
Model with Hulthen Potential,” Journal of Physics G:
Nuclear and Particle Physics, Vol. 38, 2011, Article ID:
085001. doi:10.1088/0954-3899/38/8/085001
[10] D. B. Lichtenberg, “Energy Levels of Quarkonia in Po-
tential Models,” International Journal of Modern Physics
A, Vol. 2, 1987, p. 1669.
[11] G. F. T. del Castillo and J. V. Castro, “Schrodinger-Pauli
Equation for Spin-3/2 Particles,” Resista Mexicana de
Fisica, Vol. 50, No. 3, 2004, pp. 306-310.
[12] R. J. Lombard, J. Mars and C. Volpe, “Wave Equations
of Energy Dependent Potentials for Confined Systems,”
Journal of Physics G: Nuclear and Particle Physics, Vol.
34, 2007, pp. 1879-1888.
[13] J. Formanck, R. J. Lombard and J. Mares, “An Extended
Scenario for the Schrodinger Equation,” Czechoslovak
Journal of Physics, Vol. 54, 2006, p. 289.
[14] G. C. Joshi and A. N. Mitra, “Centrifugal and Harmonic
Oscillator Potential for Heavy Meson Spectroscopy,”
Hadronic Journal, Vol. 1, 1978, p. 1591.
[15] V. P. Iyer and L. K. Sharma, “Heavy Meson Potentials,”
Indian Journal of Pure and Applied Physics, Vol. 20,
1982, pp. 322-324.
[16] E. Cuervo-Reyes, et al., “Hadron Spectra with a Nonrela-
tivistic Model with Confining Harmonic Potential,” Re-
vista Brasilleria de Ensino de Fesica, Vol. 25, No. 1,
2003.
[17] K. J. Oyewumi and E. A. Bangudu, “Isotropic Harmonic
Oscillator plus Inverse Quadratic Potential in N-Dimen-
sional Space,” The Arabian Journal for Science and En-

P. GUPTA, I. MEHROTRA
1536
gineering, Vol. 28, No. 2A, 2003, p. 173.
[18] C. Ansler, et al., “Particle Data Group,” Physics Letters B,
Vol. 667, 2008, Article ID: 010001. http://pdg.lbl.gov.
[19] F. F. Schoberl and W. Lucha, “Solving the Schroedinger
Equation for Bound States with Mathematica,” Interna-
tional Journal of Modern Physics C, Vol. 10, 1999, pp.
607-620.
[20] R. Van Royen and V. F. Weisskoph, “Vector Meson
Leptonic Decay Width,” Il Nuovo Cimento A, Vol. 50, No.
3, 1967, pp. 617-645. doi:10.1007/BF02823542
[21] G. R. Boroum and H. Abdolmalki, “Variational and Exact
Solutions of the Wavefunction at Origin (WFO) for Hea-
vy Quarkonium by Using a Global Potential,” Physica
Scripta, Vol. 80, 2009, Article ID: 065003.
[22] H. Chen, J. Zhang, Y.-B. Dong and P.-N. Shen, “Heavy
Quarkonium Spectra in a Quark Potential Model,” Chi-
nese Physics Letters, Vol. 18, No. 12, 2001, p. 1558.
doi:10.1088/0256-307X/18/12/305