Applied Mathematics
Vol.05 No.03(2014), Article ID:42946,8 pages
10.4236/am.2014.53054
L∞-Error Estimate of Schwarz Algorithm for Noncoercive Variational Inequalities
Samira Saadi1, Allaoua Mehri2
1Laboratory LANOS, Department of Mathematics, Badji Mokhtar University, Annaba, Algeria
2Laboratory LAIG, Department of Mathematics, Guelma University, Guelma, Algeria
Email: saadisamira69@yahoo.fr, allaoua.mehri@yahoo.fr
Copyright © 2014 Samira Saadi, Allaoua Mehri. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. In accordance of the Creative Commons Attribution License all Copyrights © 2014 are reserved for SCIRP and the owner of the intellectual property Samira Saadi, Allaoua Mehri. All Copyright © 2014 are guarded by law and by SCIRP as a guardian.
ABSTRACT
Received December 20, 2013; revised January 20, 2014; accepted January 27, 2014
The Schwarz method for a class of elliptic variational inequalities with noncoercive operator was studied in this work. The author proved the error estimate in L∞-norm for two domains with overlapping nonmatching grids using the geometrical convergence of solutions and the uniform convergence of subsolutions.
Keywords:
Variational Inequalities; Schwarz Method; Subsolutions; L∞-Error Estimates
1. Introduction
More than one hundred years ago, Schwarz algorithms were proposed for proving the solvability of PDEs on a complicated domain. With parallel calculators, this rediscovery of these methods as algorithms of calculations was based on a modern variational approach. Pierre-Louis Lions was the starting point of an intense research activity to develop this tool of calculation, see, e.g., [1,2] and the references therein [3-9].
In this paper, we give a new approach to the finite element approximation for the problem of variational in- equality with noncoercive operator. This problem arises in stochastic control (see [10]). We consider a domain which is the union of two overlapping sub-domains where each sub-domain has its own generated triangulation. To prove the main result of this work, we construct two sequences of subsolutions and we estimate the errors between Schwarz iterates and the subsolutions. The proof stands on a Lipschitz continuous dependency with respect to the source term for variational inequality, while in [5] the proof stands on a Lipschitz continuous dependency with respect to the boundary condition.
The paper is organized as follows. In Sections 2, we introduce the continuous and discrete obstacle problem as well as Schwarz algorithm with two sub-domains and give the geometrical convergence theorem. In Section 3, we establish two sequences of subsolutions and their error estimates and prove a main result concerning the error estimate of solution in the 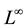 -norm, taking into account the combination of geometrical convergence and uniform convergence [11,12] of finite element approximation.
-norm, taking into account the combination of geometrical convergence and uniform convergence [11,12] of finite element approximation.
2. Schwarz Algorithm for Variational Inequalities with Noncoercive Operator
2.1. Notations and Assumptions
Let’s consider functions
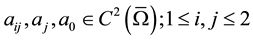 (1)
(1)
such that
 (2)
(2)
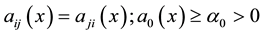 (3)
(3)
where 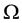 is a connected bounded domain in
is a connected bounded domain in  with sufficiently regular boundary
with sufficiently regular boundary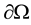 .
.
We define a second order differential operator
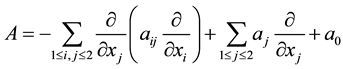 (4)
(4)
where the bilinear form associated: 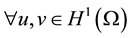
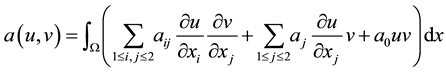 (5)
(5)
Let 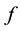 be a function in
be a function in
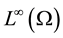 (6)
(6)
an obstacle
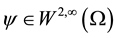 (7)
(7)
a regular function 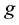 defined on
defined on 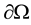 such that
such that
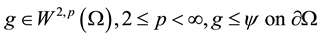 (8)
(8)
AM and a nonempty convex set
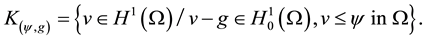 (9)
(9)
We assume there exists 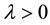 large enough and a constant
large enough and a constant  such that
such that
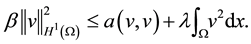 (10)
(10)
Putting

then the bilinear form 
Let 

which is equivalent to


We define 

where 


Remark 1. We call quasi-variational inequality (Q.V.I) if the right hand side 

2.2. Some Preliminary Results on the V.I Noncoercive
Thanks to [10], the problem (12) has one and only one solution, moreover 
We give a monotonicity property of the solution with respect to both the source term, the boundary condition and the obstacle. Let 

Lemma 1 [10] Under the preceding notations and assumptions (1) to (11), if 


Let 


that is equivalent to
Lemma 2 [10] Under the preceding notations and assumptions (1) to (11), the solution 

We show the Lipschitz property, which gives the continuous dependance to the data
Lemma 3 Under the preceding notations and assumptions (1) to (11), we have
where 
Proof Firstly, let
we have
then
and
if we put
then
therefore
Secondly, it is clear that
and
so, due to lemma 1, we get
which gives
by changing the roles of 

which completes the proof.
Remark 2 If 

Let 










where


We shall assume that the matrix 

is 


2.3. The Continuous Schwarz Algorithm
Consider the model obstacle problem: find 

where 

We decompose 


and 
we denote 






For 
We associate with problem (19) the following system: find 

where
Starting from 




and 



where
The following geometrical convergence is due to ([2], pages 51-63)
Theorem 1 The sequences 


2.4. The Discretization
For








where 

Starting from 




and on 


We will also always assume that the respective matrices resulting from problems (23) and (24) are 
3. Error Analysis
This section is devoted to the proof of the main result of this work. For that, we begin by introducing two auxi- liary sequences.
3.1. Auxiliary Schwarz Sequences
To simplify the notation, we take
Let 

where 



where 

It is clear that 




similarly, we have

3.2. Sequences of Sub-Solutions
The following theorems will play a important role in proving the main result of this paper.
3.2.1. Part One―Discrete Sub-Solution
We construct a discrete function 

Theorem 2 Let 




Proof Let us give the proof for




then
so, due to lemma 2 (discrete case), it follows that

where
setting 

which combined with (29) yields
Thus, we choose
then
and
3.2.2. Part Two―Continuous Sub-Solution
We construct a continuous function 

Theorem 3 Let 




Proof Let us give the proof for



then
so, making use of lemma 2, we obtain

where
Setting 

so, combining (31) with estimate (32) yields
Finally, choosing
we get immediately the results.
3.3. L∞-Error Estimate
Theorem 4 





Proof Thanks to theorem 2 and theorem 3, we have
therefore

moreover
let 
we choose 
then
and by inverse inequality, we get
4. Conclusion
We have established a convergence order of Schwarz algorithm for two overlapping subdomains with non- matching grids. This approach developed in this paper relies on the geometrical convergence and the error estimate between the continuous and discrete Schwarz iterates. The constant c in error estimate is independent of Schwarz iterate n.
References
- P. L. Lions, “On the Schwarz Alternating Method. I,” 1st International Symposium on Domain Decomposition Methods for Partial Differential Equations, SIAM, Philadelphia, 1988, pp. 1-42.
- P. L. Lions, “On the Schwarz Alternating Method. II, Stochastic Interpretation and Order Proprieties,” In: T. F. Chan, et al., Eds., Domain Decomposition Methods, SIAM, Philadelphia, 1989, pp. 47-70.
- L. Badea, “On the Schwarz Alternating Method with More than Subdomains for Nonlinear Monotone Problems,” SIAM Jour- nal of Numerical Analysis, Vol. 28, 1991, pp. 197-204.
- L. Badea, X. Cheng and J. Wang, “Convergence Rate Analysis of a Multiplicative Schwarz Method for Variational Inequalities,” SIAM Journal of Numerical Analysis, Vol. 41, No. 3, 2003, pp. 1052-1073. http://dx.doi.org/10.1137/S0036142901393607
- M. Boulbrachene and S. Saadi, “Maximum Norm Analysis of an Overlapping Nonmatching Grids Method for the Obstacle Problem,” Hindawi Publishing Corporation, Cairo, 2006, pp. 1-10.
- M. Haiour and E. Hadidi, “Uniform Convergence of Schwarz Method for Noncoercive Variational Inequalities,” International Journal of Contemporary Mathematical Sciences, Vol. 4, No. 29, 2009, pp. 1423-1434.
- M. Haiour and S. Boulaares, “Overlapping Domain Decomposition Methods for Elliptic Quasi-Variational Inequalities Related to Impulse Control Problem with Mixed Boundary Conditions,” Proceedings of Indian Academy of Sciences (Mathematical Sciences), Vol. 121, No. 4, 2011, pp. 481-493.
- J. Zeng and S. Zhou, “On Monotone and Geometric Convergence of Schwarz Methods for Two-Sided Obstacle Problems,” SIAM, Journal on Numerical Analysis, Vol. 35, No. 2, 1998, pp. 600-616. http://dx.doi.org/10.1137/S0036142995288920
- J. Zeng and S. Zhou, “Schwarz Algorithm of the Solution of Variational Inequalities with Nonlinear Source Terms,” Applied Mathematics and Computations, Vol. 97, 1998, pp. 23-35.
- A. Bensoussan and J. L. Lions, “Applications of Variational Inequalities in Stochastic Control (English Version),” North-Holland Publishing Company, Amsterdam, 1982.
- M. Boulbrachene, “The Noncoercive Quasi-Variational Inequalities Related to Impulse Control Problems,” Computers & Mathematics with Applications, Vol. 35, No. 12, 1998, pp. 101-108. http://dx.doi.org/10.1016/S0898-1221(98)00100-X
- P. Cortey-Dumont, “On Finite Element Approximation in the L∞-Norm of Variational Inequalities,” Numerische Mathematik, Vol. 47, No. 1, 1985, pp. 45-57. http://dx.doi.org/10.1007/BF01389875
- P. G. Ciarlet and P. A. Raviart, “Maximum Principle and Uniform Convergence for the Finite Element Method,” Computer Methods in Applied Mechanics and Engineering, Vol. 2, 1973, pp. 1-20.
- P. Cortey-Dumont, “Sur les Inéquations Variationnelles à Opérateur non Coercif,” Rairo, Modélisation Mathématique Et Analyse Numérique, Vol. T.19, No. 2, 1985, pp. 195-212. http://www.numdam.org/numdam-bin/feuilleter?id=M2AN_1985__19_2
























































