Applied Mathematics
Vol. 4 No. 2 (2013) , Article ID: 28217 , 9 pages DOI:10.4236/am.2013.42058
Logarithmic Sine and Cosine Transforms and Their Applications to Boundary-Value Problems Connected with Sectionally-Harmonic Functions
Engineering Faculty, OKAN University, Istanbul, Turkey
Email: midemen@gmail.com
Received September 30, 2012; revised January 8, 2013; accepted January 15, 2013
Keywords: Integral Transforms; Harmonic Functions; Wedge Problems; Boundary-Value Problems; Logarithmic Sine Transform; Logarithmic Cosine Transform
ABSTRACT
Let  stand for the polar coordinates in
stand for the polar coordinates in ,
,  be a given constant while
be a given constant while  satisfies the Laplace equation
satisfies the Laplace equation  in the wedge-shaped domain
in the wedge-shaped domain  or
or  . Here
. Here 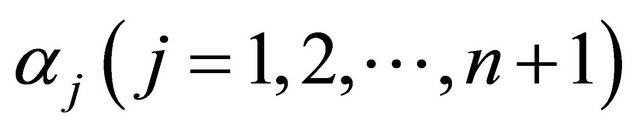 denote certain angles such that
denote certain angles such that . It is known that if
. It is known that if  satisfies homogeneous boundary conditions on all boundary lines
satisfies homogeneous boundary conditions on all boundary lines  in addition to non-homogeneous ones on the circular boundary
in addition to non-homogeneous ones on the circular boundary![]() , then an explicit expression of
, then an explicit expression of  in terms of eigen-functions can be found through the classical method of separation of variables. But when the boundary condition given on the circular boundary
in terms of eigen-functions can be found through the classical method of separation of variables. But when the boundary condition given on the circular boundary ![]() is homogeneous, it is not possible to define a discrete set of eigen-functions. In this paper one shows that if the homogeneous condition in question is of the Dirichlet (or Neumann) type, then the logarithmic sine transform (or logarithmic cosine transform) defined by
is homogeneous, it is not possible to define a discrete set of eigen-functions. In this paper one shows that if the homogeneous condition in question is of the Dirichlet (or Neumann) type, then the logarithmic sine transform (or logarithmic cosine transform) defined by  (or
(or ) may be effective in solving the problem. The inverses of these transformations are expressed through the same kernels on
) may be effective in solving the problem. The inverses of these transformations are expressed through the same kernels on  or
or . Some properties of these transforms are also given in four theorems. An illustrative example, connected with the heat transfer in a two-part wedge domain, shows their effectiveness in getting exact solution. In the example in question the lateral boundaries are assumed to be non-conducting, which are expressed through Neumann type boundary conditions. The application of the method gives also the necessary condition for the solvability of the problem (the already known existence condition!). This kind of problems arise in various domain of applications such as electrostatics, magneto-statics, hydrostatics, heat transfer, mass transfer, acoustics, elasticity, etc.
. Some properties of these transforms are also given in four theorems. An illustrative example, connected with the heat transfer in a two-part wedge domain, shows their effectiveness in getting exact solution. In the example in question the lateral boundaries are assumed to be non-conducting, which are expressed through Neumann type boundary conditions. The application of the method gives also the necessary condition for the solvability of the problem (the already known existence condition!). This kind of problems arise in various domain of applications such as electrostatics, magneto-statics, hydrostatics, heat transfer, mass transfer, acoustics, elasticity, etc.
1. Introduction
Boundary-value problems connected with sectionallyharmonic functions in wedge-shaped domains

and
 where
where  stand for the polar coordinates in R2 while a > 0 is a given constant, are important from both pure scientific and engineering points of view. Figure 1 epitomizes a simple case of D0 which corresponds to n = 2. The sub-regions determined by
stand for the polar coordinates in R2 while a > 0 is a given constant, are important from both pure scientific and engineering points of view. Figure 1 epitomizes a simple case of D0 which corresponds to n = 2. The sub-regions determined by  and
and  model the regions filled with different materials having different constitutive parameters. The field function
model the regions filled with different materials having different constitutive parameters. The field function  satisfies the basic equation
satisfies the basic equation
 (1a)
(1a)

Figure 1. A composite region D0 filled with two different materials.
in the sense of distribution under the boundary conditions

and

shown on the figure. Here 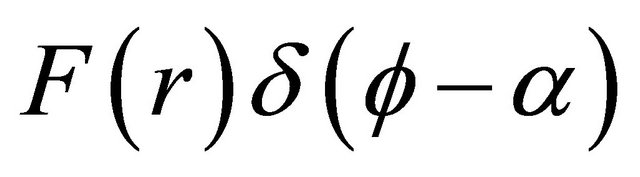 stands for the density of the exciting sources concentrated on the interface
stands for the density of the exciting sources concentrated on the interface  (if any) while
(if any) while  and
and  are given linear (differential) boundary operations. The boundary conditions in question may also involve certain terms representing the sources localized on the boundary (if any). As to the function
are given linear (differential) boundary operations. The boundary conditions in question may also involve certain terms representing the sources localized on the boundary (if any). As to the function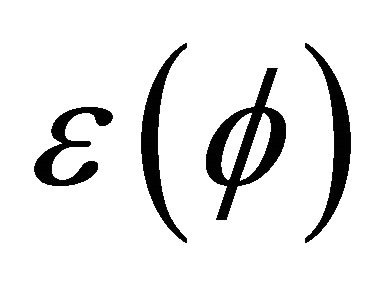 , it has constant values e1 and e2 in the sub-regions in question. Thus on the interface between the sub-regions two transmission conditions of the following forms are satisfied:
, it has constant values e1 and e2 in the sub-regions in question. Thus on the interface between the sub-regions two transmission conditions of the following forms are satisfied:
 (1b)
(1b)
 (1c)
(1c)
As is well-known, when , one can define a set of orthogonal eigen-functions which permit us to obtain an explicit expression of
, one can define a set of orthogonal eigen-functions which permit us to obtain an explicit expression of  in terms of these eigen-functions. The coefficients in the eigen-function series are determined by using the nonhomogeneous boundary condition given on the boundary r = a (i.e. through
in terms of these eigen-functions. The coefficients in the eigen-function series are determined by using the nonhomogeneous boundary condition given on the boundary r = a (i.e. through ) together with the regularity condition to be stated at r = 0. When
) together with the regularity condition to be stated at r = 0. When , at least one of the functions
, at least one of the functions  and
and 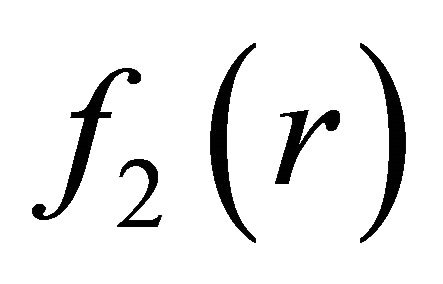 must be different from zero in order to have a non trivial solution
must be different from zero in order to have a non trivial solution . In this case it is not possible to define a set of discrete eigen-functions. To overcome such kind of a difficulty, in the midst of the last century some methods, which are effective when the region consists of D0, were proposed. Among them we can mention, for example, the finite Sturm-Liouville transforms introduced by Eringen [1] and Churchill [2], and the finite Mellin transform introduced by Naylor [3,4] (see also [5]). The finite Sturm-Liouville transforms are not appropriate in the case considered here because they are based on the set of eigen-functions which can not be defined in the present case. As to the finite Mellin transforms, they are defined as follows (see, for ex., [5, pp. 462-467]):
. In this case it is not possible to define a set of discrete eigen-functions. To overcome such kind of a difficulty, in the midst of the last century some methods, which are effective when the region consists of D0, were proposed. Among them we can mention, for example, the finite Sturm-Liouville transforms introduced by Eringen [1] and Churchill [2], and the finite Mellin transform introduced by Naylor [3,4] (see also [5]). The finite Sturm-Liouville transforms are not appropriate in the case considered here because they are based on the set of eigen-functions which can not be defined in the present case. As to the finite Mellin transforms, they are defined as follows (see, for ex., [5, pp. 462-467]):
 (2a)
(2a)
 (2b)
(2b)
One can easily check that the first or the second transform is appropriate to reduce the Laplace equation written in the circular polar coordinates to an ordinary differential equation when Dirichlet or Neumann type conditions are prescribed, respectively, on the circular part r = a of the boundary. The inverse transforms consist then of the classical Mellin type integrals.
The aim of this note is to show that the transforms of the forms
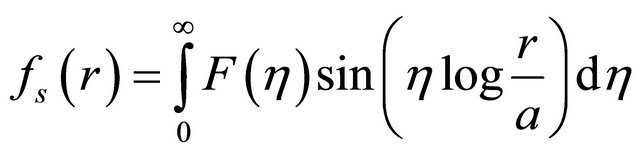 (3a)
(3a)
and
 (3b)
(3b)
are effective in getting explicit expressions to the solutions of the problems connected with sectionally-harmonic functions defined in D0 and D¥ mentioned above when the boundary condition on the arc r = a is homogeneous and of the Dirichlet or Neumann type. The simplicity of these transforms is that their inverses are also given with the same kernel (see Section 2B below). To clarify the essential properties of the representations ((3a), (b)), in what follows we will consider, without loss of generality, the case where n = 1 (see Figures 2 and 3).
To the best of our knowledge a representation of the form (3b) was first considered by Smythe (see [6, pp. 71-72]) to find the electrostatic potential due to a line source located parallel to a dielectric wedge for which . His discussion is based on moot physical arguments and some particular restrictions. As we will show later on, a representation of the form (3b) is not suitable when
. His discussion is based on moot physical arguments and some particular restrictions. As we will show later on, a representation of the form (3b) is not suitable when  because only the data known for
because only the data known for  or
or  is sufficient to uniquely
is sufficient to uniquely

Figure 2. A wedge-shaped region involving only the singular point r = 0.
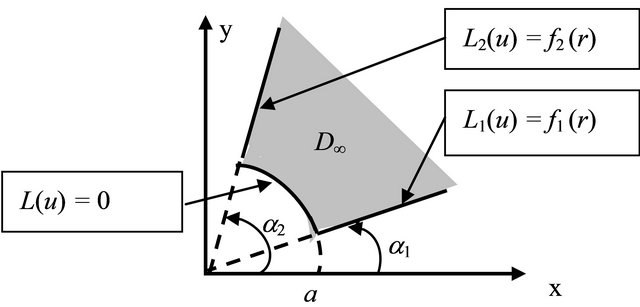
Figure 3. A wedge-shaped region involving only the singular point r = ¥.
determine  (i.e. the inverse transform). Furthermore, when (3b) is used to express
(i.e. the inverse transform). Furthermore, when (3b) is used to express  for all
for all , it gives
, it gives , which is not acceptable from physics point of view.
, which is not acceptable from physics point of view.
2. Logarithmic Sine and Cosine Transforms
Let a > 0 be a given constant while  is a given function. Then consider the functions
is a given function. Then consider the functions  and
and  defined through the convergent integrals taking place in (3a) and (3b). There log stands for the principal branch of the logarithm function. We will refer
defined through the convergent integrals taking place in (3a) and (3b). There log stands for the principal branch of the logarithm function. We will refer 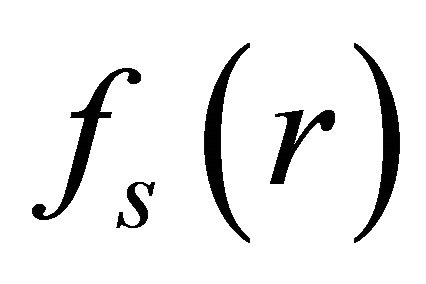 and
and  to as the logarithmic sine transform and logarithmic cosine transform of
to as the logarithmic sine transform and logarithmic cosine transform of , respectively. In what follows we will also denote them by the symbols
, respectively. In what follows we will also denote them by the symbols  and
and . Some interesting and important properties of these transforms are stated in the theorems given below.
. Some interesting and important properties of these transforms are stated in the theorems given below.
A) Limit Values for r ( 0, r ( a and r ( (
As we will see later on (see Section 2B), the expression of the function  (or
(or ) known only in the interval
) known only in the interval  or
or  is sufficient to determine the function
is sufficient to determine the function  uniquely. The functions
uniquely. The functions  and
and  may be piece-wise continuous in these intervals. That means that the limit values of the integrals taking place in (3a) and (3b) as r tends to the end point
may be piece-wise continuous in these intervals. That means that the limit values of the integrals taking place in (3a) and (3b) as r tends to the end point 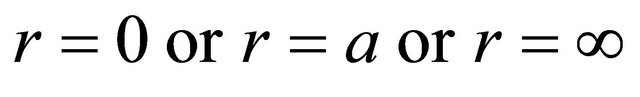 may be different from the values obtained by replacing directly
may be different from the values obtained by replacing directly  in those integrals. From application point of view it is the limiting values that are important. Therefore these limit values must be discussed carefully. The two theorems that follow concern this point (for their proof see Appendix).
in those integrals. From application point of view it is the limiting values that are important. Therefore these limit values must be discussed carefully. The two theorems that follow concern this point (for their proof see Appendix).
Theorem-1. If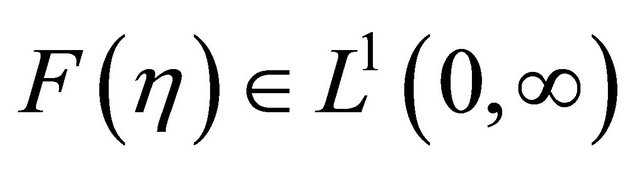 , then from (3a) one gets
, then from (3a) one gets
 (4a)
(4a)
Theorem-2. a) If , then from (3b) one gets
, then from (3b) one gets
 (4b)
(4b)
b) If one has also , then
, then
![]() (4c)
(4c)
B) Inverse Transforms
It is an interesting fact that when  (or
(or ) is known for all
) is known for all  or for all
or for all , then the function
, then the function  can be determined completely. The theorems that follow concern this inversion problem (for their proof see Appendix). Notice that when
can be determined completely. The theorems that follow concern this inversion problem (for their proof see Appendix). Notice that when  is piece-wise continuous, in what follows
is piece-wise continuous, in what follows  means
means 
Theorem-3. a) Let  be piece-wise continuous in the interval
be piece-wise continuous in the interval  and
and 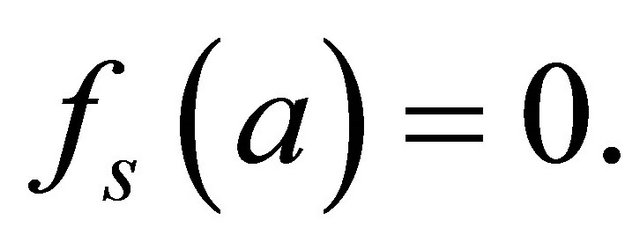 Then (3a) yields
Then (3a) yields
 (5a)
(5a)
b) If 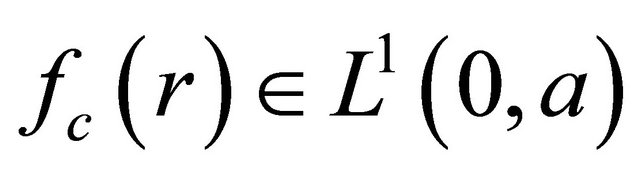 is piece-wise continuous in the interval
is piece-wise continuous in the interval , then (3b) yields
, then (3b) yields
 (5b)
(5b)
Theorem-4. a) Let  be piece-wise continuous for
be piece-wise continuous for  and
and . Then (3a) yields
. Then (3a) yields
 (6a)
(6a)
b) If  is piece-wise continuous for
is piece-wise continuous for , then from (3b) one gets
, then from (3b) one gets
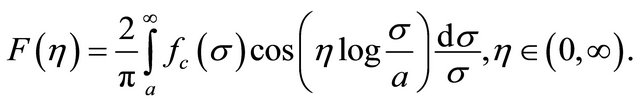 (6b)
(6b)
The proof of these theorems (except Theorem 1) can easily be achieved by using the already known ones through simple transformations (See for example [5] or [7]). For the sake of fluency of the paper, we prefer to postpone the proofs to the Appendix. In what follows we will denote the inverse transforms given by (5a) and (6a) by . Similarly, the inverse transforms given by (5b) and (6b) will be denoted by
. Similarly, the inverse transforms given by (5b) and (6b) will be denoted by .
.
3. Applications to Boundary-Value Problems Connected with Sectionally-Harmonic Functions
When one has also , by successive differentiations of (3a) and (3b) one gets
, by successive differentiations of (3a) and (3b) one gets
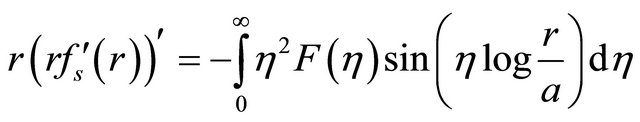 (7a)
(7a)
and
 , (7b)
, (7b)
which show that
 (8a)
(8a)
and
 . (8b)
. (8b)
Now consider a function  which is harmonic in
which is harmonic in

or

and satisfies a homogeneous boundary condition of the Dirichlet or Neumann type on the circular part of the boundary, namely:
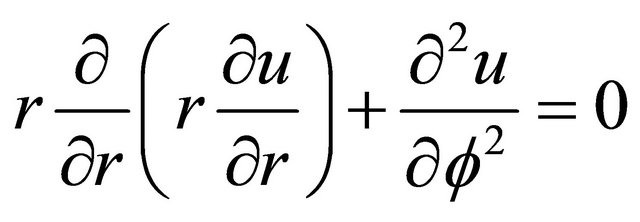 (9)
(9)
and
 (10a)
(10a)
or
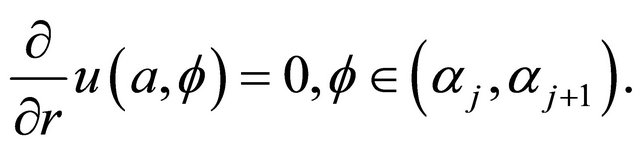 (10b)
(10b)
Application of the operator  or
or  to (9) yields
to (9) yields
 (11)
(11)
((8a), (b)) being taken into account. Here  stands for the logarithmic sine or cosine transform of
stands for the logarithmic sine or cosine transform of . From (11) one gets
. From (11) one gets
 (12)
(12)
where  and
and  are the integration constants to be determined through the boundary and transmission conditions while
are the integration constants to be determined through the boundary and transmission conditions while  and
and 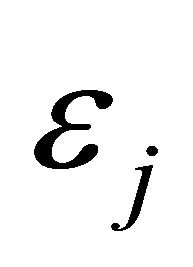 are two constants which can be chosen appropriately to facilitate the computation. They may also be dependent on h. Thus, in the sector
are two constants which can be chosen appropriately to facilitate the computation. They may also be dependent on h. Thus, in the sector
 one has the following expressions
one has the following expressions
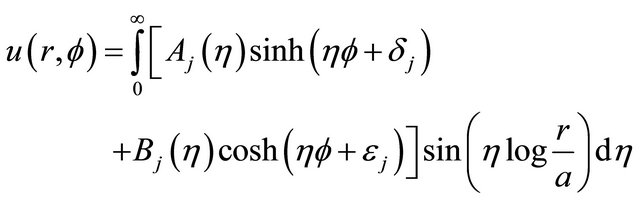 (13a)
(13a)
or
 . (13b)
. (13b)
(13a) is valid for the condition (10a) while (13b) is valid for the case of (10b).
4. An Illustrative Application
To show the effectiveness of the representations ((13a), (b)), in what follows we will give an illustrative example which concerns the heat conduction in a two-part composite region shown in Figure 4. A point source of amount Q exists at the point (b, 0) while the circular part of the boundary is coated by an insulating material. The physical properties of the lateral boundaries (i.e. the boundary conditions on  and
and ) will be defined later on. Thus the field function (temperature)
) will be defined later on. Thus the field function (temperature)  satisfies the following field equation under the given boundary conditions:
satisfies the following field equation under the given boundary conditions:
 (14)
(14)
 (15a)
(15a)
 (15b)
(15b)
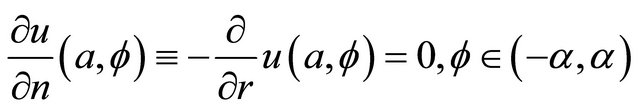 (15c)
(15c)
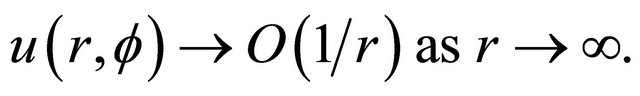 (15d)
(15d)
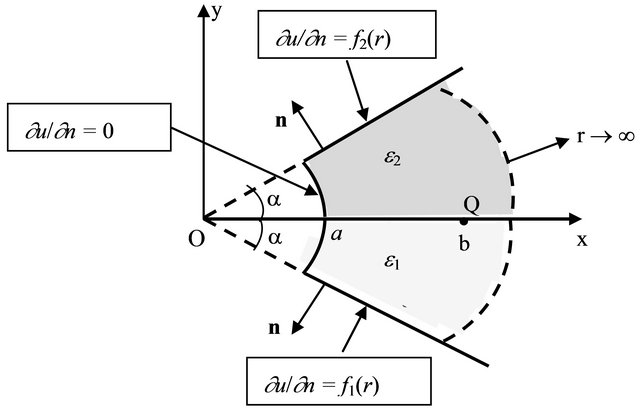
Figure 4. A wedge-shaped region with Neumann type boundary conditions.
Here  has constant values e1 and e2 in the indicated sub-regions.
has constant values e1 and e2 in the indicated sub-regions.
Remark that the problem posed by (14)-(15d) has a solution if the following necessary condition is satisfied by the boundary conditions :
 (16a)
(16a)
or, more explicitly,
 (16b)
(16b)
This is obtained by first integrating (14) on  and then applying the Green’s theorem. In what follows we will assume that (16b) is satisfied (it will be used later on!).
and then applying the Green’s theorem. In what follows we will assume that (16b) is satisfied (it will be used later on!).
In accordance with the definitions of  and
and let us write
let us write
 (17)
(17)
Since the field Equation (14) is equivalent to the equations
 (18a)
(18a)
 (18b)
(18b)
and
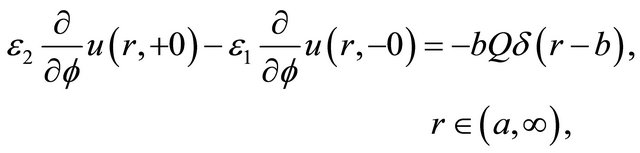 (18c)
(18c)
one can write
 (19a)
(19a)
 (19b)
(19b)
The coefficients  and
and 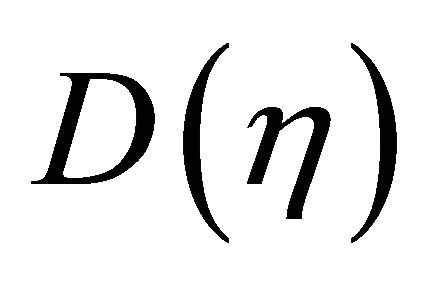 are determined through the conditions (15a), (15b), (18b) and (18c) as follows:
are determined through the conditions (15a), (15b), (18b) and (18c) as follows:
 (20a)
(20a)
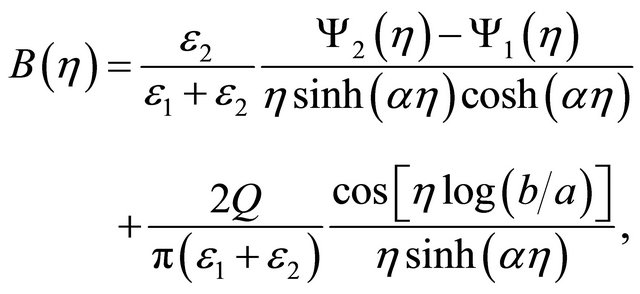 (20b)
(20b)
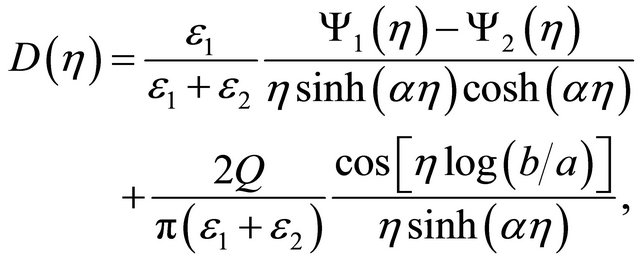 (20c)
(20c)
Here we put
 (21)
(21)
If we first insert (20a)-(20c) into ((19a), (19b)) and then use the Euler formula to write the cos function through exponential functions, then we get
 (22a)
(22a)
and
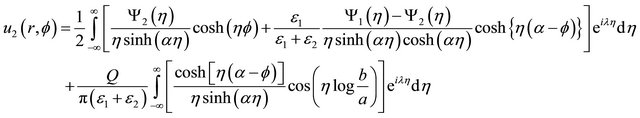 (22b)
(22b)
where l is defined with
 (22c)
(22c)
Now it is important to observe that the point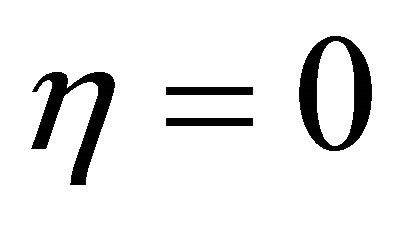 , which is located on the integration line, seems to be a double pole of the integrands in (22a) and (22b). But because of the relation (16b) these poles are removable. Indeed, the removability of these poles requires the condition
, which is located on the integration line, seems to be a double pole of the integrands in (22a) and (22b). But because of the relation (16b) these poles are removable. Indeed, the removability of these poles requires the condition
 (23)
(23)
which is equivalent to (16b), (21) being taken into account. Since the expressions taking place in the brackets in (22a) and (22b) are even functions of h, (23) guarantees the removability of the singularity at h = 0. Thus, on the basis of Jordan’s lemma, the integrals in ((22a), (b)) can be computed through the residues at the poles located in the upper  or lower
or lower  half-planes. The residue series coming from the poles which occur at the zeros of sinh(ah) and cosh(ah) are connected with the geometry of the wedge in question and, hence, consist of the eigen-functions series. But the terms coming from the poles of
half-planes. The residue series coming from the poles which occur at the zeros of sinh(ah) and cosh(ah) are connected with the geometry of the wedge in question and, hence, consist of the eigen-functions series. But the terms coming from the poles of  and
and  (if any!) are independent of the geometry of the wedge and have no connection with the eigen-functions.
(if any!) are independent of the geometry of the wedge and have no connection with the eigen-functions.
Remark. It is worthwhile to notice here that the convergence of the inverse transform integrals in (22a) and (22b) requires the relation (23). That means that the relation stated by (23) is in fact a condition for the existence of the solution. One can easily check that this is nothing but (16b) (or (16a)). This shows that the application of the transformation in question does not only permit us to obtain an explicit expression of the solution but rather shows also the necessary conditions for the existence of a solution.
4.1. A Particular Case. Point Sinks Located on the Lateral Boundaries
Assume more particularly that
 (24a)
(24a)
where  and M stand for two given constants such that (Cf. (23) or (16b))
and M stand for two given constants such that (Cf. (23) or (16b))
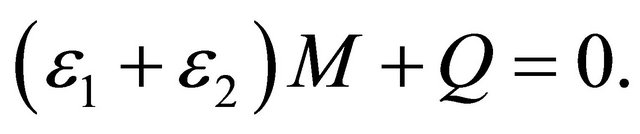 (24b)
(24b)
In this case the lateral boundaries consist of insulating materials and carry sinks at the points  and
and . By straightforward computations one gets
. By straightforward computations one gets
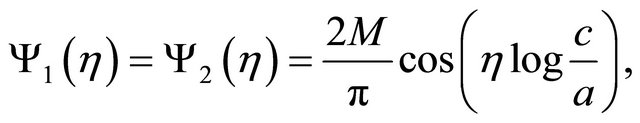 (24c)
(24c)
which reduces (22a) to
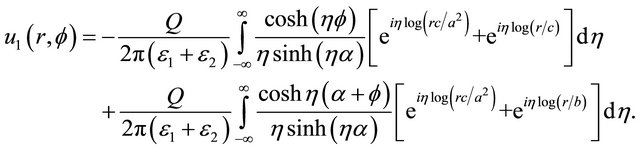 (25)
(25)
Since in the present case one always has

which yields
 as
as 
 as
as by the Jordan’s lemma, the first parts of these integrals can be computed by considering the residues of the poles taking place in the upper half-plane
by the Jordan’s lemma, the first parts of these integrals can be computed by considering the residues of the poles taking place in the upper half-plane . But, depending on the relative positions of r and c, one can get
. But, depending on the relative positions of r and c, one can get  or
or . Therefore the second part of the first integral involves the contributions of the poles existing in the half-plane
. Therefore the second part of the first integral involves the contributions of the poles existing in the half-plane  or
or  (similar situation is also valid for the second part of the second integral). Thus, we have to consider the following four cases separately:
(similar situation is also valid for the second part of the second integral). Thus, we have to consider the following four cases separately:
Case 1:  case 2:
case 2: 
Case 3: 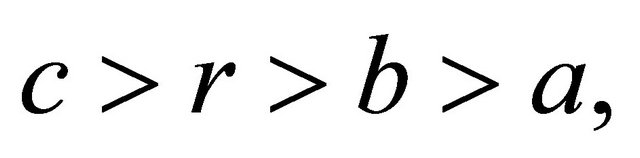 case 4:
case 4: 
By straightforward computations we get the following results:
 (26a)
(26a)
 (26b)
(26b)
 (26c)
(26c)
 (26d)
(26d)
By comparing (22b) with (22a) one observes that , where
, where . It is also interesting to compare (26a)-(26d) with the result pertinent to the case of
. It is also interesting to compare (26a)-(26d) with the result pertinent to the case of . Thus one concludes that the present two-part wedge problem is equivalent to the homogeneous wedge problem with constitutive parameter
. Thus one concludes that the present two-part wedge problem is equivalent to the homogeneous wedge problem with constitutive parameter  .
.
5. Conclusions and Concluding Remarks
From the analysis made above one concludes that the logarithmic transformations defined by (3a) and (3b) may be appropriate in getting explicit expressions of sectionally-harmonic functions which satisfy the homogeneous Dirichlet or Neumann boundary conditions on the circular part of the boundary of wedge shaped domains. This kind of problems arise in various domain of applications such as electrostatics, magneto-statics, hydrostatics, heat transfer, mass transfer, acoustics, elasticity, etc.
REFERENCES
- A. C. Eringen, “The Finite Sturm-Liouville Transform,” Quarterly Journal of Mathematics, Vol. 5, No. 1, 1954, pp. 120-129. doi:10.1093/qmath/5.1.120
- R. V. Churchill, “Generalized Finite Fourier Cosine Transforms,” Michigan Mathematical Journal, Vol. 3, No. 1, 1955, pp. 85-94. doi:10.1307/mmj/1031710540
- D. Naylor, “On a Mellin Type Integral Transform,” Journal of Mathematics and Mechanics, Vol. 12, No. 2, 1963, pp. 265-274.
- D. Naylor, “On an Integral Transform of the Mellin Type,” Journal of Engineering Mathematics, Vol. 14, No. 2, 1980, pp. 93-99. doi:10.1007/BF00037619
- I. N. Sneddon, “The Use of Integral Transforms,” McGrawHill Co., New York, 1972.
- W. R. Smythe, “Static and Dynamic Electricity,” McGrawHill Co., New York, 1950.
- E. C. Titchmarsh, “An Introduction to the Theory of Fourier Integrals,” Oxford University Press, 1948.
Appendix. Proof of Theorems
1. A Proof for Theorem-1
 is quite obvious. To find the limit of
is quite obvious. To find the limit of  for
for![]() , consider first the case when
, consider first the case when  and assume that an arbitrarily fixed (small)
and assume that an arbitrarily fixed (small)  is given. Since
is given. Since , we can find an
, we can find an  such that
such that
 (27a)
(27a)
Thus, from (3a) one gets

which yields
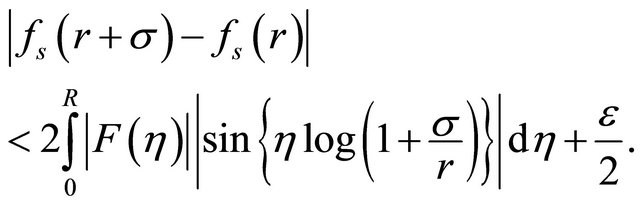 (27b)
(27b)
Now, by taking into account the inequalities (see Figure A1).
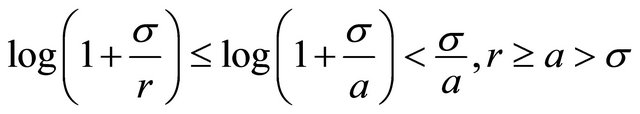 (27c)
(27c)
and

we can choose s so small that
 (27d)
(27d)
From (27b) and (27d) we conclude that for every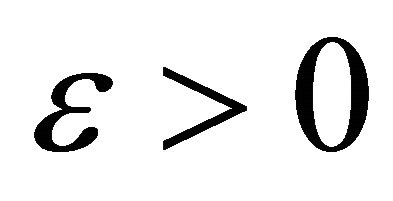 , however it is small, we can find
, however it is small, we can find  such that
such that

which permits us to write

For ![]() this gives the first equation in (4a).
this gives the first equation in (4a).
To prove the same equality for the case of , we choose an arbitrary number
, we choose an arbitrary number  and repeat the arguments made above by replacing
and repeat the arguments made above by replacing ![]() by
by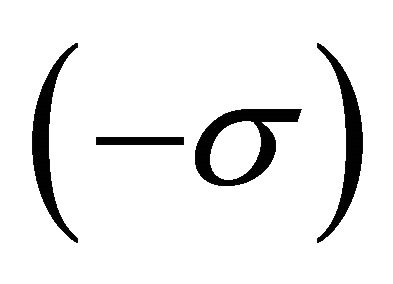 . All the lines except (27b) and (27c) remain unchanged while the latter become now as follows:
. All the lines except (27b) and (27c) remain unchanged while the latter become now as follows:

Figure A1. Logarithm functions.
 (28a)
(28a)
and
 (28b)
(28b)
Here  stands for a suitable number which does not depend on s (For detail see Figure A1). Thus, by choosing s sufficiently small, we guarantee
stands for a suitable number which does not depend on s (For detail see Figure A1). Thus, by choosing s sufficiently small, we guarantee
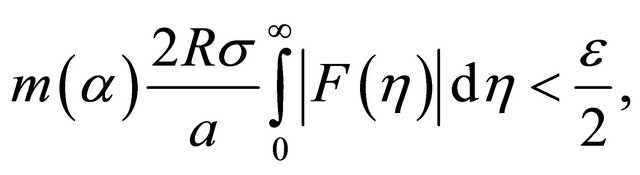 (28c)
(28c)
which yields

and
 (28d)
(28d)
For r = a the latter reduces to the first equality in (4a) when 
To see the limit of 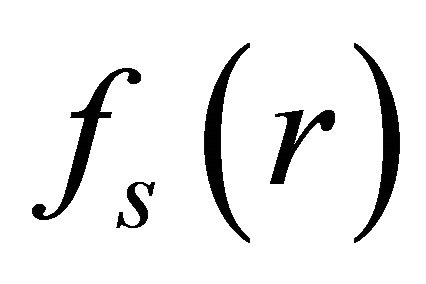 as r®0, consider an arbitrarily given (small)
as r®0, consider an arbitrarily given (small) 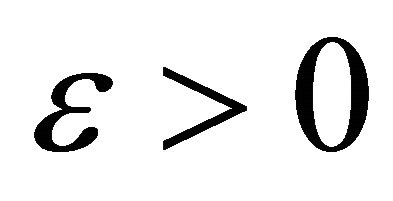 and choose
and choose 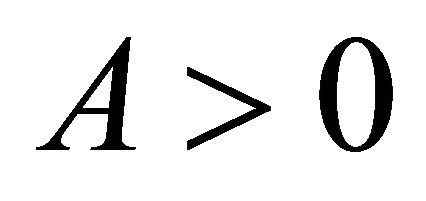 such that the second part in
such that the second part in
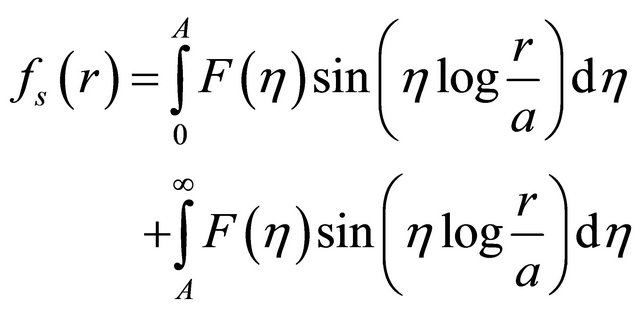 (29a)
(29a)
meets the following inequality:
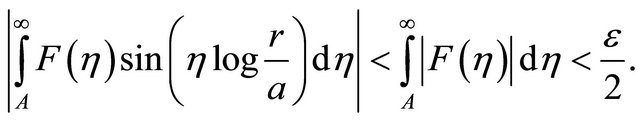 (29b)
(29b)
After having fixed A, let us make . By virtue of the well-known Riemann-Lebesgue lemma [5, p. 30], the first part in (29a) tends to zero when
. By virtue of the well-known Riemann-Lebesgue lemma [5, p. 30], the first part in (29a) tends to zero when . Therefore, for sufficiently small r one has also
. Therefore, for sufficiently small r one has also
 (29c)
(29c)
From (29a)-(29c) one concludes that for sufficiently small r one has  for every
for every  This proves the second equality in (4a).
This proves the second equality in (4a).
(29a)-(29c) are also valid for r ® ¥, which shows the last equality in (4a)
2. A Proof for Theorem-2
The equalities given in (4b) can be shown by repeating the reasoning made in proving Theorem-1. As to the equality given in (4c), owing to the assumption

and
 (30)
(30)
it is reduced to the first equality in (4a).
3. Proofs of Theorem-3 and 4
Proof of these theorems are based on the following wellknown lemma (see [5, p. 34]).
Lemma (Fourier’s integral theorem). If  is piece-wise continuous and absolutely integrable in
is piece-wise continuous and absolutely integrable in , then for all
, then for all 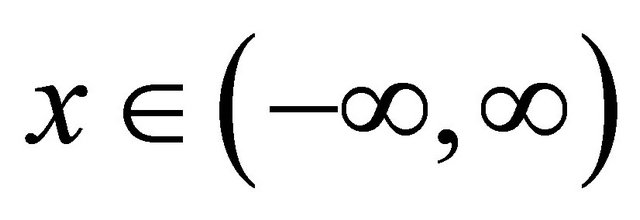 one has
one has
 (31)
(31)
Let us insert (5a) into the right-hand side of (3a) and make the substitutions
 (32a)
(32a)
which yield
 (32b)
(32b)
and
 (33a)
(33a)
Now let us define the odd function

as follows (notice that ):
):
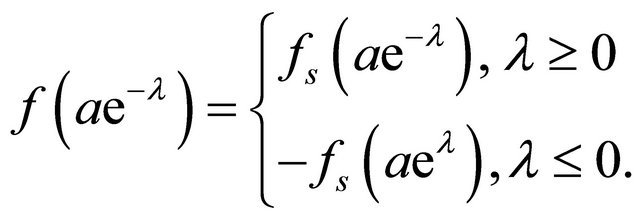 (33b)
(33b)
Then (33a) can also be written as
 (33c)
(33c)
or
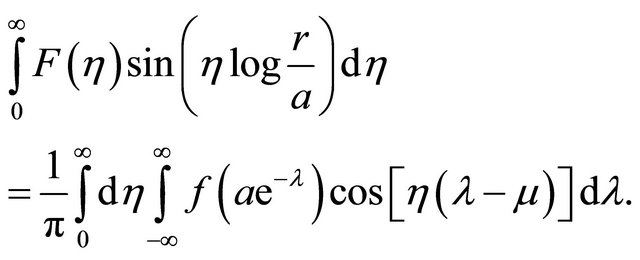 (33d)
(33d)
Since the function  meets the requirements mentioned in the lemma, from the last expression one gets
meets the requirements mentioned in the lemma, from the last expression one gets
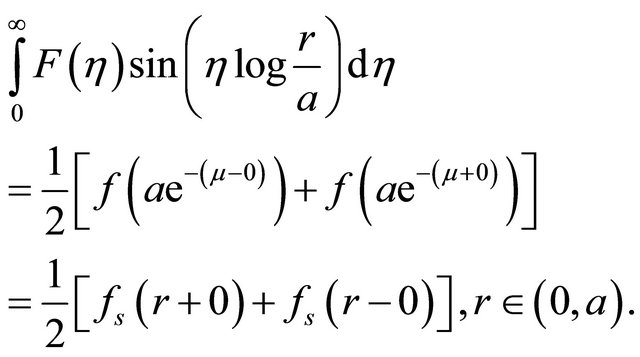 (34)
(34)
This proves (5a).
To prove (5b), one starts from (3b) and repeats (32a)- (34) with the only exception that (33b) is replaced now by the even function

Proof of theorem-4 is quite similar to that of Theorem-3. The only difference is that l and m defined in (32a) are replaced now by

and
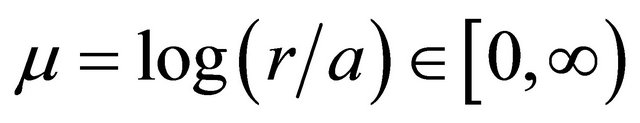 .
.

