Abstract

In the present paper, we show that there exists a unique common fixed point for four self maps in a fuzzy metric space where two of the maps are reciprocally continuous and the other two maps are z-asymptotically commuting.

Keywords

T-Norm, Fuzzy Metric Space, Reciprocally Continuous, Z - Asymptotically Commuting Maps

Share and Cite:

Received 27 January 2016; accepted 28 March 2016; published 31 March 2016

1. Introduction

L. Zadeh’s [1] investigation of the concept of fuzzy set in the year 1965, has led to a rich growth of fuzzy mathematics. Today, it has become a well-accepted system to embrace upon uncertainties springing in numerous physical situations. The theory of fixed point equations is one of the extrusive basic tools to exploit various physical formulations. Theorems on fixed points in fuzzy mathematics are emerging with flourishing hope and vital certainty.

Many authors have introduced the concept of fuzzy metric space in various ways and have shown that every metric induces a fuzzy metric. There have been several endeavors to formulate fixed point theorems in fuzzy mathematics. In 1975, Kramosil and Michalek [2] generalized the statistical metric space and defined the fuzzy metric space which was later modified by George and Veeramani [3] [4] by introducing the concept of continuous t-norms. Recently, many researchers [5] - [9] have enormously developed the theory by studying various aspects of the theory and extending the concept of fuzzy metric through applying several contractive, expansive, continuity and compatibility conditions on the fuzzy metric and producing different results.

Pant [10] introduced the notion of reciprocally continuous mappings and established a fixed point theorem. S. N. Mishra, Nilima Sharma, S. L. Singh [11] defined z-asymptotically commuting maps in fuzzy metric spaces which may be seen as a comparable formulation given by Trivari-Singh [12] in metric spaces. These mappings are more general than commuting and weakly commuting maps.

The aim of this paper is to show that the self maps in a fuzzy metric space satisfying certain properties and inequalities possess a common fixed point which is unique.

2. Preliminaries

Here, we shall recall some prefaces:

Definition 2.1 ( [13] ): A binary operation 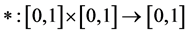is said to a continuous t-norm if 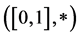is an abelian topological monoid with unit whenever & .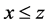

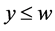
2.1(α) Basic continuous t-norms are:

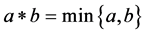・ (minimum t-norm)

・ (product t-norm)

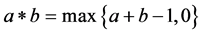・ (Lukasiewicz t-norm)

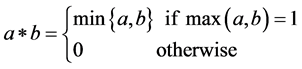・ (weakest t-norm, the drastic product)

Definition 2.2 ( [3] ): Let X be any non-empty set, 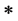is a continuous t-norm and M is a fuzzy set on X × X × (0, ∞) satisfying

a)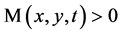

b) Û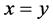

c)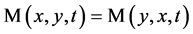

d)

e) is continuous where,

Here, denotes the degree of nearness between x, y with respect to “t”.

・ Grabiec ( [14] ) had shown that is non-decreasing.

Definition 2.3 ( [3] ): A sequence in a fuzzy metric space said to converge to Û as.

Definition 2.4 ( [3] ): A sequence in a fuzzy metric space is said to be a Cauchy sequence if for each, , there exists such that for all.

Definition 2.5 ( [3] ): If every Cauchy sequence in a fuzzy metric space X is convergent, then X is said to be complete.

Definition 2.6 ( [10] ): Two self maps A and B of a fuzzy metric space are said to be reciprocally continuous on X if

and

whenever is a sequence in X such that

and

for some.

Definition 2.7 ( [11] ): Two self maps A and B of a fuzzy metric space X are said to be z-asymptotically commuting if and only if

whenever is a sequence in X such that

for some and.

Lemma 2.8 ( [14] ): Let be a fuzzy metric space. If there exists such that for all and t > 0, then.

Succeeding the Grabiec’s approach to fuzzy contraction principle, Mishra. S. N., Nilima Sharma, Singh. S. L. [11] had obtained common fixed point theorem for asymptotically commuting maps in fuzzy metric spaces.

Theorem 2.9 ( [11] ): Let be a complete fuzzy metric space with, and . If there exist continuous maps and a constant such that

1) ST = TS

2) {P, S} and {Q, T} are asymptotically commuting pairs

3)

4)

for all, t > 0 and then P, Q, S, T have a unique common fixed point.

3. Main Results

Theorem 3.1: Let be a complete fuzzy metric space & be any of the continuous t-norms given in 2.1(α) and let A, B, S, T be self maps of X satisfying

・ The pair {A, S} is reciprocally continuous

・ The pair {B, T} is z-asymptotically commuting

・ The pairs {B, S} and {T, S} commute with each other

・ (1)

where, , then A, B, S, T have a unique common fixed point in X.

Proof: {A, S} is reciprocally continuous:

⇒ and

whenever is a sequence in X such that

and for some (2)

{B, T} is z-asymptotically commuting:

⇒

whenever is a sequence in X such that

for some (3)

・ To prove that:

Put and in (1), we get

Letting, we have

(Since from (2) and (3))

(Since)

(from lemma 2.8) (4)

i.e., we can find a such that (2) and (3) holds simultaneously.

Consider (3)

(Since from (3))

(since) (5)

・ To prove that:

Put and in (1), we get

(Since the pairs {B, S} and {S, T} commute with each other).

Taking on both sides, we have

(from lemma (2.8))

But from (4), we get (6)

・ To prove:

Put and in (1)

Taking limit on both sides, we get

(Since from (2))

(Since from (5))

(from lemma (2.8))

But from (4), we get (7)

From (5), (6) and (7) we have (8)

・ To prove:

Consider

(9)

⇒ from (8) and (9), we have

z is a common fixed point of A, B, S, T.

・ To prove Uniqueness of z:

Let us assume that A, B, S, T have another common fixed point in X say p where

i.e.,

Now we prove that.

Consider

(using lemma (2.8))

There exists a unique common fixed point of A, B, S, T in X. W

Example 3.2: Let X = [0, 2], where and be the continuous t-norm given by.

Clearly, is a Complete fuzzy metric space.

Let A = 1, , S = x, be self maps on X.

Let be a sequence in X.

and

and where.

⇒ A and S are reciprocally continuous.

Let be a sequence in X.

and where.

⇒ B and T are z-asymptotically commuting where z = 1.

Also, the four maps satisfies (iii) and (iv) of theorem 3.1.

⇒ A, B, S, T have a Unique common fixed point in X i.e., at x = 1. W

Conflicts of Interest

The authors declare no conflicts of interest.

References