Experimental Design in Solvent Extraction: A Study for Divalent Metals Separation in D2EHPA/Isoparaffin System ()
1. Introduction
Since the 1950s, the solvent extraction (SX) has been increasingly used in the minerals processing industry. Today, solvent extraction has become an important economical, practical and clean hydrometallurgical process and the technique is one of the most versatile methods used for the extraction, separation and recovery of metallic species from aqueous media [1,2].
Various solvent extraction reagents and processes have been proposed for the recovery and separation of metal ions in aqueous solution. Di-(2-ethylhexyl) phosphoric acid (abbreviated as D2EHPA or simply HA) has been used successfully to extract many divalent metal ions from sulphate media as has been reported elsewhere, with varying studies focused, determination of parameters such as rate constant, pH, distribution coefficients, equilibrium constant and composition of extracted species in different conditions. These parameters can be determined via graphical or numerical analysis of experimental data. Nathasarma and Devi (2006) reported the order of extraction of eight metal ions from a sulphate solution using D2EHPA, which is reported as Fe3+ > Zn2+ > Cu2+ > Co2+ > Ni2+ > Mn2+ > Mg2+ > Ca2+ [3-7].
On account of the dimerization of acid phosphoorganic extractants, it is reported that in the formation of complexes with divalent metals ions, these extractants take part as a monomer rather than dimmer, in isoparaffin [8,9]. The reaction of a divalent metal extraction with D2EHPA can be written as Equation (1), when M to be the divalent metal [5]:
(1)
Solutions of sulfuric acid have been frequently used as a leaching agent for manganese, cobalt, nickel and copper ore. However, the dissociation of bisulfate ion into hydrogen and sulfate ion is incomplete and a thermodynamic analysis of the acid solution is not trivial [10-20]. Thus, within the process of divalent metals extraction by D2EHPA the aim was to evaluate the influence of four variables as: concentrations of metals (Mn, Co, Ni, and Cu) in the synthetic leach liquor, concentration of extractant D2EHPA, the A/O ratio and the final equilibrium pH upon the percentage of extraction of the metals studied, leading to defining the optimum ranges for operation of the process of solvent extraction systems as studied. The metal concentrations in the synthetic leach liquor were based on the composition of a liquor generated from the leaching of the manganese ore from Mina AzulPará state, Brazil [20].
2. Theoretical
The class of experimental design most often used to adjust models to investigate interaction effects of first order (linear) and second order (quadratic) is the central composite design. This experimental design has a configuration “star + cube”, which consists of a factorial classic experiment of three levels (–1, 0 and +1) a distance of ±1 of the central point, plus 2k axial points (star) at a distance ±1 α of the center point and nc point central [21].
To assess the influence of independent variables on the dependent variable, we conducted a factorial design experimental plan with three levels (–1, 0 and +1) plus a star configuration, third order model, in a total of 40 laboratory experiments, using a factorial design matrix shown in Table 1 [22].
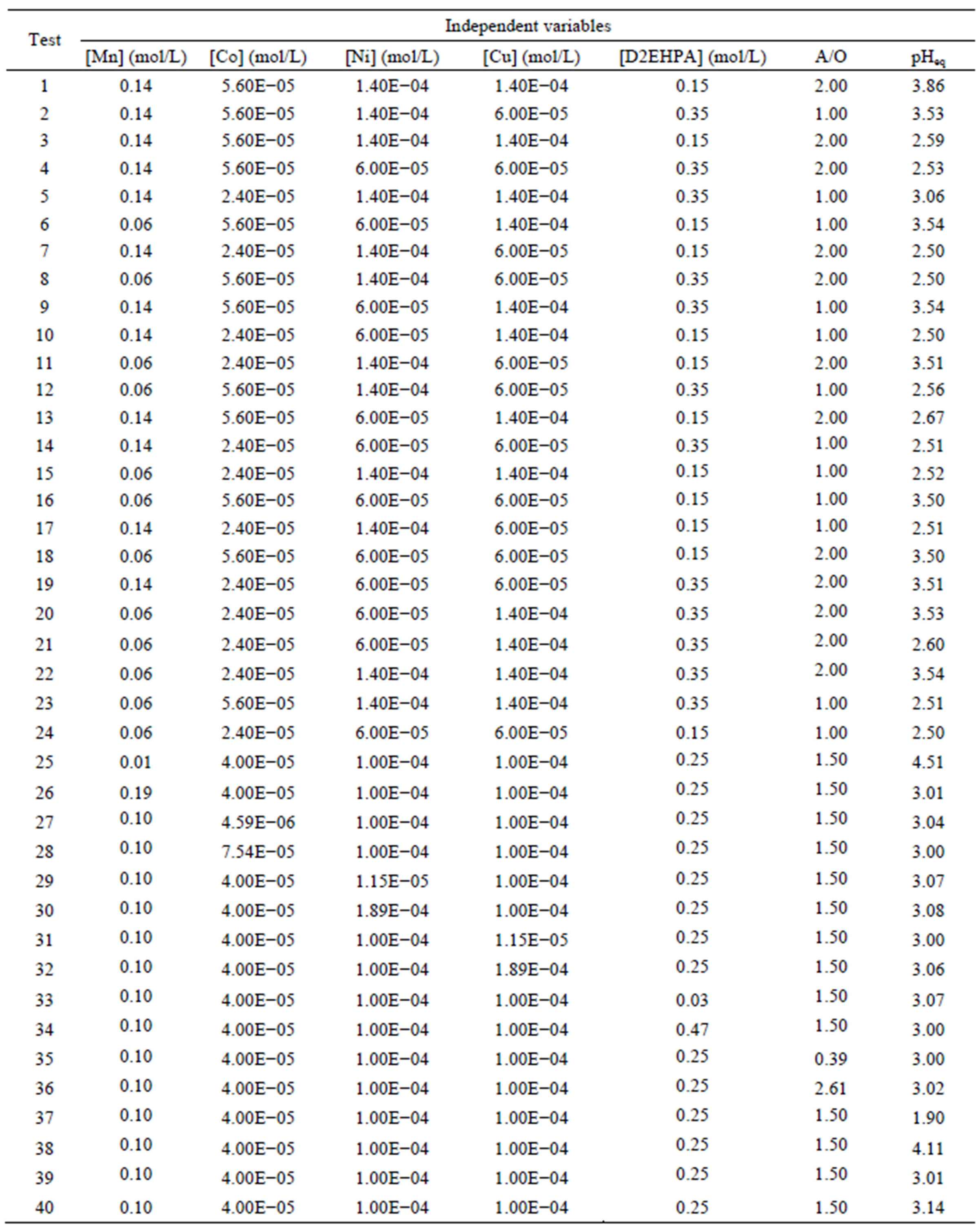
Table 1. Experimental design, ratio A/O and pH equilibrium.
The proposed model for the nonlinear regression is that of Equation (2) where βi’s are the estimated parameters obtained in this model with the second order with central point. It is typically used when one is interested in detecting curvature in a response function, since the regression model is given by:
(2)
According to the F test and their calculated probabilities, if p calculated is less than 0.05 (95% confidence) the effect of that variable or interaction is considered significant. Then, parameters can be ruled out where p values were larger than 0.05 (see Table 2). Statistical analysis will be performed later using the software STATISTICA® for comparison also for the developed model.
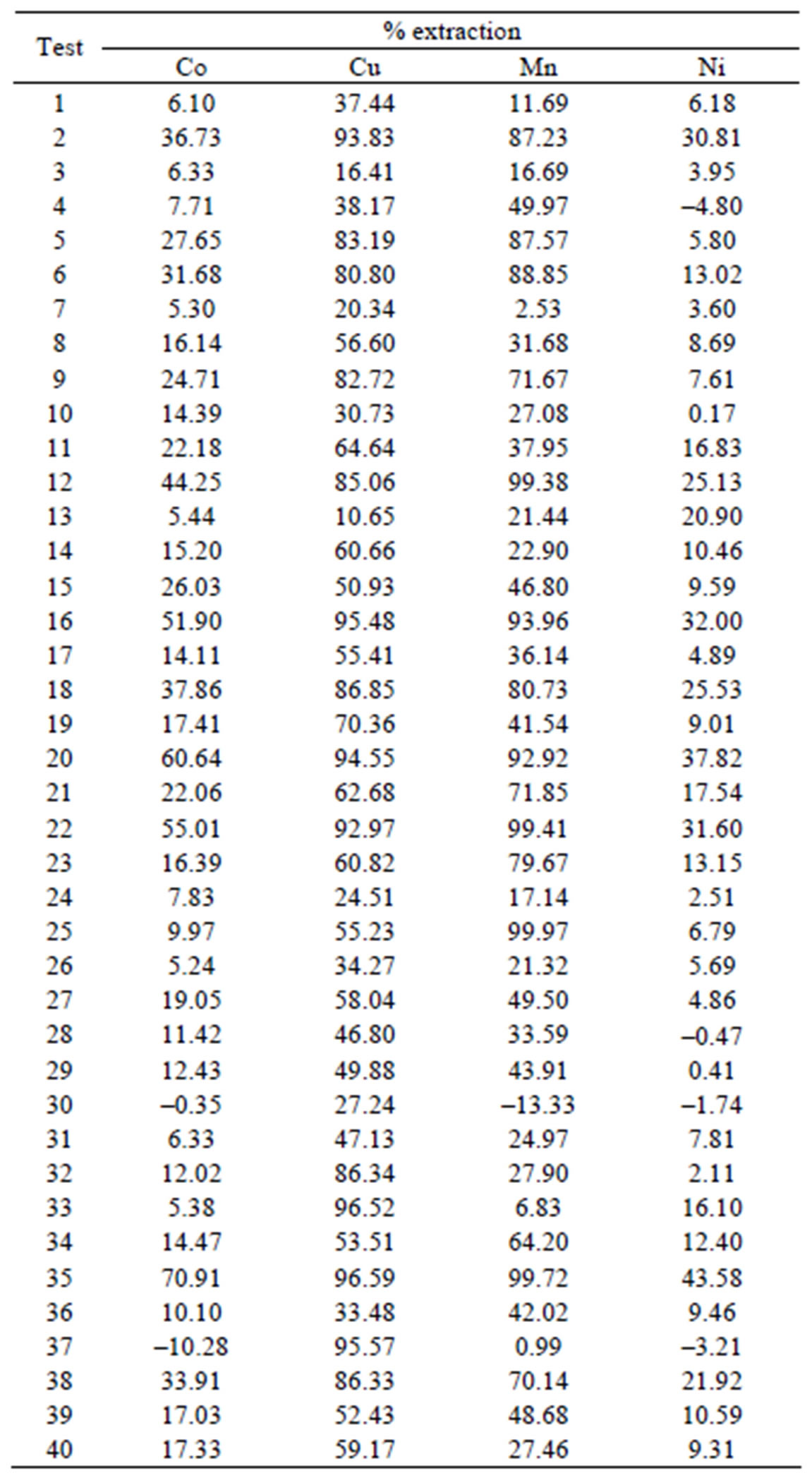
Table 2. Percentage of extraction in the design experimental.
3. Experimental
The similar solutions of synthetic leach liquor were prepared by dissolution of MnSO4 CoSO4·7H2O, NiSO4·6H2O and CuSO4·5H2O (VETEC) in deionised water. The initial pH of the aqueous solutions was controlled by the addition of diluted H2SO4. The solution of Di-(2-ethylhexyl) phosphoric acid (Sigma Aldrich) was prepared by dissolution in Isoparaffin 17/21 (UNIPAR) without any prior purification.
Equal aliquots (25 mL) of aqueous (Vaq) and organic (Vorg) solutions were contacted in a 100 mL glass reactor by a magnetic stirrer (IKA) for 10 min at 298.15 K. After assessment of the balance of the solution was added with the aid of a burette, a solution NaOH 0.5 M, with constant stirring, until reaching the pH determined by experimental design.
Upon reaching a steady pH (unchanging for a period of 5 minutes) the aqueous and organic solutions were decanted into a separating funnel, and the aqueous phase taken for analysis, after the set time of contact, the aqueous and organic phases (Sousa Junior et al., 2010).
The variables considered with the potential to influence the divalent metal extraction (dependent variable) was the concentration of the metals themselves, the volume ratio A/O, the concentration of organic extractant and pH balance (independent variables).
Concentrations of divalent metal in the aqueous phases 
were determined by atomic absorption spectrophotometer (Varian, model 50B), and the concentrations 
in the were organic phase determined using the mass balance for manganese. The pH was measured by a pH meter (DIGIMED).
4. Results
The results observed in the dependent variable the percentage extraction of Co, Mn, Cu and Ni were representative. The model was based on linear and quadratic effects of all independent variables, according to Table 2 and Figure 1 at 8.
Table 3 lists all the values of effects of the variables and coefficients regression for iterations linear and quadratic in model extraction of divalent metals present in the synthetic bleach.
According to the Pareto chart, shown in Figure 1, considering the linear and quadratic interactions of extraction of cobalt present in a synthetic leach liquor is observed that in addition to the A/O (linear) and pH equilibrium (linear), the relationship A/O quadratic and the concentration of manganese (linear) are important variables in the extraction of the metal that present in synthetic leach liquor.
Figure 2 shows the residual values observed versus