The Significance of the Distinction between a Necessary Condition and a Sufficient Condition

Eliahu Comay

Charactell Ltd., Tel Aviv-Yafo, Israel.

DOI: 10.4236/oalib.1109721                

Abstract

The distinction between the concepts of a Necessary Condition and a Sufficient Condition is a fundamental element of mathematics. This issue certainly applies to physics which has a mathematical structure. However, this work shows that sometimes physical textbooks ignore this distinction. An analysis of the conserved 4-current of the Noether theorem proves that this issue is extremely important. The analysis uses the dimension of the Lagrangian density and the corresponding dimension of the quantum function of physical theories. It is proved that the QED theory of a Dirac electron yields a coherent expression for the 4-current and the QED interaction term. In construct, the Klein-Gordon theory of a charged particle as well as the electroweak theory of the W± particles violates Maxwellian electrodynamics. Unlike the Dirac electron, these theories have no coherent interaction term between the 4-current of the charged particle and the electromagnetic fields. This result relies on new necessary conditions that are required for the compatibility of the 4-current of a charged quantum particle. The new necessary conditions prove that the continuity equation of the Noether theorem is not a sufficient condition for an acceptable 4-current.

Keywords

The Conserved 4-Current, Quantum Electrodynamics, The Klein-Gordon Theory, The Electroweak Theory

1. Introduction

The notions of Necessary Condition and Sufficient Condition are different concepts that are used in mathematics [1] as well as in daily life. Mathematical arguments do not use unnecessary words. Hence, the terms mentioned above indicate that these concepts are not identical. Scientific papers use the expression necessary and sufficient condition in order to emphasize that the two different conditions hold (see e.g., [2] ). For example, in most places on the globe, cloudy sky is a necessary condition for rainfall. However, in these places, there are circumstances of cloudy sky but no rain. This example proves that at these places, although cloudy sky is a necessary condition for rainfall this situation is not a sufficient condition for rainfall. This work analyzes the distinction between these notions and proves that it is an important element for the coherence of a physical theory.

Electrodynamics is a physical theory that describes the time evolution of two physical objects―electrically charged matter and electromagnetic fields [3] [4]. Maxwell equations describe the time evolution of electromagnetic fields. The standard form of the inhomogeneous Maxwell equations is (see e.g. [3] : section 30)

$F_{\mathrm{,}\nu }^{\mu \nu }=-4\pi j^{\mu }\mathrm{,}$ (1)

where $F^{\mu \nu }$ is the antisymmetric tensor of the electromagnetic fields. Hence, the 4-divergence of (1)

$F_{,\nu ,\mu }^{\mu \nu }=-4\pi j_{,\mu }^{\mu }=0$ (2)

means that

$j_{,\mu }^{\mu }=0.$ (3)

The null value of the 4-divergence of the 4-current $j^{\mu }$ is called the continuity equation (see e.g. [3] : section 29; [4] : p. 549).

This argument proves that a legitimate 4-current $j^{\mu }$ of a charged particle must satisfy the continuity equation. However, Maxwellian electrodynamics is a vast theory that comprises many relationships between physical entities. Quite lengthy textbooks describe classical electrodynamics [3] [4]. Similarly, lengthy textbooks describe the quantum domain of electrodynamics [5]. It turns out that a 4-current that satisfies the continuity equation does not necessarily satisfy every required relationship of Maxwellian electrodynamics.

This work examines details of electrodynamics and proves that although the continuity Equation (2) is a necessary condition for a theory that is consistent with Maxwellian electrodynamics, this equation is not a sufficient condition for such a theory. It means that there are theories that provide a 4-current that satisfies the continuity equation but violates other necessary conditions of Maxwellian electrodynamics. It turns out that electrically charged particles of the Klein-Gordon (KG) theory and the W^± particles of the electroweak theory violate crucial requirements of electrodynamics.

Units where $\hslash =c=1$ are used. Therefore, just one dimension is required and the dimension of length $\left[L\right]$ is used. The Minkowski metric $g_{\mu \nu }$ is diagonal and its entries are (1, −1, −1, −1). Formulas take the tensorial structure. The Dirac ${\gamma }^{\mu }$ matrices take the form of [6]. All expressions are written in the standard notation and readers may consult the relevant references.

2. The Noether Theorem

A contemporary quantum field theory (QFT) depends on the variational principle that applies to the action of a Lagrangian density $\mathcal{L}$

$S={\displaystyle \int \mathcal{L}{\text{d}}^{4}x}\mathrm{.}$ (4)

A well-known textbook states: “All field theories used in current theories of elementary particles have Lagrangians of this form” (see [7] : p. 300). This work adheres to this principle. A self-evident result of this approach is that in the unit system where $\hslash =c=1$, the dimension of all terms of a Lagrangian density $\mathcal{L}$ is $\left[L^{-4}\right]$. This property determines the dimension of the quantum functions that are used in the relevant Lagrangian density.

The Euler-Lagrange equation

$\frac{\partial \mathcal{L}}{\partial \psi }-\frac{\partial }{\partial x^{\mu }}\frac{\partial \mathcal{L}}{\partial \left(\partial \psi /\partial x^{\mu }\right)}=0$ (5)

(see [7] : p. 300; [8] : p. 16; [9] : p. 17) is derived from such a Lagrangian density. This equation determines the time evolution of the relevant physical object. One attribute of the Noether theorem states that if the Lagrangian density of a given theory does not explicitly depend on the coordinates $x^{\mu }$ then the theory conserves energy-momentum (see e.g., [8] : p. 310).

Let us examine the quantum function of an electrically charged particle and the utilization of the Noether theorem for the derivation of its conserved 4-current (see [10] : p. 314; [8] : p. 18). The analysis examines a Lagrangian density where each term that comprises the quantum function $\psi$ also comprises its complex conjugate ${\psi }^{†}$. The standard form of the QED Lagrangian density (see [8] : p. 78)

${\mathcal{L}}_{QED}=\bar{\psi }\left(i\text{}\text{}\text{}\cancel{\partial }-m\right)\psi -\frac{1}{4\pi }{F}_{\mu \nu }{F}^{\mu \nu }-e\bar{\psi }{\gamma }^{\mu }\psi A_{\mu }$ (6)

illustrates this issue. Here $\bar{\psi }={\psi }^{†}{\gamma }_0$. This Lagrangian density is invariant under the global phase transformation of the charged particle’s quantum function

$\psi \to e^{i\alpha }\psi ,$ (7)

where $\alpha$ denotes a mathematically real constant. In this case, the Noether theorem yields an expression for a conserved 4-current of the theory of the examined Lagrangian density (see [10] : eq. (14.11), p. 314). The required expression is

$j^{\mu }=a\frac{\partial \mathcal{L}}{\partial {\psi }_{\mathrm{,}\mu }}\psi \mathrm{,}$ (8)

where a is an appropriate numerical coefficient and $j^0$ is the particle’s density. Hence, a is fixed so that ${\displaystyle \int j^0{\text{d}}^{3}x}=1$. This 4-current satisfies the continuity equation (3).

An application of the Noether theorem for the 4-current (8) of the QED Lagrangian density (6) yields the Dirac expression

$j^{\mu }=\bar{\psi }{\gamma }^{\mu }\psi$ (9)

(see [6] : p. 23). It is important to note that the Dirac 4-current (9) is used in the electromagnetic interaction term of the QED Lagrangian term (6) where it is coupled to the electromagnetic 4-potential $A_{\mu }$.

The $\left[L^{-4}\right]$ dimension of the Lagrangian density and the term $\bar{\psi }m\psi$ of the QED Lagrangian density (6), prove that the dimension of the Dirac function $\psi$ is $\left[L^{-3/2}\right]$. Hence, the dimensionless of the numerical ${\gamma }^{\mu }$ matrices proves that the dimension of the Dirac 4-current (9) is $\left[L^{-3}\right]$ which is the required property of a 4-current. Furthermore, the Dirac 4-current (9) is free of a derivative ${\psi }_{\mathrm{,}\mu }$ of the quantum function. The significance of these attributes is discussed later.

The continuity equation is a well-known necessary condition of Maxwellian electrodynamics (see [3] : section 29; [4] : p. 549). However, the 4-current should also be compatible with all other requirements of Maxwellian electrodynamics. This self-evident issue is the main objective of this work.

3. The Klein-Gordon Equation

Let us analyze the quantum function $\varphi$ of the charged KG particle. The Lagrangian density of this particle is

${\mathcal{L}}_{KG}=\left({\varphi }_{\mathrm{,}\mu }^{\mathrm{<mo>\; *\; </mo>}}-ie{A}_{\mu }{\varphi }^{\mathrm{<mo>\; *\; </mo>}}\right)\left({\varphi }^{\mathrm{,}\mu }+ie{A}^{\mu }\varphi \right)-m^2{\varphi }^{\mathrm{<mo>\; *\; </mo>}}\varphi \mathrm{,}$ (10)

where $A_{\mu }$ is the electromagnetic 4-potential (see [11] : eq. (37) on p. 198). This Lagrangian density determines the Euler-Lagrange equation of the KG quantum function $\varphi$

$\left({\partial }_{\mu }-ie{A}_{\mu }\right)\left({\partial }^{\mu }-ie{A}^{\mu }\right)\varphi +m^2\varphi =0$ (11)

(see [11] : eq. (39) on p. 198).

An application of the Noether theorem to the Lagrangian density of the KG charged particle (10) yields this form of a conserved 4-current

$j_{\mu }=i\left({\varphi }_{\mathrm{,}\mu }{\varphi }^{\mathrm{<mo>\; *\; </mo>}}-{\varphi }_{\mathrm{,}\mu }^{\mathrm{<mo>\; *\; </mo>}}\varphi \right)-2e{A}_{\mu }{\varphi }^{\mathrm{<mo>\; *\; </mo>}}\varphi$ (12)

(see [11] : eqs. (42), (43) on p. 199). The Noether theorem proves that the 4-current (12) satisfies the continuity equation which is a necessary condition for Maxwellian electrodynamics.

Let us examine the compatibility of the KG 4-current (12) with other requirements of Maxwellian electrodynamics. Assume that the KG 4-current (12) is OK. Then its contraction with the electromagnetic 4-potential is the electromagnetic interaction term

${\mathcal{L}}_{KG\mathrm{<mo>\; \_\; </mo>}Int}=-\left\{i\left({\varphi }_{\mathrm{,}\mu }{\varphi }^{\mathrm{<mo>\; *\; </mo>}}-{\varphi }_{\mathrm{,}\mu }^{\mathrm{<mo>\; *\; </mo>}}\varphi \right)-2e{A}_{\mu }{\varphi }^{\mathrm{<mo>\; *\; </mo>}}\varphi \right\}e{A}^{\mu }\mathrm{.}$ (13)

This KG interaction comprises (up to an irrelevant constant factor) the term

${\mathcal{L}}_{KG}=e^2{A}_{\mu }{A}^{\mu }{\varphi }^{\mathrm{<mo>\; *\; </mo>}}\varphi +\cdots$ (14)

Please note that the Lagrangian density (10) of the Pauli-Weisskopf KG article [11] also comprises this term. One should realize that the interaction term (14) depends on the quadratic factor of the 4-potentials $A_{\mu }{A}^{\mu }$.

Let us see how this term affects the equations of motion of electromagnetic fields. The 4-potentials $A_{\mu }$ are the generalized coordinates of Maxwellian fields (see [3] : p. 78). Hence, an application of the Euler-Lagrange Equation (5) to the quadratic expression $A_{\mu }{A}^{\mu }$ proves that for the charged KG particle, the Euler-Lagrange equations of electromagnetic fields explicitly depend on the 4-potential $A_{\mu }$. Hence, the KG theory of [11] is unacceptable because it violates gauge invariance of the equation of motion of electromagnetic fields, namely, Maxwell equations.

There is another aspect of this contradiction. The erroneous interaction term (14) which describes a single charged KG particle, has the quadratic factor $e^2$. This charge-dependent term certainly violates Maxwellian electrodynamics because this interaction does not distinguish between positively and negatively charged particles.

Conclusions: Here are two more necessary conditions for the compatibility of a given theory with Maxwellian electrodynamics:

Each of these requirements is an independent necessary condition for Maxwellian electrodynamics, and they join the continuity Equation (3). In particular, it is proved above that the KG theory of a charged particle is unacceptable because it violates the necessary conditions NC.1 and NC.2.

4. The Electroweak Theory

Besides the KG theory, this work also analyzes the electroweak theory. Unlike the KG theory which is derived from an explicit form of its Lagrangian density (10), textbooks do not publish the entire form of the Lagrangian density of the electroweak theory. In particular, the electroweak theory regards the charged particles W^± as crucial theoretical elements. It turns out that QFT textbooks refrain from writing a Lagrangian density that comprises a coherent expression for the interaction of the 4-current of the W^± particles with the 4-potentials $A_{\mu }$ of electromagnetic fields. However, despite this discrepancy, this work relies on fundamental physical properties and proves the existence of uncorrectable problems of the electroweak theory of the W^± [12].

Let us examine the electroweak quantum function of the W^± particles that takes a mathemtically complex form. The mass term of the Lagrangian density of these particles is

${\mathcal{L}}_{WM}=-M^2{W}_{\mu }^{†}{W}^{\mu }\mathrm{,}$ (15)

(see [13] : p. 309). This expression and the $\left[L^{-4}\right]$ dimension of the Lagrangian density, prove that the dimension of the electroweak W^± quantum functions is $\left[L^{-1}\right]$.

The mathematically real attribute of the 4-current means that each quantum function of the 4-current of the W^± particles must contain the product of the functions $W^{†}W$. The dimension of this product is $\left[L^{-2}\right]$. For this reason, the $\left[L^{-3}\right]$ dimension of the 4-current means that the required 4-current of the W^± particles must contain an additional factor whose dimension is $\left[L^N\right]$, where N is an odd integer.

Two candidates can be used for this end: the coordinates $x^{\mu }$ and the partial derivative ${\partial }_{\mu }$. Here one should note that below (5) of section 2, it is proved that the coordinates $x^{\mu }$ cannot be used because the theory must conserve energy-momentum. Moreover, a 4-current $j^{\mu }$ of an electrically charged particle must be derivative-independent of the quantum function ${\varphi }_{\mathrm{,}\mu }$. Indeed, if an expression for $j^{\mu }$ depends on such a derivative, then due to the Noether theorem (8), the interaction term $e{j}^{\mu }{A}_{\mu }$ modifies the 4-current that is used in the interaction term of the Lagrangian.

The fact that this argument depends on solid requirements of dimension coherence of physical expressions justifies its validity. This analysis proves another necessary condition for a coherent 4-current of a charged particle.

This analysis examines the dimension of physical quantities and explains why the electroweak theory cannot properly define a coherent 4-current that is required for the electromagnetic interaction of the W^± particles. This argument also applies to the charged KG particles.

5. Discussion

The dimensional problems of the electroweak theory of the 4-current of the W^± also appear in other parts of this theory. Here are details of several publications that present an electromagnetic interaction term of the W^± particles. This term takes the form (see [14] : p. 518),

${\mathcal{L}}_W=ae{F}_{\mu \nu }\left(W^{+\mu }{W}^{-\nu }-W^{-\mu }{W}^{+\nu }\right)+\cdots$ (16)

where $F_{\mu \nu }$ is the electromagnetic fields’ tensor and a is a numerical constant. An analogous term is shown in [8] : p. 752; [15] : p. 113; [16] : eq. (3); [17] : eq. (3.31). An examination of expression (16) proves that it is unacceptable. Indeed, the electric charge e of a Lagrangian density should be multiplied by an expression that describes its density. The electromagnetic field $F_{\mu \nu }$ is charge-independent. Hence the W functions of (16) should account for charge density. However, the dimension of the product of two W functions is $\left[L^{-2}\right]$ whereas the dimension of density is $\left[L^{-3}\right]$. This is certainly a gross error.

The necessary conditions of Maxwellian electrodynamics that are written at the end of sections 3 and 4, indicate the novelty of this work. These necessary conditions are used in an examination of the QED theory of a spin-1/2 charged Dirac particle, the theory of a charged KG particle, and the electroweak theory of the charged W^± particles. The Noether expression for the QED 4-current of a charged Dirac particle is $j^{\mu }=\bar{\psi }{\gamma }^{\mu }\psi$ (see (9) above). The dimension of this 4-current takes the required value $\left[L^{-3}\right]$, and it is free of a derivative of the quantum function ${\psi }_{\mathrm{,}\mu }$. These issues indicate the compatibility of QED Dirac theory. On the other hand, uncorrectable problems hold for the KG charged particle and for the W^± particles of the electroweak theory.

6. Concluding Remarks

This work explains the significance of the distinction between a necessary condition and a sufficient condition [1] that are relevant to relations between physical expressions. The distinction between these concepts is a well-known mathematical element. Specific new necessary conditions for a coherent 4-current of a charged quantum particle are proved in sections 3 and 4 of this work. They are listed below:

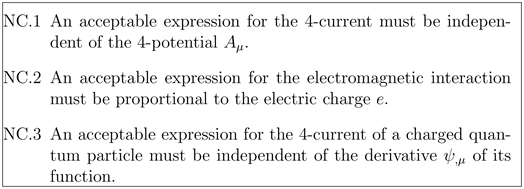
Unfortunately, mainstream QFT textbooks treat the Noether 4-current of a quantum charged particle as a sufficient condition for a coherent 4-current (see e.g. [8] : pp. 308-310). Ignoring the additional necessary conditions for a coherent 4-current that are proved above, is the primary reason for the adoption of erroneous theories like the KG theory [11] and the electroweak theory of the W^± particles [8] [13].

It is interesting to point out that the inherent contradictions of the KG theory that are proved above support Dirac’s lifelong objection to this theory (see [18] : pp. 3-4).

Conflicts of Interest

The author declares no conflicts of interest.

References

[1]	Necessity and Sufficiency. https://en.wikipedia.org/wiki/Necessity_and_sufficiency
[2]	Ye, M.D. (2014) On a Sufficient and Necessary Condition for Graph Coloring. Open Journal of Discrete Mathematics, 4, 1-5. https://doi.org/10.4236/ojdm.2014.41001
[3]	Landau, L.D. and Lifshitz, E.M. (2005) The Classical Theory of Fields, Elsevier, Amsterdam.
[4]	Jackson, J.D. (1975) Classical Electrodynamics. John Wiley, New York.
[5]	Berestetskii, V.B., Pitaevskii, L.P. and Lifshitz, E.M. (1982) Quantum Electrodynamics. Elsevier, Oxford.
[6]	Bjorken, J.D. and Drell, S.D. (1964) Relativistic Quantum Mechanics. McGraw-Hill, New York.
[7]	Weinberg, S. (1995) The Quantum Theory of Fields, Vol. I. Cambridge University Press, Cambridge. https://doi.org/10.1017/CBO9781139644167
[8]	Peskin, M.E. and Schroeder, D.V. (1995) An Introduction to Quantum Field Theory. Addison-Wesley, Reading.
[9]	Bjorken, J.D. and Drell, S.D. (1965) Relativistic Quantum Fields. McGraw-Hill, New York.
[10]	Halzen, F. and Martin, A.D. (1984) Quarks and Leptons, an Introductory Course in Modern Particle Physics. John Wiley, New York.
[11]	Pauli, W. and Weisskopf, V. (1934) über die Quantisierung der skalarenrelativistischenWellengleichung. Helvetica Physica Acta, 7, 709-731. (English Translation: Miller, A.I. (1994) Early Quantum Electrodynamics. Cambridge University Press, Cambridge, 188-205.)
[12]	Comay, E. (2022) Not Even Wrong—A Reexamination. Open Access Library Journal, 9, e9458. https://doi.org/10.9734/psij/2022/v26i130303 https://www.scirp.org/journal/paperinformation.aspx?paperid=121166
[13]	Weinberg, S. (1996) The Quantum Theory of Fields, Vol. II. Cambridge University Press, Cambridge. https://doi.org/10.1017/CBO9781139644174
[14]	Sterman, G. (1993) An Introduction to Quantum Field Theory. Cambridge University Press, Cambridge, 518. https://doi.org/10.1017/CBO9780511622618
[15]	Cottingham, W.N. and Greenwood, D.A. (2007) An Introduction to the Standard Model of Particle Physics. Second Edition, Cambridge University Press, Cambridge. https://doi.org/10.1017/CBO9780511791406
[16]	Aad, G., et al. (2012) ATLAS Collaboration. Physics Letters B, 712, 289.
[17]	Pich, A. (2012) The Standard Model of Electroweak Interactions. https://arxiv.org/abs/1201.0537
[18]	Dirac, P.A.M. (1978) Mathematical Foundations of Quantum Theory. Academic, New York. https://doi.org/10.1016/B978-0-12-473250-6.50005-4