Exact Traveling Wave Solutions for Nano-Solitons of Ionic Waves Propagation along Microtubules in Living Cells and Nano-Ionic Currents of MTs ()
1. Introduction
The nonlinear partial differential equations of mathematical physics are major subjects in physical science [1] . Exact solutions for these equations play an important role in many phenomena in physics such as fluid mechanics, hydrodynamics, optics, and plasma physics. Recently many new approaches for finding these solutions have been proposed, for example, tanh-sech method [2] - [4] , extended tanh-method [5] - [7] , sine-cosine method [8] - [10] , homogeneous balance method [11] [12] , F-expansion method [13] - [15] , exp-function method [16] [17] , trigonometric function series method [18] ,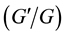 
expansion method [19] - [22] , Jacobi elliptic function method [23] - [26] and so on.
The objective of this article is to apply the extended Jacobian elliptic function expansion method for finding the exact traveling wave solution of nano-solitons of ionic waves propagate on along microtubules in living cells and nano-ionic currents of MTs which play an important role in biology and mathematical physics.
The rest of this paper is organized as follows: In Section 2, we give the description of the extended Jacobi elliptic function expansion method. In Section 3, we use this method to find the exact solutions of the nonlinear evolution equations pointed out above. In Section 4, conclusions are given.
2. Description of Method
Consider the following nonlinear evolution equation
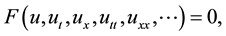(2.1)
where F is polynomial in u(x, t) and its partial derivatives in which the highest order derivatives and nonlinear terms are involved. In the following, we give the main steps of this method [23] - [26] .
Step 1. Using the transformation
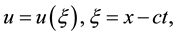(2.2)
where k and c are the wave number and wave speed, to reduce Equation (2.1) to the following ODE:
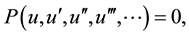(2.3)
where P is a polynomial in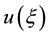 
and its total derivatives, while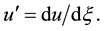 
Step 2. Making good use of ten Jacobian elliptic functions, we assume that (2.3) have the solutions in these forms:
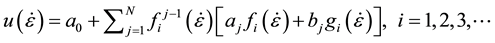(2.4)
with
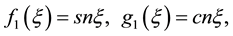
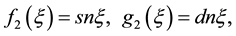
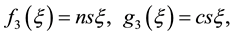
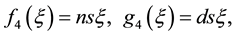
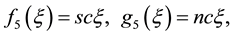
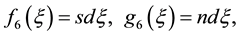(2.5)
where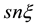
, 
, 
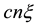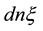, are the Jacobian elliptic sine function. The jacobian elliptic cosinefunction and the Jacobian elliptic function of the third kind and other Jacobian functions which is denoted by Glaisher’s symbols and are generated by these three kinds of functions, namely
![]()
(2.6)
that has the relations
![]()
(2.7)
with the modulus m (0 < m < 1): In addition we know that
![]()
(2.8)
The derivatives of other Jacobian elliptic functions are obtained by using Equation (2.8). To balance the highest order linear term with nonlinear term we define the degree of u as D[u] = n which gives rise to the degrees of other expressions as
![]()
(2.9)
According the rules, we can balance the highest order linear term and nonlinear term in Equation (2.3) so that n in Equation (2.4) can be determined.
In addition we see that when m → 1, ![]()
, ![]()
, ![]()
degenerate as![]()
, ![]()
, ![]()
, respectively, while when therefore Equation (2.5) degenerate as the following forms
![]()
, (2.10)
![]()
, (2.11)
![]()
, (2.12)
![]()
. (2.13)
Therefore the extended Jacobian elliptic function expansion method is more general than sine-cosine method, the tan-function method and Jacobian elliptic function expansion method.
3. Application
3.1. Example 1: Nano-Solitons of Ionic Waves Propagation along Microtubules in Living Cells [27]
We first consider an inviscid, incompressible and non-rotating flow of fluid of constant depth (h). We take the direction of flow as x-axis and z-axis positively upward the free surface ingravitational field. The free surface elevation above the undisturbed depth h is ƞ(x; t), so that the wave surface at height z = h + ƞ(x; t), while z = 0 is horizontal rigid bottom.
Let j(x; z; t) be the scalar velocity potential of the fluidlying between the bottom (z = 0) and free space ƞ(x; t), then we could write the Laplace and Euler equation with the boundary conditions at the surface and the bottom, respectively, as follows:
![]()
(3.1)
![]()
(3.2)
![]()
(3.3)
![]()
(3.4)
It is useful to introduce two following fundamental dimensionless parameters:
![]()
(3.5)
where ![]()
is the wave amplitude, and l is the characteristic length-like wavelength. Accordingly, we also take a complete set of new suitable non-dimensional variables:
![]()
(3.6)
where ![]()
is the shallow-water wave speed, with g being gravitational acceleration.
In term of (3.5) and (3.6) the initial system of Equation (3.1)-(3.4) now reads
![]()
(3.7)
![]()
(3.8)
![]()
(3.9)
![]()
(3.10)
Expanding ![]()
in terms of ![]()
![]()
, (3.11)
and using the dimensionless wave particles velocity in x-direction, by definition![]()
, then substituting of
(3.11) into (3.7)-(3.9), with retaining terms up to linear order of small parameters ![]()
in (3.8), and second order in (3.9), we get
![]()
(3.12)
![]()
(3.13)
Making the differentiation of (3.12) with respect to x, and rearranging (3.13), we get
![]()
(3.14)
![]()
(3.15)
Returning back to dimensional variables ƞ(x; t) and![]()
, (3.14) now reads
![]()
(3.16)
We could define the new function V(x, t) unifying the velocity and displacement of water particles as follows:
![]()
(3.17)
implying that (3.16) becomes
![]()
(3.18)
We seek for traveling wave solutions with moving coordinate of the form ![]()
and with wave speed v, which reduces Equation (3.18) into ordinary nonlinear differential equation as follows:
![]()
(3.19)
Integrating Equation (3.19) once, and setting![]()
, we get
![]()
(3.20)
Balancing ![]()
and ![]()
yields, N + 2 = 2N → N = 2. Therefore, we can write the solution of Equation (3.20) in the form
![]()
(3.21)
![]()
(3.22)
![]()
(3.23)
Substituting (3.21) into (3.23), setting the coefficients of (sn4, sn3, sn3cn, sn2, sn2cn, sncn, sn, cn, sn0) to zero, we obtain the following underdetermined system of algebraic equations for (a0, a1, a2, b1, b2):
![]()
(3.24)
![]()
(3.25)
![]()
(3.26)
![]()
(3.27)
![]()
(3.28)
![]()
(3.29)
![]()
(3.30)
![]()
(3.31)
![]()
(3.32)
Solving the bove system with the aid of Mathematica or Maple, we have the following solution:
![]()
(3.33)
Sothat the solution of Equation (3.20) will be in the form:
![]()
(3.34)
if m →1, we have the hyperbolic solution:
![]()
(3.35)
3.2. Example 2. Nano-Ionic Currents of MTs
The nano ionic currents are elaborated in [27] take the form
![]()
(3.36)
where ![]()
is the resistance of the ER with length, ![]()
, ![]()
is the maximal capacitance of the ER, ![]()
is conductance of pertaining NPs and ![]()
is the characteristic impedance of our system parameters ![]()
and x describe nonlinearity of ER capacitor and conductance of NPs in ER, respectively. In order to solve Equation (3.36) we use the travelling wave transforma-
tions ![]()
with![]()
, to reduce Equation (3.36) to the following non-
linear ordinary differential equation:
![]()
(3.37)
which can be written in the form
![]()
(3.38)
![]()
(3.39)
Thus Equation (3.38) takes the form
![]()
(3.40)
Balancing ![]()
and ![]()
yields,![]()
. Consequently, we get
![]()
(3.41)
where![]()
, ![]()
, ![]()
, ![]()
, ![]()
are arbitrary constants such that ![]()
or![]()
. From Equation (3.41), it is easy to see that
![]()
(3.42)
![]()
(3.43)
Substituting Equations (3.41)-(3.43) into Equation (3.40) and equating the coefficients of![]()
, ![]()
,![]()
, ![]()
, ![]()
, sncndn, sndn, cndn and dn to zero, we obtain
![]()
(3.44)
![]()
(3.45)
![]()
(3.46)
![]()
(3.47)
![]()
(3.48)
![]()
(3.49)
![]()
(3.50)
![]()
(3.51)
![]()
(3.52)
Solving the above system with the aid of Mathematica or Maple, we have the following solution:
Case 1.
![]()
Case 2.
![]()
So that the solution of Equation (3.40) will be in the form:
Case 1.
![]()
(3.53)
Case 2.
![]()
(3.54)
If m → 1, we have the hyperbolic solution:
Case 1.
![]()
(3.55)
Case 2.
![]()
(3.56)
4. Conclusion
The nano waves propagating along microtubules in living cells play an important role in nano biosciences and cellular signaling where the propagation along microtubules shaped as nanotubes is essential for cell motility, cell division, intracellular trafficking and information processing within neuronal processes. Ionic waves propagating along microtubules in living cells have been also implicated in higher neuronal functions, including memory and the emergence of consciousness and we presented an in viscid, incompressible and non-rotating fluid of constant depth (h). The extended Jacobian elliptic function expansion method has been successfully used to find the exact traveling wave solutions of some nonlinear evolution equations. According to the suggested method we obtained a new and more accurate traveling wave solution of nano ionic-solitons waves’ propagation along microtubules in living cells and nano-ionic currents of MTs. Let us compare between our results obtained in the present article with the well-known results obtained by other authors using different methods as follows: Our results of nano-solitons of ionic waves propagation along microtubules in living cells and nano-ionic currents of MTs are new and different from those obtained in [27] . Figures 1-3 show solitary wave
![]()
Figure 1. Plot of solution of Equation (3.35).
![]()
Figure 2. Plot of solution of Equation (3.56).
![]()
Figure 3. Plot of solution of Equation (3.55).
solution. It can be concluded that this method is reliable and proposes a variety of exact solutions NPDEs. The performance of this method is effective and can be applied to many other nonlinear evolution equations.