Simple Landau Model of the Liquid-RII-RI Rotator Phases of Alkanes ()
1. Introduction
During the last two decades much progress has taken place in the field of rotator phases. Rotator phases are among the most interesting condensed states of matter exhibited by normal alkanes (
), alcohols, and other hydrocarbon chain systems consisting of layered structures with three dimensional crystalline order of the center of mass, but no long range orientational order of the molecules about their long axes. Rotator phases have a number of unique and unusual properties which include surface crystallization, anomalous heat capacity, negative thermal compressibilities and unusually high thermal expansions. Five different rotator phases had been identified. The rotator-II (
) phase is usually described as composed of molecules that are untilted with respect to the layers that are packed in a hexagonal lattice. The layers are stacked in an ABCABC... trilayer stacking sequence. This phase is also referred to as the rhombohedral phase. In the rotator-I (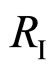
) phase the molecules are also untilted with respect to the layers and there is a rectangularly distorted hexagonal lattice. The layers are stacked in an ABAB... bilayer stacking sequence. This phase is referred to as the face-centered-orthorhombic (FCO) phase. In shorter chain lengths the phase sequence was reported to be Liquid-RII-RI-Crystal.
A large number of experimental studies [1-10] are devoted to describe the structure and the phase transitions of the rotator phases. According to the X-ray scattering study by Sirota et al. [3], the RII-RI transition is first order with jump of the distortion order parameter and sharp peak on the heat capacity data [4]. The binary mixtures of normal alkanes [11-14] also shows a first order character of the RII-RI transition. Sirota et al. [15] carried out a high pressure study on the RII-RI transition and confirmed the first order character of the RII-RI transition. Zammit et al. [7,8] studied the IL-RII and RII-RI transitions in pure and binary mixtures of alkanes. Over the IL-RII transition, they observed the single peak in both the specific heat and latent heat in the pure material, splits into two features at different temperatures. This indicates the first order character of the IL-RII transition. They also confirmed the first order character of the RII-RI transitions. The presence of the thermal hysteresis at these transitions indicates the first order character of the transition.
Theoretical studies of the RII-RI transition follow two main lines. The first approach consists of Monte Carlo and molecular dynamics simulations [16-22] which confirms the first order character of the Liquid-RII (IL-RII) and RII-RI transitions. The second approach is pursued by Wurger [23] and Mukherjee [24-34]. Wurger [23] developed a microscopic model for the pair interaction of hydrocarbon chains and discussed the detailed structure of the RI and RII phases in terms of a molecular-field approximation. In a series of paper Mukherjee [24-26] discussed RII-RI phase transition within Landau phenomenological approach and discussed in detail the various aspect of this transition including the elastic properties.
To the best of the author’s knowledge, there is so far no detailed theoretical studies on the IL-RII-RI phase sequence and the transitions between them. The purpose of the present paper is to investigate the IL-RII-RI phase sequence and the transitions between them within Landau theory. We define a new order parameter to describe the IL-RII and IL-RI phase transitions.
2. Model
Order Parameters
The RI phase differs from the RII phase only in the distortion of the hexagonal lattice. Following [5,35] we define the lattice distortion parameter
where a and b are the major and minor axes of an ellipse draws through the six nearest neighbors. The distortion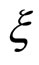 
is defined with respect to a plane whose normal is parallel to the long molecular axes. 
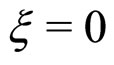for the RII phase. Thus we take 
as an order parameter for the RII-RI transition.
Now to define the order parameter of the IL-RII or IL-RI transitions. The low temperature crystal phase of n-alkanes is found to exist in layered structures. In these cases the molecules stay in some layer stacking and the probability that a single molecule is present (partially) in two simultaneous layers is almost zero. When the temperature rises further the stacking breaks, i.e. the molecules start occupying positions which are shared by more than one layer stacking. We may choose to represent alternate layer sequences with suffixes 
etc. The bilayer stacking can be represented as 
and the trilayer stacking can be represented as 
We represent the probability of the k-th molecule of the system to occupy any of the j-th layer sequence as
. For bilayer the only feasible cases are 
and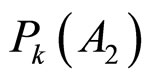
, one of which is 1 and the other being 0 in perfect layer ordering. Now we define a correlation factor 
for the k-th molecule in a N-layer stacking as
(1)
This is clearly 0 in ordered layer phase and non-zero in other phases with lower or higher order.
The rotator phases of n-alkanes are found to exist in bilayer and trilayer structures. If we consider the correlation factor for the highest possible layer structure in the alkanes, i.e. trilayer we find that for the k-th molecule
(2)
It is 0 (or almost zero) in RII and RI phases which in nature are bilayer and trilayer respectively. 
does have a finite value in the liquid phase which can be calculated. In isotropic liquid phase the probability density of the k-th molecule is constant everywhere in space. So,
,
is the total number of layers. Hence 
can be expressed as
(3)
Thus 
is independent of k. Hence we define the correlation order parameter 
as
(4)
Here the average correlation factor is calculated for all molecules together.
Thus 
is 0 is the isotropic liquid phase and has a value >0 in the RI and RII phases.
3. Free Energy
Thus we take 
and 
as two order parameters involved in the RI-RII, IL-RII and IL-RI phase transitions. For simplicity we neglect the weak interlayer interaction between the stacking layers in different rotator phases so that the problem becomes two dimensional. The distortion is a two component order parameter; its components are expressed the distortion amplitude 
and the azimuthal angle
. The multiplier 2 comes from the fact that the distortion is a symmetric traceless tensor. Since the the free energy is a scalar quantity, negative and positive 
and 
result in inequivalent structures, causing the Landau free energy expansion contain 
and 
terms, thus resulting in a first order transition. Expanding the total free energy in terms of the above mentioned order parameters yields
(5)
where 
is free energy of the isotropic liquid phase. The coefficients a and 
are assumed to vary strongly with an external parameter. For
, free energy (5) describe a first order RI-RII transition for b > 0 and c > 0. In this case the minimum free energy occurs at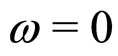
, for b > 0 and at 
for b < 0. According to the experimental observations, in the RI phase,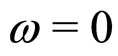
. H is the coupling constant.
, 
, b, c and H are chosen positive.
The material parameters a and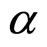 
can be assumed as 
and
. 
and 
are virtual transition temperatures. 
and 
are constants.
From the experimental phase diagrams [3] one observes, 
and 
can be portrayed as
and
where 
is some specific chain length of the molecules. u and v are positive constants.
Minimization of Equation (5) with respect to 
and 
yields the following phases:
1) Isotropic liquid (IL) phase: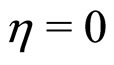
,
.
This phase exists for 
and a > 0.
2) RII phase:
,
.
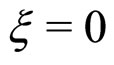The RII phase exists when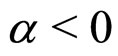 
and a > 0.
3) RI phase:
,
.
The RI phase exists for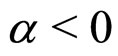 
and a < 0.
Thus it is clear from the solutions that three types of transition are possible: 1) IL-RII; 2) IL-RI; 3) RII-RI.
The sufficient condition for the RI phase to be stable are
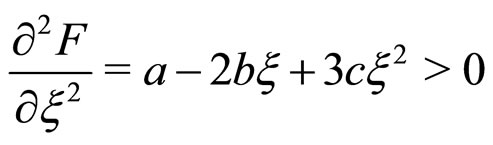(6)
(7)
(8)
These three conditions determine the stability of the RI phase explicitly. The sufficient condition for the stability of the RII phase reads
(9)
For the IL phase the stability conditions are 
and a > 0.
By lowering the temperature from the isotropic liquid phase, the RII and RI phases can appear sequentially or in partial sequence. The RII and RI phases can arise either directly from the IL phase along the curves IL-RII and IL-RI or along the curve RII-RI. In the spirit of Landau theory all the phase transitions IL-RII, IL-RI and RII-RI are first order because of the cubic invariant in the free energy expansion. If all the phase transitions involved are first order ones, then one can observe the IL-RII-RI triple point as observed in experiment [3].
The conditions for the first order RII-RI transition can be obtained as
(10)
The conditions for the first order IL-RI transition are given by
(11)
The conditions for the first order IL-RII transition read
(12)
Solving (10)-(12) simultaneously will determine the various phase transition lines. Figure 1 shows a typical phase diagram for the IL-RI, IL-RII and RII-RI phase transitions. As can be seen from the Figure 1, the RII and RI phases arise from the isotropic phase along the curves IL-RII and IL-RI or along the curve RII-RI respectively. The IL-RII and IL-RI transitions are first order because of the cubic invariant in the free energy expansion. The line of the RII-RI transition starts at the IL-RII-RI triple point as shown in Figure 1. When the temperature of the IL-RII and of RII-RI transitions coincide, a triple point appears.
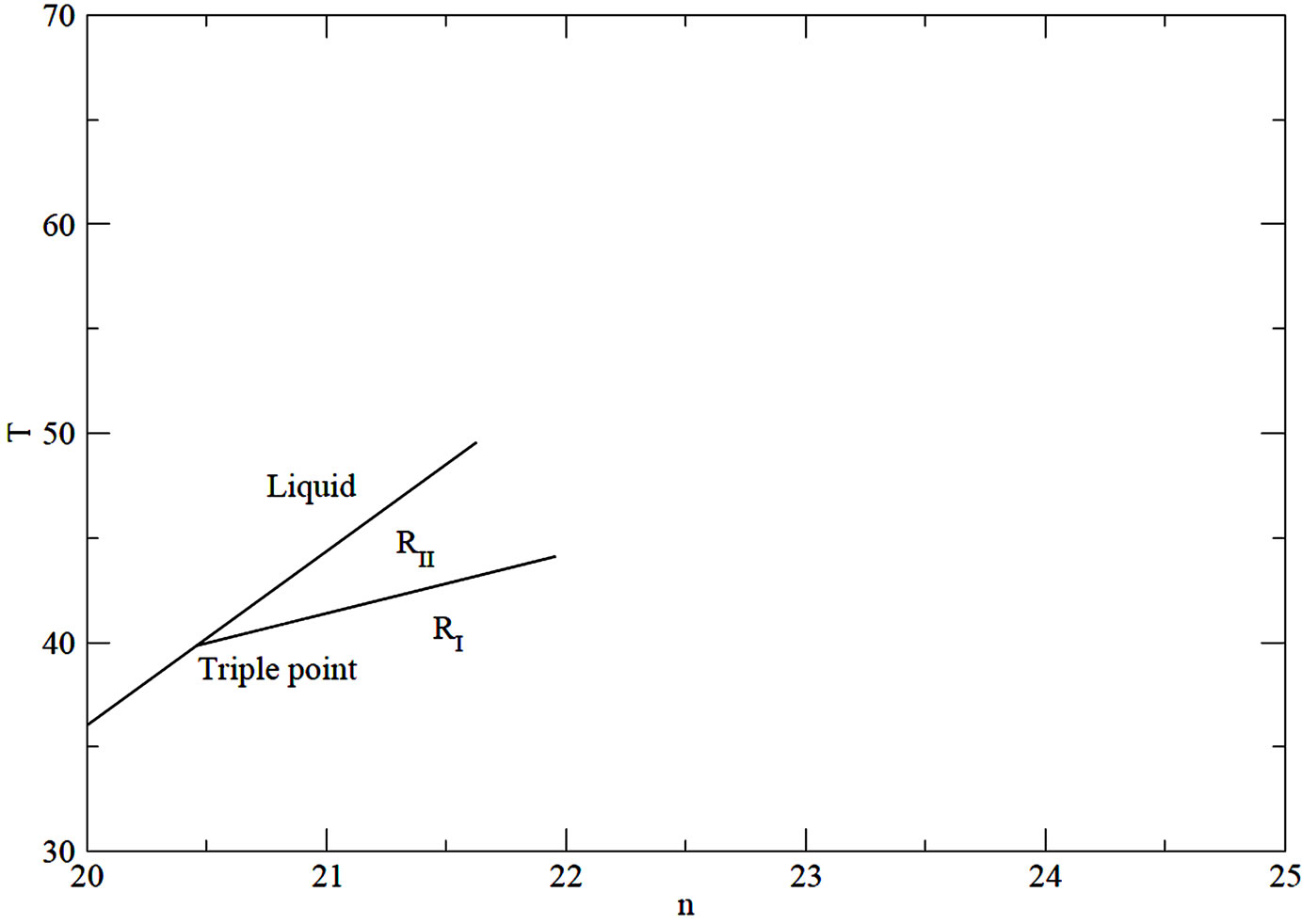
Figure 1. Possible chain length (n)-temperature (T) phase diagram in the vicinity of the Liquid-RII-RI triple point.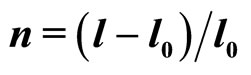
.
The region of the RI phase shrinks and finally disappears when the IL-RII transition takes place. In experimental studies [3], alkanes C20-C27 show such a IL-RII transition via triple point. Thus the above analysis of the IL-RII-RI triple point agrees well with the experimental observations [3]. Thus there is always a direct IL-RII transition is possible within the framework of our model free energy (6) satisfying the above stability conditions. Of course the IL-RI transition could proceed before the IL-RII the transition temperature 
is reached. To prevent this, 
has to be larger than the IL-RI transition temperature 
Thus we have always 
. The cubic coefficient 
in the free energy (5) yields a first order IL-RII transition at
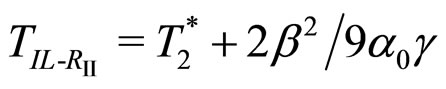
with an order parameter jump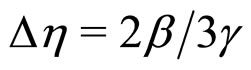 
and a latent heat of
. The so-called temperature hysteresis is related to the existence of metastable states within a certain temperature range. The above analysis qualitatively agrees with experimental observations[3,4,7,8].
4. Conclusion
A simple model free energy has been constructed to describe the IL-RII-RI phase sequence and transitions between them. The order parameters are identified for different phase transitions. The model predicts the first order character of the IL-RII, IL-RI and RII-RI transitions and IL-RII-RI triple point in the 
phase diagram. The proposed interpretation of the IL-RII transition allow us to explain the various types of phase behavior observed experimentally. These results are in qualitative agreement with all experiments reported so far.
5. Acknowledgements
PKM thanks the Alexander von Humboldt Foundation for equipment and book grant.
NOTES