Plasmas Created in the Interaction of Antiprotons with Atomic and Ionized Hydrogen Isotopes. Suggested Fuels for Space Engines

Mohamed Assaad Abdel-Raouf^1*, Abdelfattah T. Elgendy¹, Amr Abd Al-Rahman Youssef²

¹Physics Department, Faculty of Science, Ain Shams University, Cairo, Egypt.
²Mathematics Department, Faculty of Science, Al-Azhar University, Cairo, Egypt.

DOI: 10.4236/jhepgc.2022.81002                

Abstract

The main objective of the present work is to investigate the properties of plasmas created by injecting a thermalized beam of antiprotons in two types of media. The first is hydrogen, deuterium, or tritium atoms localized in palladium crystals. The second medium is composed of protons, deuterons, or tritons localized in a magnetic cavity. Particularly, it is demonstrated that huge amounts of energy are released in both cases which could be used as fuels for space shuttle engines. A novel mathematical scheme is employed to calculate the energy yields in real space at different incident energies of the antiprotons.

Keywords

Antiprotons, Antiprotonic Hydrogens, Antiprotonic Plasma, Fuel for Space Engines, Plasmas in Molecular Crystals, Palladium as Host for Neutral Plasma, Antiprotons-Ionized Hydrogen Isotopes Plasma

1. Introduction

With the discovery of antiprotons (p) by Chamberlain et al. [1] at Fermilab in the fifties of the preceding century, the ultimate proof of possible formation of antiparticles in laboratory was rigorously confirmed. Consequently, Dirac’s theory of holes [2] was acknowledged [3] as one of the fundamental theories of particle physics. The interest in the formation of cold antiprotons was demonstrated through the development of the “Low Energy Antiproton Ring (LEAR)” at CERN [4], in the early eighties of the preceding century which led in 1995 to the formation of the first antiatom in laboratory, namely the ANTIHYDROGEN by the ATHEN experiment [5] [6] (For more study about Antihydrogen see [7] [8] [9] ). Other interesting experiments were performed after the development of the ATRAP machine at CERN ( [10] [11] [12] ) most of them seeking on the one hand, the production of large number of antihydrogen atoms, protoniums and protonium ions [13]. On the other hand, efforts were made to test the behavior of antimatter from the gravity point of view [14]. Recently, several applications of matter-antimatter interactions at low energy were explored ( [15] [16] [17] ).

Experimental investigations of plasmas involving antiprotons were carried out at the AEgIS, (for a review see [18] [19] ). In this case nonneutral plasmas were studied in detail [20]. Formation of Matter-antimatter plasma, particularly high energetic electron-positron plasms, was also proposed as plausible explanation for the mysterious radiation emitted from pulsers. The motive of the present work is the experimentally confirmed fact that matter-antimatter annihilation occurring by magnetically confined plasma releases much higher energy per unit mass comparative to any other source of propulsion [20] (For a comprehensive account on plasma theory and applications see [21] - [28] ).

Our present work is concerned with two problems. The first is the formation of plasma through the interaction of antiprotons with highly populated hydrogen, deuterium or tritium atoms localized in a material host (e.g., palladium crystal). The second problem is to study plasmas formed by injecting a beam of antiprotons in a magnetic trap filled with protons, deuterons, or tritons.

The main objective of our investigations is to show that huge number of energies could be released from the plasmas created in both cases. Particularly, the employment of this plasma as fuel for engines of space shuttles is strongly emphasized.

The present paper falls in other three sections followed by a complete list of references mentioned in the text. The next section deals with the mathematical formalism of our problems. In Section 3 the results of our calculations are displayed and discussed. In Section 4 the main conclusions drawn from our investigations are presented. The paper concludes with the list of references mentioned in the text.

2. Mathematical Formalism

The present section is split into two parts, the first is devoted to the treatment of antiproton-atom plasmas and the second deals with the antiproton-nucleon plasmas.

2.1. Antiproton-Atom Plasmas

Consider a plasma which is composed of antiprotons (P^-), electrons (e⁻) and hydrogen ions (H⁺), (deuteron ions or triton ions, i.e., D⁺ or T⁺, respectively). For simplicity, it is assumed that the fluid of particles is forming an ideal gas.

Hence, the pressure of each stream is defined by $p_{\alpha }=\frac{3}{2}{k}_B{T}_{\alpha }n$, where $p_{\alpha }$ ; $T_{\alpha }$ ;

$n_{\alpha }$ are, respectively, pressure, the temperature, and density of the particle gas, whilst k_B is Boltzmann constant. The model is explained by the fluid equations.

The continuity equation for particles is given by.

$\frac{\partial n_{\alpha }}{\partial t}+\frac{\partial }{\partial x}\left(n_{\alpha }{u}_{\alpha }\right)=0.$ (1)

The equation of motion for particle has the form.

$\frac{\partial u_{\alpha }}{\partial t}+u_{\alpha }\frac{\partial u_{\alpha }}{\partial x}+\frac{1}{m_{\alpha }{n}_{\alpha }}\frac{\partial p_{\alpha }}{\partial x}+\frac{q_{\alpha }}{m_{\alpha }}\frac{\partial \varphi }{\partial x}=0$, (2)

where α stands for e⁻; P^-; H⁺; D⁺; or T⁺.

Poisson’s equation is expressed by

$\frac{{\partial }^2\varphi }{\partial x^2}=\frac{e}{ϵ}\left(n_{P^{-}}+n_e-n_i\right)$ (3)

where m_α; u_α; and q_α denote, respectively, the mass, velocity and charge of the particle α in the stream. $\varphi$ is the electrostatic potential and the index i refers to H⁺; D⁺; or T⁺. The fluid system of Equations (1)-(3) are normalized by the dimensionless variables $x\to {\omega }_{i}x/C_s$ ; $t\to {\omega }_{i}x/C_s$ ; $N=n/n_0$ ; $U_{\alpha }=u_{\alpha }/C_s$ and $\varphi =e\varphi /2k_B{T}_e$ ; where ${\omega }_i$ is the plasma frequency of particle and n₀ is the

equilibrium density, and ${\mathcal{C}}_s=\sqrt{\frac{2k_B{T}_e}{m_i}}$ is the acoustic wave speed and T_e is the

electron temperature respectively. Thus, the normalized system is described by the equations.

$\frac{\partial N_{\alpha }}{\partial t}+\frac{\partial }{\partial x}\left(N_{\alpha }{U}_{\alpha }\right)=0$ (4)

$\begin{array}{l}\frac{\partial U_{\alpha }}{\partial t}+U_{\alpha }\frac{\partial U_{\alpha }}{\partial x}+{\nu }_{\alpha 0}{N}_{\alpha }^1\frac{\partial N_{\alpha }}{\partial x}+{\nu }_{\alpha 1}\frac{\partial \Phi }{\partial x}=0\\ \frac{{\partial }^2\Phi }{\partial x^2}=N_{P^{-}}+N_e-N_i\end{array}$ (5)

where ${\nu }_{\alpha 0}=\frac{3}{4}{M}_{i\alpha }{\tau }_{e\alpha }$, ${\nu }_{\alpha 1}=Q_{\alpha }{M}_{i0}$ ; $M_{i\alpha }=m_i/m_{\alpha }$, ${\tau }_{e\alpha }=T_{\alpha }/T_e$ and

$Q_{\alpha }=q_{\alpha }/e$ with $Q_{e,p^{-}}=-1$ ; and $Q_i=1$. Stretching coordinates are defined by $\xi ={\epsilon }^{1/2}\left(x-vt\right)$ ; and $\tau ={\epsilon }^{3/2}vt$ ; and

$\begin{array}{l}{N}_{\alpha }=N_{\alpha 0}+\epsilon N_{\alpha 1}+{\epsilon }^2{N}_{\alpha 2}+\cdots ,U_{\alpha }=U_{\alpha 0}+\epsilon U_{\alpha 1}+{\epsilon }^2{U}_{\alpha 2}+\cdots \\ \text{and}\Phi =\epsilon {\Phi }_1+{\epsilon }^2{\Phi }_2+\cdots \end{array}$ (6)

$N_{i0}=N_{P^{-}0}+N_{e0}$ (7)

$\frac{\partial {\Phi }_1}{\partial \tau }+2A_1{\Phi }_1\frac{\partial {\Phi }_1}{\partial \xi }+B_1\frac{{\partial }^3{\Phi }_1}{\partial {\xi }^3}=0$ (8)

and

$h_{p^{-}0}+h_{e0}-h_{i0}=0.$ (9)

Hence, the solution of kdv Equation (8) and the first order perturbation of velocity and density of particle are given by

$\begin{array}{l}{\Phi }_1=\frac{3v}{2A_1}{\text{sech}}^2\left(\sqrt{v/4B_1}Y\right)\\ U_{\alpha 1}=\frac{3{\nu }_{\alpha 1}v}{2A_1}\left(v-U_{\alpha 0}\right){\eta }_{\alpha 0}{\text{sech}}^2\left(\sqrt{v/4B_1}Y\right)\\ N_{\alpha 1}=\frac{3{\nu }_{\alpha 1}v}{2A_1}{N}_{\alpha 0}{\eta }_{\alpha 0}{\text{sech}}^2\left(\sqrt{v/4B_1}Y\right)\end{array}$ (10)

where, $A_1=\frac{h_{i2}-h_{p^{-2}}-h_{e2}}{h_{i1}-h_{p^{-}1}-h_{e1}}$, $B_1=\frac{1}{h_{i1}-h_{p{r}^{-}1}-h_{e1}}$, $h_{\alpha 1}=2v{\eta }_{a0}\left(v-U_{\alpha 0}\right)h_{\alpha 0}$, $h_{\alpha 2}=\frac{{\nu }_{\alpha 1}}{2}{\eta }_{\alpha 0}^2{h}_{\alpha 0}\left(3{\left(v-U_{\alpha 0}\right)}^2-{\nu }_{\alpha 0}\right)$, $h_{\alpha 0}=\frac{{\nu }_{\alpha 1}{N}_{\alpha 0}}{{\left(v-U_{\alpha 0}\right)}^2-{\nu }_{\alpha 0}{N}_{\alpha 0}^2}$, ${\eta }_{\alpha 0}=\frac{1}{{\left(v-U_{00}\right)}^2-{\nu }_{00}}$ and $Y=\xi -v\tau$. The normalized plasma kinetic energy of zero and first perturbations for velocities and densities is.

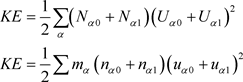
2.2. Antiproton-Nucleon Plasma

Consider a system of fluid plasma consisting of two kinds of oppositely charged particles, namely negatively charged antiprotons (P^-) and positively charged Protons (P), Deuterons (D) or Tritons (T). Furthermore, assume that all plasmas are ideal gases. Consequently, the pressure of each plasma is calculated by $p_{\alpha }=\frac{3}{2}{k}_B{T}_{\alpha }n$, where $p_{\alpha }$, $T_{\alpha }$, and $n_{\alpha }$ stand, respectively, for the pressure, temperature, and density of particle stream, whilst k_B is Boltzmann constant. The model is explained by the following system of equations.

The continuity equation

$\frac{\partial n_{\alpha }}{\partial t}+\frac{\partial }{\partial x}\left(n_{\alpha }{u}_{\alpha }\right)=0$, (13)

the equation of motion

$\frac{\partial u_{\alpha }}{\partial t}+u_{\alpha }\frac{\partial u_{\alpha }}{\partial x}+\frac{1}{m_{\alpha }{n}_{\alpha }}\frac{\partial p_{\alpha }}{\partial x}+\frac{q_{\alpha }}{m_{\alpha }}\frac{\partial \varphi }{\partial x}=0$, (14)

with α = P^-, P, D, T) and

Poisson’s equation

$\frac{{\partial }^2\varphi }{\partial x^2}=\frac{e}{ϵ}\left(n_{P^{-}}-n_i\right)$ (15)

where m_α, u_α, and q_α are the mass, velocity, and charge of the particle α. $\varphi$ is the electrostatic potential and the index i refers to P, D, or T.

The Plasma system of Equations (1)-(3) are normalized by the dimensionless variables $x\to {\omega }_{i}x/C_s$, $t\to {\omega }_{i}x/C_s$, $N=n/n_0$, $U_{\alpha }=u_{\alpha }/C_s$ and

$\varphi =e\varphi /2k_B{T}_e$, where ${\omega }_i$ is the plasma frequency of the particlei and n₀ is the equilibrium density, and ${\mathcal{C}}_s=\sqrt{\frac{2k_B{T}_e}{m_i}}$ is the acoustic wave speed and T_e is the electron temperature respectively. Thus, the normalized system is described by.

$\begin{array}{l}\frac{\partial N_{\alpha }}{\partial t}+\frac{\partial }{\partial x}\left(N_{\alpha }{U}_{\alpha }\right)=0\\ \frac{\partial U_{\alpha }}{\partial t}+U_{\alpha }\frac{\partial U_{\alpha }}{\partial x}+{\nu }_{\alpha 0}{N}_{\alpha }^{-1}\frac{\partial N_{\alpha }}{\partial x}+{\nu }_{\alpha 1}\frac{\partial \Phi }{\partial x}=0\\ \frac{{\partial }^2\Phi }{\partial x^2}=N_{P^{-}}-N_i\end{array}$ (16)

where ${\nu }_{\alpha 0}=\frac{3}{4}{M}_{i\alpha }{\tau }_{e\alpha }$, ${\nu }_{\alpha 1}=Q_{\alpha }{M}_{i0}$ ; $M_{i\alpha }=m_i/m_{\alpha }$, ${\tau }_{e\alpha }=T_{\alpha }/T_e$ and $Q_{\alpha }=q_{\alpha }/e$ with $Q_{e,p^{-}}=-1$, and $Q_i=1$. The stretching coordinates are defined by.

$\xi ={\epsilon }^{1/2}\left(x-vt\right)$,

and

$\tau ={\epsilon }^{3/2}vt$,

and

$\begin{array}{l}{N}_{\alpha }=N_{\alpha 0}+\epsilon N_{\alpha 1}+{\epsilon }^2{N}_{\alpha 2}+\cdots ,U_{\alpha }=U_{\alpha 0}+\epsilon U_{\alpha 1}+{\epsilon }^2{U}_{\alpha 2}+\cdots \\ \text{and}\Phi =\epsilon {\Phi }_1+{\epsilon }^2{\Phi }_2+\cdots \end{array}$

$N_{i0}=N_{P^{-}0}$

$\frac{\partial {\Phi }_1}{\partial \tau }+2A_1{\Phi }_1\frac{\partial {\Phi }_1}{\partial \xi }+B_1\frac{{\partial }^3{\Phi }_1}{\partial {\xi }^3}=0$ (20)

and

$h_{P-0}-h_{i0}=0$. (21)

Hence, the solution of kdv Equation (8) and the first order perturbation of the velocity and density of the particle are given by

$\begin{array}{l}{\Phi }_1=\frac{3v}{2A_1}{\text{sech}}^2\left(\sqrt{v/4B_1}Y\right)\\ U_{\alpha 1}=\frac{3{\nu }_{\alpha 1}v}{2A_1}\left(v-U_{a0}\right){\eta }_{\alpha 0}{\text{sech}}^2\left(\sqrt{v/4B_1}Y\right)\\ N_{\alpha 1}=\frac{3{\nu }_{\alpha 1}v}{2A_1}{N}_{\alpha 0}{\eta }_{\alpha 0}{\text{sech}}^2\left(\sqrt{v/4B_1}Y\right)\end{array}$ (22)

$A_1=\frac{h_{i2}-h_{P-2}}{h_{i1}-h_{P-1}},B_1=\frac{1}{h_{i1}-h_{P-1}},h_{\alpha 1}=2v{\eta }_{\alpha 0}\left(v-U_{\text{a}0}\right)h_{\alpha 0}.$

$h_{\alpha 2}=\frac{{\nu }_{\alpha 1}}{2}{\eta }_{a0}^2{h}_{a0}\left(3{\left(v-U_{a0}\right)}^2-{\nu }_{o0}\right)$, $h_{\alpha 0}=\frac{{\nu }_{\alpha 1}{N}_{a0}}{{\left(v-U_{a0}\right)}^2-{\nu }_{\alpha 0}{N}_{\alpha 0}^2}$,

${\eta }_{\alpha 0}=\frac{1}{{\left(v-U_{\alpha 0}\right)}^2-{\nu }_{\alpha 0}}$ and $Y=\xi -v\tau$.

The normalized kinetic energies of the plasma corresponding to the zero and first order perturbations of the velocities and densities are determined by

$\begin{array}{l}KE=\frac{1}{2}\underset{\alpha }{{\displaystyle \sum }}\left(N_{\alpha 0}+N_{\alpha 1}\right){\left(U_{\alpha 0}+U_{\alpha 1}\right)}^2\\ KE=\frac{1}{2}{\displaystyle \sum m_{\alpha }\left(n_{\alpha 0}+n_{\alpha 1}\right){\left(u_{o0}+u_{\alpha 1}\right)}^2}\end{array}$.

3. Results and Discussion

As shown in tables. The following values of particle masses have been employed in the present work.

Proton mass m_P = 1.6726231 × 10²⁷ kg, Deuteron mass m_D = 3.3476 × 10⁻²⁷ kg; and triton mass m_T = 5.0225 × 10⁻²⁷ kg, $M_{\bar{p}}$ = M_P = 1.0073 × 10⁻⁶ g, M_D = 2.0160 × 10⁻⁶ g, M_T = 3.0246 × 10⁻⁶ g, $M_{e^{-}}$ = 5.4858 × 10⁻² g, $M_{PP{e}^{-}}$ = 3.02245 × 10⁻⁶ g; $M_{DP{e}^{-}}$ = 5.03985 × 10⁻⁶ g; $M_{TP{e}^{-}}$ = 7.05705 × 10⁻⁶ g.

Normalized kinetic energy (KE) against Y for electrons, antiprotons and hydrogen, deuterium, or tritium are demonstrated in Figures 1-3 and for antiprotons and protons, deuterons, and tritons are displayed in Figures 4-6.

Data Availability Statements

The data of the calculated energy that support the findings of this study are derivative in at https.//doi.org/10.1016/j.physleta.2014.10.006, reference number [22] [23].

Table 1. Stretching velocity for protons, deuterons, or tritons with electrons and antiprotons for different velocities of antiprotons, u_e = C_s, u_i = 9 × 10⁷C_s; N_e = 1, N_P = 1, and N_i = 2.

Table 2. Frame stretching velocity for protons, deuterons, or tritons with electrons and for different velocities of antiprotons u_i = 9 × 10⁷C_s; N_P = 1; and N_i = 1.

Table 3. The non-normalized kinetic energy KE (J) of Plasma in Palladium for n₀ = 6.0221367 × 110²³ mol⁻¹; ε = 13.7; u_i = 9 × 10⁷C_s; u_e = C_s, (H; D; or T, with P; e) Y = 0 at T_e (K) = 273.

Figure 1. u_P = 0.9C_s; P = 1487.2.

Figure 2. u_P = 0.09C_s; P = 14.872.

Figure 3. u_P = 0.009C_s; P = 0.14872.

Table 4. For (P; D; or T, with P) Y = 0.

Figure 4. u_P = 0.9C_s; P = 1487.2.

Figure 5. u_P = 0.09C_s; P = 14.87.

Table 5. For T_e (K) = 300, 320, 340, 360.

Figure 6. u_P = 0.009C_s; P = 0.14872.

4. Conclusion

The one-dimensional hydrodynamic model of linear and nonlinear analyses in the term of electron-positron and proton-antiproton are studied. The linear analyses show a positive root that indicates ion-acoustic waves in the system and the possibility of existing soliton waves. Also, we obtain the calculations of the nonlinear analysis of the deformed kdv equation and the energy released of the formed plasma. The results, see Tables 1-5 and Figures 1-6, show that the lifetime of formed plasma increases inside the palladium crystal 13.7 times concerning the free space. We have the same energy of formed plasma in the case of free space and palladium crystal. The renormalized example study assumes that when the velocity of the antiproton is around the ion-acoustic wave the released energy of the plasma could be reached to 1.15723 × 10⁶ J/mole. On the other hand, if the velocity of the antiproton decreases, the total energy of the plasma system decreases. This result supports very much the conclusion that we suggested model for obtaining huge amount of energy by constructing a plasma state between thermalized hydrogen and antihydrogen in molecular crystals with approximate velocity less than the velocity of ion acoustic wave of the system. This energy could find interesting application in cold fusion and building up engines for space shuttles.

Conflicts of Interest

The authors declare no conflicts of interest regarding the publication of this paper.

References

[1]	Chamberlain, O., Segrè, E., Wiegand, C. and Ypsilantis, T. (1955) Observation of Antiprotons. Physical Review Journals Archive, 100, 947-950. https://doi.org/10.1103/PhysRev.100.947
[2]	Dirac, P.A.M. (1930) A Theory of Electrons and Protons. Proceedings of the Royal Society A, 126, 360-365. https://doi.org/10.1098/rspa.1930.0013
[3]	Dirac, P.A.M. (1931) Quantized Singularities in the Electromagnetic Field. Proceedings of the Royal Society A, 133, 60-72. https://doi.org/10.1098/rspa.1931.0130
[4]	Hardt, W., Lefevre, P., Mohl, D. and Plass, G. (1980) The CERN Low Energy Antiproton Ring (LEAR) Project. 11th International Conference on High-Energy Accelerators, Geneva, 7-11 July 1980, 819-824. https://doi.org/10.1007/978-3-0348-5540-2_119
[5]	Baur, G., et al. (1996) Production of Antihydrogen. Physics Letters B, 368, 251-258. https://doi.org/10.1016/0370-2693(96)00005-6
[6]	Blanford, G., et al. (1998) Observation of Atomic Antihydrogen. Physical Review Letters, 80, 3037-3040. https://doi.org/10.1103/PhysRevLett.80.3037
[7]	Amoretti, M., et al. (2002) Production and Detection of Cold Antihydrogen Atoms. Nature, 419, 456-459. https://doi.org/10.1038/nature01096
[8]	Gabrielse, G., et al. (2002) Background-Free Observation of Cold Antihydrogen with Field-Ionization Analysis of Its States. Physical Review Letters, 89, Article ID: 213401. https://doi.org/10.1103/PhysRevLett.89.213401
[9]	Amoretti, M., et al. (2002) Positron Plasma Diagnostics and Temperature Control for Antihydrogen Production. Physical Review Letters, 91, Article ID: 055001. https://doi.org/10.1103/PhysRevLett.91.055001
[10]	Gabrielse, G., et al. (2002) Stacking of Cold Antiprotons. Physics Letters B, 548(3-4), 140-145. https://doi.org/10.1016/S0370-2693(02)02850-2
[11]	Bonomi, G., et al. (2005) Antihydrogen Production Mechanisms in ATHENA. Nuclear Physics A, 752, 97-100.
[12]	Amoretti, M., et al. (2004) Antihydrogen Production Temperature Dependence. Physics Letters B, 583(1-2), 59-67.
[13]	Zurlo, N., et al. (2002) Production of Slow Protonium in Vacuum. Hyperfine Interactions, 172, 97-105. https://doi.org/10.1007/s10751-007-9529-0
[14]	Rizzini, L. (2012) Further Evidence for Low Energy Protonium Production in Vacuum. The European Physical Journal Plus, 127, Article No. 124. https://doi.org/10.1140/epjp/i2012-12124-9
[15]	Alpha Collaboration (2020) Investigation of the Fine Structure of Antihydrogen. Nature, 578, 375-380. https://doi.org/10.1038/s41586-020-2006-5
[16]	Abdel-Raouf, M.A. (2009) Matter-Antimatter Physics at Low Energy. Journal of Physics: Conference Series, 1253, Article ID: 12006.
[17]	Abdel-Raouf, M.A. (2020) Novel Consequences of Coexistence of Matter and Antimatter in Nature. Journal of High Energy Physics, Gravitation and Cosmology, 6, 251-258. https://doi.org/10.4236/jhepgc.2020.62019
[18]	Aghion, S., et al. (2018) Compression of a Mixed Antiproton and Electron Non-Neutral Plasma to High Densities. The European Physical Journal D, 72, Article No. 76.
[19]	Higaki, H. (2005) On the Possibility of Non-Neutral Antiproton Plasmas and Antiproton-Positron Plasmas. AIP Conference Proceedings, 793, Article No. 351. https://doi.org/10.1063/1.2121997
[20]	Lapointe, M. (1991) Antiproton Powered Propulsion with Magnetically Confined Plasma Engines. Journal of Propulsion and Power, 7, 749-759. https://doi.org/10.2514/3.23388
[21]	Elgendy, A.T. (2021) A Global Model of the Collisional Plasma Boundary Sheath Using Step Model. IEEE Transactions on Plasma Science, 49, 711-717. https://doi.org/10.1109/TPS.2021.3049302
[22]	El-Tantawy, S.A., Elgendy, A.T. and Ismail, S. (2017) Cylindrical Freak Waves in a Non-Maxwellian Dusty Bulk-Sheath Plasma. An Approximate Solution for the Cylindrical Nonlinear Schrödinger Equation. Physics Letters A, 381, 3465-3471. https://doi.org/10.1016/j.physleta.2017.08.054
[23]	El-Tantawy, S.A., Ali Shan, S., Akhtar, N. and Elgendy, A.T. (2018) Impact of Electron Trapping in Degenerate Quantum Plasma on the Ion-Acoustic Breathers and Super Freak Waves. Chaos, Solitons & Fractals, 113, 356-364. https://doi.org/10.1016/j.chaos.2018.04.037
[24]	Elgendy, A.T. (2019) Plasma Boundary of Nonlinear Sheath Dynamics for Arbitrary Waveforms in Capacitive Discharge. Journal of Physics: Conference Series, 1253, Article ID: 012010. https://doi.org/10.1088/1742-6596/1253/1/012010
[25]	Kouser, S., Shah, K.H., Qureshi, M.N.S. and Shah, H.A. (2020) Nonlinear Ion-Acoustic Waves in e-p-i Plasmas with (r, q) Distributed Electrons and Positrons. AIP Advances, 10, Article ID: 55123. https://doi.org/10.1063/5.0011128
[26]	Malik, H.K., Singh, S. and Dahiya, R.P. (1994) Kadomtsev-Petviashvili Solitons in Inhomogeneous Plasmas with Finite Temperature Drifting Ions. Physics Letters A, 195, 369-372. https://doi.org/10.1016/0375-9601(94)90044-2
[27]	Singh, S. and Honzawa, T. (1993) Kadomtsev-Petviashivili Equation for an Ion-Acoustic Soliton in a Collisionless Weakly Relativistic Plasma with Finite Ion Temperature. Physics of Fluids B: Plasma Physics, 5, 2093-2097. https://doi.org/10.1063/1.860745
[28]	Petrov, A.E., Sarksyan, K.A., Skvortsova, N.N. and Kharchev, N.K. (2001) Observation of Nonlinear Coupling between Drift and Ion-Acoustic Oscillations in Low-Frequency Plasma Turbulence. Plasma Physics Reports, 27, 56-61. https://doi.org/10.1134/1.1339484