Negative Magnetoresistance Behaviour and Variable Range Hopping Conduction in Insulating NbSi Amorphous Alloys at Very Low Temperature with aagnetic Field ()
1. Introduction
For few decades, the negative magnetoresistance (NMR) of doped semiconductors has been the subject of investigations, as much in the metallic regime [1-3] as in insulating systems [4,5]. The most recent theories are based on the quantum corrections, which are enhanced in the neighbourhood of the Metal-Insulator Transition (MIT). The quantum interference models seem to describe accurately the NMR of metallic materials [6,7].
For insulating samples, theories on quantum interference effects in the variable range hopping (VRH) regime [8] is in a good agreement with experiment for very low magnetic fields. On the other hand, Toyozawa [9] proposed that the negative magnetoresistance is a consequence of alignment of local magnetic moments by the applied magnetic field. This alignment decreases the magnetic scattering of electrons and reduces the electrical resistivity. The experimental magnetoresistance (MR) are plotted as a function of magnetic field in Figure 1. A negative MR is observed below 10 K with an unusual magnitude of about 10% at the lowest temperatures.
In order to consider only the negative part of the MR (Figure 2), the present paper is devoted to a tentative analysis of the negative MR of insulating a-NbSi sample in the temperature range 4.2 - 20 k and in magnetic fields up to 2.5 T using several theoretical models. Bishop et al. [10] have obtained a Metal-Insulator Transition (MIT) in this a-NbSi system at an atomic concentration of 88.7% Si. Our sample is situated in the insulating side of the MIT and referenced Nb11Si89. The Variable Range Hopping (VRH) conduction has been observed in this sample, and all the conductivity data, for different magnetic fields, follows a universal VRH law given by:
 (1)
(1)
where . When
. When , the Density of State (DOS) is constant and
, the Density of State (DOS) is constant and , corresponding to the Mott VRH regime. But when
, corresponding to the Mott VRH regime. But when , the DOS varies in the vicinity of the Fermi level and
, the DOS varies in the vicinity of the Fermi level and , corresponding to the Efros and Schklovskii (ES) VRH regime. In fact, The VRH of the three dimensional disordered systems, like doped semiconductors were shown by Mott [11,12] to behave like
, corresponding to the Efros and Schklovskii (ES) VRH regime. In fact, The VRH of the three dimensional disordered systems, like doped semiconductors were shown by Mott [11,12] to behave like . This dependence
. This dependence

Figure 1. Negative MR versus magnetic field for several temperatures.
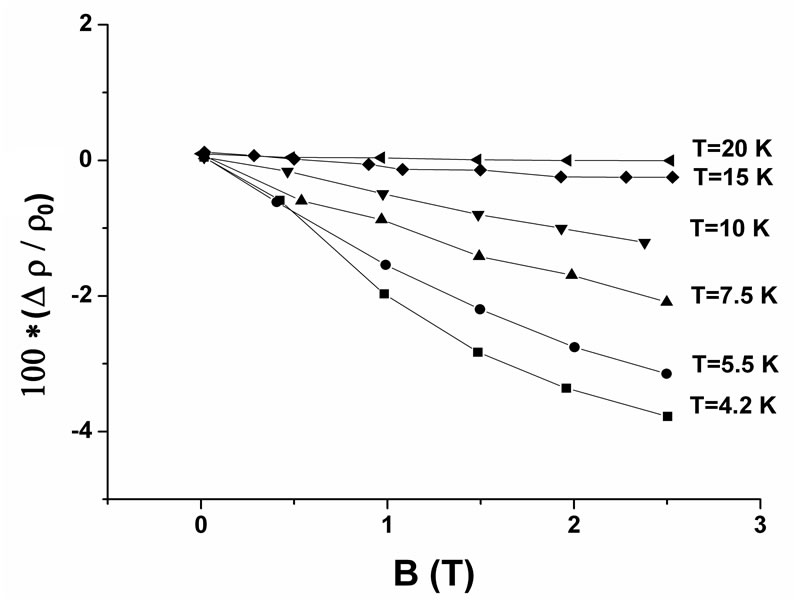
Figure 2. Negative MR versus magnetic field for several temperatures in magnetic fields up to 2.5 T.
was obtained by optimizing the hopping probability and assuming a slowly varying DOS in the vicinity of the Fermi level. Mott VRH has been widely observed in many types of disordered materials [13-16].
On the contrary, ES [17,18] have predicted that long range electron-electron interaction reduces the DOS at the Fermi level and creates a soft Coulomb Gap (CG), which takes the form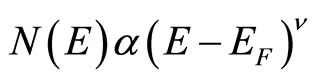 , with
, with . The existence of the CG leads to the ES VRH regime of the conductivity. However, the experimental situation has been confusing for some time, with both values of
. The existence of the CG leads to the ES VRH regime of the conductivity. However, the experimental situation has been confusing for some time, with both values of  being observed [19,20].
being observed [19,20].
In Figures 3 and 4, we plot respectively  against
against  and
and  for several values of magnetic field. It can be seen that it is difficult to choose between the Mott VRH regime with
for several values of magnetic field. It can be seen that it is difficult to choose between the Mott VRH regime with  and the ES VRH regime with
and the ES VRH regime with .
.
In this work we have attempted to decide for one of the two VRH regimes by studying the behaviour of negative magnetoresistance.
2. Quantum Interference
The manifestation of quantum interference has received
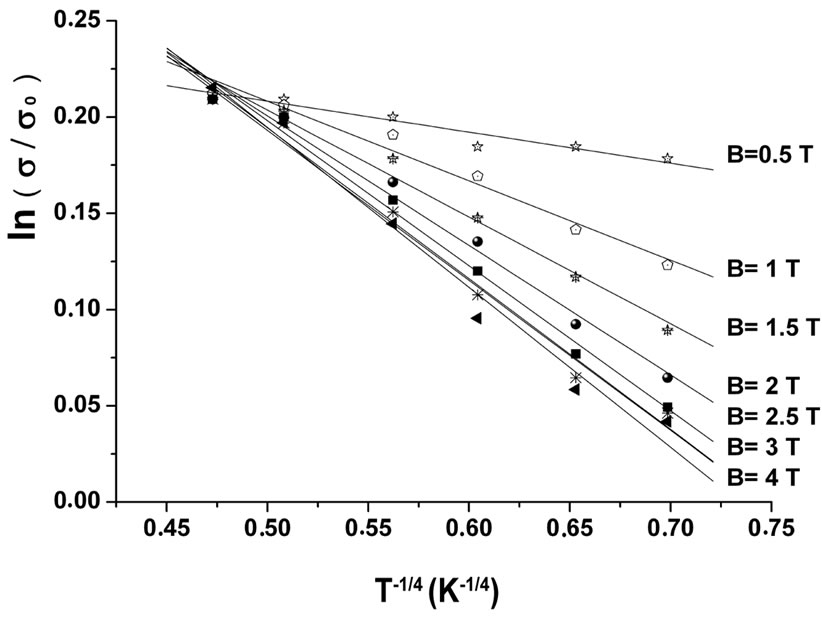
Figure 3.  against
against  for several values of magnetic field.
for several values of magnetic field.
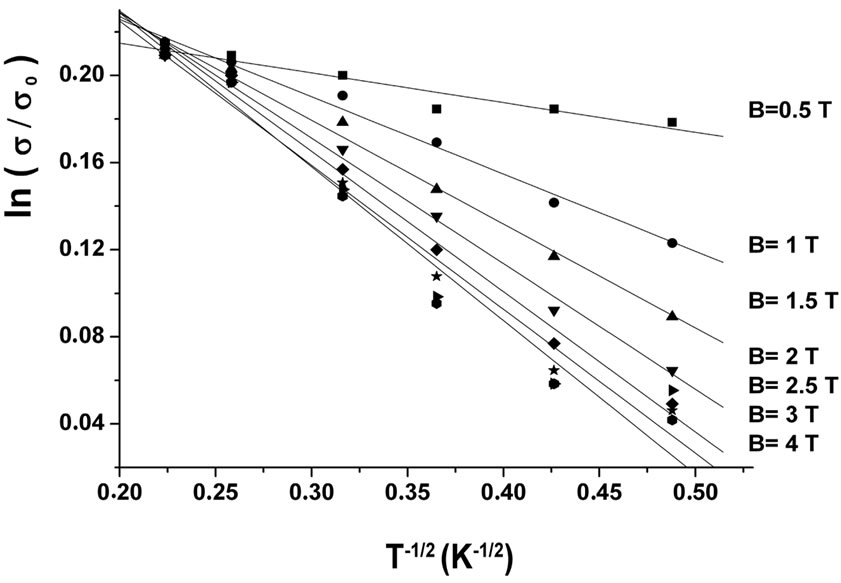
Figure 4.  against
against 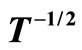 for several values of magnetic field.
for several values of magnetic field.
considerable attention in the properties of disordered systems. The low field NMR in disordered conductors has been studied and understood in terms of a suppression of quantum localization corrections in the Drude equation for the conductivity [1,9]. As Holstein [21] first noted in his seminal work on the Hall coefficient, quantum interference is also an important issue in disordered insulators where the transport at low temperature is dominated by phonon-assisted hopping. Theoretical studies [22-25] indicate that the magnetoconductance (MC) is positive in the VRH regime, as it is for weak localization. The positive MC in the VRH regime has been attributed to the effect of a magnetic field on the quantum interference between forward-scattering events.
Theories on quantum interference effects in the VRH regime have been proposed in references [22,23] and [24,25]. In these references, interference among the paths are associated to the hopping between two sites at a distance 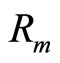 apart, where
apart, where  is the optimum hopping length. It is shown that this interference affects the hopping probability between these two sites. In the first case [22,23], by averaging numerically the logarithm of the conductivity over many random impurity realizations, a net linear decrease in resistivity with magnetic field is found. In the second case [24,25], the calculations are based on a critical path analysis and NMR, quadratic in the flux through an effective area
is the optimum hopping length. It is shown that this interference affects the hopping probability between these two sites. In the first case [22,23], by averaging numerically the logarithm of the conductivity over many random impurity realizations, a net linear decrease in resistivity with magnetic field is found. In the second case [24,25], the calculations are based on a critical path analysis and NMR, quadratic in the flux through an effective area  is predicted, where
is predicted, where  is the typical distance between impurities.
is the typical distance between impurities.
This gives:
 (2)
(2)
where  is the applied magnetic field.
is the applied magnetic field.
For a given sample and in small magnetic field where  is at most weak function of the field:
is at most weak function of the field:
 (3)
(3)
The temperature dependence of the hopping length 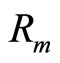 should determine the temperature dependence of the magnetoresistance. In three-dimensional systems where Mott VRH regime is observed
should determine the temperature dependence of the magnetoresistance. In three-dimensional systems where Mott VRH regime is observed  and Equation (3) predicts that:
and Equation (3) predicts that:
 (4)
(4)
In the present of a CG in the DOS associated with Coulomb corrections, the hopping length diverges more rapidly as  , and one expects:
, and one expects:
 (5)
(5)
Quadratic NMR with magnetic field has been observed experimentally in the VRH regime in a number of insulating materials [26,27]. Schirmacher [28] proposed another analysis of NMR in presence of VRH regime using the following expression:
 (6)
(6) 
3. Zeeman Effect
Fukuyama and Yosida [29] have proposed a mechanism to explain NMR behaviour in the VRH regime with magnetic field. According to these authors, the application of a magnetic field induces a cleavage of eigenstates degenerate to Zeeman sub-levels, and the repopulation of energy levels in which the majority of the holders are in the Zeeman higher state [29]. This state is closer to the mobility edge  . The wave function that corresponds to this state has a larger spatial extension, causing therefore a NMR given by:
. The wave function that corresponds to this state has a larger spatial extension, causing therefore a NMR given by:
 (7)
(7)
where  with
with  is the characteristic temperature depending on magnetic field.
is the characteristic temperature depending on magnetic field.  corresponding to the Mott VRH regime with
corresponding to the Mott VRH regime with  and
and 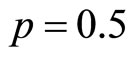 to the ES VRH regime with
to the ES VRH regime with .
.
4. Model of Localized Magnetic Moments
According to Toyozawa [9], the conduction electrons are scattered by the localized magnetic moments of impurities. The application of an external magnetic field orders these magnetic moments, so that the conductivity increases.
Yosida [30] has likened this system to a set of paramagnetic spins. The negative MR is then approached by a Curie-Weiss law:
 (8)
(8)
where  is the magnetic field and
is the magnetic field and 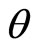 a temperature which may be considered as the Curie temperature of the paramagnetic system.
a temperature which may be considered as the Curie temperature of the paramagnetic system.
On the other hand, if magnetic moments exist, the magnetoresistance is proportional to the square of the average magnetization of impurities, which is described by a Brillouin function  in the classical theories of paramagnetic systems:
in the classical theories of paramagnetic systems:
 (9)
(9)
where 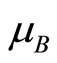 is the Bohr magneton,
is the Bohr magneton,  the effective magnetic moment of scattering centers,
the effective magnetic moment of scattering centers,  the concentration of scattering centers and
the concentration of scattering centers and  indicates the total moment of localized electrons, as in the case of negative MR study, the magnetization becomes proportional to a classical Langevin function:
indicates the total moment of localized electrons, as in the case of negative MR study, the magnetization becomes proportional to a classical Langevin function:
 (10)
(10)
where
 (11)
(11)
Thus, the negative MR stemming from the spin diffusion takes the following form:
 (12)
(12)
where  is a constant. We emphasize here that the total MR is, in fact, the sum of both negative and positive contributions:
is a constant. We emphasize here that the total MR is, in fact, the sum of both negative and positive contributions:

where the positive MR is given by the expression of Shklovskii and Efros [31] for the classically moderate field:
 (13)
(13)
The parameter  has been evaluated for each temperature in the high field region and the positive MR has been subtracted from the total MR, in order to consider only the negative part of the MR (Figure 2). In previous papers we have analyzed the positive MR given by Equation (13) in the different samples of CdSe [32,33] and InP [34,35].
has been evaluated for each temperature in the high field region and the positive MR has been subtracted from the total MR, in order to consider only the negative part of the MR (Figure 2). In previous papers we have analyzed the positive MR given by Equation (13) in the different samples of CdSe [32,33] and InP [34,35].
5. Results, Discussion and Conclusion
In this work, we will try to apply these theoretical models with our experimental results of NMR. First, we used the model of quantum interference. During the adjustments, the magnetic field is reduced so as to take the part of the magnetoresistance which we can apply this theory. Figure 5, shows the evolution of the NMR  with the magnetic field B at different temperatures. We observed that in the limit of low fields, the NMR varies linearly with
with the magnetic field B at different temperatures. We observed that in the limit of low fields, the NMR varies linearly with  and the slopes
and the slopes  of these straight lines decrease when the temperature increases. We also noticed that the value of magnetic field
of these straight lines decrease when the temperature increases. We also noticed that the value of magnetic field , from which the linearity deviation occurs increases with temperature, which is in good agreement with the model of Schirmacher [28]. We have determined the slopes
, from which the linearity deviation occurs increases with temperature, which is in good agreement with the model of Schirmacher [28]. We have determined the slopes  for each temperature Equation (6). Figure 6 shows the variation of
for each temperature Equation (6). Figure 6 shows the variation of  against
against . We have noticed that
. We have noticed that  varies with temperature
varies with temperature  as
as  . The value of the slope
. The value of the slope  is very close to the value −7/8 predicted by theory [28].
is very close to the value −7/8 predicted by theory [28].
For , the variation of NMR becomes linear with
, the variation of NMR becomes linear with  (Figure 7), which is in good agreement with
(Figure 7), which is in good agreement with

Figure 5. Negative MR against b at different temperatures.

Figure 6. Variation of ln(f2(T)) with ln(T).

Figure 7. Negative MR against b2 at different temperatures.
the theory proposed by Entin-Wolhman [25]. The NMR has been adjusted using the following from:  . To show the dependence of the NMR with the temperature, we plot in Figure 8 the variation of
. To show the dependence of the NMR with the temperature, we plot in Figure 8 the variation of  versus
versus . We observed that the curve obtained can be adjusted by a straight lines that give us the law:
. We observed that the curve obtained can be adjusted by a straight lines that give us the law:  (Equation (5)). The slope is equal to −1.48, which is very close to −3/2 predicted by the theory in ES VRH regime (Equation (5)).
(Equation (5)). The slope is equal to −1.48, which is very close to −3/2 predicted by the theory in ES VRH regime (Equation (5)).
We noticed that this variation can be associated with the ES VRH regime  . Several experimental measurements of NMR have been confronted with the theoretical expression of Entin-Wohlman on many insulating materials. The authors found consistent results with theoretical predictions of quantum interference in the two VRH regimes of Mott and of Efros Shklovskii [26,37- 39].
. Several experimental measurements of NMR have been confronted with the theoretical expression of Entin-Wohlman on many insulating materials. The authors found consistent results with theoretical predictions of quantum interference in the two VRH regimes of Mott and of Efros Shklovskii [26,37- 39].
On the other hand, within the limits of the fields where the NMR has been studied theoretically using the model of quantum interference, the approximations suggest an independence or weak dependence of parameters 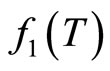 and
and , and in particular of the localization length
, and in particular of the localization length  with the magnetic field. If we go back to examine the variation of
with the magnetic field. If we go back to examine the variation of 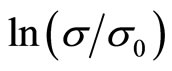 with
with  (Figure 3) we noticed that curves show straight lines of negative slope
(Figure 3) we noticed that curves show straight lines of negative slope
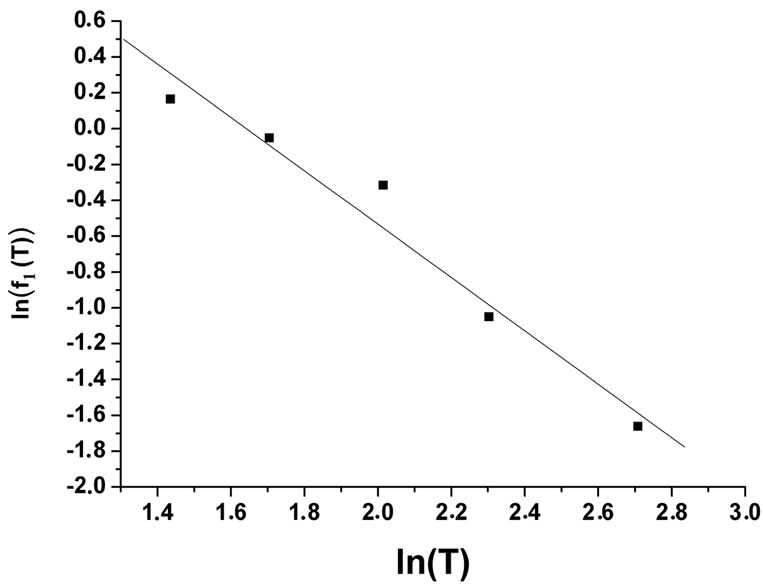
Figure 8. Variation of the parameter ln(f1(T)) versus ln(T).
which decreases with the magnetic field. This behaviour confirms the variation and the dependence of the localization length  with magnetic field.
with magnetic field.
We concluded that in this a-Nb11Si89 sample, the NMR can be interpreted by quantum interference effects and show the existence of the ES VRH conduction regime.
Secondly, we try to adjust our NMR using the theory of Zeeman effect expressed by Equation (7). We apply this expression of the NMR to our experimental results for different temperatures (Figure 9) with an adjustable parameter . The adjusted values of the parameter
. The adjusted values of the parameter  are shown in Figure 10.
are shown in Figure 10.  varies with temperature as
varies with temperature as . These results are in good agreement with the presence of the ES VRH regime
. These results are in good agreement with the presence of the ES VRH regime  . On the other hand, if we assume, as Benzaquen [39], that in the range of low magnetic field, the characteristic temperature is independent of the magnetic field
. On the other hand, if we assume, as Benzaquen [39], that in the range of low magnetic field, the characteristic temperature is independent of the magnetic field  , we can make a comparison between the adjusted values and theoretical values of
, we can make a comparison between the adjusted values and theoretical values of  (Table 1).
(Table 1).
Despite the good agreement in the dependence of parameter  as a function of temperature between the adjusted values and theoretical values in the ES VRH regime
as a function of temperature between the adjusted values and theoretical values in the ES VRH regime  confirming the existence of the ES VRH conduction regime in our a-Nb11Si89 sample. The qualitative disagreement is accentuated (Figure 9) as in the case of CuInSe2 [39] and GaAs [40]. A relatively low magnetic field lifts the degeneracy of the spin eigenstates situated near the Fermi level. We believe that the theoretical model of the Zeeman effect would be particularly appropriate in the critical region of the metal-insulator transition on the insulator side.
confirming the existence of the ES VRH conduction regime in our a-Nb11Si89 sample. The qualitative disagreement is accentuated (Figure 9) as in the case of CuInSe2 [39] and GaAs [40]. A relatively low magnetic field lifts the degeneracy of the spin eigenstates situated near the Fermi level. We believe that the theoretical model of the Zeeman effect would be particularly appropriate in the critical region of the metal-insulator transition on the insulator side.
Third, we try to analyze our NMR behaviour using the model of magnetic model. In a first approach, following the Equation (12), the Curie temperature  has been evaluated by plotting
has been evaluated by plotting  as a function of the temperature for each value of the magnetic field
as a function of the temperature for each value of the magnetic field . Figure 11, clearly exhibits a set of straight lines which, if extended to the low temperature limit, are secant at a point
. Figure 11, clearly exhibits a set of straight lines which, if extended to the low temperature limit, are secant at a point

Figure 9. Variation of experimental NMR and theoretical NMR (solid line) using the theory of Zeeman effect with magnetic field.

Figure 10. Variation of the adjusted parameter a1 in Equation (7) with temperature.
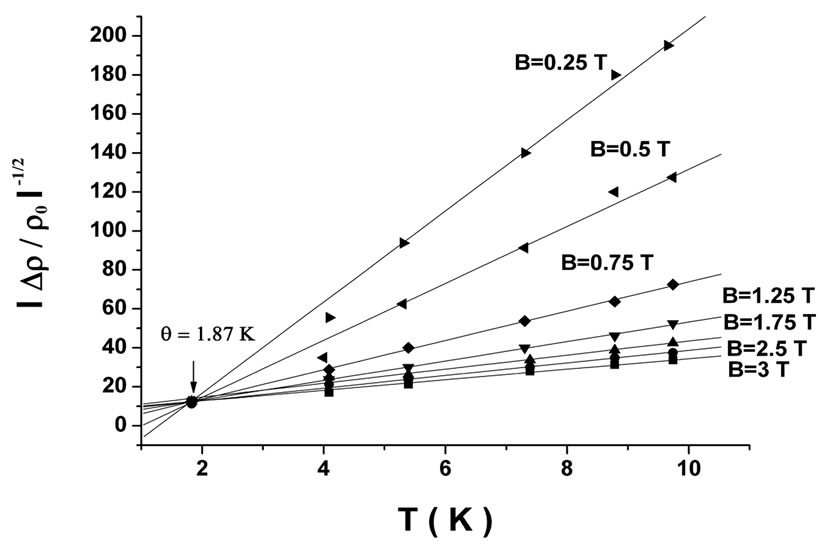
Figure 11. Inverse square root of the NMR against temperature at deferent magnetic fields.
of coordinates  . The Curie temperature
. The Curie temperature  is estimated at about 1.87 K. the fit of the negative MR by the Langevin function allows a more accurate evaluation of
is estimated at about 1.87 K. the fit of the negative MR by the Langevin function allows a more accurate evaluation of . On the Figure 12 is plotted
. On the Figure 12 is plotted  versus the ratio
versus the ratio  , for temperature varying
, for temperature varying
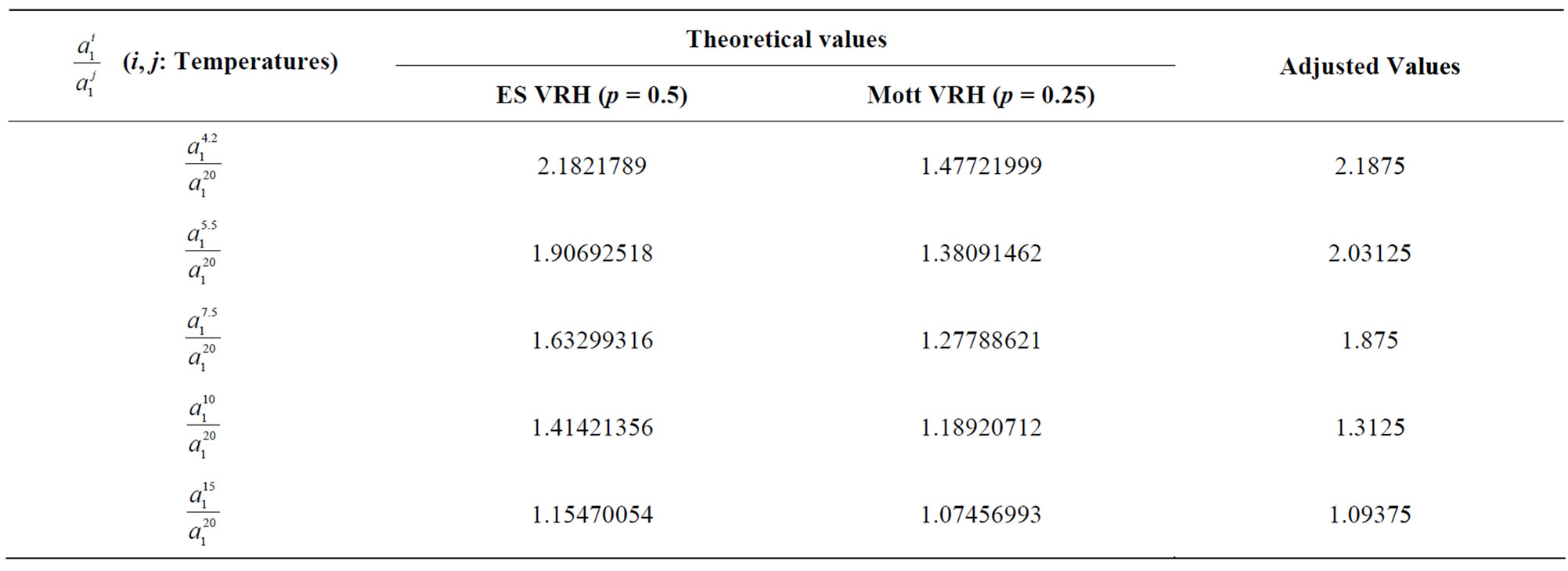
Table 1. Adjusted and theoretical values of parameter a1 in Equation (7).
between 4.2 K and 20 K and magnetic fields between  and
and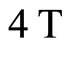 . The better fit is obtained for
. The better fit is obtained for  and
and 

According to Toyozawa [9], the existence of a negative MR is based on the assumption that the value of  lies between 5 and
lies between 5 and 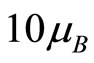 . This interval is explained as the result of the collective nature of the magnetic moment in the study of statistical distribution of the localized spins. This value of
. This interval is explained as the result of the collective nature of the magnetic moment in the study of statistical distribution of the localized spins. This value of  is about twice as large as 10 who is the maximum of n proposed by Toyosawa [9]. This theoretical model does not give us a value of n in the range proposed by Toyozawa (5 < n < 10). We conclude that our negative magnetoresistance cannot be explained by this model.
is about twice as large as 10 who is the maximum of n proposed by Toyosawa [9]. This theoretical model does not give us a value of n in the range proposed by Toyozawa (5 < n < 10). We conclude that our negative magnetoresistance cannot be explained by this model.
The model of localized moments for negative MR has been previously tested successfully on metallic InP [41], insulating CdAs2 [42] and Al-doped ZnO metallic thin films [43] samples. In these materials, the theoretical model is very consistent with experience and the effective magnetic moment values are included in the range defined by Toyozawa.
NOTES