Einstein-Podolsky-Rosen Steering and Nonlocality in Open Quantum Systems

Abdelaziz Sabik

Department of Physics, College of Science, Imam Mohammad Ibn Saud Islamic University (IMSIU), Riyadh, Saudi Arabia.

DOI: 10.4236/jmp.2024.154021                

Abstract

We investigate the dynamical behavior of quantum steering (QS), Bell nonlocality, and entanglement in open quantum systems. We focus on a two-qubit system evolving within the framework of Kossakowski-type quantum dynamical semigroups. Our findings reveal that the measures of quantumness for the asymptotic states rely on the primary parameter of the quantum model. Furthermore, control over these measures can be achieved through a careful selection of these parameters. Our analysis encompasses various cases, including Bell states, Werner states, and Horodecki states, demonstrating that the asymptotic states can exhibit steering, entanglement, and Bell nonlocality. Additionally, we find that these three quantum measures of correlations can withstand the influence of the environment, maintaining their properties even over extended periods.

Keywords

Quantum Steering, Dynamical Semigroups, Bell Nonlocality, Open Quantum System, Asymptotic Dynamics, Entanglement

1. Introduction

Quantum entanglement (QE) has emerged as a fascinating subject and a fundamental aspect of quantum mechanics since the pioneering efforts of Einstein and colleagues [1] . This phenomenon represents a type of non-local correlation manifesting in the inseparability of states, and it has played a significant role in advancing key tasks in quantum information processing and transmission [2] - [8] . Notably, correlations derived from local measurements on entangled systems can exhibit nonlocal properties [9] . The theory of local hidden variables imposes constraints on the measurement statistics for a broad set of quantum systems [10] . Nonlocality, an essential feature of QE, was highlighted through the “EPR paradox,” central to understanding nonlocality [1] . This paradox questions quantum theory’s ability to explain what has been termed “spooky action at a distance.” Schrödinger further elucidated this by introducing the concept of quantum steering (or EPR steering), which allows local measurements to control a distant quantum subsystem without direct interference [11] . Quantum steering (QS) is widely applied as a quantifier of the quantum correlation that exhibits the Einstein-Podolsky-Rosen (EPR) paradox. In the field of modern quantum information theory, it is considered a form of quantum correlation between quantum entanglement (QE) and Bell’s nonlocality. Entangled states are regarded as a superclass of steerable states [12] . Recently, QS has attracted significant attention in both experimental and theoretical research [13] - [19] . Steerable states can be applied in channel discrimination [20] , cryptography [21] , and teleportation [22] . Additionally, Quantum Steering (QS) is attributed to operational descriptions [23] . Both discrete and continuous quantum systems exhibit some QS inequalities, which are derived using the uncertainty relation in terms of entropy [24] [25] [26] . There have been significant experimental and theoretical developments aimed at explaining this form of nonlocality [25] [26] [27] [28] . Among these developments, significant standards for QS have been established by demonstrating QS in various aspects [29] [30] . The study of the Bell plan facilitates the expression of Bell-like inequalities for QS. Furthermore, the criteria for QS utilize the relationship between Quantum Entanglement (QE) and the uncertainty relation [29] [30] .

In addition to characterizing and quantifying correlations, understanding the behavior of these correlations under the influence of decoherence is another significant issue. Practical quantum systems inevitably interact with their surroundings, which during their dynamical evolution leads to decoherence [31] . In recent decades, studies have primarily focused on relaxation and dephasing in Markovian and non-Markovian open quantum systems. Consequently, dynamic evolution is an important property of quantum systems, which renders the quantum of finite time intriguing [32] . In nearly all models used to depict open quantum systems, the coherence of a state asymptotically descends to zero. Additionally, the entanglement dynamics often exhibit sudden death in a decoherent environment [33] . Thus, the asymptotic dynamics of various physical quantities offer ideal systems for examining the quantum properties crucial to information and quantum optics. Various systems, including quantum dot systems [34] [35] [36] [37] [38] , impurity systems [39] [40] [41] [42] [43] , and trapped ions [44] [45] [46] , implement these architectures. The performance of quantum properties is often characterized by environmental noise present in quantum systems. Therefore, developing a method for estimating noise levels is critical to mitigate the phenomenon of decay under decoherence. Controlling the environmental conditions of a quantum system is essential for managing its degradation effects. In this manuscript, we investigate the dynamical behavior of quantum systems (QS), Bell nonlocality, and quantum entanglement (QE) in open quantum systems. We examine the development of a two-qubit system within the framework of Kossakowski-type quantum dynamical semigroups. This study highlights how the fundamental criteria of the quantum model impact the quantumness measures of the asymptotic states. We present various scenarios where the asymptotic states exhibit steerability, entanglement, and Bell nonlocality. Additionally, we demonstrate the resilience of quantum measures of correlations against environmental influences, maintaining their integrity over extended periods.

The manuscript is organized as follows: Section 2 discusses the measures of quantumness, which describe the quantum correlations. Section 3 introduces the quantum model for the open quantum system and presents the numerical results. A summary is provided in the final section.

2. Quantum Steering, Quantum Nonlocality and Nonlocal Correlation

The local hidden state model for discrete observables has been developed [25] [26] [30] . Through the consideration of the positivity of the relative entropy, it is possible to evaluate the local hidden state constraints in the scenario involving discrete variables: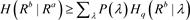, here ${\hat{R}}_i^a$ ( ${\hat{S}}_i^a$ ) and ${\hat{R}}_i^b$ ( ${\hat{S}}_i^b$ ) represent, respectively, discrete observables with outcomes $\left\{R_i^a\right\}$ ( $\left\{S_i^a\right\}$ ) and $\left\{R_i^b\right\}$ ( $\left\{S_i^b\right\}$ ). $H_q\left(R^b|\lambda \right)$ describes the discrete Shannon entropy of $P_q\left(R^b|\lambda \right)$ with $H\left(R\right)=-{\displaystyle {\sum }_{i}P\left(R_i\right)\ln P\left(R_i\right)}$ . The steering inequality for the discrete variables is acquired by [30] :

$H\left(S^b|S^a\right)+H\left(R^b|R^a\right)\ge \log \left({\Omega }^b\right),{\Omega }^b\equiv {\min }_{i,j}\left(1/{\left|\langle R_i|S_j\rangle \right|}^2\right)$ . (1)

For quantum systems defined in two dimensional space, the inequality of the entropy uncertainty relation (EUR) steering is given by [30]

$H\left({\sigma }_z^b|{\sigma }_z^a\right)+H\left({\sigma }_y^b|{\sigma }_y^a\right)+H\left({\sigma }_x^b|{\sigma }_x^a\right)\ge 2,$ (2)

where QS occurs when the inequality is violated. Consider a bipartite system of qubits in the X-state

$R_X=\left(\begin{array}{cccc}{R}_{11}& 0& 0& R_{14}\\ 0& R_{22}& R_{23}& 0\\ 0& R_{23}& R_{33}& 0\\ R_{14}& 0& 0& R_{44}\end{array}\right),$ (3)

where $R_{ij}$ are real. By performing a convenable local unitary transformation, the state $R_X$ defined in equation (3) according to the Bloch decomposition can be written as

(4)

where $\stackrel{\to }{s}$ and $\stackrel{\to }{r}$ are the vectors of Bloch and ${\sigma }_j^{a,b}$ representing the Pauli matrices. Then, based on the definition of the QS inequality in equation (2) and using the matrix state $R_x$ in equation (3), the QS inequality in the context of Pauli measurements is given by [47]

$\begin{array}{l}\underset{j=1,2}{{\displaystyle \sum }}\left[\left(1+{\beta }_j\right){\log }_2\left(1+{\beta }_j\right)+\left(1-{\beta }_j\right){\log }_2\left(1-{\beta }_j\right)\right]-\left(1+r\right){\log }_2\left(1+r\right)\\ -\left(1-r\right){\log }_2\left(1-r\right)+\frac{1}{2}\left(1+{\beta }_3+r+s\right){\log }_2\left(1+{\beta }_3+r+s\right)\\ +\frac{1}{2}\left(1+{\beta }_3-r-s\right){\log }_2\left(1+{\beta }_3-r-s\right)+\frac{1}{2}\left(1-{\beta }_3-r+s\right){\log }_2\left(1-{\beta }_3-r+s\right)\\ +12\left(1-{\beta }_3+r-s\right){\log }_2\left(1-{\beta }_3+r-s\right)\le 2,\end{array}$ (5)

where

${\beta }_1=2\left(R_{23}+R_{14}\right)$

${\beta }_2=2\left(R_{23}-R_{14}\right)$

${\beta }_3=R_{11}+R_{44}-R_{22}-R_{33}$

$r=R_{11}+R_{22}-R_{33}-R_{44}$

$s=R_{11}-R_{22}+R_{33}-R_{44}$

with $R_{ij}$ are the diagonal ( $i=j$ ) and anti-diagonal ( $i\ne j$ ) elements of the state $R_x$ . Additionally, an inequality has been developed to assess whether a bipartite quantum state is steerable [29] . Recently, Cavalcanti et al. proposed other parameters for the quantification of Quantum Steering (QS) [48] . Subsequently, a measure of steering was introduced, taking into account the maximal violation of the steering inequalities [49] .

We introduce the Bell Clauser-Horen-Shimony-Holt (CHSH) to test the quantum nonlocality. As stated by the Horodecki criterion [10] , $Be=2\sqrt{{\max }_{i<j}\left({\mu }_i+{\mu }_j\right)}$ with $i,j=1,2,3$ . The elements ${\mu }_i$ design the eigenvalues of $U={\mathcal{T}}^t\mathcal{T}$ with $\mathcal{T}$ is obtained from the coefficients [10] [50]

$t_{ij}=\text{Tr}\left(R{\sigma }_i\otimes {\sigma }_j\right)$ . (6)

Their eigenvalues are given by

${\mu }_1=4{\left(\left|R_{14}\right|+\left|R_{23}\right|\right)}^2,{\mu }_2=4{\left(\left|R_{14}\right|-\left|R_{23}\right|\right)}^2,{\mu }_3={\left(R_{11}-R_{22}-R_{33}+R_{44}\right)}^2\text{.}$

It is obvious to note that ${\mu }_1$ is greater than ${\mu }_2$ , and then the Bell inequality maximum violation is introduced by the formula [47]

$Be=2\max \left\{B{e}_1,B{e}_2\right\},B{e}_1=\sqrt{{\mu }_1+{\mu }_2},B{e}_2=\sqrt{{\mu }_1+{\mu }_3}\text{.}$ (7)

We utilize the entanglement of formation (EOF) developed by the Wootters [51] [52] to exhibit the entanglement in the qubits state

$\mathcal{E}\left(R\right)=\mathcal{H}\left[1+\frac{\sqrt{1-{\mathcal{C}}^2\left(R\right)}}{2}\right],$ (8)

where the function $\mathcal{H}$ is stated by

$\mathcal{H}\left(a\right)=-a{\log }_{2}a-\left(1-a\right){\log }_2\left(1-a\right),$ (9)

and the concurrence by

$\mathcal{C}\left(R\right)=\max \left\{0,\sqrt{{\alpha }_1}-\sqrt{{\alpha }_2}-\sqrt{{\alpha }_3}-\sqrt{{\alpha }_4}\right\},$ (10)

where ${\alpha }_i$ define the eigenvalues of $R\tilde{R}$ in decreasing order and $\tilde{R}$ is given by

$\tilde{R}=\left({\sigma }_y\otimes {\sigma }_y\right)R^{\text{*}}\left({\sigma }_y\otimes {\sigma }_y\right)$ (11)

where $R^{\text{*}}$ is the complex conjugate of R. The degree of QE varies from $\mathcal{E}=0$ for the case of separables states to $\mathcal{E}=1$ for the case of Bell states.

3. Asymptotic States and Numerical Results

In this section, we present the physical model of asymptotic quantum states, considering a system that evolves according to Kossakowski-type quantum dynamical semigroups. Furthermore, we provide results describing the evolution of the quantifiers.

Consider two qubits placed in an environment, analyzed using standard weak-coupling limit techniques [53] . The reduced irreversible quantum dynamics of this system is characterized by a single parameter known as quantum dynamical semigroups, represented by $\delta \left(t\right)=\exp \left(tL\right)$ . The behavior of this system is described by the master equation: ${\partial }_t\rho \left(t\right)=L\left[\rho \left(t\right)\right]$ where the generator L accounts for the influences of the external environment through the elements of the Kossakowski matrix [53] [54] [55] . Officially, we have

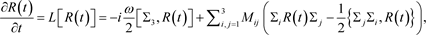(12)

where the parameter $\omega$ designs the system frequency, ${\Sigma }_i:=\mathbb{I}\otimes {\sigma }_i+{\sigma }_i\otimes \mathbb{I}$ , $\mathbb{I}$ is the 2 × 2 identity matrix, ${\sigma }_i$ represent the Pauli matrices and the M matrix is given by

$M=\left[M_{ij}\right]=\left(\begin{array}{ccc}1& i\gamma & 0\\ -i\gamma & 1& 0\\ 0& 0& 1\end{array}\right)\gamma \in ℝ,{\gamma }^2\le 1.$ (13)

This illustrates that the semigroup obtained by using the main equation includes purely positive maps $\delta \left(t\right)$ for all $t\ge 0$ . Using ${\sigma }_i^{\left(1\right)}={\sigma }_i\otimes \mathbb{I}$ and ${\sigma }_i^{\left(2\right)}=\mathbb{I}\otimes {\sigma }_i$ , the fully dissipative contribution to the quantum state is

$D\left[R\left(t\right)\right]={\displaystyle {\sum }_{i,j=1}^3{M}_{ij}}{\displaystyle {\sum }_{a,b=1}^2\left({\sigma }_i^{\left(a\right)}R\left(t\right){\sigma }_j^{\left(b\right)}-\frac{1}{2}\left\{{\sigma }_j^{\left(b\right)}{\sigma }_i^{\left(a\right)},R\left(t\right)\right\}\right)}\text{.}$ (14)

In this manner, there exist six Kraus operators ${\sigma }_i^{\left(a\right)}$ ( $a=1,2$ ; $i=1,2,3$ ) and

6 × 6 Kossakowski matrix is written

$K=\left(\begin{array}{cc}M& M\\ M& M\end{array}\right)\text{.}$ (15)

From open systems theory [53] [56] , the coefficients $M_{ij}^{\left(\alpha \beta \right)}$ related to the i^th Pauli matrix of the α^th qubit and, respectively, the j^th Pauli matrix of the β^th qubit, $\alpha ,\beta =1,2$ ; $j=1,2,3$ , are defined through the use of Fourier transorms of the time-correlation functions with regard to the equilibrium state of the environment.

Now, we are going to examine the temporal evolution of the two qubits defined initially in the state

$R\left(0\right)=A|1\rangle \langle 1|+D|2\rangle \langle 2|+B|3\rangle \langle 3|+C|4\rangle \langle 4|+E|1\rangle \langle 2|+F|2\rangle \langle 1|\text{.}$ (16)

Here the parameters A, B, C and D are considered to be real constants with the normalization condition $A+B+C+D=1$ , and that elements $\left\{|1\rangle ,|2\rangle ,|3\rangle ,|4\rangle \right\}$ form an orthonormal basis related to the basis $\left\{|00\rangle ,|01\rangle ,|10\rangle ,|11\rangle \right\}$ by

$$|1\rangle =|00\rangle ,|2\rangle =|11\rangle ,|3\rangle =1/\sqrt{2}|01\rangle +1/\sqrt{2}|10\rangle ,|4\rangle =1/\sqrt{2}|01\rangle -|10\rangle 1/\sqrt{2}\text{.}$$ (17)

Here $|3\rangle$ and $|4\rangle$ define the Bell states.

The density matrix (16) can be represented as

$R\left(0\right)=\left(\begin{array}{cccc}A& 0& 0& E\\ 0& B/2+C/2& B/2-C/2& 0\\ 0& B/2-C/2& B/2+C/2& 0\\ F& 0& 0& D\end{array}\right)\text{.}$ (18)

Equation (18) is entangled when ${\left(A+B\right)}^2/4<E^2$ or $AD<{\left(B-C\right)}^2/4$ . The both conditions cannot be met at the same time. The density operator (18) covers a large family of states for the system of qubits, including Bell states [57] [58] , Werner states [59] , Horodecki states [59] , and so on.

The temporal evolution of $R\left(t\right)$ can be obtained as

$\begin{array}{c}R\left(t\right)=A\left(t\right)|1\rangle \langle 1|+D\left(t\right)|2\rangle \langle 2|+B\left(t\right)|3\rangle \langle 3|+C\left(t\right)|4\rangle \langle 4|\\ +E\left(t\right)|1\rangle \langle 2|+F\left(t\right)|2\rangle \langle 1|\text{.}\end{array}$ (19)

where the time-dependent coefficients are given by

$\begin{array}{l}A\left(t\right)={\left(1-\gamma \right)}^{2}3+{\gamma }^{2}K+2\left(1+\gamma \right)A-{\left(1-\gamma \right)}^2\left(B+D\right)3+{\gamma }^2{H}_{+}\left(t\right)\\ +\sqrt{1-{\gamma }^2}{\left(1+\gamma \right)}^{2}A-2\left(1-\gamma \right)D+{\left(1+\gamma \right)}^{2}B\left(1+\gamma \right)\left(3+{\gamma }^2\right)H_{-}\left(t\right)\\ D\left(t\right)={\left(1+\gamma \right)}^{2}3+{\gamma }^{2}K-{\left(1+\gamma \right)}^{2}A-2\left(1+\gamma \right)D+{\left(1+\gamma \right)}^{2}B3+{\gamma }^2{H}_{+}\left(t\right)\\ -\sqrt{1-{\gamma }^2}2\left(1+\gamma \right)A-{\left(1-\gamma \right)}^2\left(B+D\right)\left(1-\gamma \right)\left(3+{\gamma }^2\right)H_{-}(\; t\; )\end{array}$

$\begin{array}{l}B\left(t\right)={\left(1-\gamma \right)}^{2}3+{\gamma }^{2}K+2\left(1+{\gamma }^2\right)B-\left(1-{\gamma }^2\right)\left(A+D\right)3+{\gamma }^2{H}_{+}\left(t\right)\\ +\sqrt{1-{\gamma }^2}{\left(1+\gamma \right)}^{3}A+{\left(1-\gamma \right)}^{3}D-2\left(1-{\gamma }^2\right)B\left(1-{\gamma }^2\right)\left(3+{\gamma }^2\right)H_{-}\left(t\right)\\ C\left(t\right)=C\\ E\left(t\right)=R\exp \left(-12t\right)\\ F\left(t\right)=F\exp \left(-12t\right)\end{array}$ (20)

with

$\begin{array}{l}K=A+B+D,H_{+}\left(t\right)={\text{e}}^{-8t}\cosh 4t\sqrt{1-{\gamma }^2},\\ H_{-}\left(t\right)={\text{e}}^{-8t}\sinh 4t\sqrt{1-{\gamma }^2}.\end{array}$ (21)

It is acknowledged that, in certain situations, the coupling of the environment does not necessarily destroy coherence but can instead generate it. This phenomenon may also be observed in the current model due to the reduced dynamics described in equation (12).

We now examine three classes of correlations in the asymptotic dynamics for different families of initial states. Based on equations (6), (8), and (9), we demonstrate the performance of Quantum Steerability (QS), Quantum Nonlocality, and Quantum Entanglement (QE) in qubit states with respect to the parameters of the model. The quantum correlations for diverse sets of initial states, assuming various values of the parameter γ, are depicted in Figures 1-3. Generally, it is observed that changes in the parameter γ significantly affect the quantum dynamics and the measures of quantum correlations for different states.The measures of the Bell nonlocality and Quantum Steering (QS) can exhibit similar behavior during their time evolution.It is interesting to note that by carefully selecting the parameter $\gamma$ and the initial state of the two qubits, we can control and manipulate these quantum measures in the asymptotic dynamics. When starting with an initial separable state of two qubits, we observe that the state of the qubits is unsteerable and does not demonstrate quantum nonlocality, despite the generation of entanglement, as illustrated in Figure 1. This indicates that both steering and Bell nonlocalities, starting from the initial separable state, are satisfied and accompanied by an entangled state in the asymptotic dynamics, for different values of γ. In the case of an initial Horodecki state, the quantum measures firstly decrease from their maximal value, where the two qubits’ state is maximally steerable (for the case of $a=1$ ), quantum nonlocality with the Tsirelson bound and maximally entangled state, and thereafter the measures decrease with the time exhibiting the sudden death phenomenon of entanglement with the absence of QS and Bell nonlocality. We obtain that QS and Bell nonlocality disappear before the entanglement. Moreover, we observe that the change in the parameter γ does not largely affect the behaviour of the quantum measures during the dynamics, as seen in Figure 2. In the third example, specifically for an initial Werner state, we observe that the qubits’ state exhibits steerability and Bell nonlocality, indicating the presence of entanglement during the asymptotic dynamics. This observation is depicted in Figure 3 for the case of $a=0.8$ , where both CHSH and steering inequalities are violated, confirming the entanglement of the qubits’ state. Conversely, for the case of $a=0.5$ as presented in Figure 3, the qubits’ state remains entangled without violating steering and CHSH inequalities. Additionally, variations in the values of γ can enhance the correlations throughout the dynamics.

Figure 1. Asymptotic dynamics of the correlations for the initial state, $R\left(0\right)=\left(|3\rangle \langle 3|+|4\rangle \langle 4|\right)/2$ , is plotted as a function of the time t for $\gamma =0.3$ and $\gamma =0.8$ . The axis $y=2$ represents the case for which the QS inequality defined in equation (5) holds the value 2. The blue dash-dotted curve displays the variety of the QS inequality, red dashed curve represents the variation of the Bell inequality and green solid line shows the variety of the QE. The panel (a) is for $\gamma =0.3$ and panel (b) is for $\gamma =0.8$ .

Figure 2. Asymptotic dynamics of the correlations for the initial Horodecki state, $R\left(0\right)=a|3\rangle \langle 3|+\left(1-a\right)|1\rangle \langle 1|$ , is plotted as a function of the time t for the case of $a=0.8$ (entangled state) and $$a=1$$ (bell state). The blue dash-dotted curve displays the variation of the QS inequality, red dashed curve represents the variation of the Bell inequality and green solid line shows the variety of the QE. The panels (a) and (b) are respectively for $\gamma =0.3$ and $\gamma =0.8$ with $a=1$ (bell state). The panels (c) and (d) are respectively for $\gamma =0.3$ and $\gamma =0.8$ with $a=0.8$ (entangled mixed state). The axis $y=2$ represents the case for which the QS inequality defined in equation (5) holds the value 2.

Figure 3. Asymptotic dynamics of the correlations for the initial Werner state, $R\left(0\right)=a|3\rangle \langle 3|+\left(1-a\right)|1\rangle \langle 1|$ , is plotted as a function of the time t for the case of $a=0.8$ and $a=0.5$ . The blue dash-dotted curve displays the variation of the QS inequality, red dashed curve represents the variation of the Bell inequality and green solid line shows the variety of the QE. The panels (a) and (b) are respectively for $\gamma =0.3$ and $\gamma =0.8$ with $a=0.8$ . The panels (c) and (d) are respectively for $\gamma =0.3$ and $\gamma =0.8$ with $a=0.5$ . The axis $y=2$ represents the case for which the QS inequality defined in equation (5) holds the value 2.

4. Conclusion

We have investigated the dynamic behavior of quantum steering (QS), Bell nonlocality, and entanglement of formation in open quantum systems. Our study introduced a system comprising two qubits within the Kossakowski quantum dynamical semigroup framework. It was demonstrated that the quantum characteristics of the asymptotic states are influenced by the primary parameter of the model under consideration, and that manipulation of these characteristics can be achieved through careful selection of these parameters. Our analysis spanned various scenarios, encompassing Werner states, Bell states, and Horodecki states, wherein the asymptotic states exhibit steerability and entanglement, coupled with Bell nonlocality. Additionally, we demonstrated that the three measures of quantum correlations are capable of withstanding the effects of external environments, thereby preserving their integrity over extended durations.

Conflicts of Interest

The author declares no conflicts of interest regarding the publication of this paper.

References

[1]	Einstein, A., Podolsky, B. and Rosen, N. (1935) Physical Review, 47, 777-780. https://doi.org/10.1103/PhysRev.47.777
[2]	Nilsen, M.A. and Chuang, I.L. (2000) Quantum Computation and Quantum Communication. Cambridge University Press, Cambridge.
[3]	Bennett, C.H. and Di Vincenzo, D.P. (2000) Nature (London), 404, 247-255. https://doi.org/10.1038/35005001
[4]	Berrada, K. and Eleuch, H. (2019) Physical Review D, 100, Article ID: 016020. https://doi.org/10.1103/PhysRevD.100.016020
[5]	Berrada, K. (2013) Physical Review A, 88, Article ID: 013817. https://doi.org/10.1103/PhysRevA.88.035806
[6]	Zheng, S.B. and Guo, G.C. (2000) Physical Review Letters, 85, 2392-2395. https://doi.org/10.1103/PhysRevLett.85.2392
[7]	Castelano, L.K., Fanchini, F.F. and Berrada, K. (2016) Physical Review B, 94, Article ID: 235433. https://doi.org/10.1103/PhysRevB.94.235433
[8]	Berrada, K. (2017) Journal of the Optical Society of America B, 34, 1912-1917. https://doi.org/10.1364/JOSAB.34.001912
[9]	Bell, J.S. (1964) Physics (Long Island City, N.Y.), 1, 195-200. https://doi.org/10.1103/PhysicsPhysiqueFizika.1.195
[10]	Horodecki, R., Horodecki, P., Horodecki, M. and Horodecki, K. (2009) Reviews of Modern Physics, 81, 865-942. https://doi.org/10.1103/RevModPhys.81.865
[11]	Schrödinger, E. (1936) Mathematical Proceedings of the Cambridge Philosophical Society, 32, 446-452. https://doi.org/10.1017/S0305004100019137
[12]	Wiseman, H.M., Jones, S.J. and Doherty, A.C. (2007) Physical Review Letters, 98, Article ID: 140402. https://doi.org/10.1103/PhysRevLett.98.140402
[13]	Saunders, D.J., Jones, S.J., Wiseman, H.M. and Pryde, G.J. (2010) Nature Physics, 6, 845-849. https://doi.org/10.1038/nphys1766
[14]	Wollmann, S., Walk, N., Bennet, A.J., Wiseman, H.M. and Pryde, G.J. (2016) Physical Review Letters, 116, Article ID: 160403. https://doi.org/10.1103/PhysRevLett.116.160403
[15]	Algarni, M., Berrada, K., Abdel-Khalek, S. and Eleuch, H. (2021) Symmetry, 13, Article No. 2445. https://doi.org/10.3390/sym13122445
[16]	Berrada, K. and Eleuch, H. (2023) Laser Physics Letters, 20, Article ID: 085201. https://doi.org/10.1088/1612-202X/acde73
[17]	Kalaga, J.K. and Leoński, W. (2017) Quantum Information Processing, 16, Article No. 175. https://doi.org/10.1007/s11128-017-1627-6
[18]	Abdel-Khalek, S., Berrada, K., Algarni, M. and Eleuch, H. (2021) Entropy, 23, Article No. 1442. https://doi.org/10.3390/e23111442
[19]	Berrada, K. and Eleuch, H. (2021) Physica E: Low-Dimensional Systems and Nanostructures, 126, Article ID: 114412. https://doi.org/10.1016/j.physe.2020.114412
[20]	Piani, M. and Watrous, J. (2015) Physical Review Letters, 114, Article ID: 060404. https://doi.org/10.1103/PhysRevLett.114.060404
[21]	Branciard, C., Cavalcanti, E.G., Walborn, S.P., Scarani, V. and Wiseman, H.M. (2012) Physical Review A, 85, Article ID: 010301. https://doi.org/10.1103/PhysRevA.85.010301
[22]	He, Q., Rosales-Zárate, L., Adesso, G. and Reid, M.D. (2015) Physical Review Letters, 115, Article ID: 180502. https://doi.org/10.1103/PhysRevLett.115.180502
[23]	Jones, S.J., Wiseman, H.M. and Doherty, A.C. (2007) Physical Review A, 76, Article ID: 052116. https://doi.org/10.1103/PhysRevA.76.052116
[24]	Skrzypczyk, P., Navascués, M. and Cavalcanti, D. (2014) Physical Review Letters, 112, Article ID: 180404. https://doi.org/10.1103/PhysRevLett.112.180404
[25]	Walborn, S.P., Salles, A., Gomes, R.M., Toscano, F. and Souto Ribeiro, P.H. (2011) Physical Review Letters, 106, Article ID: 130402. https://doi.org/10.1103/PhysRevLett.106.130402
[26]	Zhen, Y.-Z., Zheng, Y.-L., Cao, W.-F., Li, L., Chen, Z.-B., Liu, N.-L. and Chen, K. (2016) Physical Review A, 93, Article ID: 012108. https://doi.org/10.1103/PhysRevA.93.012108
[27]	Bowles, J., Vértesi, T., Quintino, M.T. and Brunner, N. (2014) Physical Review Letters, 112, Article ID: 200402. https://doi.org/10.1103/PhysRevLett.112.200402
[28]	He, Q.-Y. and Reid, M.D. (2013) Physical Review Letters, 111, Article ID: 250403.
[29]	Cavalcanti, E.G., Jones, S.J., Wiseman, H.M. and Reid, M.D. (2009) Physical Review A, 80, Article ID: 032112. https://doi.org/10.1103/PhysRevA.80.032112
[30]	Schneeloch, J., Broadbent, C.J., Walborn, S.P., Cavalcanti, E.G. and Howell, J.C. (2013) Physical Review A, 87, Article ID: 062103. https://doi.org/10.1103/PhysRevA.87.062103
[31]	Scully, M.O. and Zubairy, M.S. (1997) Quantum Optics. Cambridge U.P., New York. https://doi.org/10.1017/CBO9780511813993
[32]	Tsang, M. (2013) Physical Review A, 88, 021801(R). https://doi.org/10.1103/PhysRevA.88.021801
[33]	Yu, T. and Eberly, J.H. (2006) Physical Review Letters, 97, Article ID: 140403. https://doi.org/10.1103/PhysRevLett.97.140403
[34]	Shulman, D., et al. (2012) Science, 336, 202-205. https://doi.org/10.1126/science.1217692
[35]	Schuetz, M.J.A., Kessler, E.M., Vandersypen, L.M.K., Cirac, J.I. and Giedke, G. (2014) Physical Review B, 89, Article ID: 195310. https://doi.org/10.1103/PhysRevB.89.195310
[36]	Borras, A. and Blaauboer, M. (2011) Physical Review B, 84, Article ID: 033301. https://doi.org/10.1103/PhysRevB.84.033301
[37]	Hiltunen, T. and Harju, A. (2014) Physical Review B, 89, Article ID: 115322. https://doi.org/10.1103/PhysRevB.89.115322
[38]	Culcer, D., Cywinski, L., Li, Q.Z., Hu, X.D. and Das Sarma, S. (2009) Physical Review B, 80, Article ID: 205302. https://doi.org/10.1103/PhysRevB.80.205302
[39]	Kennes, D.M., Meden, V. and Vasseur, R. (2014) Physical Review B, 90, Article ID: 115101. https://doi.org/10.1103/PhysRevB.90.115101
[40]	Vasseur, R., Trinh, K., Haas, S. and Saleur, H. (2013) Physical Review Letters, 110, Article ID: 240601. https://doi.org/10.1103/PhysRevLett.110.240601
[41]	White, S.R. (1992) Physical Review Letters, 69, 2863-2866. https://doi.org/10.1103/PhysRevLett.69.2863
[42]	Vidal, G. (2004) Physical Review Letters, 93, Article ID: 040502. https://doi.org/10.1103/PhysRevLett.93.040502
[43]	Schollwock, U. (2011) Annals of Physics, 326, 96-192.
[44]	Porras, D. and Cirac, J.I. (2004) Physical Review Letters, 92, Article ID: 207901. https://doi.org/10.1103/PhysRevLett.92.207901
[45]	Leibfried, D., et al. (2003) Nature (London), 422, 412-415. https://doi.org/10.1038/nature01492
[46]	Schmidt-Kaler, F., et al. (2003) Nature (London), 422, 408-411. https://doi.org/10.1038/nature01494
[47]	Sun, W.-Y., Wang, D., Shi, J.-D. and Ye, L. (2017) Scientific Reports, 7, Article No. 39651.
[48]	Cavalcanti, E.G., He, Q.Y., Reid, M.D. and Wiseman, H.M. (2011) Physical Review A, 84, Article ID: 032115. https://doi.org/10.1103/PhysRevA.84.032115
[49]	Costa, A.C.S. and Angelo, R.M. (2016) Physical Review A, 93, Article ID: 020103. https://doi.org/10.1103/PhysRevA.93.020103
[50]	Horodecki, R., Horodecki, P. and Horodecki, M. (1995) Physics Letters A, 200, 340-344. https://doi.org/10.1016/0375-9601(95)00214-N
[51]	Bennett, C.H., Di Vincenzo, D.P., Smolin, J.A. and Wootters, W.K. (1996) Physical Review A, 54, 3824-3851. https://doi.org/10.1103/PhysRevA.54.3824
[52]	Wootters, W.K. (1998) Physical Review Letters, 80, 2245-2248. https://doi.org/10.1103/PhysRevLett.80.2245
[53]	Alicki, R. and Lendi, K. (2007) Quantum Dynamical Semigroups and Applications (Lecture Notes in Physics). Springer, Berlin, 717.
[54]	Breuer, H.-P. and Petruccione, F. (2002) The Theory of Open Quantum Systems. Oxford University Press, Oxford. https://doi.org/10.1007/3-540-44874-8_4
[55]	Benatti, F., Liguori, A.M. and Pluzzano, G. (2010) Journal of Physics A: Mathematical and Theoretical, 43, Article ID: 045304. https://doi.org/10.1088/1751-8113/43/4/045304
[56]	Spohn, H. (1980) Reviews of Modern Physics, 52, 569-615. https://doi.org/10.1103/RevModPhys.52.569
[57]	Nielsen, M. (2010) Quantum Computation and Quantum Information. Cambridge University Press, Cambridge.
[58]	Werner, R.F. (1989) Physical Review A, 40, 4277-4281. https://doi.org/10.1103/PhysRevA.40.4277
[59]	Horodecki, M., Horodecki, P. and Horodecki, R. (2001) Quantum Information: An Introduction to Basic Theoretical Concepts and Experiments. Springer, Berlin, 151.