The Local Theory of Completely 1-Summing Mapping Spaces

Yafei Zhao¹, Yuanyi Wang^2*

¹Department of Mathematics, Zhejiang International Studies University, Hangzhou, China.
²College of Science and Technology, Ningbo University, Ningbo, China.

DOI: 10.4236/jamp.2023.116103                

Abstract

In this paper, we investigate local properties in the system of completely 1-summing mapping spaces. We introduce notions of injectivity, local reflexivity, exactness, nuclearity and finite-representability in the system of completely 1-summing mapping spaces. First we obtain that if V has WEP, V is locally reflexive in the system (Ⅱ₁(⋅,⋅), π₁(⋅)) if and only if it is locally reflexive in the system (Ⅰ(⋅,⋅), t(⋅)). Furthermore we prove that an operator space V ⊆ B(H) is exact in the system (Ⅱ₁(⋅,⋅), π₁(⋅)) if and only if V is finitely representable in {M_n}_n∈N in the system (Ⅱ₁(⋅,⋅), π₁(⋅)). At last, we show that an operator space V is finitely representable in {M_n}_n∈N in the system (Ⅱ₁(⋅,⋅), π₁(⋅)) if and only if V = C.

Keywords

Completely 1-Summing Mapping Space, Injectivity, Nuclearity, Local Reflexivity, Exactness, Finite-Representability and Operator Space

1. Introduction

The theory of operator spaces is a natural non-commutative quantization of Banach space theory. Many problems in operator spaces are naturally motivated by both Banach space theory and operator algebra theory. Some local properties such as injectivity, nuclearity, local reflexivity and exactness have been intensively studied in [1] [2] [3] . In particular, we have for any operator space V,

$V\text{is}\text{nuclear}\Rightarrow V\text{is}\text{exact}\Rightarrow V\text{is}\text{locally}\text{reflexive}\mathrm{.}$ (1)

The first implication of (1) was proved in [3] and the second in [2] . In [2] , Effros, Ozawa and Ruan showed that an operator space V is nuclear if and only if V is locally reflexive and $V^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}$ is injective. As pointed out in [2] , local reflexivity is an essential condition in this result since Kirchberg [4] has constructed a separable non-nuclear operator space V for which $V^{**}={\displaystyle {\prod }_{n=1}^{+\infty }}{M}_n$ . Turning to $C^{\mathrm{<mo>\; *\; </mo>}}$ -algebra theory, using Conne’s deep work in [5] , Choi and Effros proved the following result in [6] [7] :

$\text{A}{C}^{\mathrm{<mo>\; *\; </mo>}}\text{-algerba}\mathcal{A}\text{is}\text{nuclear}\iff \text{its}\text{second}\text{dual}{\mathcal{A}}^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}\text{is}\text{injective}\mathrm{.}$

In [8] , Dong and Ruan showed that an operator space V is exact if and only if V is locally reflexive and $V^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}$ is weak* exact.

For the convenience of the readers, we recall some basic notations and results in operator spaces, the details of which can be found in [9] [10] . Given abstract operator spaces V and W and a linear mapping $\phi \mathrm{<mo>\; :\; </mo>}V\to W$ , for each $n\in N$ there is a corresponding linear mapping ${\phi }_n\mathrm{<mo>\; :\; </mo>}{M}_n\left(V\right)\to M_n\left(W\right)$ defined by

${\phi }_n\left(v\right)=\left[\phi \left(v_{i\mathrm{,}j}\right)\right]$

for all $v=\left[v_{i\mathrm{,}j}\right]\in M_n\left(V\right)$ . We define the completely bounded norm of $\phi$ by

${\Vert \phi \Vert }_{cb}=\text{sup}\left\{\Vert {\phi }_n\Vert \mathrm{<mo>\; :\; </mo>}n\in N\right\}$

(this might be infinite). It is evident that the norms $\Vert {\phi }_n\Vert$ form an increasing sequence

$\Vert \phi \Vert \le \Vert {\phi }_2\Vert \le \cdots \le \Vert {\phi }_n\Vert \le \cdots \le {\Vert \phi \Vert }_{cb}\mathrm{.}$

We say that $\phi$ is completely bounded (respectively, completely contractive) if ${\Vert \phi \Vert }_{cb}<\infty$ (respectively, ${\Vert \phi \Vert }_{cb}\le 1$ ). It is a simple matter to verify that ${\Vert \cdot \Vert }_{cb}$ is a norm on the linear space $\mathcal{C}\mathcal{B}\left(V\mathrm{,}W\right)$ of completely bounded linear mappings $\phi \mathrm{<mo>\; :\; </mo>}V\to W$ . We say that $\phi$ is a complete quotient mapping if each ${\phi }_n$ is a quotient mapping (i.e. ${\phi }_n$ maps $M_n{\left(V\right)}_{\Vert \cdot \Vert <1}$ onto $M_n{\left(W\right)}_{\Vert \cdot \Vert <1}$ ), and that $\phi$ is an exact complete quotient mapping if each ${\phi }_n$ is an exact quotient mapping (i.e. ${\phi }_n$ maps $M_n{\left(V\right)}_{\Vert \cdot \Vert \le 1}$ onto $M_n{\left(W\right)}_{\Vert \cdot \Vert \le 1}$ ).

We use the notations $V\stackrel{⌣}{\otimes }W$ and $V\hat{\otimes }W$ for the injective, projective operator space tensor products (see [11] [12] ). In the following, we give some definitions of local properties in the system $\left(CB\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\Vert \cdot \Vert }_{cb}\right)$ for operator spaces. Given an operator space V, we define:

(a) Injectivity (see [9] ). If for any operator space $X\subseteq Y$ and a complete contraction $\phi \mathrm{<mo>\; :\; </mo>}X\to V$ , there is a completely contractive extension $\tilde{\phi }\mathrm{<mo>\; :\; </mo>}Y\to V$ .

(b) Local reflexivity (see [9] ). If for any finite dimensional operator space L, every complete contraction $\phi \mathrm{<mo>\; :\; </mo>}L\to V^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}$ is the point-weak^* limit of a net of complete contractions ${\phi }_{\alpha }\mathrm{<mo>\; :\; </mo>}L\to V$ . It follows from Theorem 14.3.1 in [9] that an operator space V is locally reflexive if and only if $CB\left(L\mathrm{,}{V}^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}\right)=CB{\left(L\mathrm{,}V\right)}^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}$ for any finite dimensional operator space L.

(c) Exactness (see [9] or [10] ). If for any finite dimensional subspace L of V and every $\epsilon >0$ , there exists an integer n and a subspace $S\subseteq M_n$ such that $d_{cb}\left(L\mathrm{,}S\right)<1+\epsilon$ . This is equivalent to the following definition: there exists a diagram of complete contractions.

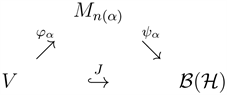
which approximately commute in the point-norm topology.

(d) Nuclearity (see [9] ). There exists a diagram of complete contractions

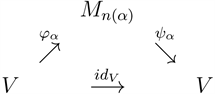
which approximately commute in the point-norm topology.

(e) Finitely representable in ${\left\{W_{\alpha }\right\}}_{\alpha \in I}$ (see [1] [3] ). Given operator spaces E and F, we define the completely bounded Banach-Mazur distance introduced in [3] :

$d_{cb}\left(E\mathrm{,}F\right)=\inf \left\{{\Vert r\Vert }_{cb}\cdot {\Vert r^{-1}\Vert }_{cb}\mathrm{<mo>\; :\; </mo>}E\stackrel{r}{\cong }F\right\}\mathrm{.}$ (2)

Given a family of operator spaces ${\left\{W_{\alpha }\right\}}_{\alpha \in I}$ and the distance (2), for every finite-dimensional subspace L of V and $\epsilon >0$ , there exists a subspace S of some $W_{\alpha }$ such that $d_{cb}\left(L,S\right)<1+\epsilon$ .

(f) The weak expectation property (or simply, WEP) (see [9] ). Given an operator space V, for any completely isometric inclusion $\tau \mathrm{<mo>\; :\; </mo>}V$ ↪ $\mathcal{B}\left(\mathcal{H}\right)$ , there exists a completely contractive mapping $P\mathrm{<mo>\; :\; </mo>}\mathcal{B}\left(\mathcal{H}\right)\to V^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}$ such that $P\circ \tau ={\text{ł}}_V$ , where ${\text{ł}}_V\mathrm{<mo>\; :\; </mo>}V$ ↪ $V^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}$ is the canonical inclusion.

Mapping spaces naturally arise in both the theory and applications of functional analysis. Grothendieck introduced the theory of nuclear mapping spaces ${\mathcal{N}}^B\left(\cdot \mathrm{,}\cdot \right)$ , integral mapping spaces ${\mathcal{I}}^B\left(\cdot \mathrm{,}\cdot \right)$ and 1-summing mapping spaces ${\Pi }_1^B\left(\cdot \mathrm{,}\cdot \right)$ in [13] . Nuclear mappings play an important part in the theory of Schwartz spaces and their applications to differential equations and quantum field theory. Grothendieck also used 1-summing mappings to investigate the Dvoretzky-Rogers theorem. It has been said that Grothendieck’s theory of 1-summing mappings is in some sense at the heart of Banach space theory.

With the results in [14] [15] [16] , it is now evident that major components of Grothendieck’s program make sense in the context of operator spaces. An operator space mapping space O is an assignment to each pair of operator spaces $V\mathrm{,}W$ of a linear space $O\left(V\mathrm{,}W\right)$ of completely bounded mapping $\phi \mathrm{<mo>\; :\; </mo>}V\to W$ , together with an operator space matrix norm ${\Vert \cdot \Vert }_O$ , such that for each $\phi \in M_n\left(O\left(V\mathrm{,}W\right)\right)$ ,

(a) ${\Vert \phi \Vert }_{cb}\le {\Vert \phi \Vert }_O$ ;

(b) for any linear mapping $r\mathrm{<mo>\; :\; </mo>}U\to V$ and $s\mathrm{<mo>\; :\; </mo>}W\to X$ ,

${\Vert s_n\circ \phi \circ r\Vert }_O\le {\Vert s\Vert }_{cb}\cdot {\Vert \phi \Vert }_O\cdot {\Vert r\Vert }_{cb}\mathrm{.}$

We define the completely nuclear mapping space $\mathcal{N}\left(V\mathrm{,}W\right)$ to be the image of the canonical mapping $\Phi \mathrm{<mo>\; :\; </mo>}{V}^{\mathrm{<mo>\; *\; </mo>}}\hat{\otimes }W\to V^{\mathrm{<mo>\; *\; </mo>}}\stackrel{⌣}{\otimes }W\subseteq CB\left(V\mathrm{,}W\right)$ , with the quotient operator space structure determined by the identification

$\mathcal{N}\left(V\mathrm{,}W\right)\cong V^{\mathrm{<mo>\; *\; </mo>}}\hat{\otimes }W\ker \Phi \mathrm{.}$

We let $\nu$ be the corresponding norm on $\mathcal{N}\left(V\mathrm{,}W\right)$ .

Given operator spaces V and W, we define a mapping $\phi \mathrm{<mo>\; :\; </mo>}V\to W$ to be completely integral if

$\iota \left(\phi \right)=\sup \left\{\nu \left({\phi |}_S\right):\text{finite}\text{dimensional}S\subseteq V\right\}<\infty .$

We let $\mathcal{I}\left(V\mathrm{,}W\right)$ denote the set of all completely integral mappings from V into W. It is known from Lemma 12.3.1 in [9] that the following assertions are equivalent:

(a) $\iota \left(\phi \right)\le 1$ ;

(b) for all finite dimensional operator spaces L,

$\Vert i{d}_L\otimes \phi \mathrm{<mo>\; :\; </mo>}L\stackrel{⌣}{\otimes }V\to L\hat{\otimes }W\Vert \le \mathrm{1\; <mo>\; ;\; </mo>}$

(c) for all operator spaces U,

$\Vert i{d}_U\otimes \phi \mathrm{<mo>\; :\; </mo>}U\stackrel{⌣}{\otimes }V\to U\hat{\otimes }W\Vert \le \mathrm{1\; <mo>\; ;\; </mo>}$

if $\phi \mathrm{<mo>\; :\; </mo>}V\to W$ is a linear mapping of operator spaces, then we define ${\pi }_1\left(\phi \right)$ in $\left[\mathrm{0,}\infty \right]$ by

$\begin{array}{c}{\pi }_1\left(\phi \right)=\Vert i{d}_{T_{\infty }}\otimes \phi \mathrm{<mo>\; :\; </mo>}{T}_{\infty }\stackrel{⌣}{\otimes }V\to T_{\infty }\hat{\otimes }W\Vert \\ =\sup \left\{\Vert i{d}_{T_r}\otimes \phi \mathrm{<mo>\; :\; </mo>}{T}_r\stackrel{⌣}{\otimes }V\to T_r\hat{\otimes }W\Vert \mathrm{<mo>\; :\; </mo>}r\in N\right\}\mathrm{.}\end{array}$ (3)

If ${\pi }_1\left(\phi \right)<\infty$ , we say that $\phi$ is a completely 1-summing mapping from V into W and we refer to (3) as the completely 1-summing norm of $\phi$ . We let ${\Pi }_1\left(V\mathrm{,}W\right)$ denote the space of all completely 1-summing mappings from V into W.

For given operator spaces V and W, analogues of four of the most important Banach mapping spaces (nuclear mapping spaces, integral mapping spaces, absolutely summing mapping spaces and bounded mapping spaces) are the completely nuclear mapping spaces $\left(\mathcal{N}\left(V\mathrm{,}W\right)\mathrm{,}\nu \right)$ , the completely integral mapping spaces $\left(\mathcal{I}\left(V\mathrm{,}W\right)\mathrm{,}\iota \right)$ , the completely 1-summing mapping spaces $\left({\Pi }_1\left(V\mathrm{,}W\right)\mathrm{,}{\pi }_1\right)$ and the completely bounded mapping spaces $\left(CB\left(V\mathrm{,}W\right)\mathrm{,}{\Vert \cdot \Vert }_{cb}\right)$ . We have the following contractive inclusions:

$\mathcal{N}\left(V\mathrm{,}W\right)\subseteq \mathcal{I}\left(V\mathrm{,}W\right)\subseteq {\Pi }_1\left(V\mathrm{,}W\right)\subseteq CB\left(V\mathrm{,}W\right)\mathrm{.}$

As we know, mapping spaces provide a fundamental tool for studying Banach spaces and operator spaces. In this note, we are interested in the local properties in the system of completely 1-summing mapping spaces $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1\right)$ . As we have seen, the local theory in the system of completely 1-summing mapping spaces is quite different from the original local theory of completely bounded mapping spaces.

2. Completely 1-Summing Mapping Space

We let ${\Pi }_1\left(V\mathrm{,}W\right)$ denote the space of all completely 1-summing mappings from V into W. This is again an operator space since we may use the embedding

${\Pi }_1\left(V\mathrm{,}W\right)$ ↪ $CB\left(T_{\infty }\stackrel{⌣}{\otimes }V\mathrm{,}{T}_{\infty }\hat{\otimes }W\right)\mathrm{<mo>\; :\; </mo>}\varphi \mapsto id\otimes \varphi \mathrm{.}$

For any matrix $\varphi =\left[{\varphi }_{i\mathrm{,}j}\right]\in M_m\left({\Pi }_1\left(V\mathrm{,}W\right)\right)$ ,

${\pi }_{\mathrm{1,}m}\left(\phi \right)={\Vert id\otimes \phi =\left[id\otimes {\varphi }_{i\mathrm{,}j}\right]\mathrm{<mo>\; :\; </mo>}{T}_{\infty }\stackrel{⌣}{\otimes }V\to M_m\left(T_{\infty }\hat{\otimes }W\right)\Vert }_{cb}\mathrm{.}$

Let $r\mathrm{<mo>\; :\; </mo>}V\to V\mathrm{,}s\mathrm{<mo>\; :\; </mo>}W\to X\mathrm{,}\varphi \mathrm{<mo>\; :\; </mo>}V\to M_m\left(W\right)$ ,

$T_{\infty }\stackrel{⌣}{\otimes }V\stackrel{id\otimes r}{\to }{T}_{\infty }\hat{\otimes }V\stackrel{id\otimes \varphi }{\to }{M}_m\left(T_{\infty }\hat{\otimes }W\right)\stackrel{{\left(id\otimes s\right)}_m}{\to }{M}_m\left(T_{\infty }\hat{\otimes }X\right)\mathrm{.}$

We have ${\pi }_{\mathrm{1,}m}\left(s_m\circ \varphi \circ r\right)\le {\Vert s\Vert }_{cb}{\pi }_{\mathrm{1,}m}\left(\varphi \right){\Vert r\Vert }_{cb}$ .

Hahn-Banach theorem is the key initial ingredient of classical functional analysis. So we first investigate its analogue in the system of completely 1-summing mapping spaces.

Definition 2.1. We say an operator space V is injective in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if for any operator spaces $W_0\subseteq W$ , every completely 1-summing mapping ${\phi }_0\in {\Pi }_1\left(W_0\mathrm{,}V\right)$ has a linear extension $\phi \in {\Pi }_1\left(W\mathrm{,}V\right)$ satisfying ${\pi }_1\left(\phi \right)={\pi }_1\left({\phi }_0\right)$ .

Lemma 2.2. A nuclear operator space V is injective in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if and only if for any inclusion of operator spaces $W_0\subseteq W$ , the restriction mapping

$\rho \mathrm{<mo>\; :\; </mo>}{\Pi }_1\left(W\mathrm{,}V\right)\to {\Pi }_1\left(W_0\mathrm{,}V\right)$

is an exact completely quotient mapping.

Proof. From Corollary 15.5.3 in [9] , if V is a nuclear operator space, then we have the isometry $\mathcal{I}\left(V\mathrm{,}W\right)={\Pi }_1\left(V\mathrm{,}W\right)$ for all operator space W. By the Lemma 2.2 in [17] , we get the conclusion.¨

Proposition 2.3. If an operator space U is injective in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ and $\Phi \mathrm{<mo>\; :\; </mo>}U\to V$ is a completely contractive projection, then $V=\Phi \left(U\right)$ is again injective in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ .

Proof. Let $W_0\subseteq W$ and ${\phi }_0\in {\Pi }_1\left(W_0\mathrm{,}V\right)$ with ${\pi }_1\left({\phi }_0\right)\le 1$ .

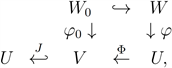
where J is the inclusion mapping. It follows from the completely 1-summing injectivity of U that there exists an extension $\phi \in {\Pi }_1\left(W\mathrm{,}U\right)$ with ${\phi |}_{W_0}=J\circ {\phi }_0$ and

${\pi }_1\left(\phi \right)={\pi }_1\left(J\circ {\phi }_0\right)\le {\pi }_1\left({\phi }_0\right)\le 1.$

Since for any $w_0\in W_0$ , ${\phi }_0\left(w_0\right)\in V=\Phi \left(U\right)$ . Thus we have

$\Phi \circ \phi \left(w_0\right)=\Phi \circ J\circ {\phi }_0\left(w_0\right)=\Phi \circ {\phi }_0\left(w_0\right)={\phi }_0(\; w\; 0\; )$

and this implies that $\Phi \circ \phi \mathrm{<mo>\; :\; </mo>}W\to V$ is an extension of ${\phi }_0\mathrm{<mo>\; :\; </mo>}{W}_0\to V$ with

${\pi }_1\left(\Phi \circ \phi \right)\le {\Vert \Phi \Vert }_{cb}\cdot {\pi }_1\left(\phi \right)\le {\pi }_1\left(\phi \right)\le 1.$

Therefore $V=\Phi \left(U\right)$ is again injective in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ .¨

Definition 2.4. (Definition 2.1 in [18] ) An operator space V is nuclear in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if there exists a diagram of linear mappings with ${\pi }_1\left({\phi }_{\alpha }\right)\le 1$ , ${\pi }_1\left({\psi }_{\alpha }\right)\le 1$

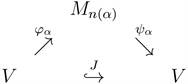
which approximately commute in the point-norm topology.

Theorem 2.5. An operator space V is nuclear in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if and only if V is nuclear and ${\pi }_1\left(i{d}_V\right)\le 1$ .

Proof. From Corollary 13.2.2 in [9] , we have ${\pi }_1\left(\psi \right)\le \iota \left(\psi \right)$ . If an operator space $V\subseteq \mathcal{B}\left(\mathcal{H}\right)$ is nuclear in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ , it is nuclear in the system $\left(\mathcal{I}\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}\iota (\cdot )\right)$ . By Theorem 3.4 in [17] , we have V is nuclear and $\iota \left(i{d}_V\right)\le 1$ . We get ${\pi }_1\left(i{d}_V\right)\le 1$ . Conversely, suppose that V is nuclear and ${\pi }_1\left(i{d}_V\right)\le 1$ . By nuclearity of V, there exists a diagram of complete contractions

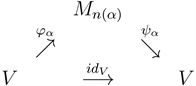
which approximately commute in the point-norm topology. Thus we have

${\pi }_1\left({\phi }_{\alpha }\right)={\pi }_1\left({\phi }_{\alpha }\circ i{d}_V\right)\le {\Vert {\phi }_{\alpha }\Vert }_{cb}\cdot {\pi }_1\left(i{d}_V\right)\le 1$

and

${\pi }_1\left({\psi }_{\alpha }\right)={\pi }_1\left(i{d}_V\circ {\psi }_{\alpha }\right)\le {\pi }_1\left(i{d}_V\right)\cdot {\Vert {\psi }_{\alpha }\Vert }_{cb}\le 1.$

Therefore V is nuclear in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ .¨

Definition 2.6. (Definition 3.1 in [18] ) An operator space V is $\lambda$ -nuclear in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if there exists a diagram of linear mappings with ${\pi }_1\left({\phi }_{\alpha }\right)\le \lambda$ , ${\pi }_1\left({\psi }_{\alpha }\right)\le \lambda$

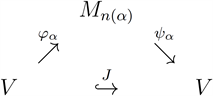
which approximately commute in the point-norm topology.

Theorem 2.7. An operator space V is $\lambda$ -nuclear in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if and only if V is nuclear and ${\pi }_1\left(i{d}_V\right)\le \lambda$ .

Proof. The proof is similar to that of Theorem 2.5.¨

Definition 2.8. An operator space V is locally reflexive in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if for any finite dimensional operator space L,

${\Pi }_1\left(L\mathrm{,}{V}^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}\right)={\Pi }_1{\left(L\mathrm{,}W\right)}^{\mathrm{<mo>\; *\; </mo>\; <mo>\; *\; </mo>}}\mathrm{.}$

Proposition 2.9. If V has WEP, V is locally reflexive in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if and only if it is locally reflexive in the system $\left(\mathcal{I}\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}\iota (\cdot )\right)$ .

Proof. From Corollary 15.5.2 in [9] , if V has WEP, we have the isometry $\mathcal{I}\left(L\mathrm{,}V\right)={\Pi }_1\left(L\mathrm{,}V\right)$ for all operator spaces L.¨

Proposition 2.10. If $A$ is an injective $C^{\mathrm{<mo>\; *\; </mo>}}$ -algebra, $A$ is locally reflexive in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if and only if it is locally reflexive in the system $\left(\mathcal{I}\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}\iota (\cdot )\right)$ .

Proof. From Proposition 15.5.1 in [9] , $A$ is an injective $C^{\mathrm{<mo>\; *\; </mo>}}$ -algebra, we have the isometry $\mathcal{I}\left(L\mathrm{,}A\right)={\Pi }_1\left(L\mathrm{,}A\right)$ for all operator spaces L.¨

Definition 2.11. An operator space $V\subseteq \mathcal{B}\left(\mathcal{H}\right)$ is exact in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if there exists a diagram of linear mappings with ${\pi }_1\left({\phi }_{\alpha }\right)\le 1$ , ${\pi }_1\left({\psi }_{\alpha }\right)\le 1$

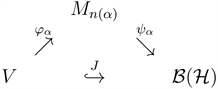
which approximately commute in the point-norm topology.

Proposition 2.12. An operator space V is nuclear in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ , then it is nuclear in the system $\left(\mathcal{I}\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}\iota (\cdot )\right)$ .

Proof. From Corollary 13.2.2 in [9] , we have ${\pi }_1\left(\psi \right)\le \iota \left(\psi \right)$ .¨

Proposition 2.13. An operator space V is exact in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ , then it is nuclear in the system $\left(\mathcal{I}\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}\iota (\cdot )\right)$ .

Proof. From Corollary 13.2.2 in [9] , we have ${\pi }_1\left(\psi \right)\le \iota \left(\psi \right)$ .¨

Corollary 2.14. An operator space V is nuclear in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if and only if $V=ℂ$ .

Proof. From Proposition 2.12, the operator space V is also nuclear in the system $\left(\mathcal{I}\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}\iota (\cdot )\right)$ . From Theorem 3.11 in [17] , we have $V=ℂ$ . The converse is obvious.¨

From the work of Kirchberg and Pisier ( [3] [19] ) we see that an operator space V is exact if and only if it is finitely representable in ${\left\{M_n\right\}}_{n\in \mathbb{N}}$ . In this note, we will consider the analogue in the system of completely 1-summing mapping spaces. Given operator spaces $E\mathrm{,}F$ , we define

$d_{{\pi }_1}\left(E,F\right)=\inf \left\{{\pi }_1\left(\phi \right)\cdot {\pi }_1\left({\phi }^{-1}\right):E\stackrel{\phi }{\cong }F\right\}.$

Definition 2.15. Given a family of operator spaces ${\left\{W_{\alpha }\right\}}_{\alpha \in I}$ . We say that an operator space V is finitely representable in ${\left\{W_{\alpha }\right\}}_{\alpha \in I}$ in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if for every finite-dimensional subspace L of V and $\epsilon >0$ , there exists a subspace S of some $W_{\alpha }$ such that $d_{{\pi }_1}\left(L,S\right)<1+\epsilon$ .

In this paper, we are mainly interested in the finite-representability in ${\left\{M_n\right\}}_{n\in \mathbb{N}}$ in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ .

Theorem 2.16. An operator space $V\subseteq \mathcal{B}\left(\mathcal{H}\right)$ is exact in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if and only if V is finitely representable in ${\left\{M_n\right\}}_{n\in \mathbb{N}}$ in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ .

Proof. Suppose that $V\subseteq \mathcal{B}\left(\mathcal{H}\right)$ is exact in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ . By Definition 2.11, there exists a diagram of linear mappings with ${\pi }_1\left({\phi }_{\alpha }\right)\le 1$ , ${\pi }_1\left({\psi }_{\alpha }\right)\le 1$

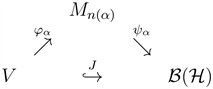
which approximately commute in the point-norm topology. Thus for any $\epsilon >0$ and any finite dimensional subspace $L\subseteq V$ , we choose Auerbach basis $\left\{x_1\mathrm{,}\cdots \mathrm{,}{x}_n\right\}\subseteq L$ and $\left\{f_1\mathrm{,}\cdots \mathrm{,}{f}_n\right\}\subseteq L^{\mathrm{<mo>\; *\; </mo>}}$ . It follows from the exactness of V in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ that we can find linear mappings

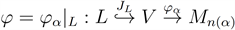
and

$\psi ={\psi }_{\alpha }\mathrm{<mo>\; :\; </mo>}{M}_{n\left(\alpha \right)}\to \mathcal{B}(\; H\; )$

such that

${\displaystyle \underset{i=1}{\overset{n}{\sum }}}\Vert f_i\Vert \cdot \Vert \psi \circ \phi \left(x_i\right)-x_i\Vert <\epsilon$

and ${\pi }_1\left(\phi \right)={\pi }_1\left({\phi }_{\alpha }\circ J_L\right)\le {\pi }_1\left({\phi }_{\alpha }\right)\le 1$ , ${\pi }_1\left(\psi \right)={\pi }_1\left({\psi }_{\alpha }\right)\le 1$ . Lemma 15.3.3 in [9] implies that there is a complete isomorphism $T\mathrm{<mo>\; :\; </mo>}\mathcal{B}\left(\mathcal{H}\right)\to \mathcal{B}\left(\mathcal{H}\right)$ such that $T\left(\psi \circ \phi \left(x_i\right)\right)=x_i$ , where ${\Vert T\Vert }_{cb}<1+\epsilon$ and ${\Vert T^{-1}\Vert }_{cb}<{\left(1-\epsilon \right)}^{-1}$ . Thus

$\phi \mathrm{<mo>\; :\; </mo>}L\to \phi \left(L\right)\subseteq M_{n(\; \alpha \; )}$

is a linear isomorphism with inverse ${\phi }^{-1}=T\circ \psi :\phi \left(L\right)\to L$ satisfying

${\pi }_1\left(\phi \right)\le 1\text{and}{\pi }_1\left({\phi }^{-1}\right)={\pi }_1\left(T\circ \psi \right)\le {\Vert T\Vert }_{cb}\cdot {\pi }_1\left(\psi \right)<1+\epsilon \mathrm{.}$

So V is finitely representable in ${\left\{M_n\right\}}_{n\in \mathbb{N}}$ in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ .

Conversely, suppose that V is finitely representable in ${\left\{M_n\right\}}_{n\in \mathbb{N}}$ in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ . By Definition 2.15, for any $\epsilon >0$ and any finite dimensional subspace $L\subseteq V$ , there exist an integer n, a subspace $S\subseteq M_n$ and a linear isomorphism

$\phi \mathrm{<mo>\; :\; </mo>}L\to S$

such that ${\pi }_1\left(\phi \right)\cdot {\pi }_1\left({\phi }^{-1}\right)<1+\epsilon$ . Since

${\pi }_1\left({\phi }^{-1}\mathrm{<mo>\; :\; </mo>}S\to \mathcal{B}\left(\mathcal{H}\right)\right)\le {\pi }_1\left({\phi }^{-1}\mathrm{<mo>\; :\; </mo>}S\to L\right)\mathrm{,}$

it follows from the injectivity of $\mathcal{B}\left(\mathcal{H}\right)$ in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ that there exists an extension $\Psi \in {\Pi }_1\left(M_n\mathrm{,}\mathcal{B}\left(\mathcal{H}\right)\right)$ with

${\pi }_1\left(\Psi \right)={\pi }_1\left({\phi }^{-1}\mathrm{<mo>\; :\; </mo>}S\to \mathcal{B}\left(\mathcal{H}\right)\right)\le {\pi }_1\left({\phi }^{-1}\mathrm{<mo>\; :\; </mo>}S\to L\right)\mathrm{.}$

Similarly, since

${\pi }_1\left(\phi \mathrm{<mo>\; :\; </mo>}L\to M_n\right)\le {\pi }_1\left(\phi \mathrm{<mo>\; :\; </mo>}L\to S\right)\mathrm{,}$

the injectivity of $M_n$ in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ implies that we have an extension $\Phi \in {\Pi }_1\left(V\mathrm{,}{M}_n\right)$ with

${\pi }_1\left(\Phi \right)={\pi }_1\left(\phi \mathrm{<mo>\; :\; </mo>}L\to M_n\right)\le {\pi }_1\left(\phi \mathrm{<mo>\; :\; </mo>}L\to S\right)\mathrm{.}$

Thus we have the following diagram

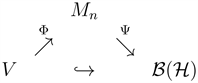
with ${\pi }_1\left(\Phi \right)\cdot {\pi }_1\left(\Psi \right)<1+\epsilon$ and $\Psi \circ {\Phi |}_L=i{d}_L$ . It is routine to show that V is exact in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ .¨

Proposition 2.17. An operator space V is finitely representable in ${\left\{M_n\right\}}_{n\in \mathbb{N}}$ in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ , then it is nuclear in the system $\left(\mathcal{I}\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}\iota (\cdot )\right)$ .

Proof. From Corollary 13.2.2 in [9] , we have ${\pi }_1\left(\psi \right)\le \iota \left(\psi \right)$ .¨

Corollary 2.18. An operator space V is finitely representable in ${\left\{M_n\right\}}_{n\in \mathbb{N}}$ in the system $\left({\Pi }_1\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}{\pi }_1(\cdot )\right)$ if and only if $V=ℂ$ .

Proof. From the above proposition, the operator space V is also finitely representable in ${\left\{M_n\right\}}_{n\in \mathbb{N}}$ in the system $\left(\mathcal{I}\left(\cdot \mathrm{,}\cdot \right)\mathrm{,}\iota (\cdot )\right)$ . From Theorem 4.3 in [17] , we have $V=ℂ$ . The converse is obvious.¨

3. Conclusion

In this paper, we have investigated local properties in the system of completely 1-summing mapping spaces. Combined with the work of [17] [18] , we have provided a very complete characterization of the local properties of completely 1-summing mapping spaces, completely nuclear mapping spaces and completely Integral Mapping Spaces. We have found if an operator is WEP, the locally reflexive property is equivalent in completely 1-summing mapping space and completely nuclear mapping spaces. And if an operator is exact, the finitely representable property is equivalent in completely 1-summing mapping space and completely nuclear mapping spaces.

In future work, we will apply the theories of completely 1-summing mapping spaces to the category of C*-algebras. For example, we will study Kirchberg’s conjecture and QWEP conjecture in the system of completely 1-summing mapping spaces. In addition, we will intensively study the relationship between various mapping spaces.

Fund

Project partially supported by Zhejiang Provincial Natural Science Foundation of China (Grant No. LQ21A010015).

Conflicts of Interest

The authors declare no conflicts of interest regarding the publication of this paper.

References

[1]	Effros, E.G., Junge, M. and Ruan, Z.-J. (2000) Integral Mapping and the Principle of Local Reflexivity for Non-Commutative L1 Spaces. Annals of Mathematics, 151, 59-92. https://doi.org/10.2307/121112
[2]	Effros, E.G., Ozawa, N. and Ruan, Z.-J. (2001) On Injectivity and Nuclearity for Operator Spaces. Duke Mathematical Journal, 110, 489-521. https://doi.org/10.1215/S0012-7094-01-11032-6
[3]	Pisier, G. (1992) Exact operator spaces. In: Recent Advances in Operator Algebras, Société Mathématique, Paris, 159-186.
[4]	Kirchberg, E. (1995) On Subalgebras of the CAR-Algebra. Journal of Functional Analysis, 129, 35-63. https://doi.org/10.1006/jfan.1995.1041
[5]	Connes, A. (1976) Classification of Injective Factors Cases II1, II∞, IIIλ, λ ≠ 1. Annals of Mathematics, 104, 73-115. https://doi.org/10.2307/1971057
[6]	Choi, M.-D. and Effros, E.G. (1976) Separable Nuclear C*-Algebras and Injectivity. Duke Mathematical Journal, 43, 309-322. https://doi.org/10.1215/S0012-7094-76-04328-3
[7]	Choi, M.-D. and Effros, E.G. (1977) Nuclear C*-Algebras and Injectivity: The General Case. Indiana University Mathematics Journal, 26, 443-446. https://doi.org/10.1512/iumj.1977.26.26034
[8]	Dong, Z. and Ruan, Z.-J. (2007) Weak* Exactness for Dual Operator Spaces. Journal of Functional Analysis, 253, 373-397. https://doi.org/10.1016/j.jfa.2007.06.003
[9]	Effros, E.G. and Ruan, Z.-J. (2000) Operator Spaces. In: London Mathematical Society Monographs New Series 23, The Clarendon Press, Oxford University Press, New York.
[10]	Pisier, G. (2003) Introduction to Operator Space Theory. In: London Mathematical Society Lecture Note Series 294, Cambridge University Press, Cambridge. https://doi.org/10.1017/CBO9781107360235
[11]	Blecher, D.P. and Paulsen, V.I. (1991) Tensor Products of Operator Spaces. Journal of Functional Analysis, 99, 262-292. https://doi.org/10.1016/0022-1236(91)90042-4
[12]	Blecher, D.P. (1992) Tensor Products of Operator Spaces II. Canadian Journal of Mathematics, 44, 75-90. https://doi.org/10.4153/CJM-1992-004-5
[13]	Grothendieck, A. (1955) Une caractérisation vectorielle-métrique des espaces L1. Canadian Journal of Mathematics, 7, 552-561. https://doi.org/10.4153/CJM-1955-060-6
[14]	Effros, E.G. and Ruan, Z.-J. (1994) Mapping Spaces and Lifting for Operator Spaces. Proceedings of the London Mathematical Society, s3-69, 171-197. https://doi.org/10.1112/plms/s3-69.1.171
[15]	Effros, E.G. and Ruan, Z.-J. (1994) The Grothendieck-Pietsch and Dvoretzky-Rogers Theorem for Operator Spaces. Journal of Functional Analysis, 122, 428-450. https://doi.org/10.1006/jfan.1994.1075
[16]	Effros, E.G. and Ruan, Z.-J. (1997) On the Analogues of Integral Mappings and Local Reflexivity for Operator spaces. Indiana University Mathematics Journal, 46, 1289-1310. https://doi.org/10.1512/iumj.1997.46.1429
[17]	Dong, Z., Tao, J.-C. and Zhao, Y.-F. (2022) Nuclearity and Finite-Representability in the System of Completely Integral Mapping Spaces. Acta Mathematica Sinica, English Series. https://kns.cnki.net/kcms/detail/11.2039.O1.20221108.1403.002.html
[18]	Dong, Z. and Zhao, Y.-F. (2020) On the Nuclearity of Completely 1-Summing Mapping Spaces. Acta Mathematica Scientia, 40, 1981-1988. https://doi.org/10.1007/s10473-020-0623-6
[19]	Kirchberg, E. (1993) On Non-Semisplit Extensions, Tensor Products and Exactness of Group C*-Algebras. Inventiones Mathematicae, 112, 449-489. https://doi.org/10.1007/BF01232444