Bosonization Approach and Novel Traveling Wave Solutions of the Superfield Gardner Equation

Shuangte Wang¹, Hengguo Yu^1,3, Chuanjun Dai^2,3, Min Zhao^2,3

¹College of Mathematics and Physics, Wenzhou University, Wenzhou, China.
²School of Life and Environmental Science, Wenzhou University, Wenzhou, China.
³Key Laboratory for Subtropical Oceans & Lakes Environment & Biological Resources Utilization Technology of Zhejiang, Wenzhou University, Wenzhou, China.

DOI: 10.4236/jamp.2020.83034                

Abstract

In this paper, the bosonization of the superfield Gardner equation in the case of multifermionic parameters is presented and novel traveling wave solutions are extracted from the coupled bosonic equations by using the mapping and deformation relations. In the case of two-fermionic-parameter bosonization procedure, we provide a special solution in the form of Jacobian elliptic functions. Meanwhile, we discuss and formally derive traveling wave solutions of N fermionic parameters bosonization procedure. This technique can also be applied to treat the N = 1 supersymmetry KdV and mKdV systems which are obtained in two limiting cases.

Keywords

Supersymmetry, Superfield Gardner Equation, Bosonizaion, Traveling Wave Solutions

1. Introduction

The supersymmetry (SUSY), applied to treat fermions and bosons in a unified way in elementary particle physics since the concept first arose in 1971 by Ramond, Golfand and Likhtman, has been researched extensively during past four decades [1] - [6]. The starting point of SUSY is the supersymmetric versions of well known KdV equation first by Kupershmidt in 1984 (a simple fermionic but not supersymmetric extension) and later found independently of the work of Manin-Radual on super KP hierarchy [7] [8] [9] [10]. It was pointed out afterwards that the latter is indeed a truly N = 1 (N refers to the number of supersymmetries, for N = 1 standard, for N > 1 extended) sKdV equation which is invariant under supersymmetric transformation [8]. Since then, various properties have been established for its supersymmetric versions, such as Lax representation, bi-Hamiltonian structures, Backlund transformation (BT), Painleve analysis, N-soliton solutions, (non) local conserved quantities, etc. [9] - [15].

The integrability of sKdV equation can be established in the way to supersymmetrize the unique Gardner transformation. For the well known KdV equation

$u_t-6u{u}_x+u_{xxx}=0$ (1)

we extend the classical spacetime $x,t$ to a super-spacetime $x,t,\theta$, where $\theta$ is a Grassmannian odd number ${\theta }^2=0$. Now we write the N = 1 supersymmetric KdV (sKdV) equation accompanied with a fermionic super-variable $\Phi =\Phi \left(x,t,\theta \right)$ under the compact form

${\Phi }_t-3D^2\left(\Phi D\Phi \right)+D^6\Phi =0$ (2)

Here the covariant super-derivative D is defined by $D={\partial }_{\theta }+\theta {\partial }_x$. Mathieu found that a unique extension

$\Phi =\chi +\epsilon {\chi }_x+{\epsilon }^2\chi \left(D\chi \right)$ (3)

of establishing the integrability maps a solution of the superfield Gardner equation

${\chi }_t-3D^2\left(\chi D\chi \right)-3{\epsilon }^2\left(D\chi \right)D^2\left(\chi D\chi \right)+D^6\chi =0$ (4)

into a solution of the sKdV equation [8] [9] [15]. The Gardner equation is also called the extended KdV equation with the variable-sign cubic non-linear term or the combined KdV and mKdV (KdV-mKdV) equation. It is widely used in various branches of physics, such as plasma physics, fluid physics, nonlinear phenomena and quantum field theory, etc., and it also describes a variety of wave phenomena in plasma and solid state [16] [17] [18] [19] [20].

This map was also used to recover an infinite number of conservation laws for the sKdV equation, and construct interesting BT [6]. It is easy to show that such super equation is invariant under the supersymmetry transformation: $x\to x-\eta \theta$, $\theta \to \theta +\eta$ ( $\eta$ is an anticommuting parameter.). The component form of the above equation with the superfield $\chi =\xi +\theta u$ can be rewritten as

$u_t+u_{xxx}-6u{u}_x+3\xi {\xi }_{xx}-{\epsilon }^2\left[6u^2{u}_x-3{\left(\xi {\xi }_{x}u\right)}_x\right]=0$ (5a)

${\xi }_t+{\xi }_{xxx}-3{\left(\xi u\right)}_x-3{\epsilon }^{2}u{\left(\xi u\right)}_x=0$ (5b)

Note that $\xi$ and u are new setted fermionic and bosonic functions, the usual Gardner equation is recovered by setting the fermionic variable to be absent and the sKdV equation is the limiting case where $\epsilon \to 0$.

Nonlinear partial differential equations play an important role in nonlinear physics, even nonlinear science. Various effective methods have been proposed to derive explicit or formal solutions. Recently, a simple but powerful bosonization approach, which main idea is to consider fields in a Grassmannian algebra and rewrite a system in a basis of this algebra to arrive at a system of ordinary (commutative) evolution equations, can effectively simplify such systems containing anti-communicating fermionic fields [7] [21] [22]. In [23], B. Ren et al. used this approach in the N = 1 supersymmetric Burgers (SB) system, and the exact solutions of the usual pure bosonic systems are obtained with the mapping and deformation method and Lie point symmetries theory. In [24], the Lie point symmetries of the supersymmetric KdV-a system are considered and similarity reductions of it are conducted. Several types of similarity reduction solutions of the coupled bosonic equations are also simply obtained. The motivation and purpose of this paper is to show this procedure and outcome of the method by taking the superfield Gardner equation and to acquire novel traveling wave solutions of this equation.

The rest of this paper is organized as follows. Section 2 and 3 are brief reminders of fairly basic illustrations of the bosonization approach of the superfield Gardner equation with two and three fermionic parameters. In Section 4 we present the N fermionic parameters bosonization case. In Section 5 we give the N = 1 supersymmetric KdV and mKdV equations using parallel procedure in two particular cases, and we will also give a short summary.

2. Two-Fermionic-Parameter Bosonization

To get direct comprehension and fixed notations of the bosonizaion approach for superfield Gardner equation with multi-fermionic parameters, we first concentrate on a linear space $G\left(V\right)$. Mathematically, such uncomplicated method used for vanishing fermionic fields is based on direct sum of superspace $G\left(V\right)={\Lambda }_0\oplus {\Lambda }_1$, where ${\Lambda }_0$ and ${\Lambda }_1$ represents subspace containing even and odd elements, respectively [25]. Here and in the following we omit the exterior algebra sign $\wedge$ and denote it briefly by ordinary multiplication. Moreover, relevant solutions may involve rich (super) symmetries physically. An exact example is the cases of two and three fermionic parameters bosonization, thereby we can directly derive the usual Gardner equation and coupled equations appear below. For the case of two fermionic parameters ${\theta }_1$ and ${\theta }_2$ with ${\theta }_1^2={\theta }_2^2=0$, let the two component fields u and $\xi$ be expanded as

$u=u_0+u_1{\theta }_1{\theta }_2$, $\xi =v_1{\theta }_1+v_2{\theta }_2$, (6)

here $u_i=u_i\left(x,t\right)\left(i=0,1\right)$, $v_i=v_i\left(x,t\right)\left(i=1,2\right)$ are all usual bosonic functions with respect to spacetime variable x and t, thus we get nonlinear Partial Differential Equations (PDEs) in the component form by using (5)

$u_0{}_t+u_0{}_{xxx}-6u_0{u}_0{}_{x}f\left(u_0\right)=0$ (7a)

$v_{it}+v_{ixxx}-3{\left(v_i{u}_0\right)}_{x}f\left(u_0\right)=0$ (7b)

$u_{1t}+u_{1xxx}-6{\left(u_0{u}_1\right)}_{x}f\left(u_0\right)-6{\epsilon }^2{u}_0{u}_{0x}{u}_1+F_1=0$ (7c)

where $f\left(u_0\right)=1+{\epsilon }^2{u}_0$ and $F_1=3{\left[f\left(u_0\right)\left(v_1{v}_{2x}-v_2{v}_{1x}\right)\right]}_x$.

Next we introduce the traveling wave variable $X=kx+\omega t+x_0$ along with the constants of wavenumber k, angular frequency $\omega$ and phase $x_0$, therefore, above equations would be changed to a system consisting of ordinary differential equations(ODEs):

$\omega u_0{}_X+k^3{u}_0{}_{XXX}-6k{u}_0{u}_0{}_{X}f\left(u_0\right)=0$ (8a)

$\omega v_{iX}+k^3{v}_{iXXX}-3k{\left(v_i{u}_0\right)}_{X}f\left(u_0\right)=0\left(i=1,2\right)$ (8b)

$\omega u_{1X}+k^3{u}_{1XXX}-6k{\left(u_0{u}_1\right)}_{X}f\left(u_0\right)-6{\epsilon }^{2}k{u}_0{u}_{0X}{u}_1+F_1\left(X\right)=0$. (8c)

Note that we denote $F_1\left(X\right)=3k^2{\left[f\left(u_0\right)\left(v_1{v}_{2X}-v_2{v}_{1X}\right)\right]}_X$ in here.

The traveling waves we discuss are only in the usual spacetime $x,t$ but not in the super-spacetime $x,t,\theta$, for example, $\chi \left(x,t,\theta \right)=\chi \left(X+\theta \zeta \right)$ with Grassmannian constant $\zeta$ is different from those in the usual spacetime. In addition to a directly integrable ODE in $u_0$, the solution of the residual system which are related to third-order linear (non)homogeneous ODEs in $u_1$, $v_1$ and $v_2$, can be obtained through the variable transformation from ordinary coordinates space to phase space on the base of periodic wave solutions of usual Gardner equation. We first solve out $u_{0X}$ from Equation (8a), and the result reads

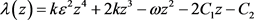$u_{0X}=\frac{a_0}{k^2}\sqrt{k\lambda \left(z\right)}$, (9)

where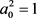, 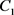and 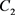are two arbitrary integral constants, here and below the new variable z stands for function.

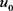To get the mapping relations of, 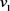and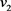, we introduce the variable transformations as follows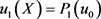, and 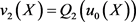. Applying the transformation via Equation (8a), the linear ODEs (8b)-(8c) are reduced to mapping and deformation relations between the traveling wave solutions of the classical Gardner equation and its supersymmetric equation by exploiting the known solutions of classical Gardner equation

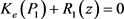(10a)

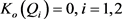(10b)

where linear operators read

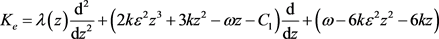(11a)

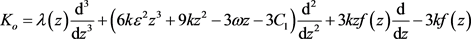(11b)

and the nonhomogeneous term 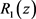is

. (12)

while is just an arbitrary integral constant. On this basis, the mapping and deformation relations are obtained as

(13a)

. (13b)

where and are arbitrary constants, and with auxiliary function . Coefficients for used are defined as , and.

Thus, we have constructed the general two-fermionic-parameter traveling wave solutions of the supersymmetric version of Gardner system

(14a)

(14b)

with the known solution or Equation (8a) of the usual Gardner equation which have been extensively studied in many literatures [18] [26]. For a special case which takes to eliminate nonhomogeneous terms, the above traveling wave solution becomes

(15)

It is interesting to see that the expression (9) is a trivial type of the symmetries or conservation quantity of standard Gardner equation ;,. In fact, for any given being a solution of the usual Gardner equation, a certain type of solutions of the bosonic-looking equation can be constructed as follows:, , , where represents any symmetry of the usual Gardner equation, and we have much freedom to choose such that it can construct solutions without restricting to the traveling wave type solutions and infinitely many symmetries of the superfield Gardner equations.

It is clear that the solution (9) can be expressed by the form of the Jacobian elliptic sine functions, i.e.,

,(16)

where, modulus m and constants are related to other known constants through following relations:

(17)

Therefore, we derive special type solutions of the superfield Gardner equation:

(18)

where

(19)

and are all arbitrary integral constants, the Jacobian elliptic functions are denoted as and, the incomplete elliptic integrals are denoted as and. We also note that for the critical modulus m = 1, above solutions read:

(20)

where

(21)

3. Three-Fermionic-Parameter Bosonization

For the case of three fermionic parameters with, let the two component fields u and be expressed as

, ( 22)

here and are all usual bosonic functions with respect to spacetime variable x and t. The symbol is the third-order Levi-Civita tensor. Then from (5), we get PDEs in component form as

(23a)

(23b)

(23c)

(23d)

where the somewhat complex nonhomogeneous terms read

(24a)

(24b)

Introducing the traveling wave variable with constants k, and and variable transformations and, similar to the previous case, the above bosonization system becomes following ODEs

(25a)

(25b)

(25c)

where nonhomogeneous terms are

(26a)

(26b)

with arbitrary integral constants. Mapping and deformation relations with respect to via traveling wave variable are constructed as

(27a)

(27b)

(27c)

where and are some arbitrary integral

constants, and functioin with integral constant.

Therefore, we have obtained the three-fermionic-parameter traveling wave solutions of the superfield Gardner system. While one of the Grassmann numbers tends to zero, the solution turns back to above section. Similar to the two-fermionic-parameter case, we write a special type solution

(28a)

(28b)

where are some constants, are arbitrary symmetries of the usual Gardner equation, and is an arbitrary solution of the usual Gardner equation.

4. N-Fermionic-Parameter Bosonization

Motivated by above sections, we repeat same procedure to get traveling wave solutions of the superfield Gardner equation via bosonizaion approach with N fermionic parameters here. The component fields u and can be expressed as

, (29)

Here and below we denote by the set of multi-indices which satisfies, and the upper bound of

summations. The elements and are bosonic smooth real or complex valued functions defined in the commutative algebra. Thus the super Gardner model (5) is transformed to a new pure bosonic-looking system with coupled nonlinear PDEs:

(30a)

(30b)

(30c)

Operators related to Gâteaux derivative of Equation (4) or Equations (5) with an operator decomposition read

, (31a)

(31b)

(31c)

and nonhomogeneous terms are

(32)

We have also used the shorthand notations

and

with

, ,

and for even case; for odd case. The parity function is defined in [25]. The symbol is the generalized Levi-Civita anti-symmetric tensor in m dimensions. Equation (30a) apparently suggests that it is the standard Gardner equation. Each solution in the case of fermionic parameters is a portion of solutions of the system consisting of n homologous Grassmannian variables. Introducing the traveling wave variable and variable transformations, mapping transformations are arranged as follows:

1) Equation of: (33a)

2) Equations of: (33b)

3) Equations of: (33c)

Here the nonhonogenerous terms are, each usual bosonic functions and are represented by and, respectively.

Finally, the mapping and deformation relations of and with N fermionic parameters can be formally rewritten as

(34a)

(34b)

in which auxiliary functions are

(35a)

(35b)

, and are some arbitrary integral constants.

5. Discussion and Summary

With relationships at hand, in case of preserving the square and cubic non-linear terms in Equations (5) or taking limitation, one can conveniently acquire the corresponding result of the integrable N = 1 sKdV regardless of some integral constants. Similarly to Section 4, the general traveling wave solutions of the N = 1 sKdV Equation (2) with N fermionic parameters are

(36a)

(36b)

where

.

Due to the case of taking limitation and the absence of non-linear quadratic term in Equation (4), traveling wave solutions of the usual integrable N = 1 smKdV Equation [10] [27]

(37)

with N-fermionic-parametric Bosonization procedure can be derived as

(38)

where

(39a)

(39b)

with

(40a)

(40b)

and

.

In summary, the bosonization approach with multi-fermionic parameters to deal with supersymmetric systems is developed in the super Gardner equation with the role of traveling wave solution. The procedure and technique are also available for N = 1 sKdV and smKdV equations derived from two particular cases. We expect this procedure exhibited in our paper could be successfully applied or formulated in the N = 1 supersymmetric sine-Gordon equation, especially in the N = 2 version of KdV (SKdVa) equations [8] [25] [28] [29] [30]. For

example, in the case of two fermionic parameters, letting, , in bosonic field, the

simple traveling wave solutions of the N = 1 supersymmetric Sine-Gordon equation are

(41a)

(41b)

where and are the usual super derivatives, S represents and, E, , , and are integral constants, is a solution of the standard sine-Gordon equation.

Acknowledgements

We thank the Editor and the referee for their comments. This research is funded by the National Natural Science Foundation of China (Grant nos. 31570364) and the National Key Research and Development Program of China (Grant No. 2018YFE0103700). This support is greatly appreciated.

Conflicts of Interest

The authors declare no conflicts of interest regarding the publication of this paper.

References

[1]	Kumarbagchi, B. (2001) Supersymmetry in Quantum and Classical Mechanics. Chapman & Hall/CRC, Boca Raton, FL.
[2]	Zee, A. (2003) Quantum Field in a Nutshell. Princeton University Princeton, Princeton, NJ.
[3]	Srednicki, M. (2007) Quantum Field Theory. Cambridge University Press, Cambridge. https://doi.org/10.1017/CBO9780511813917
[4]	Aitchison, I. (2007) Supersymmetry in Particle Physics: An Elementary Introduction. Cambridge University Press, Cambridge. https://doi.org/10.2172/903298
[5]	Tian, K. (2010) Symmetry Approach to N=1 Supersymmetric Systems. China-Japan Joint Workshop on Integrable Systems on the Occasion of Prof. Ryogo Hirota’s 77th Birthday, Shaoxing, 7-10 January 2010.
[6]	Hariton, A.J. (2006) Supersymmetric Extension of the Scalar Born-Infeld Equation. Journal of Physics A: Mathematical and General, 39, 7105-7114. https://doi.org/10.1088/0305-4470/39/22/021
[7]	Kupershmidt, B.A. (1984) A Super Korteweg-de Vries Equation: An Integrable System. Physics Letters, 102A, 6. https://doi.org/10.1016/0375-9601(84)90693-5
[8]	Mathieu, P. (1999) Open Problems for the Super KdV Equations. Lavalcphyc99c21.
[9]	Liu, Q.P. and Xie, Y.F. (2004) Nonlinear Superposition Formula for N=1 Supersymmetric KdV Equation. Physics Letters A, 325, 139-143. https://doi.org/10.1016/j.physleta.2004.03.047
[10]	Liu, Q.P., Hu, X.-B. and Zhang, M.-X. (2005) Supersymmetric Modified KortewegCde Vries Equation: Bilinear Approach. Nonlinearity, 18, 1597-1603. https://doi.org/10.1088/0951-7715/18/4/009
[11]	Liu, Q.P. and Manas, M. (1997) Darboux Transformation for the Manin-Radul Supersymmetric KdV Equation. Physics Letters B, 394, 337-342. https://doi.org/10.1016/S0370-2693(97)00026-9
[12]	Liu, Q.P. and Hu, X.-B. (2005) Bilinearization of N=1 Supersymmetric Korteweg-de Vries Equation Revisited. Journal of Physics A: Mathematical and General, 38, 6371-6378. https://doi.org/10.1088/0305-4470/38/28/009
[13]	Carstea, A.S., Ramani, A. and Grammaticos, B. (2001) Constructing the Soliton Solutions for the N=1 Supersymmetric KdV Hierarchy. Nonlinearity, 14, 1419-1423. https://doi.org/10.1088/0951-7715/14/5/325
[14]	Carstea, A.S. (2000)Extension of the Bilinear Formalism to Supersymmetric KdV-Type Equations. Nonlinearity, 13, 1645-1656. https://doi.org/10.1088/0951-7715/13/5/312
[15]	Andrea, S., Restuccia, A. and Sotomayor, A. (2009) New Conserved Quantities for N=1 SKdV, the Supersymmetric Cohomology and W-Algebras. arXiv:0911.5011v1.
[16]	Nakoulima, O., Zahibo, N., Pelinovsky, E., Talipova, T., Slunyaev, A. and Kurkin, A. (2004) Analytical and Numerical Studies of the Variable-Coefficient Gardner Equation. Applied Mathematics and Computation, 152, 449-471. https://doi.org/10.1016/S0096-3003(03)00569-1
[17]	Fu, Z.T., Liu, S.D. and Liu, S.K. (2004) New Kinds of Solutions to Gardner Equation. Chaos, Solitons and Fractals, 20, 301-309. https://doi.org/10.1016/S0960-0779(03)00383-7
[18]	Zhang, L.-H., Dong, L.-H. and Yan, L.-M. (2008) Construction of Non-Travelling Wave Solutions for the Generalized Variable-Coefficient Gardner Equation. Applied Mathematics and Computation, 203, 784-791. https://doi.org/10.1016/j.amc.2008.05.084
[19]	Wazwaz, A.-M. (2008) Solitons and Singular Solitons for the Gardner CKP Equation. Applied Mathematics and Computation, 204, 162-169. https://doi.org/10.1016/j.amc.2008.06.011
[20]	Lu, D.C. and Liu, C.L. (2010) A Sub-ODE Method for Generalized Gardner and BBM Equation with Nonlinear Terms of Any Order. Applied Mathematics and Computation, 217, 1404-1407. https://doi.org/10.1016/j.amc.2009.05.049
[21]	Andrea, S., Restuccia, A. and Sotomayor, A. (2001) An Operator Valued Extension of the Super Korteweg Cde Vries Equations. Journal of Mathematical Physics, 42, 2625-2634. https://doi.org/10.1063/1.1368139
[22]	Gao, X.N. and Lou, S.Y. (2012) Bosonization of Supersymmetric KdV Equation. Physics Letters B, 707, 209-215. https://doi.org/10.1016/j.physletb.2011.12.021
[23]	Ren, B., Gao, X.-N., Yu, J. and Liu, P. (2015) Exact Solutions of Supersymmetric Burgers Equation with Bosonization Procedure. Open Mathematics, 13, 502-509. https://doi.org/10.1515/math-2015-0047
[24]	Gao, X.N., Yang, X.-D. and Lou, S.-Y. (2012) Exact Solutions of Supersymmetric KdV-a System via Bosonization Approach. Communications in Theoretical Physics. Communications in Theoretical Physics, 58, 617-622. https://doi.org/10.1088/0253-6102/58/5/01
[25]	Fan, E.G. and Hon, Y.C. (2010) Quasiperiodic Wave Solutions of N=2 Supersymmetric KdV Equation in Superspace. Studies in Applied Mathematics, 125, 343-371. https://doi.org/10.1111/j.1467-9590.2010.00491.x
[26]	Bai, C.-L., Bai, C.-J. and Zhao, H. (2005) A New Generalized Algebraic Method and Its Application in Nonlinear Evolution Equations with Variable Coefficients. Zeitschrift für Naturforschung, 60, 211-220. https://doi.org/10.1515/zna-2005-0401
[27]	Ghosh, S. and Sarma, D. (2003) Bilinearization of N=1 Supersymmetric Modified KdV Equations. Nonlinearity, 16, 411-418. https://doi.org/10.1088/0951-7715/16/2/302
[28]	Carstea, A.S., Ramani, A. and Grammaticos, B. (2002) Linearisable Supersymmetric Equations. Chaos, Solitons and Fractals, 14, 155-158. https://doi.org/10.1016/S0960-0779(01)00130-8
[29]	Grammaticos, B., Ramani, A. and Carstea, A.S. (2001) Bilinearization and Soliton Solutions of the N=1 Supersymmetric Sine-Gordon Equation. Journal of Physics A: Mathematical and General, 34, 4881-4886. https://doi.org/10.1088/0305-4470/34/23/307
[30]	Popowicz, Z. (1998) Integrable Extensions of N=2 Supersymmetric KdV Hierarchy Associated with the Nonuniqueness of the Roots of the Lax Operator. Physics Letters A, 249, 204-208. https://doi.org/10.1016/S0375-9601(98)00731-2