Algorithms for Common Solutions to Generalized Mixed Equilibrium Problems and Fixed Point Problems under Nonlinear Transformations in Banach Spaces

Yanlai Song, Xinhong Chen

College of Science, Zhongyuan University of Technology, Zhengzhou, China.

DOI: 10.4236/jamp.2019.711179                

Abstract

The purpose of this paper is to present a new iterative scheme for finding a common solution of the generalized mixed equilibrium problems with an infinite family of inverse strongly monotone mappings and the fixed point problems of demimetric mappings under nonlinear transformations in Banach spaces. Applications are also included. The results in this paper are the extension and improvement of the recent results in the literature.

Keywords

Fixed Point, Demimetric Mapping, Generalized Mixed Equilibrium Problems, Banach Space

1. Introduction

Let H be a real Hilbert space, C be a nonempty closed convex subset of H, T be a mapping on C and. Let 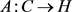be a nonlinear mapping, 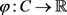be a function and F be a bifunction from to, where is the set of real numbers. Then, we consider the following generalized mixed equilibrium problem (for short, GMEP): finding 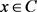such that

(1)

The set of solutions of the GMEP is denoted by 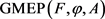(see [1] and the references therein). Here some special cases of the GMEP are stated as followings:

1) If, then the GMEP becomes the following mixed equilibrium problem (for short, MEP):

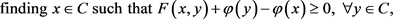(2)

which was studied by Ceng and Yao [2]. The set of solutions of the MEP is denoted by.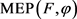

2) If 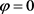and, then the GMEP becomes the following equilibrium problem (for short, EP):

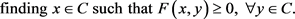(3)

This general form of the EP was first considered by Nikaido and Isoda [3]. The MEP and EP play an important role in many fields, such as economics, physics, mechanics and engineering sciences. Also, the MEP and EP include many mathematical problems as particular cases, for example, mathematical programming problems, complementary problems, variational inequality problems, Nash equilibrium problems in noncooperative games, minimax inequality problems and fixed point problems. Because of their wide applicability, equilibrium problems and mixed equilibrium problems have been generalized in various directions for the past several years; see, for example, [2] [4] - [9].

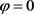3) If and, then the GMEP reduces to the following classical variational inequality problem (for short, VIP) [10] :

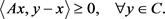(4)

Since the VIP inception by Stampacchia [10] in 1964, it has received much attention due to its applications in a large variety of problems arising in structural analysis, economics, optimization, operations research and engineering sciences. Using the projection technique, one can easily show that is equivalent to the fixed-point problem; see, [7] [8] [11] [12] and the references therein.

Motivated by Ceng and Yao [2], Nikaido and Isoda [3] and Stampacchia [10], Peng and Yao [1] introduced the GMEP, which can be viewed as development and extension of the MEP, the EP and the VIP. It shows that the GMEP has applications in physics, economics, finance, transportation, network and structural analysis, therapy, image reconstruction, and elasticity. The GMEP includes special cases, MEPs, EPs, VIPs, fixed point problems, complementarity problems, optimization problems, Nash equilibrium problems in noncooperative games, etc (see e.g., [6] [7] [8] and the references contained in them). In other words, the GMEP is a unifying model for several problems arising in several areas of study. In general, the GMEP involves nonlinear equations and there are no known methods to obtain closed form solutions for them. Consequently, several methods are being deployed to approximate their solutions, assuming existence. A number of iterative methods have been utilized to solve equilibrium problems, generalized equilibrium problems and mixed equilibrium problems (see e.g., [2] [4] [5] [13] and the references therein).

Related to the GMEP, the problem of finding the fixed points for nonlinear mappings is the subject of current interest in functional analysis. It turns out that the fixed point theory for nonlinear mappings can be applied to several nonlinear problems such as zero point problems for monotone operators, convex feasibility problems, convex minimization problems, variational inequality and equilibrium problems, and so on; see [14] - [19] for more details.

At the same time, to construct a mathematical model which is as close as possible to a real complex problem, we often have to use more than one constraint. Solving such problems, we have to obtain some solution which is simultaneously the solution of two or more subproblems or the solution of one subproblem on the solution set of another subproblem. These subproblems can be given, for example, by two or more different variational inequality problems or two or more different fixed point problems. It is natural to construct a unified approach for these problems. In this direction, several authors have introduced some iterative schemes for finding a common solution of fixed-point problems for nonlinear mappings, equilibrium problems and variational problems; see, for example, [1] [2] [9] [12] [19] [20] and the references therein.

Recently, Takahashi [21] introduced a broad class of nonlinear mappings in a Banach space called k-demimetric mapping. This class mapping contains the classes of generalized hybrid mappings, k-strict pseudo-contractions, firmly-quasi-nonexpansive mappings, quasi-nonexpansive mappings and demicontractive mappings.

Definition 1.1 Let E be a smooth Banach space and let C be a nonempty, closed and convex subset of E. Let k be a real number with. A mapping with is called k-demimetric if, for any and,

(5)

We give an example of a k-demimetric mapping which is not pseudo-contractive, hence it is not strictly pseudo-contractive.

Example 1.2 ( [22] ) Let H be the real line and. Define T on C by if and. Clearly, 0 is the only fixed point of T. Also, for, for any. Thus T is demimetric.

In order to find a common solution of fixed point problems for an finite family of demimetric mappings and the variational inequality problems for a infinite family of inverse strongly monotone mappings in a Hilbert space, Takahashi [12] recently introduced and studied the following iterative algorithm:

where is a finite family of k_j-demimetric and demiclosed mappings, and is a finite family of -inverse strongly monotone mappings. Then he obtained a strong convergence theorem under some mild restrictions on the parameters.

Very recently, Akashi and Takahashi [14] proposed the following Mann’s type iteration for finding a common solution of fixed-point problems for an infinite family of demimetric mappings without assuming that demimetric mappings are commutative:

where is an infinite family of k_j-demimetric and demiclosed mappings. Then they obtained a weak convergence theorem under certain appropriate assumptions on the parameters.

Most very recently, Takahashi [15] also introduced the following iteration process for finding a common solution of fixed-point problems with an infinite family of demimetric mappings and the variational inequality problems with an infinite family of inverse strongly monotone mappings in a Hilbert space:

where is an infinite family of k_j-demimetric and demiclosed mappings, is an infinite family of -inverse strongly monotone mappings. Then they obtained a strong convergence theorem under some mild restrictions on the parameters.

On other hand, in order to find a common solution of equilibrium problems and the set of fixed point problems with generalized hybrid mappings, Alizadeh and Moradlou [23] introduced the following Ishikawa-like iteration process by applying the hybrid projection method:

where S is a generalized hybrid mapping and f is a bifunction satisfying (A1)-(A4). Then they obtained a strong convergence theorem under certain appropriate assumptions on the parameters.

Motivated and inspired by Takahashi [12], Akashi and Takahashi [14], Takahashi [15], Alizadeh and Moradlou [23], we put forward two questions:

1) Can these corresponding results in [12] [14] [15] [23] in Hilbert spaces be extended to the framework of Banach spaces (for example, for)?

2) Can we extend corresponding results in [12] [14] [15] [23] from finding a solution of the fixed point problems of generalized hybrid mappings or a common solution of the equilibrium problems and fixed point problems of generalized hybrid mappings to the more general and challenging problem for finding a common solution of the generalized mixed equilibrium problems and the fixed point problems of demimetric mappings under nonlinear transformations?

The purpose of this paper is to give the affirmative answers to these questions mentioned above. In this paper, we present a new iterative scheme for finding a common solution of the generalized mixed equilibrium problems and fixed point problems of demimetric mappings under nonlinear transformations in Banach spaces. Applications are also included. Our results improve essentially the corresponding results in [12] [14] [15] [23]. Further, some other results are also improved; see [9] [11] [16] [17] [18] [20] [24] [25].

2. Preliminaries

We denote E the real Banach space, the dual of E, I the identity mapping on E, and the set of positive integers. The expressions and denote the strong and weak convergence of the sequence, respectively. The (normalized) duality mapping J from E to is defined by

for all, where denotes the duality product. If E is a Hilbert space, then, where I is the identity mapping on H.

The norm of a Banach space E is said to be Gâteaux differentiable if the limit

exists for all on the unit sphere. In this case, we say that E is smooth.

A Banach space E is said to be strictly convex if whenever and. It is known that if E is strictly convex, then the duality mapping J is injective, that is, and imply. It is known that E is reflexive if and only if J is surjective. Therefore, if E is a smooth, strictly convex and reflexive Banach space, then J is a single-valued bijection, see [26] for more details.

Definition 2.1 A mapping is said to be:

1) nonexpansive if for all;

2) contractive if there exists a constant such that

3) -demicontractive if there exists a constant such that

We use to denote the collection of mappings T verifying the above inequality. That is

Let D be a nonempty subset of C. A sequence of mappings of C into H is said to be stable on D (see [27] ) if is a singleton for every. It is clear that if is stable on D, then for all and.

Lemma 2.2 In a Hilbert space H, it holds for all and that

which can be extended to the more general situation: for all, , and, we have

Lemma 2.3 ( [19] ) Let be a sequence of real numbers such that there exists a subsequence of such that for all. Then there exists a nondecreasing sequence such that and the following properties are satisfied for all (sufficiently large) numbers:

In fact,.

Lemma 2.4 ( [28] ) Let be a sequence of nonnegative numbers satisfying the property:

where satisfy the restrictions:

1),

2),

3).

Then,.

Lemma 2.5 ( [21] ) Let E be a smooth, strictly convex and reflexive Banach space and let be a real number with. Let U be an -demimetric mapping of E into itself. Then is closed and convex.

Lemma 2.6 ( [16] ) Let be a metric projection from H on a nonempty closed convex subset C of H. Given and, then if and only if there holds the relation

Recall that a mapping is said to be -inverse-strongly monotone (ism) if there exists a constant such that

Lemma 2.7 ( [29] ) If is -ism and is any constant in, then the mapping is nonexpansive.

For solving the generalized mixed equilibrium problem, let us assume that the bifunction and the nonlinear mapping satisfy the following conditions:

(A1) for all;

(A2) F is monotone, i.e., for all;

(A3) for each fixed, is weakly upper semicontinuous;

(A4) for each fixed, is convex and lower semicontinuous;

(A5) for each and, there exists a bounded subset and such that, for any,

(A6) C is a bounded set.

Lemma 2.8 [2] Let C be a nonempty, closed and convex subset of H and let be a bifunction satisfying (A1)-(A4). Let be a proper lower semicontinuous and convex function. Assume that either (B1) or (B2) holds. For and, define a mapping as follows:

Then, the following conclusions hold:

1) For each, and is single-valued;

2) is a firmly nonexpansive mapping, i.e., for all,

3);

4) is closed and convex;

5) for all and.

3. Main Results

Throughout the rest of this paper, we always assume the following:

1) H is a real Hilbert space, and C is a nonempty closed subspace of H;

2) E is a smooth, strictly convex and reflexive Banach space, and J is the duality mapping on E;

3) F is a bifunction from to satisfying (A1)-(A4);

4) is a mapping defined as in Lemma 2.8;

5) is an infinite family of -ism mappings with ;

6) is a lower semicontinuous and convex function with restrictions (B1) or (B2);

7) is a bounded linear operator such that and is the adjoint operator of B;

8) is an infinite family of -demimetric and demiclosed mappings with;

9);

10) is stable on.

Theorem 3.1 For any, define a sequence as follows:

(6)

where, and satisfy the following conditions:

(i) and,

(ii) and,

(iii),

(iv) and,

(v).

Then the sequence generated by (6) converges strongly to a point, where.

Proof. Set for all and. Then we can prove that T is well defined. In fact, we have, for any and,

(7)

which implies

(8)

Thus,

Then we see the mapping converges absolutely for each x in C.

Furthermore, define for all and. Then we can prove that is nonexpansive. Indeed, it follows that is nonexpansive from (v), Lemma 2.7 and Lemma 2.8(2). We obtain from Lemma 2.5 that, for any,

Thus converges absolutely for each.

Since is nonexpansive, we have that is closed and convex. Furthermore, we know from Lemma 2.5 that is closed and convex for each. Therefore, we have that is nonempty, closed and convex (note that B is linear and continuous). Thus we have that is well defined.

We derive from Lemma 2.8 that

(9)

Noting (8), we have

(10)

It follows from (9), (10) and (v) that

By induction, we obtain

which gives that the sequence is bounded, so are, and.

We obtain from (7) that

(11)

It follows from (9), (11) and Lemma 2.2 that

which means that

(12)

Case 1. Assume there exists some integer such that is decreasing for all. In this case, we deduce that exists. From (12), conditions (i), (ii), (iii) and (v), we deduce

(13)

and

(14)

From (6) and (13), we get that

Hence, we have

(15)

Since is bounded, there exists a subsequence of satisfying. Without loss of generality, we may also assume

(16)

Because B is bounded and linear, we see that. This together with (13) implies for each. And hence,.

Next let us prove that. Noticing that a nonexpansive mapping with is 0-demimetric, then we have

This together with (14) and (15) implies, for any, that

(17)

Consider a subsequence of corresponding to the sequence. Since the subsequence of is bounded, we have that there exists a subsequence of such that. For such r, we have from Lemma 2.8 (5) that

On the other hand, since and are Lipscitz, noting (17), we infer for any that

(18)

Therefore, we obtain.

It follows from (16) and Lemma 2.6 that

(19)

Putting for all, we have from (6) that . Since is stable on, we then get by (9), (10) and Lemma 2.6 that

This together with Lemma 2.4 and (19) implies as.

Case 2: Suppose that there exists of such that for all. Then by Lemma 2.3, there exists a nondecreasing sequence in such that

(20)

Without loss of generality, there exists a subsequence of such that for some and

We show that

(21)

where. To see this, we can first obtain by a similar argument as in Case 1. Therefore, we deduce that

(22)

Like in Case 1, we can also get that

(23)

Observing that

we then find from (23) and (i) that

(24)

Putting for all, we obtain by Lemma 2.6, (9), (10) and (20) that

which means that

Noticing (22) and (24), we deduce

We can also obtain by (20) that

Consequently, we get as.

Remark 3.2 Theorem 3.1 extends, improves and develops Theorem 3.1 of Takahashi [12], Theorem 3.1 of Akashi and Takahashi [14], Theorem 3.1 of Takahashi [15] and Theorem 3.1 of Alizadeh and Moradlou [23] in the following aspects:

· Theorem 3.1 improves and develops corresponding results in [23] from generalized hybrid mappings to demimetric mappings;

· Theorem 3.1 extends, improves and develops corresponding results in [12] [14] and [15] from finding a common solution of fixed-point problems and the variational inequality problems in Hilbert spaces to the more general and challenging problem for finding a common solution of the generalized mixed equilibrium problems and the null point problems in Banach spaces;

· The proof of our Theorem 3.1 is very different from the proof of the ones given in [12] [14] [15] [23] ;

· The algorithm 6 is more advantageous and more flexible than the ones given in [12] [14] [15] [23]. Therefore, the new algorithm is expected to be widely applicable.

4. An Extension of Our Main Results

From Theorem 3.1, we deduce immediately the following results

Corollary 4.1 Suppose. For, define a sequence as follows:

(25)

where, and satisfy the following conditions:

1) and,

2) and,

3) and.

Then the sequence generated by (25) converges strongly to a point , where.

Corollary 4.2 Let be an infinite family of directed and demiclosed mappings. For, define a sequence as follows:

(26)

where, and satisfy the following conditions:

1) and,

2) and,

3),

4) and,

5).

Then the sequence generated by (26) converges strongly to a point , where.

Proof. Noticing that a directed mapping T with is 0-demimetric, then we have the desired result due to Theorem 3.1.

5. Numerical Examples

In this section, we discuss the direct application of Theorem 3.1 on a typical example on a real line.

Example 5.1 Let with the inner product defined by for all and the standard norm. Let, , and be defined by

It is easy to check that, is an infinite family of -demimetric and demiclosed mappings, is an infinite family of -ism mappings, and is -contractive on H and stable on. Let for all, then we see satisfies (A5).

Letting for all, we then see B is a bounded linear operator with its adjoint. Note that. Define a bifunction by

We then find that F satisfies (A1)-(A4). So, by Lemma 2.8, we have is nonempty and single-valued for each. Hence, for any, there exists such that

which is equivalent to

After solving the above inequality, we get, i.e..

Let us choose, , , , , and (choosing other values of these variables arbitrarily which satisfy the conditions of Theorem 3.1, the same convergence result also can be obtained). Then, , , , , and satisfy all the conditions of Theorem 3.1. Then (6) can be rewrite as

It is not hard to estimate that

which shows.

6. Conclusion

The present work has been aimed to theoretically establish a new iterative scheme for finding a common solution of the generalized mixed equilibrium problems with an infinite family of inverse strongly monotone mappings and the fixed point problems of demimetric mappings under nonlinear transformations in Banach spaces. Our results can be viewed as improvement, supplementation, development and extension of the corresponding results in some references to a great extent.

Acknowledgements

This research was supported by the Key Scientific Research Projects of Higher Education Institutions in Henan Province (20A110038).

Conflicts of Interest

The authors declare no conflicts of interest regarding the publication of this paper.

References

[1]	Peng, J.W. and Yao, J.C. (2008) A New Hybrid-Extragradient Method for Generalized Mixed Equilibrium Problems, Fixed Point Problems and Variational Inequality Problems. Taiwanese Journal of Mathematics, 12, 1401-1432. https://doi.org/10.11650/twjm/1500405033 https://projecteuclid.org/euclid.twjm/1500405033
[2]	Ceng, L.C. and Yao, J.C. (2008) A Hybrid Iterative Scheme for Mixed Equilibrium Problems and Fixed Point Problems. Journal of Computational and Applied Mathematics, 214, 186-201. https://doi.org/10.1016/j.cam.2007.02.022 https://linkinghub.elsevier.com/retrieve/pii/S0377042707001069
[3]	Nikaido, H. and Isoda, K. (1955) Note on Non-Cooperative Convex Game. Pacific Journal of Mathematics, 5, 807-815. https://doi.org/10.2140/pjm.1955.5.807 https://projecteuclid.org/euclid.pjm/1171984836
[4]	Cholamjiak, W., Cholamjiak, P. and Suantai, S. (2015) Convergence of Iterative Schemes for Solving Fixed Point Problems for Multi-Valued Nonself Mappings and Equilibrium Problems. Journal of Nonlinear Sciences and Applications, 8, 1245-1256. https://mathscinet.ams.org/mathscinet-getitem?mr=3389563
[5]	Qin, X., Cho, Y.J. and Kang, S.M. (2010) Strong Convergence of Shrinking Projection Methods for Quasi-Nonexpansive Mappings and Equilibrium Problems. Journal of Computational and Applied Mathematics, 234, 750-760. https://mathscinet.ams.org/mathscinet-getitem?mr=2606662
[6]	Moudafi, A. and Thera, M. (1999) Proximal and Dynamical Approaches to Equilibrium Problems. Lecture Notes in Economics and Mathematics Systems, 477, 187-201. https://doi.org/10.1007/978-3-642-45780-7_12 https://mathscinet.ams.org/mathscinet-getitem?mr=1737320
[7]	Blum, E. and Oettli, W. (1994) From Optimization and Variational Inequalities to Equilibrium Problems. MathStudio, 63, 123-145. https://mathscinet.ams.org/mathscinet-getitem?mr=1292380
[8]	Dafermos, S. and Nagurney, A. (1984) A Network Formulation of Market Equilibrium Problems and Variational Inequalities. Operations Research Letters, 3, 247-250. https://doi.org/10.1016/0167-6377(84)90055-5 https://mathscinet.ams.org/mathscinet-getitem?mr=777640
[9]	Yao, Y.H., Zhou, H.Y. and Liou, Y.C. (2009) Weak and Strong Convergence Theorems for an Asymptotically K-Strict Pseudo-Contraction and a Mixed Equilibrium Problem. Journal of the Korean Mathematical Society, 46, 561-576. https://doi.org/10.4134/JKMS.2009.46.3.561 https://mathscinet.ams.org/mathscinet-getitem?mr=2515136
[10]	Stampacchia, G. (1964) Formes bilinéaires coercitives sur les ensembles convexes. Comptes Rendus Mathematique Academie des Sciences, Paris, 258, 4413-4416. https://mathscinet.ams.org/mathscinet-getitem?mr=166591
[11]	Yao, Y.H., Postolache, M., Liou, Y.C. and Yao, Z.S. (2016) Construction Algorithms for a Class of Monotone Variational Inequalities. Optimization Letters, 10, 1519-1528. https://doi.org/10.1007/s11590-015-0954-8 https://mathscinet.ams.org/mathscinet-getitem?mr=3544127
[12]	Takahashi, W. (2017) Strong Convergence Theorem for a Finite Family of Demimetric Mappings with Variational Inequality Problems in a Hilbert Space. Japan Journal of Industrial and Applied Mathematics, 34, 41-57. https://doi.org/10.1007/s13160-017-0237-0 https://mathscinet.ams.org/mathscinet-getitem?mr=3625838
[13]	Cho, Y.J., Qin, X. and Kang, S.M. (2009) Convergence Theorems Based on Hybrid Methods for Generalized Equilibrium Problems and Fixed Point Problems. Nonlinear Analysis, 71, 4203-4214. https://linkinghub.elsevier.com/retrieve/pii/S0362546X09003915
[14]	Akashi, S. and Takahashi, W. (2016) Weak Convergence Theorem for an Infinite Family of Demimetric Mappings in a Hilbert Space. Journal of Nonlinear and Variational Analysis, 10, 2159-2169. https://mathscinet.ams.org/mathscinet-getitem?mr=3583324
[15]	Takahashi, W. (2017) Weak and Strong Convergence Theorem for Families of Nonlinear and Nonself Mappings in Hilbert Spaces. Journal of Nonlinear and Variational Analysis, 1, 1-23. http://jnva.biemdas.com/issues/JNVA2017-1-1.pdf
[16]	Marino, G. and Xu, H.K. (2007) Weak and Strong Convergence Theorems for Strict Pseudo-Contractions in Hilbert Spaces. Journal of Mathematical Analysis and Applications, 329, 336-349. https://doi.org/10.1016/j.jmaa.2006.06.055 https://mathscinet.ams.org/mathscinet-getitem?mr=2306805
[17]	Matsushita, S. and Kuroiwa, D. (2003) Approximation of Fixed Points of Nonexpansive Nonself-Mappings. Japanese Journal of Mathematics, 57, 171-176. https://mathscinet.ams.org/mathscinet-getitem?mr=1156581
[18]	Qin, X.L., Dehaishb, B., Latif, A. and Cho, S.Y. (2016) Strong Convergence Analysis of a Monotone Projection Algorithm in a Banach Space. Journal of Nonlinear Sciences and Applications, 9, 2865-2874. https://mathscinet.ams.org/mathscinet-getitem?mr=3491153
[19]	Mainge, P.E. (2007) Approximation Methods for Common Fixed Points of Nonexpansive Mappings in Hilbert Spaces. Journal of Mathematical Analysis and Applications, 325, 469-479. https://doi.org/10.1016/j.jmaa.2005.12.066 https://mathscinet.ams.org/leavingmsn?url=https://doi.org/10.1016/j.jmaa.2005.12.066
[20]	Takahashi, S. and Takahashi, W. (2008) Strong Convergence Theorem for a Generalized Equilibrium Problem and a Nonexpansive Mapping in a Hilbert Space. Nonlinear Analysis, 69, 1025-1033. https://doi.org/10.1016/j.na.2008.02.042 https://linkinghub.elsevier.com/retrieve/pii/S0362546X08001107
[21]	Takahashi, W. (2016) The Split Common Null Point Problem and the Shrinking Projection Method in Banach Spaces. Optimization, 65, 281-287. https://doi.org/10.1080/02331934.2015.1020943
[22]	Chidume, C. and Maruster, S. (2010) Iterative Methods for the Computation of Fixed Points of Demicontractive Mappings. Journal of Computational and Applied Mathematics, 234, 861-882. https://doi.org/10.1016/j.cam.2010.01.050 https://www.sciencedirect.com/science/article/pii/S0377042710000634
[23]	Alizadeh, S. and Moradlou, F. (2016) A Strong Convergence Theorem for Equilibrium Problems and Generalized Hybrid Mappings. Mediterranean Journal of Mathematics, 13, 379-390. https://doi.org/10.1007/s00009-014-0462-6 https://mathscinet.ams.org/mathscinet-getitem?mr=3456928
[24]	Eslamian, M., Eskandani, G.Z. and Raeisi, M. (2017) Split Common Null Point and Common Fixed Point Problems between Banach Spaces and Hilbert Spaces. Mediterranean Journal of Mathematics, 14, 119. https://doi.org/10.1007/s00009-017-0922-x https://mathscinet.ams.org/mathscinet-getitem?mr=3635959
[25]	Takahashi, S., Takahashi, W. and Toyoda, M. (2010) Strong Convergence Theorems for Maximal Monotone Operators with Nonlinear Mappings in Hilbert Spaces. Journal of Optimization Theory and Applications, 147, 27-41. https://doi.org/10.1007/s10957-010-9713-2 https://mathscinet.ams.org/mathscinet-getitem?mr=2720590
[26]	Takahashi, W. (2000) Convex Analysis and Approximation of Fixed Points, Yokohama Publishers, Yokohama. https://mathscinet.ams.org/mathscinet-getitem?mr=1875068
[27]	Aoyama, K. and Kohsaka, F. (2014) Viscosity Approximation Process for a Sequence of Quasinonexpansive Mappings. Fixed Point Theory and Applications, 2014, 17. https://doi.org/10.1186/1687-1812-2014-17 https://fixedpointtheoryandapplications.springeropen.com/articles/10.1186/1687-1812-2014-17
[28]	Xu, H.K. (2002) Iterative Algorithms for Nonlinear Operators. Journal of the London Mathematical Society, 66, 240-256. https://doi.org/10.1112/S0024610702003332 https://academic.oup.com/jlms/article/66/1/240/815281
[29]	Takahashi, W., Xu, H.K. and Yao, J.C. (2015) Iterative Methods for Generalized Split Feasibility Problems in Hilbert Spaces. Set-Valued and Variational Analysis, 23, 205-221. https://doi.org/10.1007/s11228-014-0285-4 https://sci-hub.tw/10.1007/s11228-014-0285-4