Abstract

In this piece of work, using three spatial grid points, we discuss a new two-level implicit cubic spline method of O(k² + kh² + h⁴) for the solution of quasi-linear parabolic equation, 0< x <1, t > 0 subject to appropriate initial and Dirichlet boundary conditions, where h > 0, k > 0 are grid sizes in space and time-directions, respectively. The cubic spline approximation produces at each time level a spline function which may be used to obtain the solution at any point in the range of the space variable. The proposed cubic spline method is applicable to parabolic equations having singularity. The stability analysis for diffusionconvection equation shows the unconditionally stable character of the cubic spline method. The numerical tests are performed and comparative results are provided to illustrate the usefulness of the proposed method.

1. Introduction

The use of cubic splines for the numerical solution of linear two point boundary value problems has been discussed by Bickley [1], Fyfe [2], Albasiny and Hoskins [3] and Rubin and Khosla [4]. Later, Chawla et al [5,6] have developed fourth order accurate cubic spline methods for singular two point boundary value problems. In 1983, Jain and Aziz [7] have derived fourth order cubic spline method for the solution of general two point non-linear boundary value problems. Khan and Aziz [8] have used parametric cubic spline approach for the solution of a system of second order boundary value problems. Recently, Monoj Kumar et al [9-11], and Rashidinia et al [12-13] have discussed higher order cubic spline finite difference method for singular two point boundary value problems. A cubic spline technique for the solution of 1D heat equation was discussed by Papamichael and Whiteman [14]. This technique was extended by Fleck Jr [15] and Raggett and Wilson [16] for solving one-dimensional wave equation. Archer [17] has developed a fourth order collocation method for quasi-linear parabolic equation. Jain and Lohar [18] have solved non-linear parabolic equations by using second order cubic spline method. Recently, Rashidinia et al [19] have studied nonpolynomial cubic spline methods for the solution of parabolic equations. High order finite difference methods for the solution of non-linear parabolic equations have been discussed by Jain et al [20] and Mohanty et al [21-22]. In the present paper, using three spatial grid points (see Figure 1), a new cubic spline technique similar to that of Jain and Aziz [7] is developed for the solution of one dimensional general quasi-linear parabolic equation. We use the cubic spline approximation in the space direction together with a finite difference approximation in the time direction. This approximation produces at each time level a cubic spline function, which may be used to obtain the solution at any point in the range of the space variable. In next section, we discuss the cubic spline method. In Section 3, we give the complete derivation of the method. Stability of a linear difference scheme, which is consistent with diffusionconvection equation, is discussed in Section 4 and it is shown that the linear scheme is unconditionally stable. Difficulties were experienced in the past for the high order cubic spline solution of parabolic equations with

singular coefficients. The solution usually deteriorates in the vicinity of the singularity. In this paper, we refine our technique in such a way that the solution retains its order and accuracy everywhere in the solution region. In Section 5, we compared the proposed cubic spline method with the corresponding finite difference methods discussed in [20-22]. Finally, concluding remarks are given in Section 6.

2. The Two Level Implicit Cubic Spline Method

Consider the quasi-linear parabolic equation in which the function u(x,t) satisfies

(1)

where the solution space is defined by.

The initial condition is given by

(2)

and the boundary conditions are given by

(3)

where and are given smooth functions.

The region is covered in the usual manner by a rectangular net, , where (N + 1)h = 1, and h > 0 and k > 0 are grid sizes in xand t-directions, respectively. The mesh ratio parameter is given by. Let be the exact solution value of u(x,t) and denotes a discrete approximation to u(x,t) at the grid point.

We let denote the cubic spline interpolating the value at the jth time level, and is given by

(4)

which satisfies at jth-level the following properties:

1)    coincides with a polynomial of degree three on each, j > 0

2)    and

3)    ,

and where

and

.

We consider the following approximations:

(5)

(6a)

(6b)

(7a)

(7b)

(8a)

(8b)

(9a)

(9b)

(10a)

(10b)

(10c)

where

    etc.

Further, we define

(11a)

(11b)

Then at each grid point, the cubic spline method with accuracy of O(k² + kh² + h⁴) for the solution of differential Equation (1) may be written as

(12)

where for and.

Note that, the initial and Dirichlet boundary conditions are given by (2) and (3), respectively. Incorporating the initial and boundary conditions, we can write the cubic spline method (12) in a tri-diagonal matrix form. If the differential Equation (1) is linear, we can solve the linear system using a tri-diagonal solver; in the non-linear case, we can use generalized Newton-Raphson method to solve the non-linear system (see Kelly [23], and Hageman and Young [24]).

3. Derivation of the Cubic Spline Method

For the derivation of the cubic spline method (12) for the solution of the quasi-linear parabolic Equation (1), we simply follow the approaches given by Jain and Aziz [7].

At the grid point, let us denote

(13)

Further at the grid point, we may write the differential Equation (1) as

(14a)

Similarly,

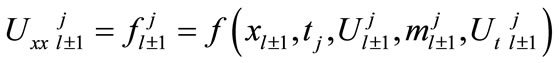(14b)

A difference method of accuracy of for the differential Equation (1) in the absence of first derivative terms may be written as

(15)

By the help of the notations (13) and simplifying (5)-(8b), we obtain

(16a)

(16b)

(17a)

(17b)

(18a)

(18b)

(19)

With the help of the approximations (5), (16a)-(18b), from (9a) and (9b), we obtain

(20a)

(20b)

Using the approximations (18a), (18b), (20a), (20b) and simplifying (10a), we get

(21)

From (21), it is easy to verify that for, we have

(22a)

Similarly, from (10b) and (10c), we have

(22b)

Finally, by the help of the approximations (5), (16a)-(17b), (22a)-(22b), from (11a) and (11b), we get

(23a)

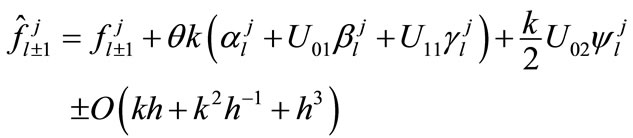(23b)

Now differentiating the differential Equation (1) with respect to ‘t’ at the grid point, we obtain a relation of the form

(24)

Using the approximations (19), (23a), (23b) and by the help of the relation (24), from (12) and (15), we obtain the local truncation error

(25)

The proposed cubic spline method (12) to be of, the coefficient of in (25) must be zero. Thus we obtain the value of, for which.

4. Cubic Spline Scheme for Parabolic Equation with Singular Coefficients

Consider the linear parabolic equation of the form

(26)

subject to appropriate initial and Dirichlet boundary conditions are prescribed by (2) and (3), respectively. Assume that the functions, and .

For the equation above represents linear parabolic equation with singular coefficients. For the above parabolic equation becomes a cylindrical and spherical problem for and 2, respectively.

Applying the formula (12) to the differential Equation (26), we get

(27)

where

,

etc.

At the grid point, let us denote

(28)

Note that, the cubic spline sheme (27) is of accuracy for the approximate solution of Equation (26). However, the scheme (27) fails to compute at l = 1 when the coefficients etc. In order to get a meaningful cubic spline scheme of accuracy in compact operator form, we need the following approximations:

(29a)

(29b)

(29c)

where

Now, by the help of the approximations (5)-(10c) and (29a)-(29c), neglecting high order terms, we can re-write the scheme (27) as a two-level implicit cubic spline scheme in operator compact form

(30)

where

and

and

are averaging and central difference operators with respect to x-direction etc. The cubic spline scheme (30) has a local truncation error of and is free from the terms, and hence, it can be solved for l = 1(1)N in the region 0 < x < 1, t > 0.

Now consider the linear singular parabolic equation

(31)

For and 2, the equation above represents the parabolic equation in cylindrical and spherical coordinates respectively. Replacing the variable x by r and substituting etc. in Equation (30), we can get a two level implicit cubic spline scheme of for the solution of linear singular parabolic Equation (31).

Now, consider the linear diffusion-convection parabolic equation

(32)

The constant terms and are called the diffusivity and convective terms, respectively. For, the problem is said to be convection dominated problem. Substituting in the formula (30), we obtain a two-level implicit cubic spline scheme

(33)

where represents the cell-Reynolds number. The scheme (33) is of and consistent with the differential Equation (32). Further note that, the scheme (33) is identical with the scheme discussed by Jain et al [20]. It has been shown that, the scheme (33) is unconditionally stable and using this scheme computational result for the solution of Equation (32) is reported in [20].

5. Numerical Results

In order to demonstrate the application of the proposed cubic spline method, we have solved the following four problems whose analytical solutions are known to us. The right-hand side homogeneous functions, initial and boundary conditions can be obtained using the exact solution as a test procedure. We have also compared the proposed cubic spline method with the corresponding finite difference method of discussed in [20-22]. We have solved the system of linear difference equations using a tri-diagonal solver and the system of non-linear difference equations by Newton-Raphson method (see Kelly [23], and Hageman and Young [24]). All computations were performed using double length arithmetic.

Example 1: (Linear parabolic equation with singular coefficients)

(34)

The exact solution is given by u(x,t) = exp(-εt) sinh x. The root mean square (RMS) errors at t = 1.0 are tabulated in Table 1 for and small values of ε > 0.

Example 2: The Equation (31) is solved whose exact solution is u(r,t)=exp(-t) cosh r. The RMS errors at t = 1.0 are tabulated in Table 2 for α = 1 and 2 for a fixed value of.

Example 3: (Burgers’ Equation )

(35)

The exact solution is given by

, where

is termed as a Reynolds number. The RMS errors at t = 1.0 are tabulated in Table 3 for different values of R_e and for a fixed value of.

Example 4: (Non-linear Parabolic Equation )

(36)

The exact solution is given by u(x,t)=exp(-t) sin(). The RMS errors at t = 1.0 are tabulated in Table 4 for various values of α for a fixed parameter value of.

6. Final Remarks

We presented a new cubic spline discretization strategy of for the solution of one-space dimensional quasi-linear parabolic partial differential equations. The proposed method with a little modification is applicable to singular parabolic problems. For singular problems, our numerical results show that the new cubic spline method may be advantageous compared with the corresponding finite difference method discussed in [20-22]. Numerical results for non-linear problems also show better as compared to the method discussed in [20-22], and numerical oscillation do not appear for high Reynolds number.

7. Acknowledgement

The authors thank the reviewers for their valuable suggestions, which substantially improved the standard of the paper.

8. References