Model for Frequency Dependence of Thermal Permeability in Order to Quantify the Effects of Thermal Exchange on Wave Propagation in Multi Layered Porous Medium ()
1. Introduction
Teagle et al. [1] derived a coupled set of fluid/structure equations for a porous medium applying asymptotic and homogenization techniques. Via this modeling it is established in [1] that there are mainly 3 modes of energy transformation: 1) The first mode is through the connection between the micro and macro structural framework of the porous skeleton, 2) the second is via the viscous boundary layer, and 3) the third interaction is through thermal (entropy) boundary layer. The combination of the viscous boundary layer and how tortuous the porous material is, results in the encapsulation of the fluid medium. This, in turn, changes the apparent mass of the structural medium. Details pertaining to these encapsulating phenomena can be found in the work by Johnson, et al. [2]. In [2] the concepts of tortuosity, viscous length, and viscous permeability are explained.
This current work concentrates in the third interaction mode. There is a boundary layer where the flow experiences changes in entropy, therefore a loss/gain of energy due to thermal exchange will occur. This thermal exchange also changes the acoustic bulk modulus at certain frequencies and in turn changing the speed of sound. This is depicted through Equation (31) found in [1]
(1)
From this equation it is understood that the compressibility factor,
, is defined as
(2)
where
is the bulk modulus of the air inside the pore.
is the
thermal permeability and is defined as
(3)
are the first terms of the asymptotic expansion (macro scale) pertaining to the temperature and pressure respectively, their equations are derived in [1]. The bulk of this work is to derive a frequency dependent general expression for the thermal permeability,
. In order to attain this goal a good understanding of the physics behind the thermal exchange process has to be accomplished. This is achieved by: 1) analyzing the intensity escaping the main acoustic mode or 2) deriving a partial differential equation incorporating the porous wall impedance due to the thermal/viscous boundary layer; both are accomplished here.
This work also models an additional energy absorbing phenomena and that is the relaxation process. This is the hysteresis effect due to compression/decompress- sion cycle of the molecules. The application of quantum mechanics, explained via the construct of Hilbert Spaces along with its dual space, facilitates the modeling of this hysteresis effect.
The main interest of the current authors is to calculate transmission loss (TL), Insertion loss (IL), and absorption coefficient (Alpha) for multi-layered porous medium. Therefore, calculation results for TL pertaining to a layered material, consisting of 2 different foams, are shown. This calculation is achieved by incorporating all of the aforementioned energy phenomena into the Finite-Cor- rection Transfer Matrix Method (FTMM) [3] [4] [5].
In addition to the goals mentioned in the last paragraph a parallel effort is underway to lay down the mathematical foundation to understand how the propagating energy transfigures as it travels through the porous medium. This creates a tool that will allow design teams to make efficient absorption comparison of porous mediums consisting of different rheological substances.
2. Formulation
2.1. Basic Statistical Mechanics: Partition Function/Boltzmann Distribution/Quantum States
One of the goals is to obtain a comprehensive set of fluid equations that incorporates any phenomena that leads to energy depletion. This means that in addition to viscous and thermal effects that transpire within their respective boundary layers, a quantification of the hysteresis effects due to the delay in thermal response should be obtained and incorporated. The most efficient tool to describe this process and for that matter any process is to apply statistical mechanic techniques. Once the Partition Function, Z, is obtained any macro level characteristic pertaining to the system can be derived. In this study Boltzmann’s
version of the combinatorial arrangement,
, is used. N represents
the number of states, and ni are the number of states at each energy level. Applying Sterling’s formula, this arrangement number becomes,
, where
, is the probability of being in the ith
energy state that has energy
. The statistical mechanic definition of Entropy, s, is the measure of information plus its distribution and is defined as finding the pis that will maximize the arrangement number, C, under the following constraints:
, and
(E = Average Energy). From this mathematical framework, the temperature,
, plays the role of a Lagrange Multiplier. The main results are that
(4)
where Z is the partition function defined as
(5)
and note that the units for
(“theorist” temperature) is in Joules. It is easily shown that the average energy and the Helmholtz Free Energy, A, are represented respectively as,
and
(6)
In quantum mechanics (see Section 2.2 below) the probability density is
defined as
, where
is a state vector of the system. Applying Schrodinger’s equation,
, and Hamilton’s operator,
defined in Equation (19a), the following differential equation is derived which expresses the propagation of probability
is the probability flux. For adiabatic processes the divergence of the probability flux is shown to be zero,
; therefore the probability density is stationary with respect to time. This means that the pis are adiabatic invariant (which in turn means that entropy is constant) and thus the following expression for pressure holds
(7)
The average potential between the particles is defined as
and under classical mechanic conditions the partition function is
(8)
is the partition function pertaining to the Ideas Gas.
In order to obtain a general expression for the average energy of the gas, Equation (6) is applied
(9)
Diatomic molecules like O2 and N2 can be modeled as stiff oscillators. As a simple representative example, a model of a simple oscillator with stiffness k will be studied. The total energy for this system is
(10)
Via the Boltzmann distribution, the Partition Function pertaining to the oscillator,
, is
(11)
By applying Gaussian integrals the Partition Function for an undamped oscillator is
(12)
As before, Equation (6) is used in order to obtain the average energy of the oscillating system
(13)
The analysis above, especially Equation (11), assumes classical mechanic conditions. Equation (13) states that the average energy of the oscillating system is
which, based on prior analysis, is connected to kinetic energy. This result is somewhat suspect since it states that regardless of how stiff the system is, the average energy is always
. In classical mechanics, the path integrals measured with respect to the Max Planck constant,
, are relatively large. An observable in classical mechanics is simply a real function in Euclidean space. In quantum mechanics every possible state of a given system corresponds to a separable Hilbert Space over the complex number field. Additionally, to every physical observable there corresponds, in the Hilbert space, a linear Hermitian operator that has a complete set of orthogonal eigenvectors. The following limit applies to systems oscillating at relatively high energy levels,
thus, in classical mechanics, it can
be assumed that the energy forms a continuous spectrum; as compared to quantum mechanics where there is now a quantization (discretization) of the energy states [6]. In quantum mechanics a unit of energy is proportional to the natural frequency of the system by the constant,
. The nth energy state is defined as
(14)
Thus, the Partition Function is
Equation (6) is utilized once more in order to obtain the average energy of the stiff oscillator
(15)
At high temperatures
and therefore Equation (15) becomes
(16)
Therefore at high temperature levels there is enough energy such that the quantization of energy is not required. The criteria to follow are the following
(17)
The cross-over point is
. Lastly, it is possible to extract the Energy’s standard deviation from the Partition Function. It is easy to show that
. What follows is the following definition
(18a)
Recognize that
, the specific heat constant at constant volume and
or
fluctuation-susceptibility Theorem (18b)
The specific heat constant at constant volume is proportional to standard deviation of the energy.
2.2. Relaxation Process: Fluid Hysteresis/Bra-Ket Vectors, Schrodinger’s Equation
The vibration due to the collision of molecules extract energy from the passing wave but releases the energy after some delay, thus the relaxation process. This delay, akin to mechanical damping systems leads to hysteretic energy losses. The internal energy can be partitioned into translated (defined relative to the average flow velocity), rotational kinetic energy and energy due to molecular natural frequency,
quantum energy level/mass
The temperatures, Ttr and Trot, related to translational and rotational energies are larger than the temperatures related to molecular oscillation. They usually satisfy the second criteria in Equation (17) and therefore can be considered using Classical Theory. For the molecular vibration case,
, and, based on Equation (17), quantum mechanics should be applied.
The first postulate in quantum theory states that everything that can be known about the state of a system can be extracted from its state vector (wave function) and this is represented as a vector in Hilbert Space; these are denoted as ket-vectors
. The bra-vectors are denoted as
, the vector space of bras is the dual space(one-to-one correspondence with the space of functionals) to the kets vector space. The Riesz representation theorem allows the following notation
.
The 1-D Hamilton operator is given as
(19)
where
is the Linear Momentum operator,
, and
is the position
operator.
and
are both observables and thus are hermitian operators on the Hilbert Space, therefore
has a purely discrete spectrum of eigenvalues,
, that has a complete set of eigenstates,
, i.e. (
). Hence, any state or ket-vector,
, can be expanded by the set of eigenstates,
. Due to Schrodinger’s equation the eigenstates pertaining to the energy operator are stationary with respect to time. For a diatomic molecule the oscillating like characteristics between the 2 atoms can be simply represented by a spring constant K. Given a particular natural frequency, fe, K can be represented as
. The potential energy pertaining to the simple oscillator is represented as
Therefore the potential operator becomes
. Hamilton’s operator can be pseudo separated as follows
(20a)
(20b)
where
. It can be shown that the commutator
and therefore
(20c)
Based on Equation (20c) the eigenvalue equation can be written as
(21)
Built on the ideas in Equation (21) the concept of annihilators,
, and creators,
, are established; the following relationships exists
corresponding eigenvalue
} annihilator
corresponding eigenvalue
} creator
Another possible solution, tied to the annihilator equation, is that
. Here,
is known as the zero-point wave-function and
is the zero-point energy level. Equation (20a) pertaining to the zero-point state results in
,
normalized solution
The eigenstates pertaining to the higher energy levels can be generated by applying the Riesz representation notation.
Thus, if
is normalized so is
As a consequence, the recurring formula for the eigenfunctions/states becomes
(22)
where
are the Hermite polynomials. Additionally due to Equation
(19a) the pertaining eigenvalues of
are
An alternative approach is to apply Schrodinger’s Equation
.
Plugging in the definition for the Hamilton Operator defined in Equation (19a) results in the following simple oscillator equation
(23)
Setting
,
, and
(24)
Schrodinger’s Equation simplifies to
(25)
When
the solutions to this equation are the Hermite
Polynomials. Note that the solution is equivalent to Equation (22). Applying this definition and solving for
, the energy levels are defined as
(26)
Due to the assumption of independence of the different energies the partition function pertaining to internal energies can be represented as
(27)
The partition function for the vibrational portion pertaining to a particular type of molecule v (e.g. O2, N2) is represented as
(28)
is the ground vibrational state energy (obtained by setting j = 0 in Equation (26)). Using Equation (26) in (28), the vibrational partition function can be rewritten as
(29)
Such that
.
Applying Equation (6), an expression for the average energy is obtained
(30)
Therefore the vibrational internal energy becomes
(31)
Differentiating this equation with respect to temperature one obtains [7] [8]
(32)
Applying the Boltzmann distribution definition for entropy
results in
(33)
Recall that
and the temperatures pertaining to Translational and rotational energy levels are high enough to be considered by classical
mechanics. The combination of kinetic energies
and positions are
considered as possible states, the application of the Boltzmann distribution results in the following equation for average energies.
such that
(34)
The instantaneous change of entropy can be represented in the following form
(35)
The
terms represent the small deviation from equilibrium. The affinities
are to be determined. The portion devoid of vibration energy is denoted from now on with subscript fr (frozen), and the portion pertaining to vibrational energy is indicated with subscript v [9].
(36)
(37)
Set
assuming ideal gas
(38)
,
= coefficient of specific heat at constant volume. (39)
Substituting Equation (38) into (37) and solve for
the following expression is obtained
(40)
Utilizing Equation (37) - (40) and applying them into Equation (35) yields
(41)
The expression in Equation (32) can be substituted into Equation (41). Since
,
can deduce to
(42)
act as the specific heat pertaining to each molecular type v.
The inclusion of these energy terms into the standard energy equations results in
(43)
Applying the conservation of mass and the heat flux the entropy balance equations become
(44)
The Navier Stokes Equation with the inclusion of the bulk viscosity becomes
(45)
Applying conservation of energy results in the following expression for the vibration energy
(46)
represents the number of collisions a molecule of type
has per unit time,
is the number of molecules of type
, and
is the average energy gained per collision. It is known that most of the high temperature resides in the translational and rotational portions, therefore
can best be expressed as
(47)
and yields
such that
(48)
More elaborate modeling for
can be achieved by applying Discrete Boltzmann Equations but these are outside the scope of this project [10]. Solving the differential equation for
results in
(49)
If T is changed by an amount
it will take a time of
for the incremental change in
is
. Therefore
is called the relaxation time. And it is this relaxation time that introduces the hysteretic energy losses [11].
The energy conservation dissipation equation is in the form of
where
(50a)
(50b)
(50c)
From the coupled system of acoustic equation, asymptotic techniques result in different dispersion relationships for 3 different regimes; for the viscous and entropy boundary layers, and the equations pertaining to the acoustic mode. These translate to different set of partial differential equations pertaining to the 3 different regimes. The vibrational relaxation terms will mostly affect the acoustic mode regime. In order to obtain the new dispersion relationship a solution in the form of
is plugged into the equation of motion (see Appendix A.1 - A.5), the ensuing is obtained
(51a)
(51b)
(51c)
Plugging this last equation into Equation (51a) the dispersion relation is obtained [12]
(51d)
For the acoustic mode and the frequency range of interest the following
approximations exist:
the last 2
expressions can be found in Kirchhoff’s dispersion relation and were considered small in [14]. Given these assumptions, the following asymptotic expansion is obtained
(51e)
The absorption coefficients are the imaginary portion of Equation (51e)
(51f)
where
(51g)
Recall that
. At relatively low frequencies,
Equation (51g) indicates that
quadratic increase w.r.t.
(51h)
and at relatively high frequencies,
, results in the following
approaches a constant value (51i)
2.3. Energy Loss: Thermal Boundary Layer-Bessel Functions
In [1], multi-scales technique is used on the coupled fluid/structure set of equations. Equation (16), in that publication, represents the partial differential equation that connects fluctuations of temperature and pressure in the fluid medium. The dimensional form of this equation is
(52)
Before deriving the thermal exchange for a general porous medium (with pores of arbitrary geometry), the thermal exchange for a typical cylindrical pore with cross-sectional radius R will be analyzed. The form this solution takes will be used as a pattern to emulate when considering solutions for the general case. Equation (52) in cylindrical form is
(53)
The Bessel function generator is defined as
(54a)
The
Bessel function is efficiently represented via Hankel’s integral
(54b)
It can be deduced from Equation (54a) that
(55a)
The ensuing 2 equations are obtained by combining the equations in (55a)
(55b)
Applying Equation (55b) results in
(55c)
Equation (55c) is integrated from 0 to R, this generates an equation that will be utilized shortly
(55d)
Teagle [1] showed that
is independent of the micro (fast) scale y. Therefore
the particular solution for Equation (53) is
. The homogeneous solution is
, where
is a derived so that boundary conditions
at the wall of the cylinder pores are met,
. Combining the particular and homogenous solutions and satisfying boundary conditions, the following expression for temperature is obtained
(56)
For statistical energy analysis calculations, average temperature of the pore cross-section suffices, therefore
(57)
For the more general case, the focus is on analyzing the energy flux escaping the acoustic mode into the entropy boundary layer. Johnson et al. [2] accomplished similar work for the viscous boundary layer.
Trilling [13] gave a detailed explanation of the separation technique utilized to obtain 3 dispersion relations from the Navier-Stokes Equation. Applying asymptotic expansion for each of these relationships, it is possible to derive 3 sets of simple partial differential equations along with their pertinent conditions. The process polarized the linear Navier-Stokes equation into 3 different flow regimes. The superposition principle can be applied to obtain the total velocity. The flow regimes or modes can be characterized by the following:
Vorticity Mode field
,
,
(58)
It is important to point out that Equation (58) is a classical diffusion equation
The entropy Mode field
(59a)
(59b)
Acoustic Mode field
(60)
Due to the superposition principle the microscopic velocity flow field can be written as
(61)
The dispersion for the vorticity and entropy modes are
(62)
Therefore the vorticity and entropy mode field extend to
and
so
(63)
Near the solid surface it can be assumed that the solution has the form
. (64)
where this relation satisfies the following equation
. Additionally, as in the aforementioned
cylindrical case, the temperature fluctuation at the wall is zero. The temperature conditions in Equations (58) (29b) (60) produces the following
(65)
Applying Equation (64) into Equations (58), (59a), (59b) and splitting the “del” operator into tangential and normal components (where the tangential portion will have a subscript T) yields the following
(66)
(67)
(68)
At z = 0 plane Equation (61) will give the following boundary condition
(69)
and applying the
(horizontal divergence) along with Equation (67), Equation (69) can be rewritten the following way
(70)
Remember that
= isothermal/adiabatic bulk modul (71)
Additionally, using Equation (68) and (70) the subsequent conditions are obtained in the direction normal to the surface
(72)
Recall that the main goal is to obtain an expression for the energy that is being dissipated and this is dictated by the net power flowing out of the acoustic (ac) mode close to the boundary. Multiplying Equation (72) by the complex conjugate of the acoustic mode pressure,
, and solving for
, which is the intensity leaving the acoustic mode, provides the following equation
(73)
Recall that the relative velocity at the wall is equal to zero. From the first relation in Equation (60) it can be deduced that
(74)
The second term in the right hand side of Equation (73) will average out to zero given enough surface area. Equation (74) will be applied in the third term of Equation (73) to finally obtain the following relation
(75)
For the mechanical dissipation the first term in the right hand side is used by [2]. The second term will be utilized in this study to treat the thermal dissipation and derive the acoustic bulk modulus.
The thermal counterpart of dynamic permeability and tortuosity are
(76)
(77)
where
and
satisfy the following
 (78)
(78)
The real constant 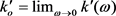 acts like a thermal form of a static thermal permeability, i.e. the thermal counterpart to Darcy’s static equation of
acts like a thermal form of a static thermal permeability, i.e. the thermal counterpart to Darcy’s static equation of .
.
A volume of porous sample enclosed by a thin membrane is considered. In this particular case, it is assumed that the thin membrane acts like an adiabatic wall. The interest is to know where the singularities to 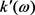 and
and  lie. The existence of a singularity means that there exists a nontrivial solution to the above microscopic equation while the macroscopic input is zero, i.e.
lie. The existence of a singularity means that there exists a nontrivial solution to the above microscopic equation while the macroscopic input is zero, i.e. . This is equivalent to saying that there is no mechanical power entering the element. Therefore Equation (78) becomes
. This is equivalent to saying that there is no mechanical power entering the element. Therefore Equation (78) becomes
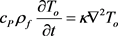 (79)
(79)
To guarantee that no thermal power is entering the element, the following has to hold
 (80)
(80)
Since the membrane is adiabatic and since conditions listed in Equation (78) are being satisfied the integral in Equation (80) is consequentially fulfilled. Pre multiplying the Fourier equation of heat diffusion equation, Equation (79), by the complex conjugate , assuming a solution of the form
, assuming a solution of the form , and then perform a volume integral in terms of the microscopic variable yields
, and then perform a volume integral in terms of the microscopic variable yields
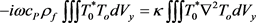 (81)
(81)
Making use of the usual identification
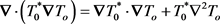 (82)
(82)
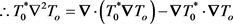 (83)
(83)
So
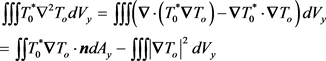 (84)
(84)
The first term in the right hand side of Equation (84) goes to zero because of boundary condition found in Equation (80). Inserting this result in Equation (81) and solving for 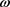 the following is obtained
the following is obtained
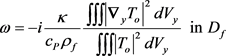 (85)
(85)
Due to the shifting rule, this indicates that the singularity for  lies in the negative imaginary axis. In the meantime consider Equation (77) where the boundary condition is that of
lies in the negative imaginary axis. In the meantime consider Equation (77) where the boundary condition is that of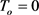 . Additionally, no mechanical power is entering through the adiabatic wall. The aim is to find where the singularities of
. Additionally, no mechanical power is entering through the adiabatic wall. The aim is to find where the singularities of ![]() lie. Equation (78) is multiplied by
lie. Equation (78) is multiplied by![]() . A volume integral results in
. A volume integral results in
![]() (86)
(86)
In [1] it is shown that ![]() is independent of the micro scale variable and therefore it is considered as an external source of power for this thermal element. Applying Equation (82), (83), and the Green’s theorem to the first term in Equation (86), the same result of Equation (85) is obtained; again, the singularities of
is independent of the micro scale variable and therefore it is considered as an external source of power for this thermal element. Applying Equation (82), (83), and the Green’s theorem to the first term in Equation (86), the same result of Equation (85) is obtained; again, the singularities of ![]() also lie in the negative imaginary axis.
also lie in the negative imaginary axis.
Following the same steps performed in [2] in deriving the relation for viscous length, the last term in the right hand side of Equation (73)
![]() is studied. It is understood that this term repre-
is studied. It is understood that this term repre-
sents the thermal portion of the energy flux exiting the main acoustic mode. The third term in the RHS of Equation (50a) is the thermal energy. Multiplying this term by the speed of sound, c, the energy flux entering the pore is attained. The ensuing equation represents the ratio of thermal energy that is leaving the acoustic mode to that of thermal energy that is entering the thermal element.
![]() (87)
(87)
Recall temperature condition ![]() and from Equation (59b),
and from Equation (59b),
![]() . Additionally from Equation (60),
. Additionally from Equation (60),![]() . Lastly,
. Lastly,
applying Equation (71), Equation (87) simplifies to
![]() (88)
(88)
In Equation (88),![]() . The integration takes place at the micro level and it has been established that
. The integration takes place at the micro level and it has been established that ![]() is independent of the micro scale variable, y, and thus the first equality sign in Equation (88).
is independent of the micro scale variable, y, and thus the first equality sign in Equation (88). ![]() is the Thermal Length and is
is the Thermal Length and is
defined as![]() . Using Equation (57) as a pattern solution, the general solution
. Using Equation (57) as a pattern solution, the general solution
for average temperature is defined as (assuming ideal gas conditions)
![]() (89)
(89)
In Equation (89), as increases the thinner the entropy boundary layer, ![]() , is and therefore the limit as
, is and therefore the limit as ![]() the acoustic mode condition (ideal gas)
the acoustic mode condition (ideal gas)
![]() applies for the whole cross-section. This equation for
applies for the whole cross-section. This equation for ![]() is
is
plugged into Equation (3). The following expression for thermal permeability
is obtained
![]() (90)
(90)
The second term in Equation (90) is obtained by applying the definition of![]() . This term can asymptotically (as
. This term can asymptotically (as![]() )be represented as
)be represented as
![]() (91)
(91)
where, ![]() is the thermal permeability as
is the thermal permeability as![]() . A combined asymptotic representation that interpolates thermal permeability for the whole frequency range is represented as
. A combined asymptotic representation that interpolates thermal permeability for the whole frequency range is represented as
![]() (92)
(92)
Finally, this is the term that is used in Equation (2) to obtain the compressibility factor of the fluid of the porous medium.
3. Conclusions and Closing Remarks: Absorption
Consider the acoustic medium near the wall of a tube (the x axis is taken to be along the tube). Set ![]() as the angle of incident and recall that the solution to the wave equation tangent to the tube wall will have the form of
as the angle of incident and recall that the solution to the wave equation tangent to the tube wall will have the form of
![]() therefore
therefore![]() . Also recognize that
. Also recognize that
![]() . With these definitions, the
. With these definitions, the ![]() term in Equation (73) can be represented as
term in Equation (73) can be represented as
![]() (93)
(93)
An expression for the wall impedance can be given as
![]() (94)
(94)
The objective is to obtain a simple partial differential equation that incorporates the impedance effects of the viscous and thermal boundary layer. Following techniques introduced by Crandall et al. [15], The variational form of the Helmholtz equation is
![]() (95)
(95)
The ![]() is a segment of the perimeter of the tube and
is a segment of the perimeter of the tube and ![]()
Applying the definition of impedance, the following is obtained
![]() (96)
(96)
and applying this to the variational Helmholtz Equation yields
![]() (97)
(97)
As a first order approximation, it is assumed that the parameters do not vary with respect to the cross-sectional area
![]()
![]() (a) (b)
(a) (b)
Figure 1. Left: TL comparison (dB); Right: TL difference(dB) measured data is the reference.
![]()
![]() perimeter length (98)
perimeter length (98)
Performing the variation generates
![]() (99)
(99)
For the case pertaining to constant cross-sectional area, the dispersion relationship is
![]() (100)
(100)
![]() is the absorption
is the absorption
coefficient of the wall. It is interesting to note the similarity in form between the imaginary part of Equations (100) and (88).
Equation (51g) pertaining to the absorption due to the relaxation process, ![]() , combined with the effect of the thermal permeability,
, combined with the effect of the thermal permeability, ![]() , on the bulk modulus,
, on the bulk modulus, ![]() , of the porous medium are incorporated into Transmission Matrix Method with finite size correction via Green’s functions techniques (FTMM) [16]. The parameters to this study are listed in Table 1 in [1]. Figure 1 shows the comparison between experimental data and the FTMM calculations for the multi-layer configuration shown in Figure 2. Further analysis, especially a parameter study on the different relaxation and thermal components is currently being done. This study mainly assumes air within the porous medium but there is current interest in applying these to pores with different rheological make up.
, of the porous medium are incorporated into Transmission Matrix Method with finite size correction via Green’s functions techniques (FTMM) [16]. The parameters to this study are listed in Table 1 in [1]. Figure 1 shows the comparison between experimental data and the FTMM calculations for the multi-layer configuration shown in Figure 2. Further analysis, especially a parameter study on the different relaxation and thermal components is currently being done. This study mainly assumes air within the porous medium but there is current interest in applying these to pores with different rheological make up.
Appendix A: Linear Acoustic Equation
![]() (A.1)
(A.1)
![]() (A.2)
(A.2)
![]() (A3)
(A3)
![]() (A4)
(A4)
![]() (A.5)
(A.5)
![]()
![]()
![]()
![]()
Nomenclature
![]() = Fluid Pressure, Macro Scale
= Fluid Pressure, Macro Scale
![]() = Porosity
= Porosity
![]() = Viscosity
= Viscosity
![]() = Domain Occupied by Fluid
= Domain Occupied by Fluid
![]() Cp = specific heat at constant pressure Cv = Specific heat at constant volume
Cp = specific heat at constant pressure Cv = Specific heat at constant volume
![]() = Coefficient of thermal Conductivity
= Coefficient of thermal Conductivity
![]() = Prandlt Number =
= Prandlt Number = ![]()
![]() ,
,![]() = density of the Fluid
= density of the Fluid
T = Temperature deviation
![]() = Vibrational temperature pertaining to molecular type v
= Vibrational temperature pertaining to molecular type v
![]() = theorist temperature, kT, k = Boltzmann constant
= theorist temperature, kT, k = Boltzmann constant
![]() = Dynamic Viscous Permeability
= Dynamic Viscous Permeability
![]() = Dynamic Thermal Permeability
= Dynamic Thermal Permeability
![]() = asterisk superscript means conjugate
= asterisk superscript means conjugate
![]() = special tilde
= special tilde ![]() 2nd rank tensor
2nd rank tensor
![]() = Entropy,
= Entropy, ![]() = Entropy without molecular vibration,
= Entropy without molecular vibration,
![]() = molecular vibration entropy
= molecular vibration entropy
![]() = Thermal expansion coefficient =
= Thermal expansion coefficient = ![]()