Old Mechanics, Gravity, Electromagnetics and Relativity in One Theory: Part I ()
1. Introduction
Numerous of recent books in physics discourse the summarizing of Maxwell’s four equations into two equations, without addressing the possibility to generalize this concept to the rest of the forces, and work to link them with classical physics, which is the goal of publishing this research.
Whereas we cannot link all the physical sciences in one theory, unless the base upon which this theory was built represents a good and common ground for all of these sciences, So at the beginning will get to know some mathematical concepts (for example the relationship between the matrix and the vector) in a new and concise manner, with the remarks that we will deliberately ignore some proofs and details of those concepts to shorten the pages of this research that will exceed tens of pages.
In this part of the theory we will prove that all equations of force in nature belong basically to two basic mathematical formulas, on the Figure 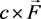 and
and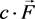 , including Lagrange equation, which will we address in the coming research, and the results obtained in this research will be applied only to the electrical and magnetic forces, with no other ones, since these forces are the most prominent in the books of physics.
, including Lagrange equation, which will we address in the coming research, and the results obtained in this research will be applied only to the electrical and magnetic forces, with no other ones, since these forces are the most prominent in the books of physics.
In the next parts we will explore the possibility of combining the theories of General Relativity and Quantum Mechanics with this theory in the minimal mathematical relationships as possible.
However, the purpose of publishing this paper can be summarized as follows
1) Introducing new mathematical ideas and concepts which will help to unify physics;
2) Unification of the physical sciences with as few equations as possible (in this part, most of the forces known as only two forms);
3) Linking modern physical science with ancient physics without resorting to any hypotheses (such as the stability of the speed of light in the theory of relativity).
2. Basic Notions
2.1. A.E Filed
In this paper, the space  is called A.E space in n-dimensional with m-index filed, in this case. We suppose that the m-index filed vector
is called A.E space in n-dimensional with m-index filed, in this case. We suppose that the m-index filed vector  in
in 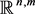 is defined by
is defined by

where
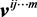 is a complex orthogonal unit, that is defined by setting
is a complex orthogonal unit, that is defined by setting
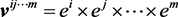
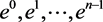 are unit vectors in the
are unit vectors in the 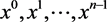 directions.
directions.
In general, the space of two vectors 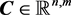 and
and  is defined as
is defined as 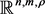 in n-dimensional with m, ρ mix-filed.
in n-dimensional with m, ρ mix-filed.
2.2. Theory
“The cross product of a set of vectors in any specified space equal to the Determinant of these vectors”
It mean that if
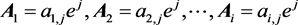 with
with 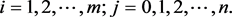 And,
And,
 , then
, then
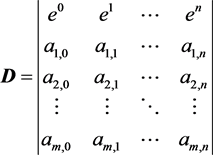
2.3. Determinants and Dual Determinants
2.3.1. The Main Determinant
The main determinant of (m + 1) × (n) matrix has been defined. Denote by 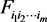 the sub determinant of the (m) × (m) matrix obtained from D by deleting the first row and choosing the
the sub determinant of the (m) × (m) matrix obtained from D by deleting the first row and choosing the ![]() -columns. Then, the main determinant of matrix denote as
-columns. Then, the main determinant of matrix denote as
![]()
where
m: the number of the vectors in the group;
![]() ―the numbers of columns which has been chosen.
―the numbers of columns which has been chosen.
![]()
2.3.2. The Dual Determinant of Matrix Denote as
![]()
and the dual sub determinant ![]() define by equation,
define by equation,
![]() (2.1)
(2.1)
where
![]() ―the numbers of columns which has not been chosen.
―the numbers of columns which has not been chosen.
![]() or
or ![]() denotes the cyclic permutation symmetry.
denotes the cyclic permutation symmetry.
Let,![]() :
:![]() , and
, and![]() , Then
, Then
![]()
![]()
![]()
Thus,
![]()
where, ![]()
► For example:
let,![]() :
: ![]()
Then, from above equation we get
![]()
The magnitude or length of the vector ![]() in
in ![]() is defined as
is defined as
![]()
From dot product, we get
![]() :
:![]() . Thus
. Thus
![]()
![]()
The last equation equals the length of the vector in ![]()
2.3.3. Calculate the Dual to ![]()
From (2.1), we can define the dual vector ![]() by equation
by equation
![]()
3. Vector Properties in ![]()
3.1. Conversion to Matrix Form Property
3.1.1. Let ![]() Be Any Vector in
Be Any Vector in![]() , It Can Be Written as the Main Matrix
, It Can Be Written as the Main Matrix ![]() in the Form
in the Form
![]() , where
, where
![]()
3.1.2. The Dual Vector![]() , It Can Be Written as the Dual Matrix
, It Can Be Written as the Dual Matrix ![]() in the Form
in the Form
![]() , then
, then
![]()
3.2. The Mix Product Property
3.2.1. The Mix Cross-Product of Two Vectors ![]() and
and ![]() Is Defined by Setting
Is Defined by Setting
![]() (3.1)
(3.1)
![]()
3.2.2. The Mix Dot-Product of Two Vectors ![]() and
and ![]() Is Defined by Setting
Is Defined by Setting
![]() (3.2)
(3.2)
where, ![]()
From (2.1), we therefore get
![]() (3.3)
(3.3)
![]()
► Now in the example at hand, we have
![]()
![]()
Let, ![]()
Then, the cross-product of two vectors ![]() and
and ![]() is defined as
is defined as
![]() (I)
(I)
On the other hand, we have from vector relations the equation
![]()
Here the last vector equals the Equation (1).
The dot-product of ![]() and
and ![]() is defined as
is defined as
![]() (II)
(II)
From (3.2), we therefore get
![]()
Here the new vector appearing on the right-hand side equals the Equation (II).
4. The Force Equations on A.E Filed
On A.E filed, there are only two types of forces namely cross and dot forces
4.1. Calculate the Cross Force Fcross
Let ![]() be 4-force in the form
be 4-force in the form
![]()
Therefore
![]() as
as
![]()
Then
![]() (4.1)
(4.1)
According to the three-orthogonal vectors e1, e2, e3 we can rewrite the field vectors as
![]() (4.2)
(4.2)
Now consider the equations
![]() (4.3)
(4.3)
Thus, the Equation (4.1) becomes
![]()
we therefore get
![]() (4.4)
(4.4)
4.2. Calculate the Dot Force Fdot
Let ![]() be 4-Force in the form
be 4-Force in the form
![]()
From Equation (3.2) we have
![]()
where
![]()
Using the three-orthogonal vectors e1, e2, e3 we can rewrite the transformation in Equation (4.2) and Equation (4.3) as
![]()
then we obtain
![]()
Thus
![]()
For the orthogonal unit vectors e1, e2, e3 the last equation becomes
![]() (4.5)
(4.5)
where
![]()
► Let in our example
![]() :
: ![]()
Then
![]() ,
, ![]()
![]()
![]()
thus from Equation (4.4) and Equation (4.5) we get
![]()
![]()
The last two equations equals the Equation (I) and the Equation (II).
5. The Relationships between the Force Equations on A.E Filed and the Conventional Force
5.1. Calculate the Conventional Ordinary Force
The 4-momentum P of a particle of mass m0 at position ![]() moving at
moving at
velocity ![]() can be written as
can be written as
![]()
The 3-velocity v of the particle is defined by![]() , then
, then
![]()
where ![]() is called an angular velocity vector of the rotating system,
is called an angular velocity vector of the rotating system,
![]()
թ is the 4-momentum of the coordinate system itself, թ = (թ0, թ)
Thus
![]()
![]()
Now, we can rewrite last equation as the following
![]() (5.1)
(5.1)
where
![]()
![]() (5.2)
(5.2)
5.2. Comparison to Cross Force
If the Equation (5.1) is equivalent to the cross force equations in (4.4), we shall have
![]() (5.3)
(5.3)
6. Some Special Results
6.1. Covariant Conventional Force
From comparison above, we have![]() , thus
, thus
![]()
![]() (6.1)
(6.1)
6.2. The Value of the Component fo
From Equation (5.3) then,
![]() (6.2)
(6.2)
6.3. The Equation of 4-Angular Velocity ![]()
Return above we have in the three-dimensional space
![]()
So, in the four-dimensional space time we Consider the ![]() and
and ![]() components are given by
components are given by ![]() bold line,
bold line, ![]() normal line, where
normal line, where ![]()
For E M case, Let A is the vector potential and թ = qA [1] then we get
![]() (6.3.1)
(6.3.1)
![]() (6.3.2)
(6.3.2)
6.4. The Value of թ0
The sub determinant of angular velocity ![]() is defined by
is defined by
![]()
From Equation (5.3) and Equation (4.3) we then get
![]()
which is equivalent to Equation (5.2).
![]()
![]()
![]()
where ∅ is scalar potential energy, so we can write the 4-coordinate momentum թ as,
![]()
For 4-vector potential A, we get [2]
![]()
6.5. Calculate the Dual to F'
We suppose that the dual force ![]() is defined by Equation (4.5) as the following
is defined by Equation (4.5) as the following
![]() (6.4)
(6.4)
where
![]()
7. Conclusion
In A.E space, all force equations [3] (e.g. Coriolis Force, Lorentz force, ordinary force, Maxwell’s Equations and others) are elegantly represented by two simple equations
![]()
8. Discussion
8.1. E M Field Tenors
Using the transformation in Equation (4.2) and Equation (4.3), we obtain
![]()
The Equation (6.3.1) and Equation (6.3.2) follow that
![]()
By assuming that![]() , then from Equation (5.3) we get
, then from Equation (5.3) we get
![]()
and so on. The overall result is [2]
![]()
By a similar argument, we can write the dual matrix as
![]()
8.2. Lorentz Force Law [4]
8.2.1. The First Force Equations on A.E Filed
Without the component![]() , the Equation (5.1) becomes
, the Equation (5.1) becomes
![]()
to get the first E M Lorentz force law. Let
![]()
It follows that
![]()
![]()
8.2.2. The Second Force Equations on A.E Filed
Without the component![]() , the the Equation (6.4) becomes
, the the Equation (6.4) becomes
![]()
to get the second E M Lorentz force law. Let
![]()
![]()
It follows that
![]()
![]()
8.3. The 4-Field Equations in Tensor Notation [2]
According to the equations above, we can define 4-Maxwell’s Equations by suppose that
![]()
8.3.1. The Inhomogeneous E M Maxwell’s Equations
By vector triple product we have
![]()
But
![]() , therefore
, therefore
![]()
8.3.2. The Homogeneous Equations
![]()
From Equation (3.3) thus
![]() , but
, but
![]() , then
, then
![]()
Acknowledgements
I have named the new space in this paper as A.E (Abou Layla-Erdogan’s) as an expression of my thanks and appreciation for the Turkish President’s humanitarian attitudes towards my people and appreciation for my Turkish friends that supported me during my high study in Turkey.