Proofs of the Density Theorem and Fatou’s Radial Limit Theorem Using the Poisson Integral

John Marafino

Department of Mathematics and Statistics, James Madison University, Harrisonburg, VA, USA.

DOI: 10.4236/oalib.1102732                

Abstract

Using only the Poisson integral and elementary convergence theorems, we prove the well-known Density theorem and Fatou’s radial limit theorem.

Keywords

Poisson Integral, Density, Radial Limits, Harmonic Functions

Subject Areas: Function Theory

1. Introduction

In most texts, [1] (p. 261), [2] (p. 187), [3] (p. 129), to name a few, the Density Theorem is proven using the fact that if f is Lebesgue integrable and if σ = òf, then σ¢ = f a.e. This result in turn is proven using the Vitali Covering Theorem. The same procedure is also used in the proof of Fatou’s radial limit theorem (see [4] , p. 129, and [5] , Vol. II, p. 362-364). We circumvent this reasoning in an attempt to make the Density and Fatou theorems more accessible to the reader. Our major reference will be Royden’s book Real Analysis, and we will restrict ourselves to its first four chapters. These include a general introduction to set theory, the real number system, Lebesgue measurable functions, and the Lebesgue integral. Our major analytical tool will be the Poisson integral and we will use some of its well-known fundamental properties.

We first introduce the background material that will be used in the course of this note. Our setting is the unit disk D and its boundary C. We shall say that a sequence {I_k} of arcs in C converges to 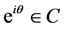and write I_k ® e^iθ, 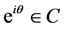, if for each k and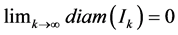. Let A be any subset of C and let m^* and m denote respectively the outer Lebesgue measure and the Lebesgue measure on C. For any measurable set E in C we define. We shall say that the derivative of σ_A at e^iθ exists if there exists a number such that for any sequence {I_k} of arcs converging to e^iθ,

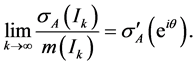
If, e^iθ is called a point of density of A and if, e^iθ is called a point of dispersion of A.

The Density Theorem states that if A is any set (measurable or not) in C, then e^iθ is a point of density for A for almost all e^iθ in A. We shall prove that this result in the case A is measurable.

Let u(z), 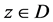, be defined by the Poisson integral,

(1)

where 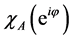is the characteristic function on the measurable set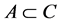, and

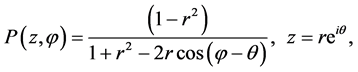
is the Poisson kernel. When A is a finite union of open arcs, then χ_A is a bounded piecewise continuous function on C. So, if ξ is a point of continuity of χ_A, then a straight-forward examination of the integral in (1) shows that the limit of u(z), as z approaches ξ exists and equals χ_A(ξ) (see [6] , Vol. II, p. 156; [7] , p. 206; and [4] , p. 130). The kernel has two nice properties:

i) 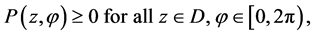and

ii) (see [8] , p. 165-167 and [9] , p. 305-307).

It follows that for all. We will use these elementary results in Sections 2 and 3.

2. The Density Theorem

Theorem: Let A be measurable. Then almost every point of A is a point of density of A.

Proof: Let B be those points of A that are not points of density of A; that is,

where I_δ, δ > 0, denotes an arc containing e^iθ having length δ. For each, let. We will first show that B can be rewritten as

where is an arc centered at e^iθ with length 2ξ_k such that ξ_k → 0 as k → ∞. This reformulation of B is

crucial to our proof. Clearly, any point in this set is in B. Now suppose. Then there exists a positive number ε and a sequence {δ_n} such that δ_n ® 0 as n ® ¥ and

For each n let s_n denote the length of the largest component of and choose k_n such that. Then

Since the last expression approaches 1 − ε/2 as k_n ® ¥, it follows that

Using this reformulation of B, the facts that for each k, is a continuous function of θ and consequently measurable, theorem 20 in [10] (p. 56), and that A is a measurable set, it readily follows that B is a measurable set. Hence, it is the union of an F-σ set and a set N of measure zero; that is,

where each F_n is a closed subset of B. If we show each F_n has measure zero, then the theorem is proved. We pick a F_n and denote it by F in order to avoid layered subscript notation. Now, where O_k are pairwise

disjoint open arcs. For each, let, χ_n be the characteristic function of D_n, and set for. Note that for all n, , and. We know from our introductory remarks that except for a finite number of e^iθ on C,. Let S_n denote this exceptional set. If we set, then for all and for all n,

(2)

Also, for all e^iθ Î C,

(3)

We now show that m(F) = 0 using an indirect argument. Before we formally proceed we indicate the direction our proof will take: We define the Poisson integral of the characteristic function on F and using Equations (2) and (3), along with the assumption that F has positive measure, find a subset of F where the radial limit of this function is 1. We then use the reformulation of B to show that this cannot happen.

So, suppose that m(F) > 0. Momentarily fix θ. For each, define. Then r_k ® 1 as k ® ¥. Since for each k, is a nonnegative and integrable function of j, we have ( [10] , p. 73) that for each ξ_k there exists δ_k > 0 such that if E is any set with m(E) < δ_k, then

(4)

For each, let. Using Egorov’s Theorem ( [10] , p. 59), we have for each k an open set M_k such that

and uniformly on M_k. (5)

Note that, and since S is at most countable, has positive measure. Set.

We now claim that if e^iθ is in, then. Let ε be an arbitrary positive number and choose K such that for k > K, ξ_k < ε/4. Now for k > K,

Since χ_n converges uniformly to χ_F on M_k we choose N = N(ε) such that on M_k. Thus,

Using (4) with the fact that one also has

Hence, for all k > K. Because, Equation (2) holds. In addition, we have for all n that χ_n = χ_F on F. Consequently, for k sufficiently large,

and since ε was arbitrary, our claim is established.

However, using the reformulation of B we know that since and, there exists a positive number η and a subsequence of arcs with the property that

Since, we have

Since the last expression approaches η/2π as or as, we have that the

and this contradicts our previous claim. Thus, the measure of F cannot be positive and so m(F) = 0.

3. Density and the Radial

In this section we establish relationships between the density of A at a point of C and the radial limit of the Poisson integral of the characteristic function on A at this point. The proofs of the first two theorems employ well-known procedures and inequalities. Theorem 3 highlights the last result in the proof of the Density Theorem. We then use these relationships and prove in Corollary 5 Fatou’s radial limit theorem.

Theorem 1. If e^iθ is a point of density of A and u(z) represents the Poisson integral of, then the radial limit of u(z) at e^iθ is 1.

Proof: Without loss of generality we can assume e^iq = eⁱ⁰ = 1 and we express the Poisson integral of χ_A over the interval [−π, π] instead of [0, 2π]. We must show that.

Since 1 is a point of density of the measurable set A, it follows that 1 is a point of dispersion of A^C, the complement of A with respect to C. Letting, where I_δ(1) denotes the arc on C centered at 1with length 2δ, we know that ε(δ) ® 0 as δ ® 0. Let z = r. Then

(6)

There are two possibilities that can happen with the function ε(δ), δ > 0. Either there exists a δ₀ such that ε(δ₀) = 0; or for all δ, ε(δ) ≠ 0.

In the first case it follows that for all. We now rewrite (6) as

In the first integral we know that; and for,. Hence, the integral is zero. For the second integral we have that and so. Now

On [0, π/2] we have that sin(x) ≥ (2/π)x. So if r > 1/2 we get, and hence

The third integral is handled like the second, and so our theorem follows.

In the second case it follows that if δ is sufficiently small, is a continuous increasing function of δ. So there exists a δ₀ such that the expression is continuous and increasing

on (0, δ₀). Consequently, the expression is a continuous increasing function of δ on (0, δ₀) with the

property that as δ→0. Using this result we know that as r ® 1, 1 − r ® 0 and so it can be

represented by for some δ_r. Furthermore, 1 - r ® 0 iff δ_r ® 0. On the interval [−δ_r, δ_r] we use the inequality to get

We now rewrite (6) as was done in the first case but using δ_r instead of δ₀. The first integral is handled above. The second integral is handled exactly as before. We get

The third integral is handled like above. Hence, our theorem follows. Using a similar argument we can obtain the second result of this section.

Theorem 2. If e^iθ is a point of dispersion of A, then the radial limit of u(z) at e^iθ is 0.

Theorem 3. If e^iθ is neither a point of density of A nor a point of dispersion of A, then the radial limit, if it exists, cannot be 1 or 0.

Proof: From the hypothesis we know there exists an ε > 0 and two sequences of arcs, where δ_n and ρ_n denote the length of and respectively, such that for all n, for all n,

for all n,

for all n,

and δ_n ® 0 and ρ_n ® 0 as n ® ¥. Since the arcs are not centered at e^iθ we define ξ_n to be the maximum of the lengths of the components of and define to be the arc centered at e^iθ with length 2ξ_n. Using we define τ_n similarly. Let and. Then,

Since the last expression approaches ε/4π as ξ_n ® 0 or as r_n ® 1, we have that the

(7)

A similar argument using shows that the. It follows that if the radial limit of u(z) at e^iθ exists, then it cannot be 1 or 0.

Corollary 1: Let A be any measurable set in C. Let Then for almost every e^iθ Î C,.

Proof: Using the Density Theorem on A and Theorem 1, we have for almost every e^iθ in A,. Since A^C is also measurable we have that almost every point of A^C is a point of density of A^C. Hence, almost every point of A^C is a point of dispersion of A. By Theorem 2 we have that for almost every e^iθ in A^C,.

Corollary 2: Let f be a simple function defined on C. Let Then for almost every e^iθ Î C,

Proof: If f is simple, then where the A_i are pair-wise disjoint and measurable and the a_i are distinct and nonzero. Using Corollary 1, our result follows.

Corollary 3: Let f be a bounded measurable function defined on C. Let Then for almost every e^iθ Î C,

Proof: Since f is bounded and measurable, there exists simple functions ψ_n, ψ_n £ f, such that uniformly on C. By adding and subtracting the appropriate terms and using the triangle inequality one can show that

We analyze each term on the right hand side of this inequality. Since the kernel P(z, j) is non-negative and its definite integral is 2π (see Section 1), and since ψ_n converges uniformly to f on C, the first term can be made arbitrarily small when n is sufficiently large. From Corollary 2 we get that the second term can be made arbitrarily small as r approaches 1. The last term approaches 0 for n sufficiently large since ψ_n converges uniformly to f on C. Consequently, our result follows.

Corollary 4: Let f be a nonnegative integrable function defined on C. Let Then for almost every,

Proof: Let. Since f is integrable we know that m(S) = 2π. For each, define. Each f_n is bounded, nonnegative, and measurable on S. The functions also satisfy the following on S: and Since P(z, j) is non-negative we have that and hence on S. By the Monotone Convergence Theorem ( [10] , p. 72) we know that

Once again, by adding and subtracting the appropriate terms and using the triangle inequality one can show that

From our above remarks, the first term can be made arbitrarily small for n sufficiently large. Using Corollary 3, the second term can be made arbitrarily small as r approaches 1. The last term approaches 0 on S as n gets large. Consequently, our result follows.

Corollary 5: (Fatou) Let f be integrable on C. Let Then for almost every e^iθÎC,

Proof: We know that where and. Since f is integrable, both and must be integrable. We can now use Corollary 4 on and to get our result.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Natanson, I.P. (1961) Theory of Functions of a Real Variable, Volume I. Frederick Ungar Publishing Co., New York.
[2]	Munroe, M.E. (1971) Measure and Integration. 2nd Edition, Addison Wesley, Massachusetts.
[3]	Saks, S. (1964) Theory of the Integral. 2nd Revised Edition, Dover Publications Inc., New York.
[4]	Tsuji, M. (1975) Potential Theory in Modern Function Theory. 2nd Edition, Chelsea Publishing Co., New York.
[5]	Hille, E. (1976) Analytic Function Theory, Volume II. 2nd Edition, Chelsea Publishing Co., New York.
[6]	Markushevich, A.I. (1977) Theory of Functions of a Complex Variable, Three Volumes in One. 2nd Edition, Chelsea Publishing Co., New York.
[7]	Nevanlinna, R. and Paatero, V. (1982) Introduction to Complex Analysis. 2nd Edition, Chelsea Publishing Co., New York.
[8]	Ahlfors, L.V. (1966) Complex Analysis. 2nd Edition, McGraw Hill Publishing Co., New York.
[9]	Churchill, R.V. and Brown, J.W. (1990) Complex Variables and Applications. 5th Edition, McGraw Hill Publishing Co., New York.
[10]	Royden, H.L. (1963) Real Analysis. The Macmillan Co., New York.