The Approximate Analytical Solution of Non-Linear Equation for Simultaneous Internal Mass and Heat Diffusion Effects ()
Received 17 May 2016; accepted 26 June 2016; published 29 June 2016

1. Introduction
In many engineering and industrial applications, catalytic processes in chemical reactors are often considered to be very useful. This induces particular attention to the study of catalytic reactions at the single-particle level [1] . Moreover, the reaction behavior of porous catalyst particles had been studied over nearly a quarter of a century [2] - [4] . Majority of chemical reactions are accompanied by heat transfer effects; they either release or absorb heat. This can lead to appreciable increase (or decrease) of temperature toward the particle centre [5] - [7] . Since chemical reaction rates vary rapidly increase with temperature, this effect could radically change the behavior of the catalyst particles. Analysis of chemical kinetics with diffusion effects usually leads to solving strongly nonlinear differential equations. Detailed reviews of mathematical models describing reactions in porous catalyst particle can be found in [8] . Assuming a flat geometry for the particle and that conductive heat transfer is negligible compared to convective heat transfer. The approximate behavior of the functional forms is sufficiently similar for various geometric forms [9] [10] so that the spherical particle is a approximation [11] [12] for most cases encountered, such as cylindrical pellets, or irregular granules. When the chemical reaction is accompanied by a heat effect, not only a mass concentration gradient, but also appreciable temperature gradients can exist within the particle. Weisz and Hicks [13] solved the non-linear mass balance equation using numerical method.
However, to the best of our knowledge, there was no rigorous analytical solution for the concentration of reactant of catalyst having been reported. The purpose of this communication is to derive simple analytical expression for concentration and utilization factor for all possible values of reaction/diffusion parameters using the modified Adomian decomposition method.
2. Mathematical Formulation of the Problem
The dimensionless mass transport equation of porous catalyst particle is [13]
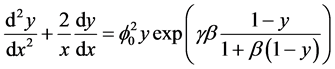 (1)
(1)
where
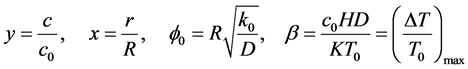 (2)
(2)
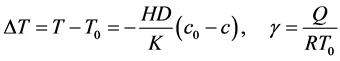 (3)
(3)
where y is the dimensionless concentration, x is the dimensionless radius of the spherical catalyst pellet, c is the dimensionless concentration of reactant, K is thermal conductivity, H is molar heat of reaction. The parameter 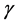 expresses the sensitivity of the reaction rate to temperature;
expresses the sensitivity of the reaction rate to temperature;  is the maximum temperature variation
is the maximum temperature variation 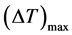 which could exist within the particle relative to the boundary temperature. The boundary conditions are
which could exist within the particle relative to the boundary temperature. The boundary conditions are
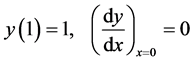 (4)
(4)
The utilization factor 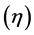 is given by
is given by
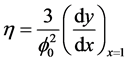 (5)
(5)
3. Analytical Expression of the Concentration Using Modified Adomian Decomposition Method (MADM)
In the recent years, much attention is devoted to the application of the Adomian decomposition method to the solution of various scientific models [14] . The MADM yields, without linearization, perturbation, transformation or discretisation, an analytical solution in terms of a rapidly convergent infinite power series with easily computable terms. The decomposition method is simple and easy to use and produces reliable results with few iterations. The rate of convergence of modified Adomian decomposition method is higher than standard Adomian decomposition method [15] - [17] . Using this method (see Appendix A), we can obtain the analytical expression of concentration (see Appendix B), of the substrate as follows:
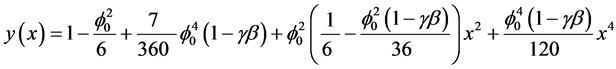 (6)
(6)
Using Equations (5) and (6), we can obtain the effectiveness factor
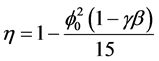 (7)
(7)
The Equation (6) and (7) represent the new and simple analytical expression of concentration of substrate and effectiveness factor provided
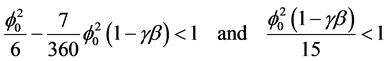 (8)
(8)
4. Numerical Simulation
The diffusion Equation (1) for the boundary condition (Equation (4)) is also solved numerically. We have used the function pdex1 in MATLAB software to solve numerically the initial-boundary value problem for the nonlinear differential equation. This numerical solution is compared with our analytical results in Figure 1 and Figure 2. Upon comparison, it gives a satisfactory agreement for all values of the dimensionless parameters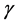 ,
, 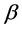 and
and . The Matlab program is also given in Appendix C.
. The Matlab program is also given in Appendix C.
5. Discussion
The nonlinear system for coupled heat and mass transfer in a spherical non-isothermal catalyst pellet is solved analytically. The concentration of substrate depends on the following three factors ![]() and
and![]() .
. ![]() is the activation energy parameter and
is the activation energy parameter and ![]() is the heat of reaction parameter which represents the ratio of the characteristic time of the enzymatic reaction to that of substrate diffusion.
is the heat of reaction parameter which represents the ratio of the characteristic time of the enzymatic reaction to that of substrate diffusion.
Figure 1(a) and Figure 1(b) show the dimensionless concentration of substrate y for various dimensionless pellet raidus x. The concentrations were computed for various values of the dimensionless parameter. From Figure 1(a) and Figure 1(b), it is evident that the value of concentration ![]() when
when ![]() and
and ![]() for all values of
for all values of ![]() and
and![]() . The concentration differs significantly for all values of parameters
. The concentration differs significantly for all values of parameters ![]() and
and![]() . The value of the concentration y decreases when
. The value of the concentration y decreases when ![]() increases.
increases.
The normalized numerical simulation of three dimensional substrate concentration y versus dimensionless pellet radius x is shown in Figures 2(a)-(c). The time independent concentration y is represented in Figures 2(a)-(c). For fixed value of![]() , concentration
, concentration ![]() is slowly decreasing when
is slowly decreasing when ![]() is increasing. Then
is increasing. Then
the concentration of ![]() when
when ![]() and also for all values of
and also for all values of![]() ,
, ![]() and
and![]() . From these figure, it should be noted that the value of the concentration of substrate decreases for all values of
. From these figure, it should be noted that the value of the concentration of substrate decreases for all values of![]() . From this Figures, it is apparent that the value of the concentration of substrate increases when the values of
. From this Figures, it is apparent that the value of the concentration of substrate increases when the values of ![]() increases.
increases.
The variation in effectiveness factor for various values of ![]() and
and ![]() using Equation (7) is shown in Figure 3 and Figure 4. From Figure 3, it is evident that the effectiveness factor increases with the increasing value of the dimensionless parameter
using Equation (7) is shown in Figure 3 and Figure 4. From Figure 3, it is evident that the effectiveness factor increases with the increasing value of the dimensionless parameter![]() . From Figure 4, it is also observed that the effectiveness factor increases with the increasing value of the dimensionless parameter
. From Figure 4, it is also observed that the effectiveness factor increases with the increasing value of the dimensionless parameter![]() .
.
6. Conclusion
In this work, we have discussed the mathematical model of catalyst particle in a porous medium through which reactants diffuses. We have obtained the approximate analytical expression for the steady state concentration of substrate for all values of ![]() and
and ![]() in a packed bed reactor using the modified Adomian decomposition method. A satisfactory agreement with the numerical result is noted. Moreover, we have also presented a closed form expression for the utilization factor. The proposed model can be used to solve the nonlinear convective mass and heat diffusion problems.
in a packed bed reactor using the modified Adomian decomposition method. A satisfactory agreement with the numerical result is noted. Moreover, we have also presented a closed form expression for the utilization factor. The proposed model can be used to solve the nonlinear convective mass and heat diffusion problems.
Acknowledgements
The authors express their gratitude to the reviewers for their valuable comments to improve the quality of the manuscript. This work was supported by the Department of Science and Technology (DST) (No. SB/SI/PC- 50/2012), New Delhi, India. The authors are thankful to the Head of the Department of Mathematics, Principal and Chairman of Sethu Institute of Technology, Kariapatti for their encouragement.
Appendix A. Basic Concept of Modified Adomian Decomposition Method [16]
Consider the nonlinear differential equation in the form
![]() (A.1)
(A.1)
with initial condition
![]() (A.2)
(A.2)
where ![]() is a real function,
is a real function, ![]() is the given function and A and B are constants. The differential operation is proposed as follows [17]
is the given function and A and B are constants. The differential operation is proposed as follows [17]
![]() (A.3)
(A.3)
So, the problem (A.1) can be written as,
![]() (A.4)
(A.4)
The inverse operator ![]() is therefore considered a two-fold integral operator, as below.
is therefore considered a two-fold integral operator, as below.
![]() (A.5)
(A.5)
Applying ![]() of (A.5) to the first three terms
of (A.5) to the first three terms ![]() of Equation (A.1) we find
of Equation (A.1) we find
![]() (A.6)
(A.6)
By operating ![]() on (A.4), we have
on (A.4), we have
![]() (A.7)
(A.7)
The Adomian decomposition method introduce the solution ![]() and the nonlinear function
and the nonlinear function ![]() by infinity series
by infinity series
![]() (A.8)
(A.8)
![]() (A.9)
(A.9)
where the components ![]() of the solution
of the solution ![]() will be determined recurrently and the Adomian polynomials
will be determined recurrently and the Adomian polynomials ![]() of
of ![]() are evaluated [22, 23, 25] using the formula
are evaluated [22, 23, 25] using the formula
![]() (A.10)
(A.10)
By substituting (A.8) and (A.9) into (A.7),
![]() (A.11)
(A.11)
Through using the Adomian decomposition method, the components ![]() can be determined as
can be determined as
![]() (A.12)
(A.12)
which gives
![]() (A.13)
(A.13)
From (A.9) and (A.12), we can determine the components![]() , and hence the series solution of
, and hence the series solution of ![]() in (A.7) can be immediately obtained.
in (A.7) can be immediately obtained.
Appendix B: General Solution of Equation (1) Using the Adomian Decomposition Method
In this appendix, we derive the general solution of nonlinear Equation (1) by using the Adomian decomposition method. We write the Equation (1) in the operator form,
![]() (B.1)
(B.1)
where![]() . Applying the inverse operator
. Applying the inverse operator ![]() on both sides of Eqn. (B.1) yields
on both sides of Eqn. (B.1) yields
![]() (B.2)
(B.2)
where A and B are the constants of integration. We let,
![]() (B.3)
(B.3)
and
![]() (B.4)
(B.4)
where
![]() (B.5)
(B.5)
In view of Equations (B. 3 - B. 5), Equation (B. 2) gives
![]() (B.6)
(B.6)
We identify the zeroth component as
![]() (B.7)
(B.7)
Using the boundary condition (4) we get,
![]() (B.8)
(B.8)
and the remaining components can be obtained using the recurrence relation
![]() (B.9)
(B.9)
where ![]() are the Adomian polynomials of
are the Adomian polynomials of![]() . We can obtain the first few
. We can obtain the first few ![]() as follows:
as follows:
![]() (B.10)
(B.10)
![]() (B.11)
(B.11)
The remaining polynomials can be generated easily, and so,
![]() (B.12)
(B.12)
![]() (B.13)
(B.13)
Adding (B. 8), (B. 12) and (B. 13) we get the Equation (6) in the text.
Appendix C: The Matlab Program to Find the Numerical Solution of Equation (1)
function pdex1
m = 2;
x = linspace(0,1);
t = linspace(0,100);
sol = pdepe(m,@pdex1pde,@pdex1ic,@pdex1bc,x,t);
u = sol(:,:,1);
surf(x,t,u)
title('Numerical solution computed with 20 mesh points.')
xlabel('Distance x')
ylabel('Time t')
figure
plot(x,u(end,:))
title('Solution at t = 2')
xlabel('Distance x')
ylabel('u(x,2)')
% --------------------------------------------------------------
function [c,f,s] = pdex1pde(x,t,u,DuDx)
c = 1;
f = DuDx;
Q=1;
B=1.5;
r=1;
s =-(Q^2)*u*exp(r*B*(1-u)/(1+B*(1-u)));
% --------------------------------------------------------------
function u0 = pdex1ic(x)
u0 = 1;
% --------------------------------------------------------------
function [pl,ql,pr,qr] = pdex1bc(xl,ul,xr,ur,t)
pl = 0;
ql = 1;
pr = ur-1;
qr = 0;
Nomenclature
CA Concentration of reactant A inside the catalyst pellet (mole/cm3)
CA,s Concentration of reactant A at the surface of catalyst pellet (mole/cm3)
![]() Effective diffusivity inside the catalyst pellet (cm2∙s−1)
Effective diffusivity inside the catalyst pellet (cm2∙s−1)
E Activation energy (kJ∙mol−1)
rA Arrhenius reaction rate (s−1)
Rg Universal gas constant (8.3145 J∙k−1∙mol−1)
T Temperature inside the catalyst pellet (K)
Tref Reference temperature (K)
Ts Temperature at the surface of catalyst pellet (K)
x Dimensionless radius of the spherical catalyst pellet (none)
y Dimensionless concentration along radial direction of catalyst pellet (none)
Greek Symbols
![]() Dimensionless heat reaction
Dimensionless heat reaction
![]() Dimensionless activation energy
Dimensionless activation energy
![]() Effectiveness factor
Effectiveness factor
![]() Thiele modulus
Thiele modulus
![]()
Submit your manuscript at: http://papersubmission.scirp.org/
NOTES
![]()
*Corresponding author.