1. The Flow Game, a Model for Gas Routing
The flow games have been introduced by E. Kalai and E. Zemel (1982), in [1] , as a class of cooperative transferable utilities games generated on a graph by means of a max-flow-min-cut algorithm. We use this model to show how this may be used for gas routing, in case that the gas extracted by several companies is sent to a common depot, while the distribution is done by a public company. An example with three companies and a graph of individual ducts belonging to each company would help in the understanding of any similar situation.
Example 1. Consider the case of a city which has wells belonging to three companies, K1, K2, K3, at the nodes A, B, C, of a graph, respectively. The companies are able to extract natural gas as follows: company K1, 11 tones at A; company K2, 13 tones at B; and company K3, 9 tones at C. The set of nodes in the graph is 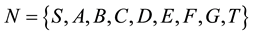 ; where S is an auxiliary common source, T is an auxiliary common sink, and the arcs are belonging to the companies as follows:
; where S is an auxiliary common source, T is an auxiliary common sink, and the arcs are belonging to the companies as follows: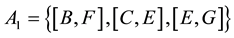 ,
,  ,
, 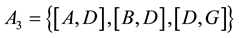 , with capacities shown in the matrix below. There is also a public duct, belonging to the city, connecting the node G to the depot node T, from which a distribution company is allocating the deliveries to customers throughout the city, on a public network. Let this arc be [G, T], with the capacity 25. We added an auxiliary node S and the auxiliary arcs [S, A], [S, B], [S, C], with capacities equal to the production power of each company, that is 11, 13, 9, respectively, for computational purposes. By inspection we easily determine that no gas producing company can send to the depot alone any amount of gas, so that if we denote the amounts provided by the singletons by
, with capacities shown in the matrix below. There is also a public duct, belonging to the city, connecting the node G to the depot node T, from which a distribution company is allocating the deliveries to customers throughout the city, on a public network. Let this arc be [G, T], with the capacity 25. We added an auxiliary node S and the auxiliary arcs [S, A], [S, B], [S, C], with capacities equal to the production power of each company, that is 11, 13, 9, respectively, for computational purposes. By inspection we easily determine that no gas producing company can send to the depot alone any amount of gas, so that if we denote the amounts provided by the singletons by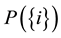 ,
,  , we have
, we have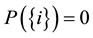 ,
,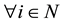 . Instead, for each pair of companies,
. Instead, for each pair of companies, 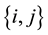 , by using all the arcs belonging to the pair
, by using all the arcs belonging to the pair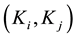 , we obtain the maximal amounts provided by the corresponding coalitions, listed as
, we obtain the maximal amounts provided by the corresponding coalitions, listed as

while the cooperation of all companies will give
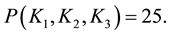
The maximal amounts for coalitions of size two are obvious from the figure; the maximal amount provided by the grand coalition should be computed in the network by a max-flow-min-cut algorithm, for example the Ford- Fulkerson algorithm (see [2] ). If there are more companies producing natural gas, the game is larger and the computation is more difficult, but the approach may be the same.
The capacities of the ducts represented by the arcs of the graph are shown in the following figure:
A graph with capacities associated to the above model.
The graph has the arcs for which there are positive capacities in the table, plus the entering and exiting auxiliary arcs, and one public arc discussed below. Now, note that the columns for S and T and the rows for G and T have been omitted, because they are empty, except a public duct, belonging to the city, [G, T], with a capacity of 25. The graph and the capacities are taken arbitrarily by the author.
As shown by the numbers that give the characteristic function of the game, the three companies should cooperate in order to get a maximum benefit. Now, the three-person cooperative TU game and any fair schedule of allocations, are obtained by using an efficient value of the game:
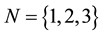
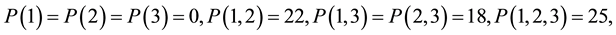
where we replaced the names of the companies by the indices and the brackets by parantheses, to keep the notation simple.
As the worth of singletons is zero, a simpler value like the Center of the Imputation Set, expressed by the formula:
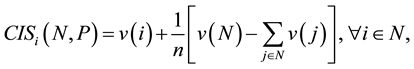
gives
![]()
which is not fair. Therefore, we shall use the Shapley Value, given by the formula:
![]()
which has more properties due to its axiomatic definition (see [2] ). By using the formula, we obtain ![]() . The Core of the game is given by the conditions
. The Core of the game is given by the conditions
![]()
Obviously, the Shapley Value does not belong to the Core, as
![]()
and all the other inequalities for coalitions of size two do not hold, that is the Shapley Value is not coalitional rational, even though it is efficient, as the sum of components makes 25. Of course, any pair of companies may break the grand coalition and make a two person coalition which may give more gain to each player, while the third player is left out of the deal, an unfair solution. This instability is due exactly to the fact that the solution is not coalitional rational, which could be a motivation for the present work.
To be able to give a fair allocation, we shall solve a connected problem that has been discussed in an earlier work of the author (see [3] ). To get the paper self contained we shall remind some concepts and results from the mentioned work. The new problem introduced there is: find out a new game, with the same Shapley Value, which is coalitional rational in the new game. This problem is connected to the Inverse Problem for the Shapley Value, introduced also in some work of the author (see [4] ).
2. The Inverse Problem and the Coalitional Rationality
In [4] , the Inverse Problem for the Shapley Value has been introduced and solved. For the Shapley Value the problem may be stated: let ![]() be the Shapley Value of a cooperative TU game with n players; find out the set of all TU games with n players, for which the Shapley Value equals L. In [3] , a connected problem has been introduced and solved: let
be the Shapley Value of a cooperative TU game with n players; find out the set of all TU games with n players, for which the Shapley Value equals L. In [3] , a connected problem has been introduced and solved: let ![]() be the Shapley Value of a cooperative TU game; find out a new game, with the same Shapley Value, in which the Shapley Value is coalitional rational. In other words, in the Inverse Set relative to the Shapley Value, find out a game in which the Shapley Value is in the Core of the game. To sketch the use of the results from [3] and [4] in the gas routing problem, we give here some earlier results.
be the Shapley Value of a cooperative TU game; find out a new game, with the same Shapley Value, in which the Shapley Value is coalitional rational. In other words, in the Inverse Set relative to the Shapley Value, find out a game in which the Shapley Value is in the Core of the game. To sketch the use of the results from [3] and [4] in the gas routing problem, we give here some earlier results.
It is well known that the set of TU games with the set of players N, forms a vector space of dimension![]() , (see [2] ). Hence, any game may be written as a vector in that space, for example in R7 for our game of Example 1. The results of Linear Algebra will be useful in this respect. A basis for the vector space was introduced in [4] , by the formulas
, (see [2] ). Hence, any game may be written as a vector in that space, for example in R7 for our game of Example 1. The results of Linear Algebra will be useful in this respect. A basis for the vector space was introduced in [4] , by the formulas
![]()
where the basic vectors are defined for ![]() by
by![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , otherwise, while
, otherwise, while![]() ,
, ![]() , otherwise.
, otherwise.
As mentioned above all these vectors, invented in [4] , are linearly independent, hence they form a basis; an easy exercise is to write the seven basic vectors for our example 1. Now, any game ![]() in the vector space may be written as a linear combination of the basic vectors as
in the vector space may be written as a linear combination of the basic vectors as
![]()
where the coefficients are constants which may be determined from this vector equation written in scalar form. To solve the Inverse Problem we used the results:
![]()
and the linearity of the Shapley Value. By applying these results in the above expansion of![]() , we get
, we get
![]()
After eliminating the constants![]() , we obtain an explicit formula for the games in the Inverse Set relative to the Shapley Value L:
, we obtain an explicit formula for the games in the Inverse Set relative to the Shapley Value L:
![]()
(see [4] , Theorem 3.5).
As mentioned above, we shall be looking for a new game in the Inverse Set relative to the Shapley Value L, which is coalitional rational. We shall confine ourselves to find such a game in the subfamily of the Inverse Set defined by all ![]() that is in the set of games
that is in the set of games
![]()
This subset of the Inverse Set will be called the almost null subfamily. Taking into account the expressions of the basic games shown above, the games in the family given by this vector formula, may be rewritten in scalar form as
![]()
![]()
![]() otherwise (*)
otherwise (*)
Now, the Core conditions written together give an inequality determining the values of parameter ![]() for those games in which the Shapley Value is coalitional rational:
for those games in which the Shapley Value is coalitional rational:
![]() (**)
(**)
where the minimum is taken over the index i.
The results already shown provide a procedure for solving our above stated problem:
・ Compute the Shapley Value of the given game. Check whether, or not, the Shapley Value is in the Core. If yes, stop, the problem is solved; otherwise.
・ Compute the game in the Inverse Set given by (*), for the Shapley Value L.
・ Take a value for cN in formula (*). to satisfy the inequality (**), and find out the desired game in the almost null subfamily.
Now, we intend to apply the results to the gas routing problem.
3. An Application to the Gas Routing Problem
Return to the Example 1 given in the first section, in which we associated to the network the cooperative TU game obtained by finding for each coalition the maximum flow that could be delivered by using the ducts owned by each company in the coalition and the final public arc. The TU game is
![]()
and we can compute the Shapley Value:![]() .
.
Obviously, this is an efficient vector, but it is not belonging to the Core, because
![]() ,
,![]() ,
,![]() ,
,
are not satisfied. Then, start the procedure described above, by deriving the inequality (**):
![]()
We choose the largest value of the parameter that satisfies the inequality, ![]() , and plug into the formulas (*):
, and plug into the formulas (*):
![]()
where the null worth of the singletons is omitted. We obtain
![]()
Further, we can compute again the Shapley Value and find out the same vector as before. This is not only efficient, but also coalitional rational, because the Core conditions hold. A similar situation and a similar strategy may be used for the Shapley Value in the general case of games with any number of players. Moreover, the procedure will work also for any other value which is efficient. A value which is not efficient is requiring a new definition of the coalitional rational value.
The procedure may be extended to this case by using the results following from the ideas developed in the joint paper [5] , which were used in the paper [6] .
Note that the present paper is an application of the results obtained in the previous paper [3] , while for more general cases the Inverse set defined in [6] is needed.