About Lorentz Invariance and Gauge Symmetries: An Alternative Approach to Relativistic Gravitation ()
1. Introduction
In the usual presentations of general relativity [1] , space-time geometry is described via any set of 4 curvilinear coordinates ; an elementary space-time path
; an elementary space-time path 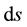 is expressed as
is expressed as 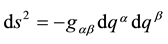 where the quadratic form
where the quadratic form 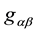 characterizes the local metrics. The stake is to express the laws of physics in that frame;
characterizes the local metrics. The stake is to express the laws of physics in that frame;
for example in the absence of other external forces than gravitation, a particle follows the minimum path 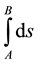
between two space-time points A and B. In the presence of a gravitation field there is no global transformation of the coordinates allowing the 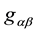 to take in the whole space-time the simple form of a Minkowski metrics; nevertheless it can take this special form in a particular point by a suitable change of coordinates which simply consists in diagonalizing
to take in the whole space-time the simple form of a Minkowski metrics; nevertheless it can take this special form in a particular point by a suitable change of coordinates which simply consists in diagonalizing 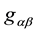 in this point [2] .
in this point [2] .
We give here an alternative presentation of a relativistic theory of gravitation, equivalent to general relativity, in which gravitation is introduced as a gauge field associated to restricting the global Lorentz invariance of special relativity to a local one.
The presentation of gravitation as a gauge field has been first introduced by Lanczos in the context of a variational approach to general relativity [3] [4] . Then it has been highlighted in an extension of the Yang-Mills approach of particle physics [5] - [7] . In the later, the fundamental interactions are accounted for by the restriction of gauge symmetries from a global invariance to a local one [8] . Those interactions (electro-magnetic, weak, strong) are associated to symmetry groups isomorphic to , whose elements are unitary transformations [9] [N.B.: by isomorphism between two groups, we mean that their Lie algebras possess the same commutation relations]. When that approach is applied to gravitation, the equivalence principle, central in general relativity [10] , is not explicitly assumed but rather derived from the fact that the gauge field is supposed to be minimally coupled.
, whose elements are unitary transformations [9] [N.B.: by isomorphism between two groups, we mean that their Lie algebras possess the same commutation relations]. When that approach is applied to gravitation, the equivalence principle, central in general relativity [10] , is not explicitly assumed but rather derived from the fact that the gauge field is supposed to be minimally coupled.
That restriction from a global invariance to a local one, associated to the emergence of a force field, is indeed a deep similarity between gravitation and the other interactions. However the Lorentz group is isomorphic to the special complex linear group  whose elements are not unitary and this is an essential difference between gravitation and the other interactions.
whose elements are not unitary and this is an essential difference between gravitation and the other interactions.
In the present work, we assume that the Lorentz group invariance is not a global symmetry of space-time but a local symmetry of a 4-dimension hyper-surface, which can be thought of as embedded in a space with a larger number of dimensions and we consider the transformations of a local set of pseudo-orthonormal coordinates and not the geometry of the 4-dimension hyper-surface described by a set of curvilinear coordinates. This is a major difference with other presentations of relativistic gravitation; its interest is to lead to facially simpler expressions although that difference is essentially formal.
2. Gauge Properties
Let us consider the 4-dimension space-time of special relativity and a pseudo-orthonormal base  with the Minkowski metrics
with the Minkowski metrics
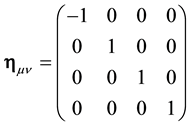 (1)
(1)
Any infinitesimal transformation R of the Lorentz group can be written as
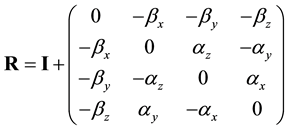 (2)
(2)
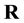 conserves the pseudo norm
conserves the pseudo norm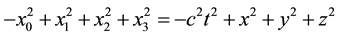 . It can alternatively be written as
. It can alternatively be written as
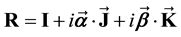 (3)
(3)
where 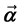 et
et ![]() are 2 vectors of the ordinary 3-dimension-space.
are 2 vectors of the ordinary 3-dimension-space. ![]() are the 6 infini- tesimal generators of the Lorentz group
are the 6 infini- tesimal generators of the Lorentz group![]() , the
, the ![]() s are hermitic
s are hermitic![]() , whereas the
, whereas the ![]() s are antihermitic
s are antihermitic![]() , the pseudo vector
, the pseudo vector ![]() and the true vector
and the true vector ![]() respectively account for rotations and for Lorentz transformations;
respectively account for rotations and for Lorentz transformations; ![]() stands for a rotation angle around the Z axis, and
stands for a rotation angle around the Z axis, and ![]() for the velocity of a Lorentz transformation along the Z axis.
for the velocity of a Lorentz transformation along the Z axis.
We introduce the 2 anti-symmetric tensors
![]() (4)
(4)
and
![]() (5)
(5)
Each component ![]() or
or ![]() is a 4 × 4 matrix so that
is a 4 × 4 matrix so that ![]() can be written as
can be written as
![]() (6)
(6)
More generally, any transformation of the Lorentz group can be figured by
![]() (7)
(7)
We now assume that the Lorentz group invariance is not a global symmetry of space-time but a local symmetry of a 4-dimension hyper-surface, which can be thought of as embedded in a space with a larger number of dimensions, for example 10 as it is envisaged in many unification theories. If we consider the tangent plane to this hyper-surface in any point M, it is possible to define in this plane a pseudo-orthonormal reference frame, and in fact an infinite set of similar frames deduced from each other by a Lorentz transformation or a rotation; in the close vicinity of M the laws of physics are invariant under the Lorentz group. We can in another point ![]() of the hyper-surface
of the hyper-surface ![]() define a similar set of pseudo-orthonormal frames. The question is: what is the correspondence between the 2 sets of reference frames attached to M and
define a similar set of pseudo-orthonormal frames. The question is: what is the correspondence between the 2 sets of reference frames attached to M and![]() ?
?
Let us first perform on the surface an infinitesimal displacement ![]() from M to
from M to![]() ; in this close vicinity of M the surface is assimilated to its tangent plane and
; in this close vicinity of M the surface is assimilated to its tangent plane and ![]() is an infinitesimal translation. Then we perform a transformation
is an infinitesimal translation. Then we perform a transformation ![]() around
around![]() . If
. If ![]() is a constant, the coordinates of
is a constant, the coordinates of ![]() are
are
![]() (8)
(8)
But if ![]() is a function of the point M, that expression becomes:
is a function of the point M, that expression becomes:
![]() (9)
(9)
Comparing the two expressions Equation (8) and Equation (9) above, we see that ![]() is transformed into
is transformed into
![]() (10)
(10)
with
![]() (11)
(11)
Now considering some function![]() , we deduce that
, we deduce that
![]() (12)
(12)
is in a similar way transformed into
![]() (13)
(13)
i.e. ![]() is replaced by
is replaced by
![]() (14)
(14)
The impulsion
![]() (15)
(15)
is the infinitesimal generator of space-time translations. Equation (14) above means that ![]() is replaced by
is replaced by
![]() (16)
(16)
The orbital angular momentum anti-symmetric tensor
![]() (17)
(17)
can be written as
![]() (18)
(18)
where ![]() denotes a rotation angle in the
denotes a rotation angle in the ![]() plan (N.B.: the 3-dimension vector components
plan (N.B.: the 3-dimension vector components ![]() and
and ![]() also satisfy the commutation relations above). Since
also satisfy the commutation relations above). Since![]() , then
, then
![]() (19a)
(19a)
can be written as
![]() (19b)
(19b)
That expression allows to evidence a gauge invariance property: it is possible to add to ![]() any function
any function ![]() without changing
without changing![]() : if
: if ![]() only depends on the invariant quantity
only depends on the invariant quantity![]() , its derivation with respect to
, its derivation with respect to ![]() is just 0. As a consequence, there is some flexibility in the determination of the
is just 0. As a consequence, there is some flexibility in the determination of the ![]() s and we can impose the 4 gauge conditions
s and we can impose the 4 gauge conditions
![]() (20)
(20)
We have here above considered the transformations of a local set of pseudo-orthonormal coordinates and not the geometry of the 4-dimension hyper-surface described by a set of curvilinear coordinates. This is the major difference with other presentations of relativistic gravitation and notably with general relativity but that difference is purely formal. As a consequence, many mathematical expressions look simpler; for example, the inva-
riant 4-dimension volume element ![]() that appears in the expression of the action integral
that appears in the expression of the action integral ![]() in the Lagrange formalism is merely
in the Lagrange formalism is merely![]() , i.e. the determinant of the metric tensor is 1.
, i.e. the determinant of the metric tensor is 1.
3. Gravitation Field Equations
We now consider a scalar particle of mass m (but the procedure can be straightforwardly generalized to a particle of any spin, be it massive or not) and the Lagrangian density
![]() (21a)
(21a)
with
![]() (21b)
(21b)
We perform the transformation ![]() so that the Lagrangian density becomes
so that the Lagrangian density becomes
![]() (22)
(22)
where we have introduced the effective metrics
![]() (23a)
(23a)
with
![]() (23b)
(23b)
We have written ![]() and
and ![]() with gothic letters instead of the usual
with gothic letters instead of the usual ![]() and
and ![]() in order to remind ourselves that we have considered a local set of pseudo Cartesian coordinates and not a set of curvilinear coordinates running over the whole surface.
in order to remind ourselves that we have considered a local set of pseudo Cartesian coordinates and not a set of curvilinear coordinates running over the whole surface.
Applying the Lagrange equations to the ![]() field, i.e.
field, i.e.
![]() (24)
(24)
gives
![]() (25)
(25)
From the expression of ![]() as a function of the
as a function of the ![]() s it is clear that
s it is clear that![]() ;
; ![]() has thus 10 components but from the gauge conditions
has thus 10 components but from the gauge conditions ![]() we derive
we derive
![]() (26)
(26)
Equation (26) shows that actually ![]() has only 6 independent components. The cross-term in Equation (25) vanishes, leading to
has only 6 independent components. The cross-term in Equation (25) vanishes, leading to
![]() (27)
(27)
The wave equation so appears as the wave equation of a free particle in which the original Minkowski metrics ![]() has been replaced by the effective metrics
has been replaced by the effective metrics![]() .
.
The gravitation field is thus described by a modification of the geometry of space-time by replacing the Minkowski metrics ![]() by the effective metrics
by the effective metrics![]() . A consequence of the modified wave equation above is that the dynamics of any particle, with or without mass, is affected by a gravitation field.
. A consequence of the modified wave equation above is that the dynamics of any particle, with or without mass, is affected by a gravitation field.
We now assume for the gravitation field itself a Lagrangian density term quadratic in ![]() and in
and in![]() . The scalar product of two vectors
. The scalar product of two vectors ![]() and
and ![]() is
is![]() , this can be extended to the scalar product of two tensors of any rank and to the metric tensor itself. So for the term quadratic in
, this can be extended to the scalar product of two tensors of any rank and to the metric tensor itself. So for the term quadratic in ![]() we assume the expression
we assume the expression
![]() (28)
(28)
![]() is the mass of the gravitation field; now
is the mass of the gravitation field; now ![]() so that this term is a constant we can hereunder discard (in fact we will see later on that formally maintaining that term in the calculation would imply
so that this term is a constant we can hereunder discard (in fact we will see later on that formally maintaining that term in the calculation would imply![]() ). In a similar way we assume for the term quadratic in
). In a similar way we assume for the term quadratic in ![]() the following expression
the following expression
![]() (29a)
(29a)
or
![]() (29b)
(29b)
[N.B.: as![]() , considering the infinitesimal variations
, considering the infinitesimal variations ![]() and
and![]() , and neglecting the second order terms gives
, and neglecting the second order terms gives![]() ; it then may be checked that the additional terms that could appear in developing the derivatives of Equation (29b) actually cancel each other].
; it then may be checked that the additional terms that could appear in developing the derivatives of Equation (29b) actually cancel each other].
The full Lagrangian density of the (field + particle) system is
![]() (30)
(30)
where ![]() is a dimensionless constant; the negative sign and the negative exponent are for commodity reasons.
is a dimensionless constant; the negative sign and the negative exponent are for commodity reasons.
Applying the Lagrange equations to![]() , i.e.
, i.e.
![]() (31)
(31)
with ![]() leads to the field equations (with the same remarks as in the N.B. here above):
leads to the field equations (with the same remarks as in the N.B. here above):
![]() (32)
(32)
These are the equations of the gravitation field and their nonlinear character is obvious. The term on the right- hand side
![]() (33)
(33)
is proportional to the energy-impulsion density tensor of the particle; it is the source term of the gravitation field. In the classical, i.e. non quantum, limit, the correspondence ![]() together with the expression of the density
together with the expression of the density![]() , changes this term into
, changes this term into
![]() (34)
(34)
So Equation (32) becomes
![]() (35)
(35)
For the sake of commodity, we will re-write it in a different, more workable way. Let us introduce the two quantities
![]() (36a)
(36a)
![]() (36b)
(36b)
Combining Equations (35) and (36) we finally get after some manipulations
![]() (37)
(37)
4. Non Relativistic Limit
In the case of a weak gravitation field, the quadratic terms in the field equations can be neglected. In the absence of matter, the linearized equations take the form of propagation like equations:
![]() (38)
(38)
If matter is present, there is a source term:
![]() (39a)
(39a)
with
![]() (39b)
(39b)
i.e.
![]() (40a)
(40a)
![]() (40b)
(40b)
![]() (40c)
(40c)
![]() (40d)
(40d)
In the non-relativistic limit ![]() and hence we get
and hence we get
![]() (41a)
(41a)
hence
![]() (41b)
(41b)
Moreover if the field is slowly varying with time, the time derivatives on the left hand side of Equation (40) vanish and those equations become:
![]() (42a)
(42a)
![]() (42b)
(42b)
![]() (42c)
(42c)
![]() (42d)
(42d)
The above expression for ![]() has to be compared with the expression of the classical gravitation potential
has to be compared with the expression of the classical gravitation potential![]() :
:
![]() (43)
(43)
where ![]() is the gravitation constant. It means that in the non-relativistic limit
is the gravitation constant. It means that in the non-relativistic limit ![]() is proportional to the Newtonian potential. The constant
is proportional to the Newtonian potential. The constant ![]() will be hereunder determined by the requirement of compatibility with Newton’s equation of motion.
will be hereunder determined by the requirement of compatibility with Newton’s equation of motion.
On the other hand, the dynamical equation of a massive particle is given by Equation (27) ![]() which in the classical limit becomes
which in the classical limit becomes
![]() (44a)
(44a)
or
![]() (44b)
(44b)
Let us put
![]() (45)
(45)
so that Equation (44b) becomes
![]() (46a)
(46a)
or
![]() (46b)
(46b)
Let us also put
![]() (47)
(47)
We proceed by successive iterations; replacing ![]() by
by ![]() in the right hand side of Equation (46b) gives
in the right hand side of Equation (46b) gives
![]() (48a)
(48a)
or
![]() (48b)
(48b)
In the non-relativistic limit ![]() so that Equation (48b) approximately becomes
so that Equation (48b) approximately becomes
![]() (49)
(49)
Moreover in the weak field limit ![]() so that from Equation (49) we finally derive
so that from Equation (49) we finally derive
![]() (50)
(50)
If we compare the above expression with the non-relativistic expression
![]() (51)
(51)
we see that the particle is undergoing an effective gravitation potential
![]() (52a)
(52a)
Since ![]() this is equivalent to
this is equivalent to
![]() (52b)
(52b)
Since from Equation (42a) ![]() the above expression of
the above expression of ![]() yields
yields
![]() (53)
(53)
By comparison with the expression of the classical gravitation potential ![]() we get
we get
![]() (54)
(54)
If we had retained the ![]() term in the expression Equation (30) of the Lagrangian density of the gravitation field, we would have in Equation (52b) an additional constant term; the comparison with the classical gravitation potential implies
term in the expression Equation (30) of the Lagrangian density of the gravitation field, we would have in Equation (52b) an additional constant term; the comparison with the classical gravitation potential implies![]() . The field equation Equation (37) now writes as
. The field equation Equation (37) now writes as
![]() (55)
(55)
5. Post-Newtonian Terms
From the expressions Equation (42) we derive in the weak, slowly varying field limit
![]() (56a)
(56a)
![]() (56b)
(56b)
![]() (56c)
(56c)
![]() (56d)
(56d)
so that
![]() (57)
(57)
In addition, Equation (48b) gives
![]() (58)
(58)
This has to be compared with the Newtonian expression at the lowest order in ![]() and
and ![]()
![]() (59a)
(59a)
or
![]() (59b)
(59b)
6. Conclusions
Introducing the Planck length ![]() and the Planck mass
and the Planck mass ![]() leads to the following expression for the full Lagrangian density of the (field + particle) system
leads to the following expression for the full Lagrangian density of the (field + particle) system
![]() (60)
(60)
![]() . According to that expression, the physical objects such as
. According to that expression, the physical objects such as ![]() have their dynamics mainly driven by the forces other than gravitation and the effective space-time metrics
have their dynamics mainly driven by the forces other than gravitation and the effective space-time metrics ![]() is the metrics generated by the external masses. For the objects such as
is the metrics generated by the external masses. For the objects such as![]() , gravitation is a major driver of their dynamics, and they generate the gravitation field as well as they undergo it.
, gravitation is a major driver of their dynamics, and they generate the gravitation field as well as they undergo it.
The usual approach of general relativity is most adequate for describing the universe on a large scale in astrophysics and cosmology. The approach of this paper, derived from particle physics and focused on local reference frames, underlines the formal similarity between gravitation and the other interactions inasmuch as they are associated to the restriction of a global symmetry to a local one.
In a 10-dimension space-time as it is considered in certain unification theories, gravitation is linked to the geometry of the 4 usual dimensions whereas the other fundamental interactions can be associated to the geometry of the 6 additional ones; in that approach extra fields (which eventually may account for dark matter and/or dark energy) naturally come out by regarding the geometry of the full 10-dimension set.