1. Introduction
We confine our discussion to the following situation.
Let 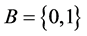 be a binary alphabet and
be a binary alphabet and 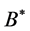 be the set of all words with finite length in the alphabet,
be the set of all words with finite length in the alphabet,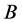 . We take the dictionary function as the following partial mapping:
. We take the dictionary function as the following partial mapping:
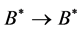 .
.
Saying a communication channel, we mean an arbitrary multi-valued mapping, having the following form:
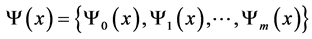 , (1)
, (1)
where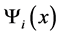 ,
, 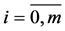 is some dictionary function.
is some dictionary function.
As to the content, equality (1) means that when the word 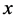 is transferred we have one of the words
is transferred we have one of the words 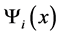 at the exit.
at the exit.
Below we take  without any loss of generality.
without any loss of generality.
We denote the set of all binary words with the length 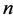 by
by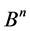 ; below the terms, “a word”and “a Boolean vector”are synonyms.
; below the terms, “a word”and “a Boolean vector”are synonyms.
Example.
1) Mapping (1) is called a standard communication channel if it has a limited number of distortions of the form: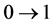 ,
, 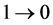 , where
, where
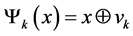 .
.
Besides, we say that there are no more than 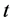 errors in the channel if
errors in the channel if ![]() does not exceed
does not exceed![]() . (Here
. (Here ![]() is Hamming weight of the word
is Hamming weight of the word![]() ). On the other hand, the following holds:
). On the other hand, the following holds:
![]() ,
,
where ![]() is the cardinality of the sphere with the radius
is the cardinality of the sphere with the radius ![]() in
in![]() .
.
The notion of the code that corrects the errors of the channel ![]() is completely analogous to the classic definition of the code, correcting the distortions of the form:
is completely analogous to the classic definition of the code, correcting the distortions of the form:![]() ,
,![]() .
.
Definition 1. The code ![]() corrects the errors of the channel:
corrects the errors of the channel:
![]() , if the following is valid:
, if the following is valid:
![]() (2)
(2)
for![]() ,
,![]() ;
;![]() ,
,![]() .
.
Condition (2) means that consequences of errors are different; hence we can restore one to one the initial information at the exit. The decision process at the exit usually is formalized in the form of the “decoding table” [1] :
![]()
Error “correction” through the table takes place as follows. According to definition, every “transferred” word ![]() is transformed by the channel
is transformed by the channel ![]() into
into![]() , which is at least in one of the columns of the table.
, which is at least in one of the columns of the table.
Then the code vector in the first row of any row is the “prototype” of the transferred word.
It is clear that if the word ![]() belongs to the only one of the columns in the table, then the “decoding” process leads to a right result.
belongs to the only one of the columns in the table, then the “decoding” process leads to a right result.
Condition (2) can be formulated in a little different way using the notion of “neighborhood” which gives certain advantage when making estimates of the cardinality of the correcting code.
The neighborhood of the ![]() th order of the word
th order of the word ![]() built up with respect to the set:
built up with respect to the set:
![]() ,
,
Is formed by the following induction:
![]() (3)
(3)
Condition (3) shows that the neighborhood of the ![]() th order of the word
th order of the word ![]() is the union of the neighborhood of the 1st order of all words belonging to the neighborhood of the
is the union of the neighborhood of the 1st order of all words belonging to the neighborhood of the ![]() th order of the word
th order of the word![]() .
.
In the term of the neighborhood condition (2) of error correction takes the following form:
![]() (4)
(4)
We denote by ![]() the code correcting the errors of the channel
the code correcting the errors of the channel![]() .
.
In the terms of the above introduced notions for the given channel ![]() the problem is to build the code of the maximum cardinality
the problem is to build the code of the maximum cardinality![]() .
.
It is obvious that this cardinality depends on the “structure” of![]() .
.
Among the codes ![]() the so called perfect codes are of special interest.
the so called perfect codes are of special interest.
Definition 2. The code ![]() is called perfect if:
is called perfect if:
![]()
Definition 3 [2] . The channel ![]() is called algebraic if:
is called algebraic if:
![]() , for all
, for all![]() .
.
Assume that for all![]() , that if
, that if![]() then
then![]()
We consider the graph![]() , adjacency of the vertices are defined as follows. The vertices
, adjacency of the vertices are defined as follows. The vertices ![]() are adjacent iff there exists a
are adjacent iff there exists a ![]() satisfying the condition
satisfying the condition ![]() or
or![]() . The distance on the graph
. The distance on the graph ![]() between any vertices
between any vertices ![]() is the minimum number of the arcs in the chain connecting the vertices
is the minimum number of the arcs in the chain connecting the vertices ![]() and the infinity if there does not exist such a chain.
and the infinity if there does not exist such a chain.
It is not difficult to prove the following condition. In the graph ![]() the distance between any two vertices from
the distance between any two vertices from ![]() no less than three it is necessary and sufficient that
no less than three it is necessary and sufficient that ![]() be an error correcting code of the algebraic channel
be an error correcting code of the algebraic channel![]() .
.
Further we discuss a special but having certain interest type of communication channel which is carried out by linear mappings,![]() .
.
2. Matrix Channels [3]
Let ![]() be a finite field of two elements and
be a finite field of two elements and ![]() be the set of matrices of the order
be the set of matrices of the order ![]() with the elements belonging to the field
with the elements belonging to the field ![]() with the usual operations of addition and multiplication for
with the usual operations of addition and multiplication for![]() . If
. If
![]() then the set
then the set![]() , defines a matrix channel in the sense of (1):
, defines a matrix channel in the sense of (1):
![]() .
.
Examples.
2) Let such “errors” take place in a “real” channel, which are connected with wrong reading of adjacent letters of the transferred vector,![]() ; and this means the following transformation:
; and this means the following transformation:
![]() .
.
This situation can be modelized by the matrix channel ![]() where
where ![]() is the unit matrix:
is the unit matrix:
![]()
Indeed, when transferring ![]() through the channel we have a vector of the following form at the exit:
through the channel we have a vector of the following form at the exit:
![]() ,
,
where![]() .
.
3) if a “drop-out” of symbols takes place in the channel, i.e. the length of the word is changed, then it can be presented in the matrix form as follows. Let ![]() is the initial word in which just one symbol can be lost. We discuss the following set of matrices belonging to
is the initial word in which just one symbol can be lost. We discuss the following set of matrices belonging to![]() :
:
![]()
Then:
![]() .
.
The notion of the code that corrects the errors of the matrix channel M is completely analogous to the classic definition of the code, correcting the distortions of the form:![]() ,
,![]() .
.
Definition 4. The code ![]() corrects the errors of the channel
corrects the errors of the channel
![]() if the following condition is valid:
if the following condition is valid:
![]()
for all![]() ,
,![]() ; and
; and![]() ,
,![]() .
.
The neighborhood of the ![]() th order of the word
th order of the word ![]() built with respect to the set
built with respect to the set![]() , is defined as in (3):
, is defined as in (3):
![]() .
.
In the terms of neighborhood the error correction condition becomes as follows:
![]() .
.
3. The Group Matrix Channels
Let ![]() be the group of the non-degenerated matrices of the order
be the group of the non-degenerated matrices of the order ![]() on the field
on the field ![]() and
and ![]() be the subgroup of
be the subgroup of![]() . We discuss the matrix channel generated by the subgroup:
. We discuss the matrix channel generated by the subgroup:
![]() ,
,
where ![]() is a divisor of the number:
is a divisor of the number:
![]()
We can consider that the group![]() , operates in the set
, operates in the set![]() , as follows:
, as follows:
![]() , where
, where![]() ,
,![]() .
.
Moreover, the transitive set:
![]() ,
,
coincides with the neighborhood of the first order of the point![]() , i.e.
, i.e.![]() .
.
These neighborhoods do not intersect and thus, form the partition![]() . Consequently, if we take an arbitrary representative from each transitive set, we will have a code, correcting the errors of the group channel,
. Consequently, if we take an arbitrary representative from each transitive set, we will have a code, correcting the errors of the group channel,![]() .
.
Lemma 1. For the group matrix channel![]() , any code containing one representative of all transitive sets, is a code with the maximum cardinality, correcting the errors of the channel
, any code containing one representative of all transitive sets, is a code with the maximum cardinality, correcting the errors of the channel![]() .
.
Proof. As it was mentioned, the code![]() , built as it was said above, corrects the errors of the matrix channel,
, built as it was said above, corrects the errors of the matrix channel,![]() . On the other hand, if some code
. On the other hand, if some code ![]() correcting the errors of the group channel
correcting the errors of the group channel ![]() contains more points that the number of the transitive sets then at least one of these transitive sets contains two points of
contains more points that the number of the transitive sets then at least one of these transitive sets contains two points of ![]() which contradicts condition (4). Q. E. D.
which contradicts condition (4). Q. E. D.
The above Lemma completely describes all the codes of the maximum cardinality, correcting the errors of the group channel![]() .
.
The cardinality of the neighborhood ![]() of an arbitrary point
of an arbitrary point ![]() can be calculated by thestabilizer of the same point or of the subgroup
can be calculated by thestabilizer of the same point or of the subgroup![]() :
:
![]() .
.
In other words, the following formula is valid:
![]() .
.
The cardinality of the code ![]() can be expressed by Burnside’s Lemma [4] [5] .
can be expressed by Burnside’s Lemma [4] [5] .
Let ![]() be the set of the motionless points of the transformation
be the set of the motionless points of the transformation ![]() or in another way (which is the same) let it be the set of the eigen vectors of the matrix
or in another way (which is the same) let it be the set of the eigen vectors of the matrix ![]() corresponding the eigen value
corresponding the eigen value![]() , that is, let it be the set of the solutions of the following equation:
, that is, let it be the set of the solutions of the following equation:
![]() .
.
Lemma (Burnside’s) 2. The following formula holds true:
![]() (5)
(5)
Examples.
4) Let ![]() be the transformation of the cyclic shift in
be the transformation of the cyclic shift in![]() :
:
![]() ,
,
and ![]() be the matrix, corresponding to this transformation:
be the matrix, corresponding to this transformation:
![]() .
.
We discuss the group matrix channel![]() . According to the definition, this channel operates as follows. If the word
. According to the definition, this channel operates as follows. If the word ![]() is put in then we get one of the cyclic shifts of this word at the exit. We call the positive integer
is put in then we get one of the cyclic shifts of this word at the exit. We call the positive integer ![]() the period of the word
the period of the word ![]() if
if ![]() is the smallest integer for which
is the smallest integer for which![]() . Then the neighborhood of the first order of the word
. Then the neighborhood of the first order of the word ![]() has the following form:
has the following form:
![]() .
.
It is clear that the first order neighborhoods carry out a partitioning of ![]() into classes of equivalence. If
into classes of equivalence. If ![]() is the number of the equivalence classes the elements of which have the period d then the following obviously holds:
is the number of the equivalence classes the elements of which have the period d then the following obviously holds:
![]() (6)
(6)
Let us note that the maximum cardinality code ![]() is any set of the representatives of the transitive sets and its cardinality is given by Formula (5) which has the following form for this case:
is any set of the representatives of the transitive sets and its cardinality is given by Formula (5) which has the following form for this case:
![]() (7)
(7)
Through the standard calculation technique, we get from (6) and (7) the well-known expression:
![]()
where ![]() is Euler’s function which gives the amount of the numbers less than
is Euler’s function which gives the amount of the numbers less than ![]() and which are coprime with respect to it.
and which are coprime with respect to it.
In particular, if ![]() is a prime number, then:
is a prime number, then:
![]() .
.
5) Let there be a communication channel through which the transmitted word:
![]() ,
,
is transformed into the binary word:
![]() ,
,
where either![]() , and
, and![]() , or
, or![]() , for
, for![]() .
.
Let us describe the physical meaning of this channel. Saying “transmittance of the word x through the communication channel” we understand successive transmittance of symbols or, as they say, transmittance of the pulses (signals)![]() , taking into account that the symbols with the odd indices
, taking into account that the symbols with the odd indices ![]() are transmitted without any distortion, and the rest of them:
are transmitted without any distortion, and the rest of them:![]() , can have distortions defined by the directly preceding symbols.
, can have distortions defined by the directly preceding symbols.
Thus, having the symbol ![]() at the exit, we can get either
at the exit, we can get either ![]() or
or![]() .
.
Now we give this description of the channel by the matrix “language”. Let we have the set of the matrices ![]() where
where ![]() is the unit matrix:
is the unit matrix:
![]()
We discuss the group matrix channel ![]() the constituent of which is the set
the constituent of which is the set![]() . As any matrix of
. As any matrix of ![]() coin-
coin-
cides with its inverse matrix in the group![]() , i.e.
, i.e.![]() , then
, then![]() ,
, ![]() , and
, and ![]() is consisted of all possible products of the matrices
is consisted of all possible products of the matrices ![]() of
of![]() ; therefore, the
; therefore, the
order of the group ![]() is
is![]() . It follows from the description of the channel
. It follows from the description of the channel ![]() that the code, correcting its errors, also corrects the errors of the channel with overlay. The converse proposition also is true.
that the code, correcting its errors, also corrects the errors of the channel with overlay. The converse proposition also is true.
Let us partition the group ![]() into the non-intersecting sets
into the non-intersecting sets![]() ,
, ![]() where
where ![]() and
and ![]() be the set of the matrices generated the products of any i different elements, belonging to M\{M0}, i.e. the matrix
be the set of the matrices generated the products of any i different elements, belonging to M\{M0}, i.e. the matrix ![]() if
if![]() , where
, where![]() ,
,![]() .
.
Talking figuratively if we enumerate the matrix rows of the group ![]() from the top to the bottom, then the set
from the top to the bottom, then the set![]() ,
, ![]() is consisted of all matrices having the dimension
is consisted of all matrices having the dimension ![]() and which have two units in their
and which have two units in their ![]() -th rows with odd numbers on their diagonal positions and immediately on the right, but in the rows numbered by
-th rows with odd numbers on their diagonal positions and immediately on the right, but in the rows numbered by ![]() the unit is only in a diagonal position. The other elements of the matrices are zero.
the unit is only in a diagonal position. The other elements of the matrices are zero.
It immediately follows from the definition of the set ![]() that
that![]() ,
, ![]() and for any matrix
and for any matrix ![]() the number of the motionless points of the transformation is:
the number of the motionless points of the transformation is:
![]()
As we have:
![]() ,
,
then it follows from Lemma 2 that for the maximum cardinality code ![]() the following holds true:
the following holds true:
![]()
Let us discuss Example 5 for![]() , i.e. that there is a word
, i.e. that there is a word ![]() which can be transformed into one of the words:
which can be transformed into one of the words:
![]() ,
,
when transmitted through the channel. For the given case the set of the matrices ![]() is the following:
is the following:
![]() .
.
The group channel ![]() having the set
having the set ![]() as its constituent is consisted of the following matrices:
as its constituent is consisted of the following matrices:
![]()
Let us find the set of the motionless points of the transformation for each element of the group![]() . As it was said above the set of the solutions of the equation:
. As it was said above the set of the solutions of the equation:
![]() (8)
(8)
corresponds to the set![]() ,
,![]() . For the matrix
. For the matrix ![]() Equation (8) is as follows:
Equation (8) is as follows:
![]() ,
,
and the set of solutions of it is the set![]() . Consequently,
. Consequently,![]() .
.
Then, from (8) for the cases:![]() ,
, ![]() ,
, ![]() , we find for
, we find for ![]() the respective sets of the solutions:
the respective sets of the solutions:
![]()
And, applying Lemma 2 we get the cardinality of the code![]() :
:
![]()
6) Let us discuss a little modified channel of Example 2. Namely, we take that when transmitting the vector ![]() some “transposition” errors of the following form take place:
some “transposition” errors of the following form take place:
![]() ,
,
taking into account that such inversions can take place in a few places.
In the terms of matrix channels the model is as follows. We have the set of the matrices![]() , where
, where ![]() is the unit matrix:
is the unit matrix:
![]() .
.
Considerations analogous in the preceding example let us establish the following facts. The matrix channel ![]() consisting of all possible products of the elements
consisting of all possible products of the elements ![]() of the set
of the set ![]() is a group one. Besides the order of the group
is a group one. Besides the order of the group ![]() is
is ![]() and the code, correcting the errors of the channel
and the code, correcting the errors of the channel ![]() also corrects the errors of the channel with the transpositions. Then, following the same logic and, using Formula (5) for the maximum cardinality code
also corrects the errors of the channel with the transpositions. Then, following the same logic and, using Formula (5) for the maximum cardinality code ![]() we get:
we get:
![]() .
.
4. The Metrics and Codes in the Additive Channel
Definition 5 (See [6] ). The arbitrary subset ![]() that carries out the function
that carries out the function ![]() , where
, where ![]() is called additive channel. Here
is called additive channel. Here![]() .
.
Definition 6 (See [7] ). The code ![]() corrects the errors of the additive channel
corrects the errors of the additive channel ![]() if
if ![]() where
where![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
,![]() .
.
As in the preceding section we define the neighborhood of ![]() -th order of the word
-th order of the word ![]() as follows:
as follows:
![]() .
.
NB 1. For the additive channel ![]() the following equality holts true:
the following equality holts true:
![]() ,
,
For any![]() ,
,![]()
Definition 7. (See [8] [9] ) The code ![]() correcting the errors of the additive channel
correcting the errors of the additive channel ![]() is called perfect if:
is called perfect if:
![]()
Note that the perfect code ![]() has maximum cardinality though the convers statement is not always valid.
has maximum cardinality though the convers statement is not always valid.
NB 2. Any word from ![]() belongs to the neighborhood of the first order of only one word of the perfect code
belongs to the neighborhood of the first order of only one word of the perfect code![]()
The standard and most used metric in code theory is Hamming’s metric [9] , i.e. the following function:
![]() .
.
It can be taken that this metric is connected with the “natural” basis ![]() in the following way:
in the following way:
![]() .
.
It is clear that if another basis is chosen, for instance, if ![]() is taken, then another metric will be generated:
is taken, then another metric will be generated:
![]() .
.
A more general procedure of metric generation shown above is as follows. For the given subset ![]() and the vector
and the vector ![]() we consider all “expansions” of
we consider all “expansions” of ![]() into
into![]() , i.e. the expression of the following form:
, i.e. the expression of the following form:
![]() (9)
(9)
and we put the following number:
![]() ,
,
into correspondence to (9).
Now choosing the least number of these we define the following norm ( [1] ; the MLM norm) connected with![]() :
:
![]() (10)
(10)
Lemma 3. The function ![]() is a metric (below, “MLM metric”) for any subset
is a metric (below, “MLM metric”) for any subset![]() .
.
In terms of graph theory the described situation is as follows. Let us give the following binary relation on the set of vertices![]() :
:
![]() for some
for some![]() .
.
This relation defines adjacency of vertices and we get a graph, i.e. the set of arcs![]() , which is given by the equality:
, which is given by the equality:![]() .
.
The distance among the vertices of this graph is given in the standard way: the minimum number of the arcs in the chain connecting these vertices; and the infinity if there is not such a chain.
Example.
7) If ![]() then the MLM norm has the following form:
then the MLM norm has the following form:
![]() .
.
In particular, for ![]() the MLM norms of the vectors in
the MLM norms of the vectors in ![]() are as follow:
are as follow:
![]()
If ![]() is an arbitrary additive channel then the set
is an arbitrary additive channel then the set ![]() generates an MLM norm in
generates an MLM norm in ![]() given by Formula (10). The statement presented below shows that the ability of the code
given by Formula (10). The statement presented below shows that the ability of the code ![]() to correct the errors of the additive channel
to correct the errors of the additive channel ![]() can be formulated in terms of the MLM norm generated by the set.
can be formulated in terms of the MLM norm generated by the set.
Lemma 4. The code ![]() corrects the errors of the additive channel
corrects the errors of the additive channel ![]() iff the following conditions hold:
iff the following conditions hold:
![]() , for
, for![]() .
.
Proof: If![]() , then according to definition:
, then according to definition:
![]() ,
,
or in another way:
![]() (11)
(11)
But it follows from (11) that the code ![]() does not correct the errors of the additive channel
does not correct the errors of the additive channel![]() .
.
And if![]() , then:
, then:
![]() ,
,
where![]() . But the equality:
. But the equality:
![]() ,
,
is impossible; hence, the code ![]() corrects the errors of the additive channel
corrects the errors of the additive channel![]() .
.
Let us discuss an arbitrary basis ![]() of the space
of the space ![]() and the linear reversible transformation
and the linear reversible transformation ![]() defined in the following way:
defined in the following way:
The image of any set ![]() is denoted by
is denoted by![]()
![]() , and
, and![]() ,
,
and the spectra ![]() and
and![]() , have no any simple connec-
, have no any simple connec-
tion. The situation will be changed to an extent if we consider different MLM norms and introduce limitations on the subject transformations.
Definition 8. The MLM metric ![]() is called a basis one if
is called a basis one if ![]() is a basis.
is a basis.
Lemma 5. For the basis MLM metrics ![]() and
and ![]() the following relations hold true:
the following relations hold true:
![]() (12)
(12)
where![]() ,
,![]() .
.
For the given MLM metric all the standard definitions of the correcting code theory can be modified replacing Hamming’s metric by any basis MLM metric. In particular, the perfect code ![]() with the distance
with the distance ![]() is a partition of the set
is a partition of the set ![]() in the union of the spheres of the radius
in the union of the spheres of the radius ![]() in the MLM metric. According to (12) the perfect codes in one metric are transformed into perfect codes in another metric. Besides Formulas (12) allow various interpretations of geometrical character. We present two facts which we use further.
in the MLM metric. According to (12) the perfect codes in one metric are transformed into perfect codes in another metric. Besides Formulas (12) allow various interpretations of geometrical character. We present two facts which we use further.
Lemma 6.
a) The subset, ![]() with the metric
with the metric ![]() and the subset
and the subset ![]() with the basis metric
with the basis metric ![]() simultaneously are spheres with the radius
simultaneously are spheres with the radius![]() .
.
b) The code ![]() with the basis metric
with the basis metric ![]() and the code
and the code ![]() with the basis metric
with the basis metric ![]() simultaneously are perfect with the distance
simultaneously are perfect with the distance![]() .
.
Example.
9) We discuss in ![]() the perfect code in Hamming’s metric
the perfect code in Hamming’s metric![]() , with the distance
, with the distance![]() . When choosing the basis
. When choosing the basis ![]() the image of this perfect code in the transformation
the image of this perfect code in the transformation ![]() is the following code
is the following code ![]() where
where ![]() is the matrix with the following vectors of the set
is the matrix with the following vectors of the set ![]() as its rows:
as its rows:
![]() .
.
Then the spheres with the unit radius having their centres at the points of the code ![]() have the following forms:
have the following forms:
![]() ,
,
![]() .
.
(See example 7). Thus ![]() is a perfect code with the distance 3 in the MLM metric:
is a perfect code with the distance 3 in the MLM metric:
![]() .
.
Though the metrics with different bases can strongly differ the spectrum of distances of the space ![]() is always the same.
is always the same.
Lemma 7. Let ![]() be the number of the points from
be the number of the points from ![]() with
with![]() . Then
. Then![]() ,
, ![]() for an arbitrary basis
for an arbitrary basis![]() .
.
Proof. According to the definition, ![]() is the number of the vectors of the basis
is the number of the vectors of the basis ![]() included into the expansion of the vector x:
included into the expansion of the vector x:
![]() .
.
Therefore, the number of the vectors ![]() with
with ![]() is equal to the number of the solutions of the following equation:
is equal to the number of the solutions of the following equation:
![]() ,
,
where![]() , i.e. it is equal to
, i.e. it is equal to![]() .
.
NB 3. If the basis ![]() is chosen as in Example 7 then the preceding statement is equivalent to the following formula:
is chosen as in Example 7 then the preceding statement is equivalent to the following formula:
![]() .
.
The preceding statements make possible to build the perfect codes in ![]() for arbitrary basis metrics if one such code is already built for one basis metric at least. In particular, if
for arbitrary basis metrics if one such code is already built for one basis metric at least. In particular, if ![]() is the basis for the subspace
is the basis for the subspace ![]() then the following statement holds true:
then the following statement holds true:
Theorem 1. The perfect codes in![]() , correcting the errors of the additive channel
, correcting the errors of the additive channel ![]() for
for![]() , exist only for the following values of m and
, exist only for the following values of m and![]() :
:
a)![]() ,
,
b)![]() .
.
Proof. We discuss the basis ![]() of the space
of the space ![]() where
where![]() ,
, ![]() and the linear reversible transformation
and the linear reversible transformation![]() , is defined above.
, is defined above.
Necessity. Let ![]() be a perfect code in
be a perfect code in ![]() with the basis metric
with the basis metric![]() .
.
It follows from Lemma 6 that the code ![]() also is perfect in
also is perfect in ![]() with the basis metric
with the basis metric![]() , correcting the errors of the channel
, correcting the errors of the channel![]() Then as
Then as ![]() and for
and for ![]() holds the following:
holds the following:![]() ,
, ![]() and we have:
and we have:
![]()
Taking this and NB 1 and 2 into account we see that the code:
![]() ,
,
is perfect in ![]() and it corrects the errors of the channel:
and it corrects the errors of the channel:
![]() ,
,
with the basis metric![]() . It is possible only for
. It is possible only for![]()
![]() or
or![]() ,
, ![]() [9] .
[9] .
Sufficiency. We discuss the perfect code ![]() where
where ![]() for
for![]() ,
, ![]() (Hamming’s code) or
(Hamming’s code) or![]() ,
, ![]() (Gallаy’s code) [10] .
(Gallаy’s code) [10] .
Now, as for any ![]() the following inequality holds true:
the following inequality holds true:
![]() ,
,
then it follows from Lemma 4 that the code ![]() corrects the errors of the channel:
corrects the errors of the channel:
![]()
On the other hand, we have:
![]() ,
,
i.e. ![]() is perfect in
is perfect in ![]() with the basis metric
with the basis metric ![]() and consequently (according to Lemma 6 the code
and consequently (according to Lemma 6 the code ![]() is perfect in
is perfect in ![]() with the basis metric
with the basis metric![]() . Q. E. D.
. Q. E. D.
5. The Upper and Lower Limits of the Cardinality of the Matrix Channel
The case of an arbitrary cannel does not make possible to obtain simple solutions for the code cardinality and even to obtain some universal Hamming and Varshamov-Gilbert type boundaries and requires some special restrictions on the structure for the matrix channel![]() .
.
Let the channel ![]() is algebraic, i.e. all the matrices in
is algebraic, i.e. all the matrices in ![]() are reversible and belong to
are reversible and belong to ![]() with their reverse ones. We introduce two parameters connected with
with their reverse ones. We introduce two parameters connected with![]() . Let:
. Let:
![]() ,
,
![]() .
.
Lemma 8. The following inequalities hold true:
![]() (13)
(13)
here ![]() is a matrix algebraic channel.
is a matrix algebraic channel.
We consider the matrix channel ![]() from Example 2. This channel exchanges the places of two neighboring letters in the word
from Example 2. This channel exchanges the places of two neighboring letters in the word![]() .
.
Let ![]() where
where![]() ,
, ![]() ,
, ![]() for
for![]() . We denote the number of the sequences of the word
. We denote the number of the sequences of the word![]() , by
, by![]() . Then
. Then ![]() and
and![]() .
.
If ![]() then
then![]() . Consequently:
. Consequently:
![]() .
.
As ![]() is the neighborhood of the first order of the word
is the neighborhood of the first order of the word ![]() then all the words obtained from
then all the words obtained from ![]() by transposition of the neighboring letter are included into
by transposition of the neighboring letter are included into![]() . Every one of these words has no more than
. Every one of these words has no more than ![]() sequences and the number of such words exactly equals to
sequences and the number of such words exactly equals to![]() . It follows from this that:
. It follows from this that:
![]() ,
,
and, according to Lemma 8 universal limitations (13) have the form:
![]() .
.
Roughness of these limits is connected with the great generality of the above considerations.
Below we present more accurate limits, taking the specification of the matrix channel ![]() into account.
into account.
6. The Upper Limit for ![]()
We partition ![]() into the two subsets
into the two subsets ![]() and
and ![]() such that the first one includes the words with their sequence number not exceeding
such that the first one includes the words with their sequence number not exceeding ![]() and the second one includes those exceeding
and the second one includes those exceeding![]() . Then we have:
. Then we have:
![]()
and
![]() (14)
(14)
The first inequality follows from the fact that the number of the words in ![]() having exactly
having exactly ![]() sequences
sequences
equals to ![]() see [2] ), and the second one results in the fact that the cardinality of the neighborhood of
see [2] ), and the second one results in the fact that the cardinality of the neighborhood of
the first order of the word ![]() equals to the number of the sequences of
equals to the number of the sequences of![]() . We choose the parameter
. We choose the parameter ![]() such that to minimize the upper limit
such that to minimize the upper limit![]() . Then we have from (14) for
. Then we have from (14) for
![]() .
.
Choosing ![]() and applying Sterling’s formula for
and applying Sterling’s formula for ![]() [2] :
[2] :
![]()
we get:
![]() .
.
7. The Lower Limit for ![]()
We discuss the following additive channel ![]() This channel corresponds to that
This channel corresponds to that
essential situation when the errors rise in pairs and in neighboring places. The connection of this channel with the matrix channel ![]() is explained by the following statement.
is explained by the following statement.
Lemma 9. Every code that corrects the errors of the additive channel ![]() also corrects the errors of the matrix channel
also corrects the errors of the matrix channel![]() .
.
Proof: We assume that the code ![]() corrects the errors of the channel:
corrects the errors of the channel:
![]() ,
,
and that there exist the matrices:
![]()
such that for some![]() ,
, ![]() takes place the equality:
takes place the equality:
![]() .
.
Hence we have:
![]() ,
,
where![]() ,
,![]() . Then taking into account that
. Then taking into account that ![]() we get:
we get:
![]() or
or![]()
Consequently, the following variants are possible:
a) ![]() and
and![]() .
.
Then the following equality takes place:
![]() ,
,
which is a contradiction.
If:
b) ![]() and
and![]() ,
,
c) ![]() and
and![]() ,
,
then the following equalities take place, respectively:
![]()
which also contradict the conditions of the Lemma. Q. E. D..
It follows from Lemma 9 that the maximum cardinality of the code, correcting the errors of the channel ![]() is
is
the lower estimation for the cardinality![]() , i.e.
, i.e.![]() . Taking into account Theorem 1 we get the lower estimation of
. Taking into account Theorem 1 we get the lower estimation of ![]() for
for![]() :
:
![]()