Some Hermite-Hadamard Type Inequalities for Differentiable Co-Ordinated Convex Functions and Applications ()
1. Introduction
Throughout this paper, let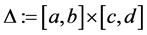 
be double intervals with
, 
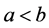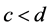in
, and a partial derivative of second order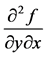 
is denoted by 
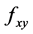for brevity.
The inequality
(1)
which holds for all convex functions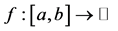 
is known as Hermite-Hadamard’s inequality [1] or simply Hadamard’s inequality.
For some results which generalize, improve, and extend the Inequality (1), please refer to [2] -[17] .
Based on the convex functions on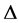
, Dragomir proposed the concept of co-ordinated convex functions in [3] , defined as follows:
Definition 1. A function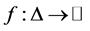 
is said to be convex on the co-ordinates on 
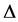if the partial mappings
are convex.
Definition 2. A function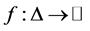 
is said to be convex on the co-ordinates on 
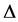if the inequality
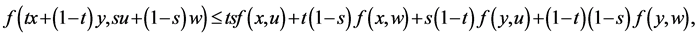(2)
holds for all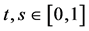 
and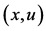
, 
, 
,
.
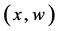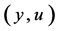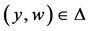Clearly, we can observe that every convex function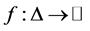 
is convex on the co-ordinates, but in some special cases, some co-ordinated convex functions are not convex (please refer to [3] ). For more relevant coordinated convex functions, please refer to [5] [6] [8] -[10] [12] .
The following extended Hadamard’s inequality for co-ordinated convex functions on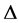 
in two variables was proved in [3] :
Theorem 1. Suppose that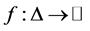 
is co-ordinated convex on
. Then the following inequalities hold:
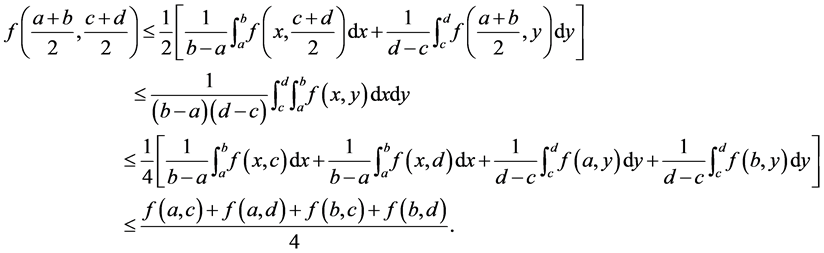(3)
The above inequalities are sharp.
In [10] , Latif and Dragomir established the following Hadamard-type inequalities that gave an estimate of the difference in the left side of the Inequalities (3) for differentiable co-ordinated convex functions on
.
Theorem 2. Let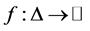 
be a partial differentiable mapping on
.
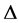(1) If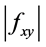 
is convex on the co-ordinates on
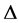, then the following inequality holds:
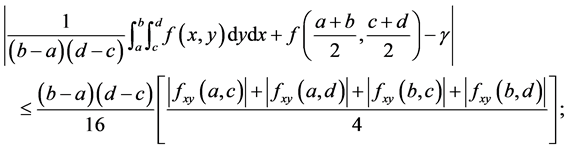(4)
(2) If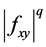 
is convex on the co-ordinates on 
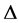and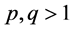
, 
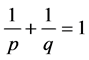, then the following inequality holds:
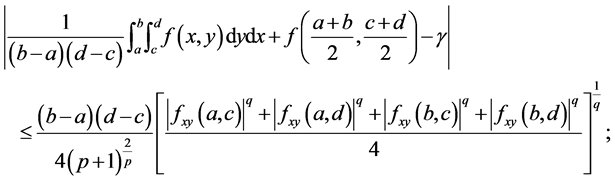(5)
(3) If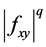 
is convex on the co-ordinates on 
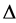and
, then the following inequality holds:
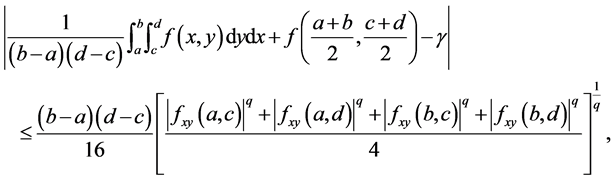(6)
where
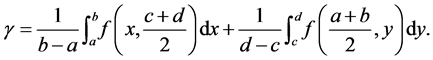
Remark 1. The Inequality (6) shows the result of giving the Inequality (5) an improved and simplified constant.
In [12] , Sarikaya et al. established the following results that gave an estimate of the difference in the right side of the Inequalities (3) for differentiable co-ordinated convex functions on
.
Theorem 3. Let 
be a partial differentiable mapping on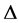
.
(1) If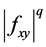 
is convex on the co-ordinates on
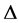, then the following inequality holds:
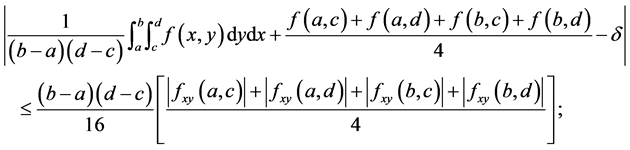(7)
(2) If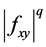 
is convex on the co-ordinates on 
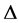and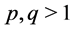
, 
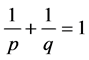, then the following inequality holds:
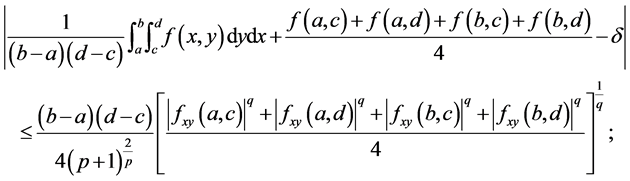(8)
(3) If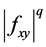 
is convex on the co-ordinates on 
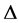and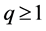
, then the following inequality holds:
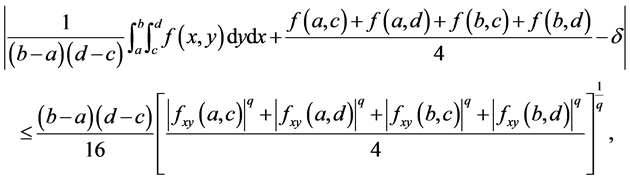(9)
where
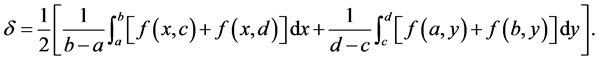
Remark 2. The Inequality (9) shows the result of giving the Inequality (8) an improved and simplified constant.
The goal of this paper is to establish an inequality which could be connected with the left side and right side of the extended Hadamard’s Inequality (3) and improve and generalize the Theorem 2 and Theorem 3. Also, the paper aims to note some consequent applications to special means.
In order to show our main results, we need the following identities (I)-(VI):
(I) For
, 
, the following four identities hold:
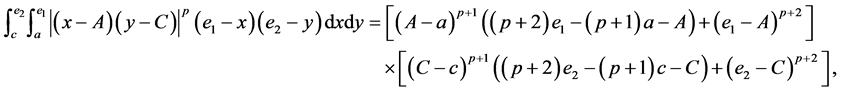
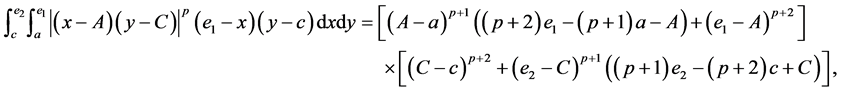
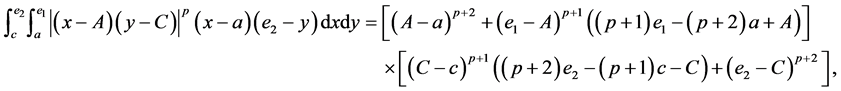
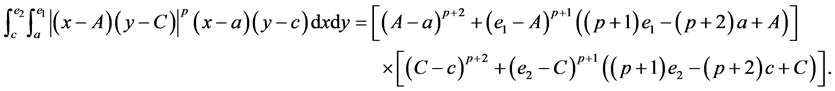
(II) For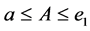
, 
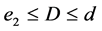, the following four identities hold:
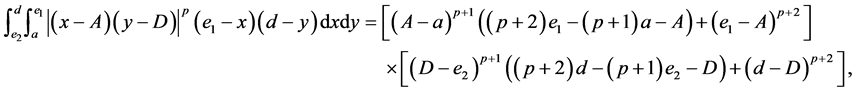
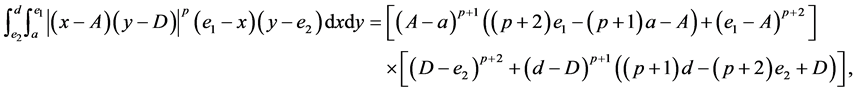
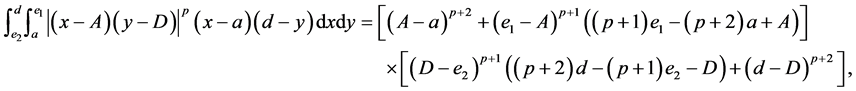
(III) For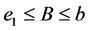
, 
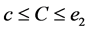, the following four identities hold:
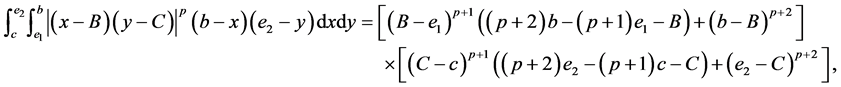
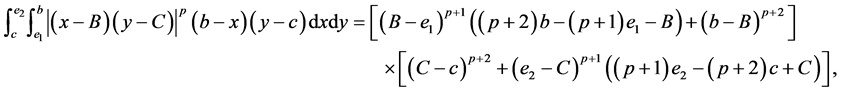
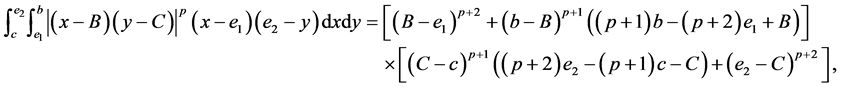
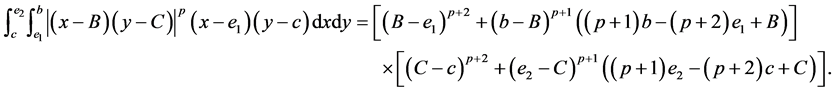
(IV) For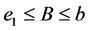
, 
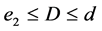, the following four identities hold:
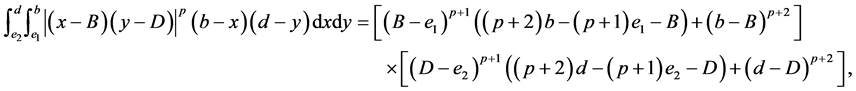
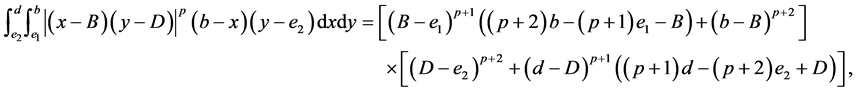
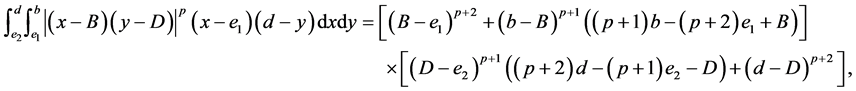
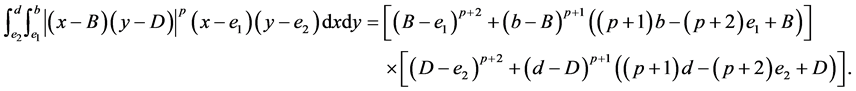
2. Main Results
In this section, let the mapping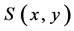 
for all 
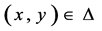be defined as follows:
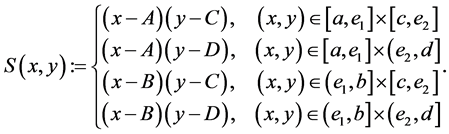(10)
In order to prove our main results, we need the following lemma:
Lemma 1. Let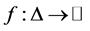 
be a partial differentiable mapping on
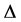. Then the following inequality holds:
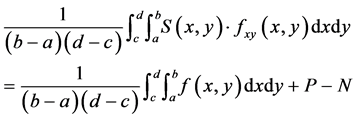(11)
where
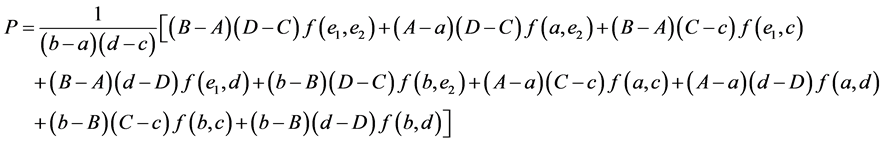
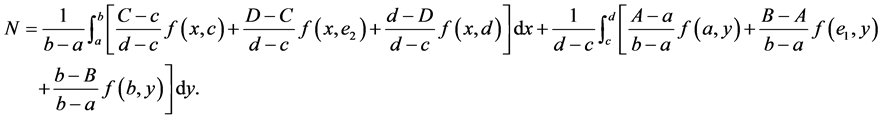
Proof. It suffices to note that
(12)
Integration by parts, we have
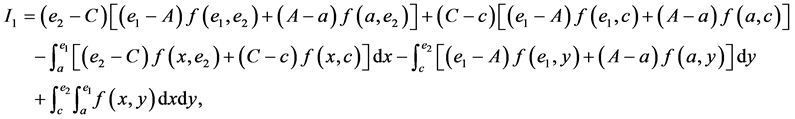
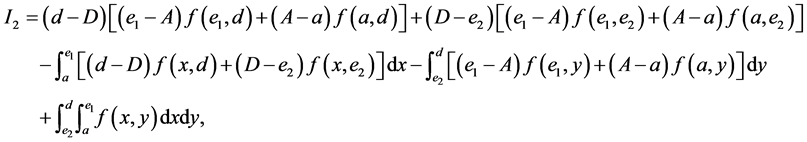
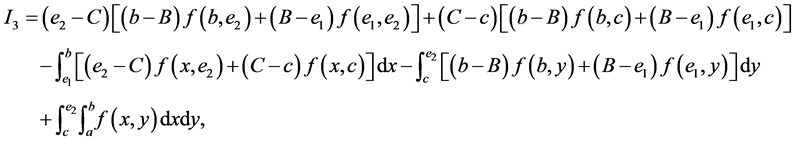
and
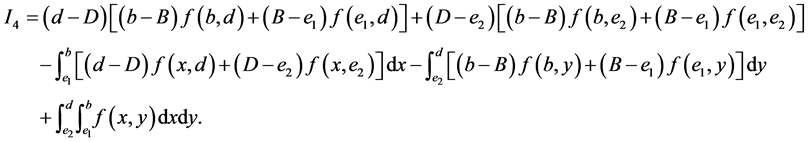
By summing the above four identities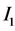
, 
, 
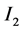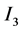and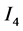 
and simplifying the result, it follows that
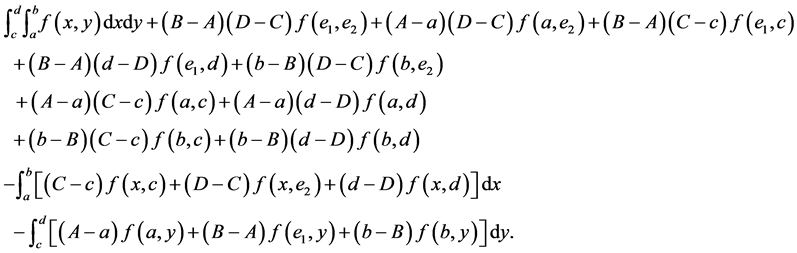(13)
Then, multiply both sides by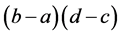 
in (12). From (12) and (13), we get the equations 
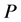and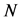
. This proof of the identity 11 is complete.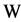 
Now, we are ready to state and prove the main results.
Theorem 1. Let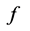 
be defined as Lemma 1. If 
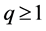and the mapping 
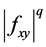is convex on the co-ordinates on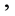 
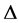then
(14)
where
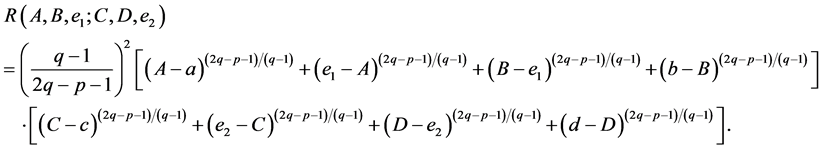
and
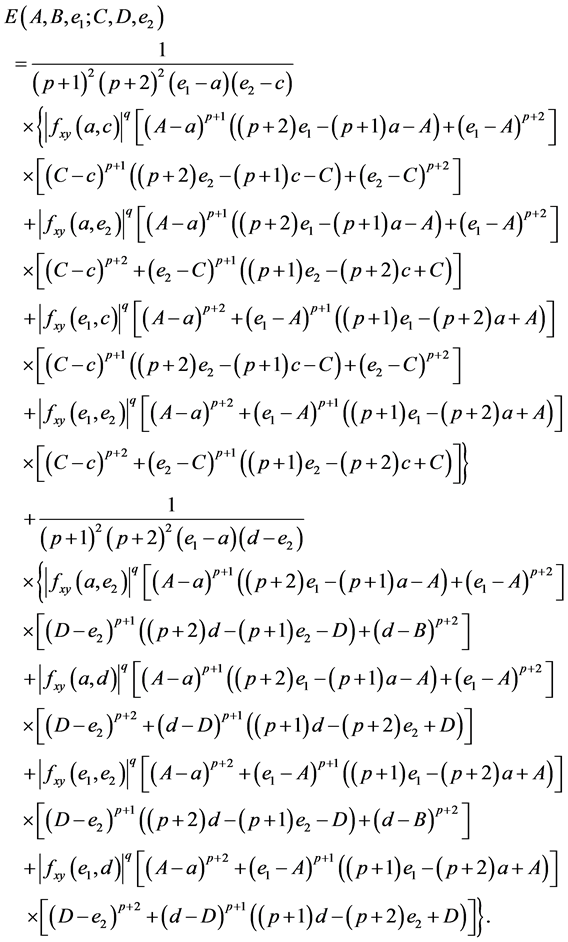
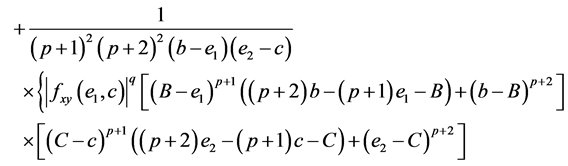
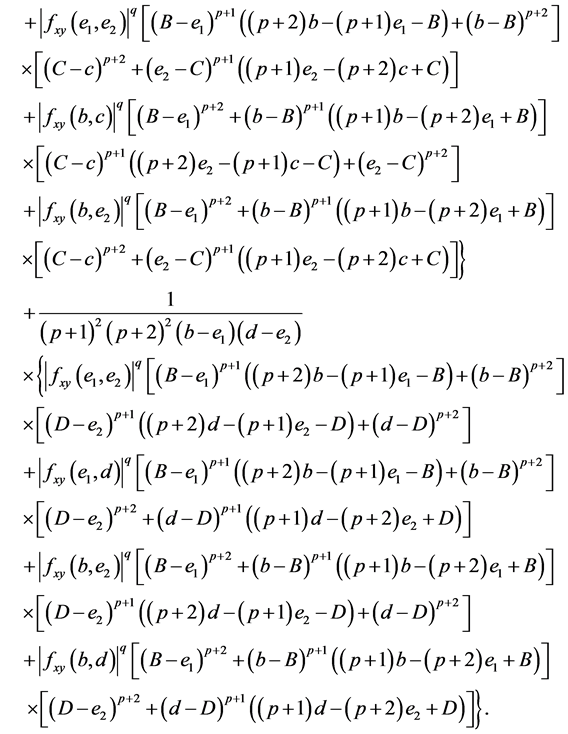
Proof. By using the identity (11), we have
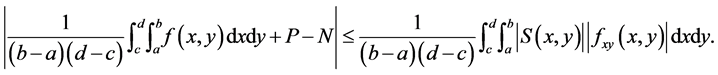
If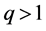
, 
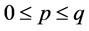, it follows from the power mean inequality that
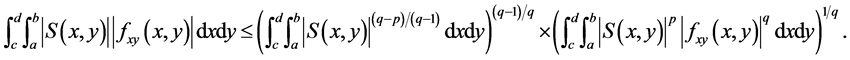(15)
We denote 
and 
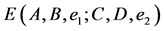by
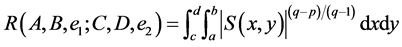
and
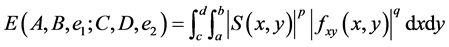
respectively, and then
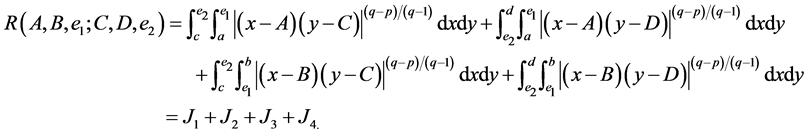(16)
By using the integration techniques, we have
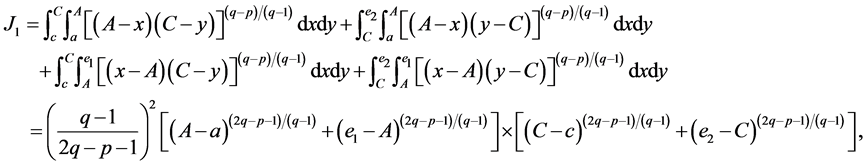
and similary we get,
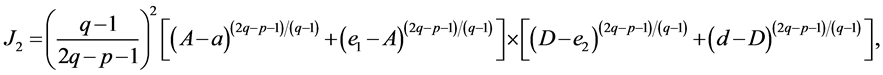
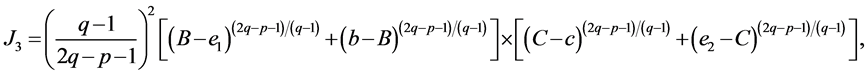
and
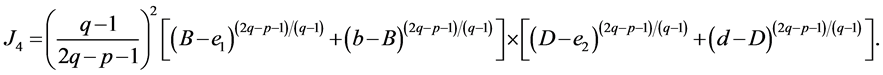
By summing the above four identities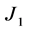
, 
, 
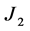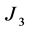and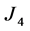 
and simplifying the result. Then according to (16), we get the estimated bound
.
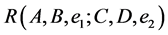On the other hand, by using the identity mappings
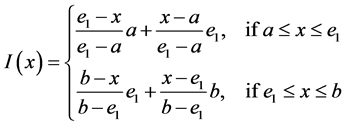
and
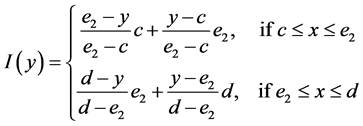
we have
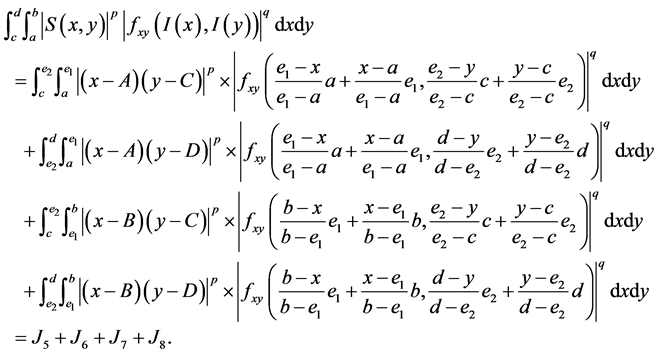(17)
By the convexit3 of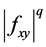 
on the co-ordinates on 
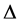and the Inequality (2) in
, 
, 
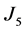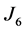and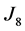
, then we have
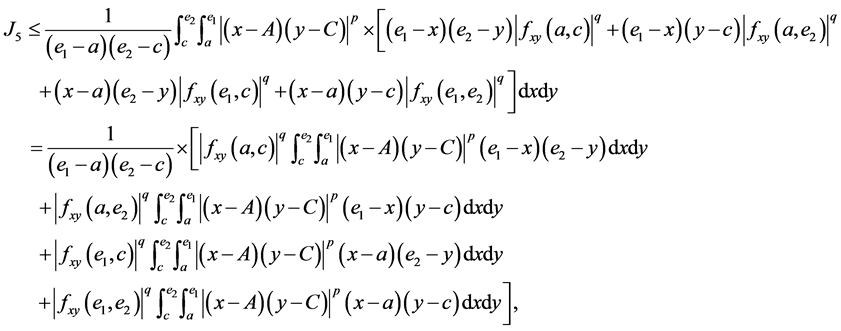
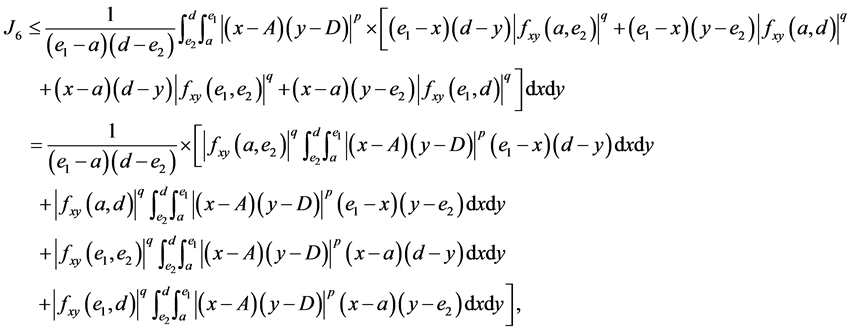
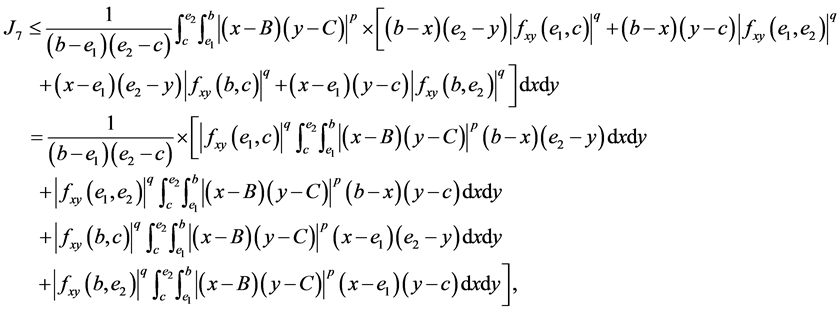
and
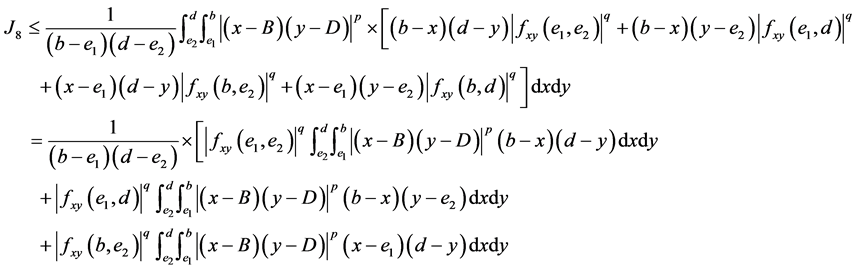
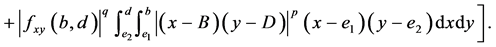
By applying the identities (I), (II), (III) and (IV) to the above four inequalities and then simplifying the results, we get the estimated bound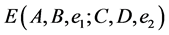 
and the Inequality (14) for
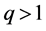. If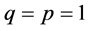
, then the Inequality (14) follows from (15) and (17). The proof of the Inequality (14) is complete. 
Corollary 1. Under the assumptions of Theorem 1 with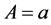
, 
, 
, 
, 
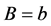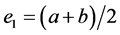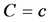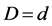and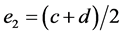
, we have
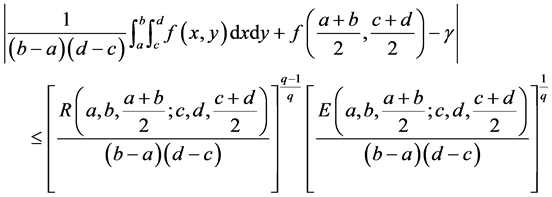(18)
where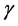 
is as given in Theorem 2,
and
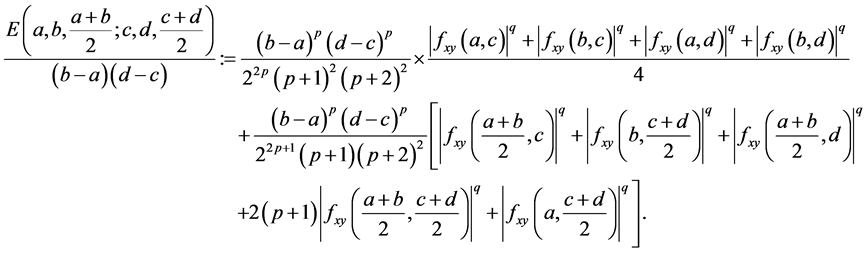
The Corollary 1 shows that we get the new estimated bound of the Inequality (6).
Corollary 2. Under the assumptions of Corollary 1 with
, we have
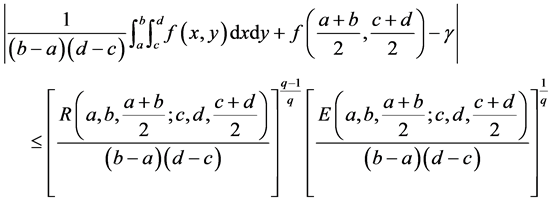(19)
where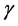 
is as given in Theorem 2,
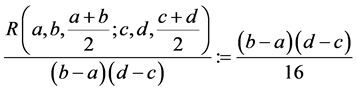
and
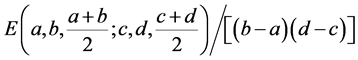
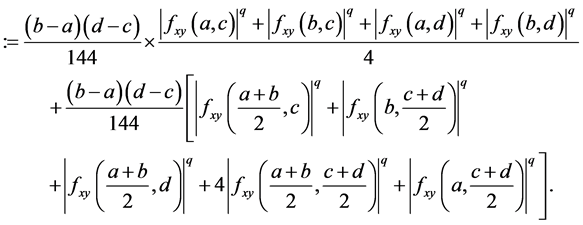
Remark 3. By using the convexity of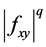 
on the co-ordinates on
, we have the inequality
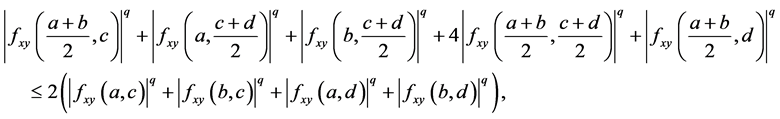
and then
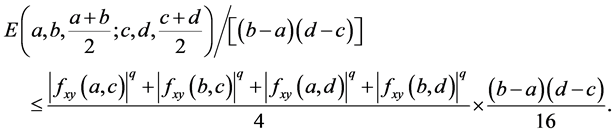
Hence the Inequality (19) improves the Inequality (6).
Remark 4. Under the assumptions of Theorem 1 with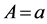
, 
, 
, 
, 
, 
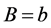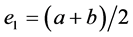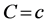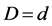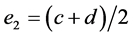and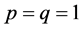
, we get the new estimated bound and it could improve the Inequality (4).
Corollary 3. Under the assumptions of Theorem 1 with
, 
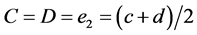, we have
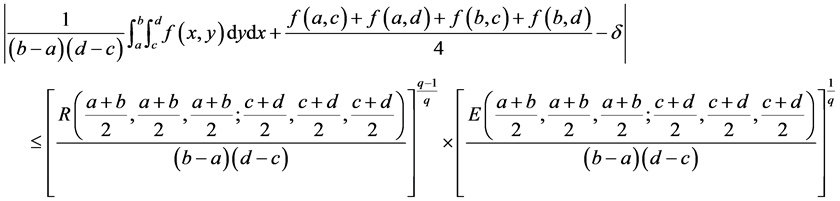(20)
where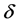 
is as given in Theorem 3,
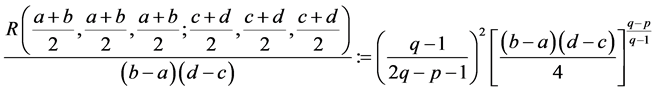
and
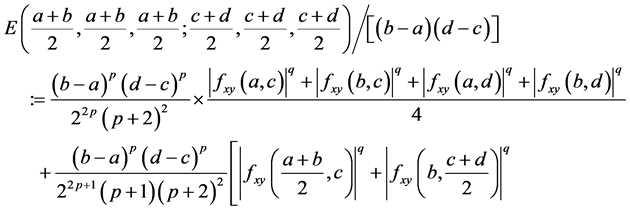
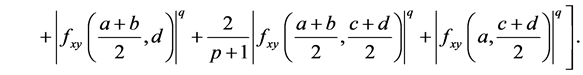
The Corollary 3 shows that we get the new estimated bound of the Inequality (9).
Corollary 4. Under the assumptions of Corollary 3 with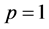
, we have
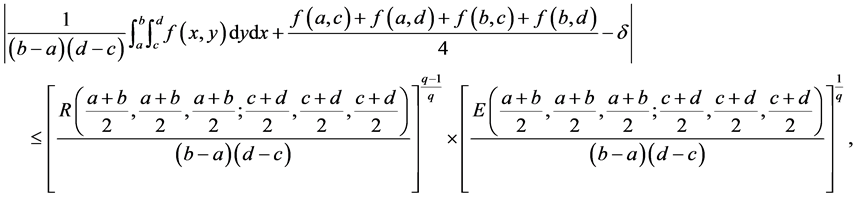(21)
where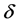 
is as given in Theorem 3,
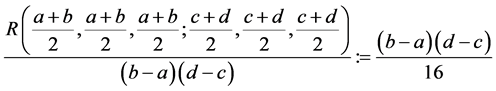
and
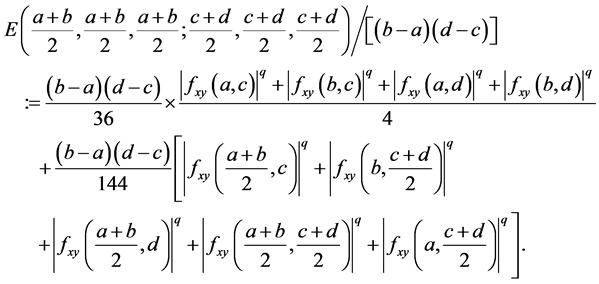
Remark 5. By using the convexity of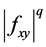 
on the co-ordinates on
, we have the inequality
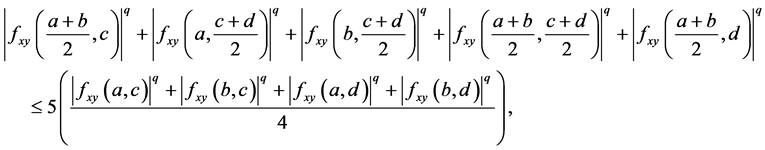
and then
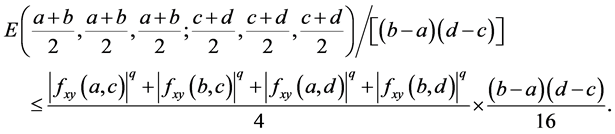
Hence the Inequality (21) improves the Inequality (9).
Remark 6. Under the assumptions of Theorem 1 with
, 
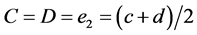and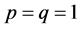
, we get the new estimated bound and it could improve the Inequality (7).
Example 1. Let the function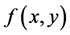 
be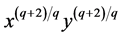
,
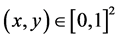. Then the result of the right-hand side of (6) or (9) is
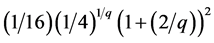, whereas the right-hand side of (19) and (21) are 
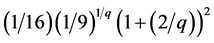and
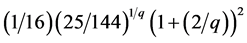, respectively.
3. Some Applications to Special Means
As in [11] we shall consider extensions of arithmetic, logarithmic and generalized logarithmic means from positive real numbers. We take
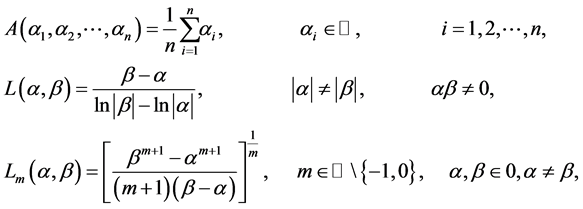
where 
is the set of integers.
Proposition 1. Let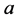
, 
, 
, 
, 
, 
, 
, 
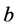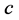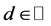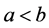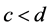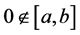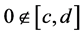, and
, 
, 
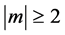and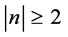
. Then, for
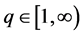, we have
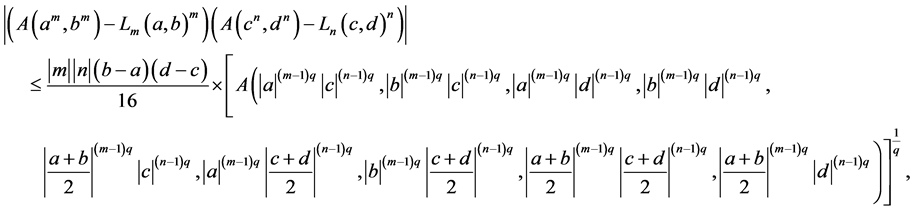(22)
Proof. The proof is immediate from Corollary 4 with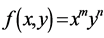
, 
, 
, 
,
.
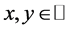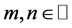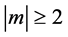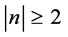Proposition 2. Suppose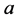
, 
, 
, 
, 
, 
, 
,
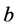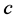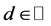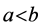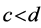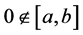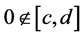. Then, for
, we have
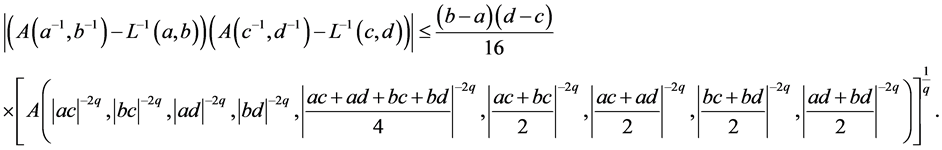(23)
Proof. The result follows from Corollary 4 with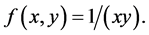 
Remark 7. The Corollary 2 could also be applied to some special means.
Acknowledgements
The author is very grateful to the reviewers for carefully reading this paper and giving constructive comments.
NOTES
*2000 Mathematics Subject Classification. Primary 26D15. Secondary 26A51.