Solutions of Zhiber-Shabat and Related Equations Using a Modified tanh-coth Function Method

Luwai Wazzan

Department of Mathematics, King Abdulaziz University, Jeddah, Saudi Arabia.

DOI: 10.4236/jamp.2016.46111                

Abstract

The modified tanh-coth function method is used to obtain new exact travelling wave solutions for Zhiber-Shabat equation and the related equations: Liouville equation, sinh-Gordon equation, Dodd-Bullough-Mikhailov equation, and Tzitzeica-Dodd-Bullough equation. Exact travelling wave solutions for each equation are derived and expressed in terms of hyperbolic functions, trigonometric functions and rational functions. The modified tanh-coth function method is easy to execute, brief, efficient, and can be used to solve many other nonlinear evolution equations.

Keywords

A Modified tanh-coth Function Method, Zhiber-Shabat Equation, Liouville Equation, sinh-Gordon Equation, Dodd-Bullough-Mikhailov Equation, Tzitzeica-Dodd-Bullough Equation, Travelling Wave Solutions, Solitary Wave Solutions

Received 20 February 2016; accepted 13 June 2016; published 16 June 2016

1. Introduction

In this study we will investigate the solution of the nonlinear Zhiber-Shabat equation 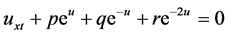[1]

(1)

where p, q and r are arbitrary constants. If q = r = 0, Equation (1) becomes the Liouville equation. If r = 0, Equation (1) becomes the sinh-Gordon equation. And for q = 0, Equation (1) reduces to the well-known Dodd- Bullough-Mikhailov equation. However, for p = 0, q = −1, r = 1, we get the Tzitzeica-Dodd-Bullough equation. These equations play an important role in many areas such as solid state physics, nonlinear optics, plasma physics, fluid dynamics, mathematical biology, nonlinear optics, dislocation in crystals, kink dynamics, and quantum filed theory [1] . The Zhiber-Shabat equation and other related equations were studied by some authors. Wazwaz in [2] and [3] applied the tanh method and the extended tanh method for handling the Zhiber-Shabat equation and other related equations: Liouville equation, sinh-Gordon, Dodd-Bullough-Mikhailov equation, and Tzitzeica- Dodd-Bullough equation. Fan and Hon in [4] have used the extended tanh method for handling Dodd-Bullough- Mikhailov equation. Wu and He in [5] solved the Dodd-Bullough-Mikhailov equation using the Exp-function

method. Wazzan in [6] solved the Zhiber-Shabat equation and other related equations using the 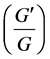expan-

sion-method. Our intention in this work is to find new solitary wave solutions for the nonlinear Zhiber-Shabat equation. Since there is no unified method that can be used to handle all types of nonlinear problems, we will use a modified tanh-coth function method [7] - [10] . Moreover, we will carry out comparisons between solutions obtained by the modified tanh-coth function method and other aforementioned methods.

2. The Modified tanh-coth Function Method

2.1. Description of the Method

To illustrate the basic concepts of the modified tanh-coth function method, we consider a given PDE in two variables given by

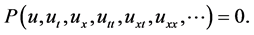(2)

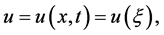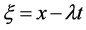We first consider its travelling solutions where or 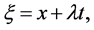then Equation (2) becomes an ordinary differential equation

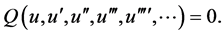(3)

The next crucial step is that the solution we are looking for is expressed in the form:

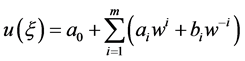(4)

and

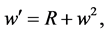(5)

where R is a parameter to be determined later, and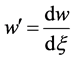. The parameter m can be found by ba-

lancing the highest order linear term with the nonlinear terms. Inserting (4) and (5) into the ordinary differential Equation (3) will yield a system of algebraic equations with respect to a₀, a_i, b_i and R (where). Because all the coefficients of wⁱ have to vanish, and using any symbolic computation program such as Maple or Mathematica, one can determine a₀, a_i, b_i and R. The Riccati Equation (5) has the following general solutions:

1) If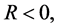

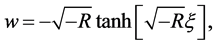
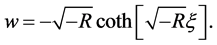
2) If

3) If

In the next section, five examples in mathematical physics are chosen to illustrate the modified tanh-coth function method.

2.2. Application

2.2.1. The Zhiber-Shabat Equation

As before, we use where, this will carry out the Zhiber-Shabat Equation (1) into

(6)

We use the Painleve property:

or equivalently

(7)

from which we find

(8)

(8)

The transformations (7) and (8) carry out (6) into the ODE

(9)

Using the modified tanh-coth function method, balancing the term with v³, gives m = 2, hence we set the modified tanh-coth function method assumption as follows:

(10)

where

(11)

and

Without loss of generality, we set

Substituting (10) into (9), and making use of Equation (11) collecting the coefficients of each power of w, and using Maple to solve the nonlinear system in a₀, a₁, a₂, b₁, b₂ and R, we obtain:

1) First set

2) Second set

3) Third set

4) Fourth set

5) Fifth set

6) Sixth set

where,

Note that, using the numerical value of, we deduce.

Recall that hence we obtain:

According to the first set, for R < 0, solutions for Equation (6) read

and

However, for R > 0, the solutions are

and

According to second set, notice that and , using the numerical value of and for R < 0, we obtain the solutions:

However, for R > 0, we obtain the travelling wave solutions:

and

where b₂ is given in 2).

According to The third set, notice that, using the numerical value of we obtain the soliton solutions, for R < 0,

However, for R > 0, we obtain the travelling wave solutions:

where, R is given in 3).

Note that, u₁, u₂, u₃ and u₄ are also obtained by Wazwaz using the tanh-function method in [2] . Other solutions are not reported in [2] .

Sets of solutions in 4)-6) will give complex solutions.

2.2.2. The Liouville Equation

As stated before, if in the Zhiber-Shabat Equation (1), we obtain the Liouville equation:

Using the wave variable we get

(12)

We again use the Painleve property:

to transform (12) into the ODE

(13)

Considering the homogeneous balance between and v³ in Equation (13), gives m = 2, and using the modified tanh-coth function method, we suppose that the solution of Equation (13) is in the form:

Proceeding as before we found:

1) First set

2) Second set

3) Third set

4) Fourth set

where R is free parameter. Recall that

According to the first set we obtain the solutions:

According to the third set we obtain the similar to the solutions of the first set.

According to the fourth set we obtain the solutions, R < 0,

and if R > 0, then

Note that, u₁ and u₂ are also obtained by Wazwaz using the tanh-function method in [2] . Other solutions are not reported in [2] .

2.2.3. The sinh-Gordon Equation

As stated before, if r = 0, q = 1, p = 1 in the Zhiber-Shabat Equation (1), we obtain the sinh-Gorden equation:

Using the wave variable we get

(14)

Using the Painleve property, Equation (14) is transformed into the ODE

(15)

The balancing process gives m = 2. We can suppose that the solution of Equation (15) is the form:

(16)

Following the same analysis presented above, we obtain:

1) First set

2) Second set

3) Third set

According to the first set, and for R < 0, we obtain

for R > 0, we obtain

According to the second set, we obtain similar solutions to the solutions of the first set.

According to the third set, we obtain, for R < 0, λ > 0,

for R < 0,

for R > 0,

and for R > 0,

Note that, u₁ and u₃ are also obtained by Wazwaz using the tanh-function method in [2] . Other solutions are not reported in [2] .

2.2.4. The Dodd-Bullough-Mikhailov Equation

If p = 1, q = 0, r = 1 in the Zhiber-Shabat Equation (1), we obtain the Dodd-Bullough-Mikhailov equation:

and by using the wave variable we get

(17)

We use the Painleve property:

(18)

to transform (17) into the ODE

(19)

Considering the homogeneous balance between and v³ in Equation (18), gives m = 2, we can suppose that the solution of Equation (15) is the form

(20)

Proceeding as before, we get

1) First set

2) Second set

3) Third set

4) Fourth set

5) Fifth set

6) Sixth set

According to the first set,we obtain the soliton solutions:

and

for λ > 0, we obtain the travelling wave solutions:

and

According to the second set, we obtain the solutions

and

for λ > 0, we obtain the travelling wave solutions:

and

According to the third set and fourth set, we obtain similar solutions to the solutions of the first set and second set, respectively.

According to fifth set, we obtain, for λ < 0, the following solutions

for λ > 0, we obtain the travelling wave solutions

According to sixth set, for λ > 0, this in turn gives the solitons solutions:

for λ < 0, we obtain the travelling wave solutions:

The solutions u₁, u₃, u₃, u₄, u₉, and u₁₀ are also obtained by Wazwaz using the tanh-function method in [3] . Other solutions are not reported in [3] .

2.2.5. The Tzitzeica-Dodd-Bullough Equation

This equation can be obtained if we set p = 0, q = −1, r = 1 in the Zhiber-Shabat Equation (1), and by using the wave variable we find the Tzitzeica-Dodd-Bullough equation:

(21)

suppose that

(22)

or equivalently

(23)

By using (23) we can transform Equation (21) to

(24)

Considering the homogeneous balance between and v⁴ in Equation (24), gives m = 1, and by using the modified tanh-coth function method we can suppose that the solution of Equation (24) is the form:

(25)

Proceeding as before, we get the following set of solutions.

1) First set

2) Second set

3) Third set

According to the first set, we obtain the solitons solutions

and

for R > 0, we obtain the solutions:

and

According to the second set, we obtain the solutions

For R > 0, we obtain the solutions:

According to the third set, we obtain the solutions

The solutions and are also obtained by Wazwaz using the tanh-function method in [3] . Other solutions are not reported in [3] .

3. Conclusion

The Zhiber-Shabat equation, and the related equations: Liouville equation, sinh-Gordon equation Dodd-Bullough- Mikhailov equation, and the Tzitzeica-Dodd-Bullough equation were investigated using a modified tanh-coth method. New travelling wave solutions were established. The modified tanh-coth function method is a robust computational tool for obtaining exact solutions for the nonlinear Zhiber-Shabat equation, and the related equations. It is also an encouraging method to solve other nonlinear evolution equations.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Sirendaoreji, J.S. (2002) A Direct Method for Solving sinh-Gordon Type Equation. Physics Letters A, 298, 133-139. http://dx.doi.org/10.1016/S0375-9601(02)00513-3
[2]	Wazwaz, A.M. (2008) The tanh Method for Travelling Wave Solutions to the Zhiber-Shabat Equation and Other Related Equations. Communications in Nonlinear Science and Numerical Simulation, 13, 584-592. http://dx.doi.org/10.1016/j.cnsns.2006.06.014
[3]	Wazwaz, A.M. (2005) The tanh Method: Solitons and Periodic Solutions for the Dodd-Bullough-Mikhailov and the Tzitzeica-Dodd-Bullough Equations. Chaos, Solitons and Fractals, 25, 55-63. http://dx.doi.org/10.1016/j.chaos.2004.09.122
[4]	Fan, F. and Hon, Y.C. (2003) Applications of Extended tanh Method to “Special” Types of Nonlinear Equations. Applied Mathematics and Computation, 141, 351-358. http://dx.doi.org/10.1016/S0096-3003(02)00260-6
[5]	He, J.-H. and Wu, X.-H. (2006) Exp-Function Method for Nonlinear Wave Equations. Chaos, Solitons and Fractals, 30, 700-708. http://dx.doi.org/10.1016/j.chaos.2006.03.020
[6]	Wazzan, L. (2015) New Exact Travelling Wave Solutions of the Nonlinear Zhiber-Shabat Equation. Far East Journal of Applied Mathematics, 90, 213-244. http://dx.doi.org/10.17654/FJAMMar2015_213_244
[7]	El-Wakil, S.A., El-labany, S.K., Zahran, M.A. and Sabry, R. (2005) Modified Extended tanh-Function Method and Its Applications to Nonlinear Equations. Applied Mathematics and Computation, 161, 403. http://dx.doi.org/10.1016/j.amc.2003.12.035
[8]	El-Wakil, S.A., El-labany, S.K., Zahran, M.A. and Sabry, R. (2002) Modified Extended tanh-Function Method for Solving Nonlinear Partial Differential Equations. Physics Letters A, 299, 179. http://dx.doi.org/10.1016/S0375-9601(02)00669-2
[9]	Wazwaz, A.M. (2006) The tanh and the sinecosine Methods for a Reliable Treatment of the Modified Equal Width Equation and Its Variants. Communications in Nonlinear Science and Numerical Simulation, 11, 148-160. http://dx.doi.org/10.1016/j.cnsns.2004.07.001
[10]	Wazzan, L. (2009) A Modified tanh-coth Method for Solving the General Burgers-Fisher and the Kuramoto-Sivashinsky Equations. Communications in Nonlinear Science and Numerical Simulation, 14, 2642-2652. http://dx.doi.org/10.1016/j.cnsns.2008.08.004