The Scaling of Entanglement Entropy for One Spatial XXZ Spin Chain ()
Received 6 December 2015; accepted 10 January 2016; published 13 January 2016
1. Introduction
The study of quantum condensed matter systems is benefiting from an infusion of ideas related to quantum information and quantum entanglement. Quantum entanglement plays an important role in distinguishing the nature between the quantum systems and classical systems. It also connects quantum information theory to the traditional quantum many-body systems. More recently, entanglement has emerged on the nearby stage of quantum many-body physics, especially for systems that exhibit quantum phase transitions [1] -[6] , where it can play the role of a diagnostic of quantum correlations. Quantum phase transitions [7] are transitions between qualitatively distinct phases of quantum many-body systems, driven by quantum fluctuations. However, quantum many body systems are very hard to study due to the exponential growth of their Hilbert space with the number of constituents. By employing the matrix product states to approximate ground states [8] [9] , the entanglement entropy for one dimensional spin system is obtained. It is thus obvious that the matrix product states with matrices of finite size cannot describe exactly the behavior of an infinite system at the critical point but we may try to find the exact amount of entanglement which is captured.
The important information is embeded in the way a state approaches the thermodynamic limit and one can extract it by using the celebrated finite size scaling technique [10] . This technique amounts to study even larger systems in a gapless phase and extract universal properties through the dependence of the physical observables on the truncation dimension of the matrix.
The rest of this paper is organised as follows. In Section 2, we recall the physics of spin-1 models with long- range interactions. Section 3 discusses the entanglement entropy and the scaling relationship of the spin-1 model and shows our simulation results for the one-dimensional spin-1 model. Finally, Section 4 contains our conclusions.
2. The One Dimensional Spin-1 XXZ Model with Uniaxial Single-Ion-Type Anisotropy
One-dimensional antiferromagnetic spin chains have been the subject of recent investigations by numerous groups. The Hamiltonian for spin-1 XXZ model with uniaxial single-ion-type anisotropy [11] is given as
where J = 1 to fix the energy scale,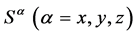 
spin-1 operator, D represents uniaxial single-ion anisotropy, Jz is the controlable parameter. The ground-state phase diagram of the spin-1 model consists of the Haldane phase, the large-D phase, XY phases, the ferromagnetic phase, and the Neel phase [12] [13] . For the integer spin, there is a gap between the first excited state and the ground state.
A gapful phase to gapful phase transition happened between the Haldane phase and large-D phase; the type of the quantum phase transition between the Neel phase and Haldane phase is the Ising transition. The central charge, which is associated with the universality class of the quantum phase transition, for the Ising transition is 0.5. Employing invariance under translations and parallelizability of local updates, matrix product states can simulate infinite systems directly, without resorting to costly, less accurate extrapolations. We obtain the approximate ground states of different truncation dimension for the spin-1 model by using matrix product states.
3. The Entanglement Entropy and Scaling Relationship for One Dimensional Spin-1 XXZ Model
The entanglement entropy is a measure of a bipartite entanglement present in a quantum state, whose behavior is, in many occasions, universal [14] . For a bipartite system AB that consists of the system A and the environment B. The reduced density matrix of the system A is
. The entanglement entropy
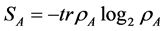, which is used to measure the bipartite entanglement between A and B. 
is become the 
in the representation of ground state by the matrix product state,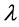 
is a diagonal matrix. For all one-dimensional gapped quantum spin systems, the entanglement entropy saturates to a constant independently of the size of the block [15] . The entanglement obeys the scaling law 
[16] [17] , 
is the truncation dimension of the matrix product state. It is clear that the entanglement entropy of half of the infinite chain with the other half will diverge as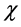 
goes to infinity. The known results [16] - [18] shown that it can be used to characterize both quantum criticality and topological phases in a variety of quantum many-body systems.
For the spin-1 model, the parameter Jz = 1, the quantum phase transitions between the Large-D phase and the Haldane phase are investigated by the entanglement entropy, which is shown in Figure 1. The critical points given by the truncation dimension are shown as: c = 20, 30, 40, 50, 60; D = 1.119, 1.073, 1.056, 1.039, 1.026, respectively. The entanglement entropy between the Haldane phase and the Neel phase is shown in Figure 2. The critical points given by the truncation dimension are shown as: c = 20, 30, 40, 50, 60; D = 0.810, 0.849, 0.876, 0.891, 0.900, respectively. The peak given by different truncation dimension is the pseudo-critical point, which is drawing near the critical point of the system.
The entanglement entropy scales logarithmically when the system becomes quantum-critical. The scaling relationship [19] -[22] between the entanglement and the correlation length is given as
![]()
where
, 
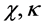is the truncation dimension of the matrix product state and the free parameter, respectively. The scaling relationship is given as
![]()
Figure 1. The entanglement entropy between the Large-D phase and the Haldane phase with Jz = 1. The critical points given by the truncation dimension are shown as: c = 20, 30, 40, 50, 60; D = 1.119, 1.073, 1.056, 1.039, 1.026, respectively.
![]()
Figure 2. The entanglement entropy between the Haldane phase and the Neel phase with Jz = 1. The critical points given by the truncation dimension are shown as: c = 20, 30, 40, 50, 60; D = 0.810, 0.849, 0.876, 0.891, 0.900, respectively.
![]()
The free parameters a and b can be obtained by fitting the entanglement entropy of the critical point given by the matrix product state and the corresponding truncation dimension. The fitting results for the quantum phase transitions between the Large-D phase and the Haldane phase and the quantum phase transition between the Haldane phase and the Neel phase are shown in Figure 3 and Figure 4. The scaling relationship between entanglement entropy S and truncation dimension c for the quantum phase transition between the Large-D phase and the Haldane phase is given with a = 0.226, b = 0.509. The scaling relationship between entanglement entropy S and truncation dimension c for the quantum phase transition between the Haldane phase and the Neel phase is given with a = 0.185, b = 0.753.
4. Conclusion
We investigate the scaling of entanglement entropy for one spatial XXZ spin chain by using matrix product states to approximate ground states. The entanglement entropy scales logarithmically with a coefficient that is determined by the associated conformal field theory, the quantum phase transitions occurred between Large-D
![]()
Figure 3. The scaling relationship between entanglement entropy S and truncation dimension c is shown with a = 0.226, b = 0.509.
![]()
Figure 4. The scaling relationship between entanglement entropy S and truncation dimension c is shown with a = 0.185, b = 0.753.
and Halde phase, Halde phase and Neel phase. We have shown that the entanglement entropy is an efficient quantity in characterizing the Ising transitions and the Gaussian transitions in one dimension. The scaling plays crucial roles on identifying a quantum system with a physically different classical system. We hope that our results will be useful in studying the quantum spin system in one dimension.
Acknowledgements
This work was financially supported by the National Natural Science Foundation of China (Grant No. 11447115) and the Science Foundation of Chongqing City Board of Education (KJ121206).
NOTES
![]()
*Corresponding author.