Theoretical Economics Letters
Vol.05 No.02(2015), Article ID:55244,7 pages
10.4236/tel.2015.52026
Pricing of a Risk Averse Monopoly in the Presence of Stochastic Demand
Kolos Csaba Ágoston
Department of Operation Research and Actuarial Sciences, Corvinus University of Budapest, Budapest, Hungary
Email: kolos.agoston@uni-corvinus.hu
Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 10 February 2015; accepted 22 March 2015; published 31 March 2015
ABSTRACT
In the paper, we investigate the pricing behavior of a risk averse monopoly. Since the focus is on the risk averse attitude of the firm, we ignore cost in our model. Demand is considered to be stochastic demand: as price decreases, the expected number of customers increases, but it has a varia- tion. Although demand is uncertain, it relates to the aggregation method of individual demands and the individual demand has the usual form. In our framework a risk neutral (or profit maximizer) monopoly does not change the product’s price as the number of clients increases. On product markets the risk averse monopoly with DARA utility function always increases the price as the number of clients grows, but in insurance markets the implication can be the opposite: the price of insurance may decrease as the number of clients increases.
Keywords:
Monopoly, Risk Aversion, Insurance, Market Size

1. Introduction
Stochastic demand is not unknown in the economic literature. In management science models it is well-studied (see e.g. [1] [2] ). In microeconomic models stochastic demand appears less frequently. If stochastic demand is considered in microeconomic models, it usually means that the demand depends on some unknown (external) parameter or it is due to some kind of bounded rationality. Barberá and Pattanaik [3] , for example, investigate the case where the decision maker chooses randomly between alternatives. In this paper, the individual demand has the usual form, but for a risk averse decision maker it is not appropriate to simply sum up the individual demands. As we will see, for a risk neutral firm summing up is acceptable, but for a risk averse decision maker the uncertainty about demand has a significant effect on the optimal price (see more on aggregation of stochastic in e.g. [4] [5] ).
It is usual in microeconomic theory that increased demand increases market price. We can think of Marshallian cross for instance. There are known exceptions, such as the Giffen goods. Hoy and Robson [6] gave an example where insurance can be a Giffen good. In our model the individual demand has a downward slope, therefore the case of Giffen good is excluded.
Pricing of a monopoly differs from pricing of a competitive firm. It can happen that a monopoly reduces its price due to the increased demand, but the decrease is related to the costs (natural monopoly for instance) or the increase of the demand is not uniform. In our model we would like to focus on risk aversion of the decision maker, thus we ignore costs. On the other side, the increase of demand is uniform.
We provide a simple framework for investigating the pricing of a risk averse monopoly. We distinguish between product market and insurance market. In the product market increased demand will cause increased monopoly price, but in the insurance market it can happen, that increased demand results in reduced price.
In the insurance economic literature there are usually two kinds of models: insurer has many (or many type of) contracts, but the insurer is risk neutral (e.g. [7] [8] ). In the other type of model the insurer is risk averse but the model investigates (one or few) typical contracts (e.g. [9] [10] ). It is very rare when a risk averse insurer is considered to have many insured, which is the everyday situation.
Insurance markets are dominated by a few (or not many) companies, which remind us to the market form of oligopoly. It depends on the situation whether the competitive economy or the monopoly is a more realistic mar- ket form. The presence of a monopolistic insurance company is accepted in the literature (see [7] ), which is also the case in our paper.
In Section 2, we present our theoretical model for both cases of product market and insurance market. In Section 3, we conclude the results.
2. Theoretical Model
In the model we consider a monopoly. It sells its product to (potential) customers. Cost is considered as sunk cost which does not affect the optimal price. The monopoly can fulfill the demand of arbitrary number of cus- tomers. An illustrative example is the case of a software vendor: the firm can sell any copies of a program with- out cost. Also an insurance company can supply insurance to many clients.
Customers can decide to buy the product or not, but they cannot buy fraction of the product. e.g. it is im- possible to buy a half of a software. In the insurance market it could be possible to buy fraction of the coverage, but we exclude this case. The clients’ decision is to buy full coverage or not to buy insurance. In the mo- nopolistic market the insurance company sets the contract’s properties and it is not interested in fractional cove- rages.
Each client has a reservation price (which differs from client to client), but the monopoly does not know the exact price, it knows only the distribution of the reservation prices within a greater community. So let 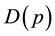 be the probability that a (particular) client buys the monopoly’s product at price
be the probability that a (particular) client buys the monopoly’s product at price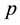 . We assume that function
. We assume that function 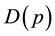 is decreasing and it is continuously differentiable. If the monopoly has more than one potential client, say
is decreasing and it is continuously differentiable. If the monopoly has more than one potential client, say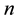 , then the demand function will not be
, then the demand function will not be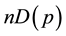 , but the number of insureds follows a binomial dis- tribution. The probability that
, but the number of insureds follows a binomial dis- tribution. The probability that  clients buy the product is:
clients buy the product is:

where 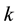 can be any integer between 0 and
can be any integer between 0 and .
.
The monopoly is risk averse and maximizes its expected utility. Its behavior can be described with a concave (risk averse) utility function .
.
2.1. Product Market
In case of a product market we can assume that , and for the simplicity we also assume that there ex- ists price
, and for the simplicity we also assume that there ex- ists price 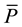 such that
such that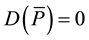 .
.
The monopoly’s expected utility at price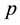 :
:
 (1)
(1)
where 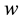 denotes the monopoly’s initial wealth.
denotes the monopoly’s initial wealth.
We assume that the expression 


If the monopoly is risk neutral (utility function is linear) then the expected utility becomes simple:
In Theorem 1 we prove that a risk averse monopoly applies a lower price than a risk neutral monopoly.
Theorem 1. A risk averse monopoly sets a lower price than a risk neutral firm.
Proof. Let us see the expected utility’s (1) derivative with respect to price:
A risk neutral decision maker sets a price 


which is negative due to concavity of 


The interpretation of Theorem 1 is that a risk averse monopoly is satisfied with a lower price which ensures higher probability of selling.
What happens if the number of clients increases? The risk aversion solely is not enough to change the mo- nopoly’s price. Let us consider e.g. exponential utility function


The optimal price is the same for all
If we assume decreasing absolute risk aversion (DARA), which is a more realistic assumption, we can state that the optimum price increases as market size increases:
Theorem 2. Let us consider a risk averse monopoly with a DARA (decreasing absolute risk averse) utility function. The monopoly determines a higher price in case of 

The proof can be found in the Appendix.
From Theorem 1 and Theorem 2 we can have the intuition that the optimal price converges to the risk neutral price as the number of clients tends to infinity. Unfortunately this intuition is false.
Example 1. Let









If we set 




2.2. Insurance Market
In case of the product market there is only one source of uncertainty: whether the client buys the product or not. In case of insurance market there is another source of uncertainty: whether a claim occurs or not. For simplicity we investigate a two state model: a claim 

From classical risk theory we know that 

The expected utility of an insurance company:

The expected utility becomes simple for a risk neutral monopoly again:


For an exponential utility function, there is a closed formula for the expected utility:
The optimal price for an exponential utility function 
The risk averse insurance monopoly takes two impacts. It would set a lower price than a risk neutral company to sell more contracts as in the case of a product market. On the other hand, we also know from the classical risk theory that a risk averse firm applies higher price for insurance than a risk neutral. So there are two opposite effects, we could not decide on whether the price will be higher or lower than the risk neutral price (we can give ex- ample for higher and lower prices as well).
From risk theory we know that the average claim amount disperses less for a greater risk community, al- though greater extreme losses can also happen. A greater risk community can be advantageous for a risk averse decision maker, and as market size increases this advantage may exceed the profit loss from a lower price. In Example 2, we demonstrate the previously described situation: the insurance company can decrease its in- surance’s price as the demand (number of clients) increases. It is an interesting situation: the increase of market size is a common interest for insurance company and insureds. In product market the interest of the monopoly and the clients are always in conflict.
Example 2. Let


As we can see, the utility function is a mixture of a risk neutral part (

the price for exponential utility function (CARA price hereafter). If
then as n grows, the common price will be closer to CARA price, if
Let parameter q and K take values such that 

For the second condition it is enough if



For numerical check: let


and



Table 1. The optimal market prices for different market size. 









We can make two important remarks: let parameter 

Let parameter 


3. Conclusions
In the paper, we presented a microeconomic framework, in which a risk averse monopoly’s behavior can be investigated. We proved that in a product market a risk averse monopoly applies a lower price than a risk neutral (profit maximizer). If the risk aversion decreases with wealth (DARA), the market price will increase with the market size.
In the insurance market the price can decrease or increase with the market size even for utility function with DARA property. We have given numeric example for both cases.
Acknowledgements
I would like to thank Aegon Hungary insurance company, Insurance Education and Research Group, Corvinus University of Budapest (BOKCS) and Association of Hungarian Insurance Companies (MABISZ) for supporting my research. I thank Miklós Pintér, Péter Bíró, Bianka Ágoston and Tamás Polereczky for their suggestions and remarks. Naturally, all errors are mine.
References
- Schmitt, A.J., Snyder, L.V. and Shen, Z.M. (2010) Inventory Systems with Stochastic Demand and Supply: Properties and Approximations. European Journal of Operational Research, 206, 313-328. http://dx.doi.org/10.1016/j.ejor.2010.02.029
- Holmberg, K. and Tuy, H. (1999) A Production-Transportation Problem with Stochastic Demand and Concave Production Costs. Mathematical Programming, 85, 157-179. http://dx.doi.org/10.1007/s101070050050
- Barberá, S. and Pattanaik, P.K. (1986) Falmagne and the Rationalizability of Stochastic Choices in Terms of Random Orderings. Econometrica, 54, 707-715. http://dx.doi.org/10.2307/1911317
- Bandyopadhyay, T., Dasgupta, I. and Pattanaik, P.K. (2002) Demand Aggregation and the Weak Axiom of Stochastic Revealed Preference. Journal of Economic Theory, 107, 483-489.
- Alcantud, J.C.R. (2006) Stochastic Demand Correspondences and Their Aggregation Properties Decisions. Economics and Finance, 29, 55-69. http://dx.doi.org/10.1007/s10203-006-0060-6
- Hoy, M. and Robson, A.J. (1981) Insurance as a Giffen Good. Economics Letters, 8, 47-51. http://dx.doi.org/10.1016/0165-1765(81)90091-4
- Stiglitz, J.E. (1977) Monopoly, Non-Linear Pricing and Imperfect Information: The Insurance Market Review of Economic Studies. Review of Economic Studies, 44, 407-430. http://dx.doi.org/10.2307/2296899
- Kliger, D. and Levikson, B. (1998) Pricing Insurance Contracts―An Economic Viewpoint. Mathematics and Econo- mics, 22, 243-249. http://dx.doi.org/10.1016/S0167-6687(98)00002-X
- Raviv, A. (1979) The Design of an Optimal Insurance Policy. American Economic Review, 69, 84-96.
- Zhou, C. and Wu, C. (2008) Optimal Insurance under the Insurer’s Risk Constraint. Mathematics and Economics, 42, 992- 999. http://dx.doi.org/10.1016/j.insmatheco.2007.11.005
- Gollier, C. (1999) The Economics of Risk and Time. GREMAQ and IDEI, University of Toulouse, Toulouse.
- Mosin, J. (1968) Aspects of Rational Insurance Purchasing. Journal of Political Economy, 76, 553-568. http://dx.doi.org/10.1086/259427
Appendix
Proof of Theorem 2
Lemma 1. For monopoly’s expected utility the following recursive relationship holds:

Proof. We start from the recursive formula for binomial numbers: 

For the sake of simplicity we introduce the following notations:
And
It is easy to check that the recursive relationship (6) also holds for expressions 

Lemma 2 
Proof.
where 
By the Cauchy’s mean value theorem there exist 
Now one thing is missing: 
of utility functions with DARA property is also a utility function with DARA property (see [11] , page 104). If
we define

is equivalent to the decrease of
Proof of Theorem 2. The first order condition of 
Which can be rearranged as

We investigate the sign of 


mine the sign of



Using the recursive relationship (6):
Applying Lemma 2 and using some algebra we can state, that
Which actually means, that
















