Vol.2, No.11, 1318-1325 (2010) Natural Science
http://dx.doi.org/10.4236/ns.2010.211160
Analytical expression of non steady-state concentration
profiles at planar electrode for the CE mechanism
Vincent Michael Raj Margret PonRani, Lakshmanan Rajendren
Department of Mathematics, The Madura College, Madurai, India;
Received 3 August 2010; revised 5 September 2010; accepted 8 September 2010.
ABSTRACT
The analytical solutions of the non-steady-state
concentrations of species at a planar micro-
electrode are presented. These simple new ap-
proximate expressions of concentrations are
valid for all values of time and possible values
of rate constants. Analytical equations are given
to describe the current when the homogeneous
equilibrium position lies heavily in favour of the
electroinactive species. Working surfaces are
presented for the variation of limiting current
with a homogeneous kinetic parameter and
equilibrium constant. Moreover, in this work we
employ the Homotopy perturbation method to
solve the boundary value problem.
Keywords: Planar Electrode; CE Mechanism;
Mathematical Modelling; Reaction/Diffusion
Equation; Homotopy Perturbation Method
1. INTRODUCTION
One of the major achievements in electroanalytical
chemistry in the 1980s was the introduction of micro-
electrodes, i.e., electrodes whose characteristic dimen-
sion is on the order of a few m

(the radius in the case
of disc and hemispheres, band width in the case of bands,
etc.). Microelectrodes have become more commonly
used in electrochemistry to probe kinetics of fast chemi-
cal reactions [1]. In this work, we are interested in find-
ing the mass transport limiting current response for the
CE mechanism at a microelectrode. For each mechanism,
the electroinactive species A is in dynamic equilibrium
with the electroactive species B via a homogeneous
chemical step. The decay of species A is described by
the first order forward rate constant
f
k and the reverse
of this process is described by the rate constant b
k,
which is first order for the CE mechanism. All species
are considered to have an diffusion coefficient D.
Oldham [2] made use of an analytical expression of
CE mechanism at a hemispherical electrode. Lavagnini
et al .[3] employed the hopscotch method and a confor-
mal map to numerically simulate CE mechanism at a
planar electrode. Values of limiting current were ana-
lysed for a range of equilibrium constants and rate con-
stants. There have been many previous theoretical de-
scriptions of the diffusion limiting current for the CE
mechanism. In Reference [4], Fleischmann et al. dem-
onstrate that Neumann’s integral theorem can be used to
simulate CE mechanism at a disc electrode. However, to
the best of the author’s knowledge, no purely analytical
expressions for the non-steady-state concentrations of
these CE mechanisms have been reported. The purpose
of this communication is to derive approximate analyti-
cal expressions for the non-steady-state concentrations
of the species for all values of 1
m, 2 m, 1 k, 2 k, 3 k
and 4
k using Homotopy perturbation method.
2. MATHEMATICAL FORMULATION OF
THE BOUNDARY VALUE PROBLEM
As a representative example of the reaction-diffusion
problems considered, the standard CE mechanism
2
A
B B eproducts


has been chosen, with initial and boundary conditions
corresponding to the potential step for all planar elec-
trodes. Under stead-state conditions, the local concentra-
tions of the species do not change. Therefore the mass
transport equations are set equal to zero. We consider the
differential equations with diffusion described by the
concentration of the two species leads to the following
equations [5]
2
20
fb
aa
Dkakb
tx



 (1)
2
20
fb
bb
Dkakb
tx



 (2)
where a and b denote the concentration of the spe-
cies
A
and B. x and t stand for space and time, respec-
tively.
f

and b

are described the forward and

V. M. R. M. PonRani et al. / Natural Science 2 (2010) 1318-1325 1319
backward rate constants respectively. The boundary
conditions reduce to
00
0; ; taabb  (3)
; ;
s
s
x
laabb 
(4)
00
; ;
x
aabb (5)
where 0 a and 0 b are the bulk concentrations of the
species
A
and B,
s
aand
s
b denote the concentra-
tions at electrode surface .The flux j can be described as
follows:
jD
x
l
b
x


 (6)
The current density is defined as:
inFj (7)
Where n is the number of electrons and F is the Faraday
constant. Using the following dimensionless parameters
2
1
2
00
2
22
0
0
23412
00 00
; ; ; ; ;
; ; ; ;
f
s
s
f
bb
kl
ab xDt
uvXT k
ab lD l
kla
klbk lab
kkkmm
DaDbD ab
 

we obtained the dimensionless non-linear reaction diffu-
sion equations for planar electrode as follows
2
12
20
uu
kuk v
TX


 (9)
2
34
20
vv
ku kv
TX


 (10)
The initial and boundary conditions becomes:
0; 1;Tu

1v (11)
12
1; ;
X
um vm  (12)
; 1; 1Xuv (13)
The dimensionless current is as follows:
1
0
()
X
il vX
nFADb


 (14)
3. ANALYTICAL SOLUTION OF THE
CONCENTRATIONS AND CURRENT
USING HOMOTOPY PERTURBATION
METHOD
Recently, many authors have applied the Homotopy
perturbation method to various problems and demon-
strated the efficiency of the Homotopy perturbation
method for handling non-linear structures and solving
various physics and engineering problems [6-9]. This
method is a combination of homotopy in topology and
classic perturbation techniques. Ji-Huan He used the
Homotopy perturbation method to solve the Lighthill
equation [10], the Duffing equation [11] and the Blasius
equation [12]. The idea has been used to solve non-linear
boundary value problems [13], integral equations
[14-16], Klein–Gordon and Sine–Gordon equations [17],
Emden –Flower type equations [18] and many other
problems. This wide variety of applications shows the
power of the Homotopy perturbation method to solve
functional equations. The Homotopy perturbation
method is unique in its applicability, accuracy and effi-
ciency. The Homotopy perturbation method [19] uses the
imbedding parameter p as a small parameter, and only a
few iterations are needed to search for an asymptotic
solution. By applying Laplace transformation to the par-
tial differential Eqs.9 and 10 and using the condition
Eq.11, the following diffential equations in Laplace
space are obtained:
2
12
2 1 0
du kuk vsu
dX   (15)
2
34
2 1 0
dv ku kvsv
dX

  (16)
Now the boundary conditions become
11
; ; Xuv
s
s
 (17)
12
1; ;
mm
Xu v
s
s
  (18)
where
s
is the Laplace variable and an overbar indi-
cates a Laplace-transformed quantity. The set of expres-
sions presented in Eqs.15-18 defines the initial and
boundary value problem in Laplace space. The Homo-
topy perturbation method method has overcome the
limitations of traditional perturbation techniques, so a
considerable deal of research has been conducted to ap-
ply the homotopy technique to solve various strong
non-linear equations.
The Homotopy perturbtion method [20-22] is used to
give the approximate analytical solutions of coupled
non-linear Eqs.15 and 16. Using this method (see Ap-
pendix – A and B) the approximate solutions of the
Eqs.9 and 10 are

1
1
(,)11 2
X
uXTmerfc T
 
 



2
11
2exp1
42
X
TX
Xerfc
TT 








 













112 2
22
kmk m








(19)

V. M. R. M. PonRani et al. / Natural Science 2 (2010) 1318-1325 1320

2
1
(,)11 2
X
vXTmerfc T
 
 



2
11
2exp1
42
X
TX
X
erfc
TT 



 










31 42 1
22
km km X

 






(20)
The Eqs.19 and 20 satisfies the boundary conditions
Eq.11 to Eq.13. These equations represent the new ap-
proximate analytical expressions for the concentration
profiles 12123
, , , , mmkk k and 4 k.The third term in
the Eqs.19 and 20 are in opposite sign when 13
kk

and 24 kk or 00 ab. Also the dimensionless
concentration u and v are equal when 12 mm

(ratio of concentration at electrode surface for the bulk
concentration) and 00 .abThe current density is

2
3142
0.56419 0.564192 0.050.5
mTkm km
TT
  
(21)
4. DISCUSSION
Eqs.19 and 20 are the new and simple approximate
analytical expressions of concentrations of the isomers
calculated using Homotopy perturbation method for the
initial and boundary conditions Eqs.11-13. The closed
analytical expression of current is represented by the
Eq.21. The dimensionless concentration profiles of u
and v versus dimensionless distance
X
are given in
Figures 1-4 and Figures 5-8 respectively. From these
figures, we can see that the value of the concentration
u and v decreases when T increases and attains the
steady- state value at 40X. When the rate constants
are small (less than 1) and 12
mm the concentration
decreases slowly and reaches the minimum value and
then increases in Figures 1 and 5. From Figures 2, it is
inferred that the concentration u attains the
steady-state value at 5X. Also, when all the parame-
ters are small and 1T, the concentration attains
maximum value at 4
X
 in Figures 3 and 4. For large
value of parameters 341
, ,kkmand 2 m, the concentra-
tion v decreases when T increases in Figures 6. For
the small values of parameter and time T (1T

),
there is no significance different in the concentration.
(Refer Figures 7(a,b), Figures 8(a,b). The dimen-
sionless current

versus T for various values of 3 k
and 4
k is given in Figures 9 and 10. From these figures
the value of current decreases as the time Tand 4 k
increases. But the value of current increases when 3 k
increases.
5. CONCLUSIONS
In this work, the coupled time dependent linear dif-
Figure 1. Normalized concentration u at mi-
croelectrode. The concentrations were com-
puted using Eq.19 for some value of 11m

,
21m

and for various values of Tand the
reaction/diffusion parameter 10.001 kand
20.005k

.
Figure 2. Normalized concentration u at micro-
electrode. The concentrations were computed
using Eq.19 for some value of 10.5m,
21m

and for various values of Tand the re-
action/diffusion parameter 11 kand 25k

.
(a)

V. M. R. M. PonRani et al. / Natural Science 2 (2010) 1318-1325 1321
(b)
(c)
Figure 3. Normalized concentration uat micro-
electrode. The concentrations were computed us-
ing Eq.19 for some fixed value of
12
0.1, 1mm
and the reaction/diffusion pa-
rameter 11k and 20.1k for various values
of (a) 0.1T; (b)1T; (c) 10T.
(a)
(b)
(c)
(d)
Figure 4. Normalized concentration uat mi-
croelectrode. The concentrations were com
puted using Eq.19 for some fixed value
of 12 0.001,0.005mm

and the reac-
tion/diffusion parameter 10.005k and
20.001k

for various values of (a) 0.1T;
(b) 1T

; (c) 10T

; (d) 100T.
Figure 5. Normalized concentration v at mi-
croelectrode. The concentrations were computed
using Eq.20 for some value of 12 1, 1mm
and for various values of Tand the reac-
tion/diffusion parameter 30.01 kand
40.05k.

V. M. R. M. PonRani et al. / Natural Science 2 (2010) 1318-1325 1322
Figure 6. Normalized concentration vat mi-
croelectrode. The concentrations were computed
using Eq.20 for some value of 12
1, 0.5mm
and for various values of Tand the reac-
tion/diffusion parameter 15kand 210k.
ferential equations at planar electrode have been solved
analytically. In the first part of the paper, we have de-
rived the analytical expressions of the concentrations of
the species for all values of rate constants for planar
electrode. In the second part of the paper we have pre-
sented approximate analytical expressions corresponding
to the species
A
and B in terms of the kinetic pa-
rameters1
k,2 k,3 k and 4 k based on the Homotopy
perturbation method. In addition, we have also presented
an analytical expression for the non-steady state current.
The kinetics of this homogeneous step can in principle
be studied by observing how the limiting current re-
(a)
(b)
(c)
Figure 7. Normalized concentration v at micro-
electrode. The concentrations were computed us-
ing Eq.20 for some value of 12
1, 0.1mm
and the reaction/diffusion parameter
34
0.1, 1kk

 and for various values of (a)
0.1 T

; (b)1T

; (c) 10T.
(a)
(b)
(c)

V. M. R. M. PonRani et al. / Natural Science 2 (2010) 1318-1325 1323
(d)
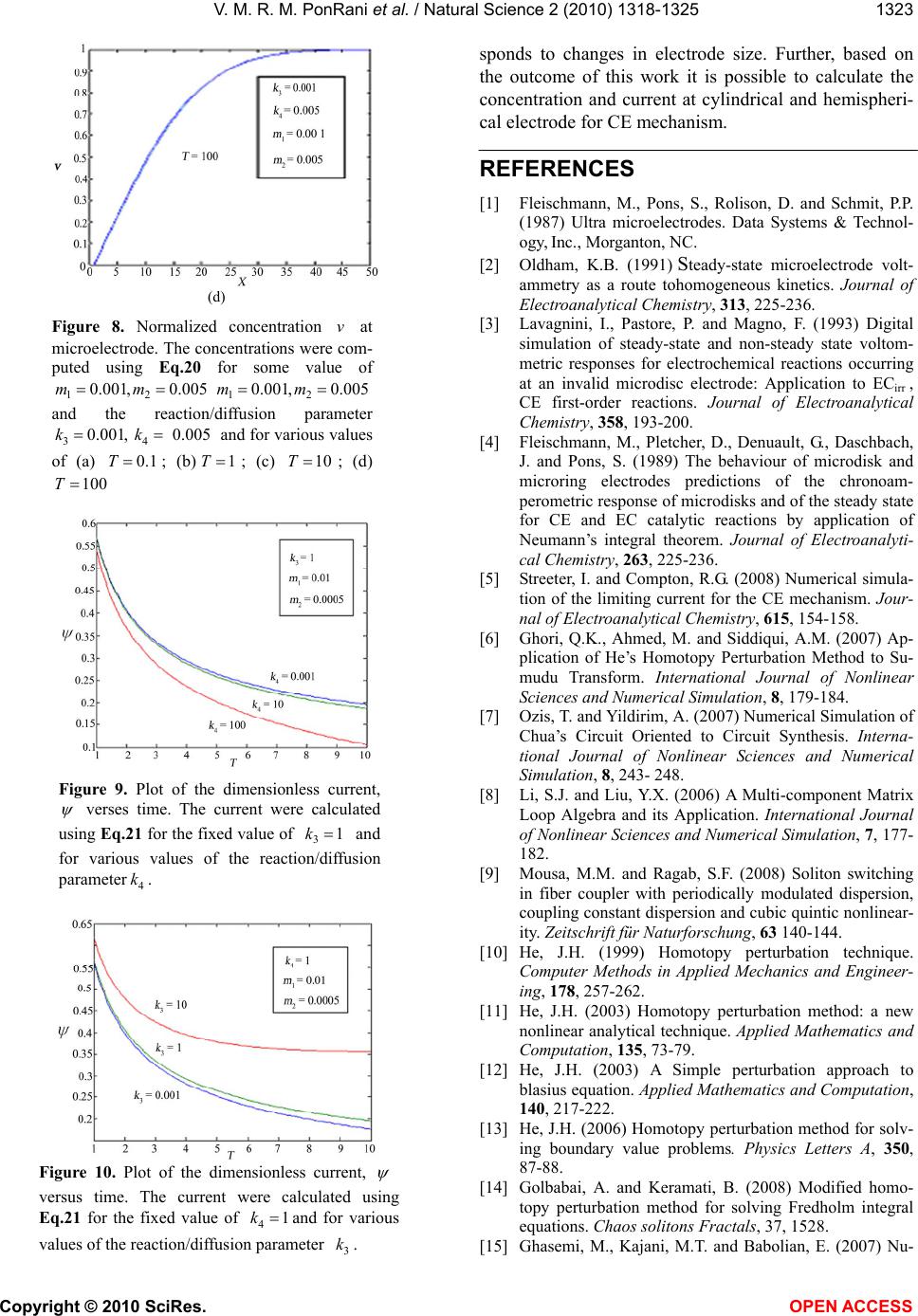
Figure 8. Normalized concentration v at
microelectrode. The concentrations were com-
puted using Eq.20 for some value of
12
0.001,0.005mm
12
0.001,0.005mm


and the reaction/diffusion parameter
34
0.001, 0.005 kkand for various values
of (a) 0.1 T; (b)1T; (c) 10T; (d)
100 T
Figure 9. Plot of the dimensionless current,

verses time. The current were calculated
using Eq.21 for the fixed value of 31k and
for various values of the reaction/diffusion
parameter 4 k.
Figure 10. Plot of the dimensionless current,

versus time. The current were calculated using
Eq.21 for the fixed value of 41kand for various
values of the reaction/diffusion parameter 3
k.
sponds to changes in electrode size. Further, based on
the outcome of this work it is possible to calculate the
concentration and current at cylindrical and hemispheri-
cal electrode for CE mechanism.
REFERENCES
[1] Fleischmann, M., Pons, S., Rolison, D. and Schmit, P.P.
(1987) Ultra microelectrodes. Data Systems & Technol-
ogy, Inc., Morganton, NC.
[2] Oldham, K.B. (1991) Steady-state microelectrode volt-
ammetry as a route tohomogeneous kinetics. Journal of
Electroanalytical Chemistry, 313, 225-236.
[3] Lavagnini, I., Pastore, P. and Magno, F. (1993) Digital
simulation of steady-state and non-steady state voltom-
metric responses for electrochemical reactions occurring
at an invalid microdisc electrode: Application to ECirr ,
CE first-order reactions. Journal of Electroanalytical
Chemistry, 358, 193-200.
[4] Fleischmann, M., Pletcher, D., Denuault, G., Daschbach,
J. and Pons, S. (1989) The behaviour of microdisk and
microring electrodes predictions of the chronoam-
perometric response of microdisks and of the steady state
for CE and EC catalytic reactions by application of
Neumann’s integral theorem. Journal of Electroanalyti-
cal Chemistry, 263, 225-236.
[5] Streeter, I. and Compton, R.G. (2008) Numerical simula-
tion of the limiting current for the CE mechanism. Jour-
nal of Electroanalytical Chemistry, 615, 154-158.
[6] Ghori, Q.K., Ahmed, M. and Siddiqui, A.M. (2007) Ap-
plication of He’s Homotopy Perturbation Method to Su-
mudu Transform. International Journal of Nonlinear
Sciences and Numerical Simulation, 8, 179-184.
[7] Ozis, T. and Yildirim, A. (2007) Numerical Simulation of
Chua’s Circuit Oriented to Circuit Synthesis. Interna-
tional Journal of Nonlinear Sciences and Numerical
Simulation, 8, 243- 248.
[8] Li, S.J. and Liu, Y.X. (2006) A Multi-component Matrix
Loop Algebra and its Application. International Journal
of Nonlinear Sciences and Numerical Simulation, 7, 177-
182.
[9] Mousa, M.M. and Ragab, S.F. (2008) Soliton switching
in fiber coupler with periodically modulated dispersion,
coupling constant dispersion and cubic quintic nonlinear-
ity. Zeitschrift für Naturforschung, 63 140-144.
[10] He, J.H. (1999) Homotopy perturbation technique.
Computer Methods in Applied Mechanics and Engineer-
ing, 178, 257-262.
[11] He, J.H. (2003) Homotopy perturbation method: a new
nonlinear analytical technique. Applied Mathematics and
Computation, 135, 73-79.
[12] He, J.H. (2003) A Simple perturbation approach to
blasius equation. Applied Mathematics and Computation,
140, 217-222.
[13] He, J.H. (2006) Homotopy perturbation method for solv-
ing boundary value problems. Physics Letters A, 350,
87-88.
[14] Golbabai, A. and Keramati, B. (2008) Modified homo-
topy perturbation method for solving Fredholm integral
equations. Chaos solitons Fractals, 37, 1528.
[15] Ghasemi, M., Kajani, M.T. and Babolian, E. (2007) Nu-

V. M. R. M. PonRani et al. / Natural Science 2 (2010) 1318-1325 1324
merical solutions of the nonlinear Volterra-Fredholm in-
tegral equations by using homotopy perturbation method.
Journal of Applied Mathematics and Computing, 188,
446-449.
[16] Biazar, J. and Ghazvini, H. (2009) He’s homotopy per-
turbation method for solving system of volterra integral
equations of the second kind. Chaos solitons fractals, 39,
770.
[17] Odibat, Z. and Momani, S. (2007) A reliable treatment of
homotopy perturbation method Klein-Gordon equations.
Physics Letters A, 365, 351-357.
[18] Chowdhury, M.S.H. and Hashim, I. (2007) Solutions of
time-dependent Emden-Fowler type equations by homo-
topy perturbation method. Physics Letters A, 368,
305-313.
[19] He, J.H. (2006) Some asymptotic methods for strongly
nonlinear equations. International Journal of Modern
Physics B, 20, 1141-1199.
[20] Donald, A.P. (2010) Homotopy perturbation method and
the natural convection flow of a third grade fluid through
a circular tube. Nonlinear Science Letters A, 1, 43-52.
[21] Ganji, D.D. and Rafei, M. (2006) Solitary wave solutions
for a generalized Hirota-Satsuma coupled KdV equation
by homotopy perturbation method. Physics Letters A,
356, 131-137.
[22] He, J.H. (2008) A elementary introduction to recently
developed asymptotic methods and nanomechanics in
textile engineering. International Journal of Modern
Physics B, 22, 3487-3578.
APPENDIX A
Solution of the Eqs 9 and 10 using Homotopy pertur-
bation method.
In this Appendix, we have used Homotopy perturba-
tion method to solve Eqs.9 and 10. Furthermore, a
Homotopy was constructed to determine the solution of
Eqs.9 and 10. Taking laplace transform Eqs.9 and 10 we
have
22
12
22
(1) 10
du du
psupkukvsu
dX dX
 

 
 
(A1)
22
34
22
(1) 10
dv dv
psvpkukvsv
dX dX
 

 
 
(A2)
The boundary conditions are
12
1; ;
mm
Xu v
s
s
  (A3)
; 1; 1Xuv (A4)
The approximate solutions of Eqs.A1 and A2 are
23
01 2 3
.......uupu pupu  (A5)
23
01 2 3
..........vvpvpvpv  (A6)
Substituting Eqs. A5 and A6 into Eqs.A1 and A2 and
comparing the coefficients of like powers of p
20
00
2
: 0
du
psu
dx  (A7)
21
1100
12
2
: 10
du
psuukv
dX


 (A8)
20
00
2
: 0
dv
psv
dX  (A9)
21
1100
34
2
: 10
dv
psvkukv
dX

 (A10)
Solving the Eqs. A7 to A10, and using the boundary
conditions (A3) and (A4), we can find the following
results

1
1
0(,) sX
m
uXs e
s

 (A11)
 


1
11 1
112 2
32 32
(,)
11
1
22
s
XsX sx
uXs
kmk m
eeXe
ss ss




 


(A12)
and

1
2
0(,) sX
m
vXs e
s

 (A13)
 


1
11 1
31 42
32 32
(,)
11
1
22
s
XsX sx
vXs
km km
eeXe
ss
ss




 


(A14)
According to the Homotopy perturbation method, we
can conclude that
001
1
( )lim()..........
p
uXuXuu

 (A15)
01
01
( )lim().........
p
vXvXvv

 (A16)
After putting Eqs.A11 and A12 into Eq.A15 and
Eqs.A13 and A14 into Eq.A16. Using inverse Laplace
transform, the final results can be described in Eqs.19
and 20 in the text. The remaining components of


n
ux
and ()
n
vx
be completely determined such that each
term is determined the previous terms.

V. M. R. M. PonRani et al. / Natural Science 2 (2010) 1318-1325 1325
APPENDIX B: NOMENCLATURE AND UNITS
Symbol Meaning Usual dimension
a Concentration of the species A mole cm-3
b Concentration of the species B mole cm-3
0
a Bulk concentration of the species A mole cm-3
0
b Bulk concentration of the species B mole cm-3
l Thickness of the planar electrode cm
f
 Forward rate constant sec-1
b
 Backward rate constant sec-1
a
D
Diffusion coefficient of the species A cm2sec-1
b
D
Diffusion coefficient of the species B cm2sec-1
j Flux of the species mole cm-2 sec-1
F Faraday constant C
n Number of electrons None
t Time sec
u Normalized concentration of the species A None
v Normalized concentration of the species B None
J
Dimensionless flux None
T Dimensionless time None
123
4
,,
and
kkk
k Dimensionless rate constants None
1
m2 m Constant None

Dimensional current None