Symmetry Reduction and Explicit Solutions of the (2 + 1)-Dimensional DLW Equation ()
1. Introduction
Soliton theory, one of the typical topics in nonlinear science, has been widely applied in optics of nonlinear media, photonics, plasmas, mean-field theory of Bose-Einstein condensates, condensed matter physics, and many other fields. For describing these nonlinear physical phenomena, the study of symmetry is a very important approach, especially in the integrable nonlinear partial differential equations (NPDEs) for the sake of the existence of symmetries in infinity. Traditionally, there are three powerful methods to find the symmetry structure of the NPDEs, that is, the Lie group method of infinitesimal transformations, the nonclassical Lie group method and the Clarkson and Kruskal (CK) direct method [1] - [5] . Among them, the classical Lie symmetries of the partial differential equations (PDEs) can be obtained through the Lie group method of infinitesimal transformations. Using the basic prolongation method and the infinitesimal criterion of invariance, one can find some particular Lie point symmetries group of the NPDEs.
In Section 2 of this paper, a (2 + 1)-dimensional dispersive long wave (DLW) equation is taken to illustrate the symmetry reduction related the CK direct method. Section 3 is a direct result which the explicit reduction solutions are solved and the soliton excitations are depicted. Section 4 is the conclusion.
2. Symmetry Structure through the Direct Approach
In the following of this paper, we fucus on the (2 + 1)-dimensional dispersive long wave (DLW) equation
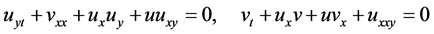(1)
The system was first derived by Boiti et al. as a compatibility for a weak Lax pair [6] . In Ref. [7] , Paquin and Winternitz showed that the symmetry algebra of Equation (1) is infinite-dimensional and a Kac-Moody-Virasoro structure. The more general symmetry algebra,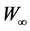 
symmetry algebra, was given in Ref. [8] . In Ref. [9] , Lou gave out nine types of two dimensional similarity reductions and thirteen types of ordinary differential equation reductions. In Ref. [10] , Lou showed that Equation (1) has no Painléve property, though the system is Lax or 1ST integrable. Abundant propagating localized excitations were also derived by Lou [11] [12] with the help of Painlvé-Bäcklund transformation and a multilinear variable separation approach. With the aid of a projective Riccati equation approach and by introducing appropriate lower-dimensional localized patterns, abundant coherent soliton excitations, that is, solitons, chaos and fractals were derived by ours [13] - [15] .
According to the Clarkson and Kruskal (CK) direct method [4] [5] , we first seek the similarity reduction of Equation (1) in the form of
(2)
where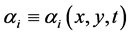
, 
and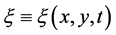
, 
, 
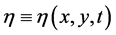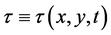are all
differentiable functions to be determined, 
, 
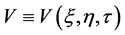satisfy the following DLW equation
as Equation (1)
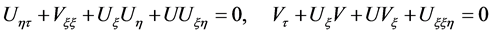(3)
The result of the symbolic computation, one can deduce
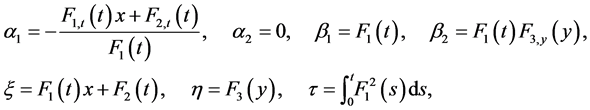(4)
where 
and 
are arbitrary functions of their own variables.
Utilizing the variable separation solution of Equation (3) which derived in Refs. [13] [14]
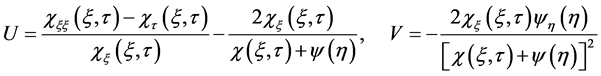(5)
the similarity solution of Equation (1) can be written
![]()
(6)
where ![]()
and ![]()
are two arbitrary variable separation functions of ![]()
and![]()
, respectively.
Second, under the transformation
![]()
(7)
with the infinitesimal parameter![]()
, we have
![]()
(8)
![]()
(9)
then Equation (2) can reduce to
![]()
(10)
![]()
(11)
![]()
(12)
The equivalent vector expression of the above symmetry is
![]()
(13)
When taking
![]()
(14)
from Equation (13), the following six operators are obtained
![]()
(15)
Hence, we obtain the commutator table listed in Table 1 with the ![]()
-th entry indicating ![]()
according to the commutator operators![]()
.
3. Reduction Solutions
Solving the following characteristic equations
![]()
(16)
with![]()
, ![]()
, ![]()
, ![]()
and
![]()
, we can obtain four types of similarity reductions of Equation (1).
1) Taking ![]()
we get the similarity reduction of Equation (1) through solving Equations (16)
![]()
(17)
where![]()
.
2) Taking ![]()
we get the similarity reduction of Equation (1) through solving Equations (16)
![]()
(18)
where ![]()
3) Taking ![]()
we get the similarity reduction of Equation (1) through solving
Equations (16)
![]()
(19)
4) Taking ![]()
we get the similarity reduction of Equation (1) through solving Equations (16)
![]()
(20)
As we all know, to derive soliton structures of a explicit solution is a meaningful task fo a nonlinear physical
equation. Now, when taking ![]()
(![]()
is a solution in Equation (17)), a fundmental soliton, that is a
dromion-like structure, is found if the constants![]()
, ![]()
, the function
![]()
and the fixed time ![]()
(Figure 1(a)). A further considering is, when the cons- tants ![]()
and the function![]()
,![]()
. For this time, a typical line soliton is derived for the fixed time ![]()
(Figure 1(b)).
The solitoff is another special type of coherernt structure for a nonlinear equation, where the wave fields
decays exponentially in all directions except for a preferred direction [16] . For the field![]()
, the solitoff
structure can also be constructed. Figure 2(a) shows a two-solitoff solution when taking the constants
![]()
, ![]()
, the function ![]()
and
![]()
. After adjusting the constants ![]()
and the
function![]()
, the field![]()
, the periodic dromion
solitons is depicted (Figure 2(b)).
4. Summary and Conclusion
In summary, we have obtained the symmetry reduction with the aid of the CK direct method and some explicit solutions through solving the characteristic equations of the (2 + 1)-dimensional DLW equation. These obtained solutions contain several free functions of variables ![]()
and![]()
, which provide us with more chose of these functions to generate the abundant soliton structures. Here, we chose several types of elementary functions to exhibit these soliton propagations related to the obtained solutions. These solutions may provide more infor- mation to further study the nonlinear physical system.
Acknowledgements
The authors are grateful to Profs Lou S. Y. and Chen Y. and Drs Xin X. P. and Hu X. X. for their helpful suggestions and fruitful discussion.
Funding
Supported by the Natural Science Foundation of Zhejiang Province, China under Grant Nos. LY14A010005 and LQ13A010013.