Fractional Optical Solitons and Fractional Noether’s Theorem with Ortigueira’s Centered Derivatives

Jorge Fujioka¹, Manuel Velasco¹, Argel Ramírez²

¹Instituto de Física, Departamento De Física-Química, Universidad Nacional Autónoma de México, México D.F., México.
¹nstituto de Física, Departamento De Física-Química, Universidad Nacional Autónoma de México, México D.F., México.
²Centro de Ciencias de la Atmósfera, Universidad Nacional Autónoma de México, México D.F., México.

DOI: 10.4236/am.2016.712118                

Abstract

This paper shows that the centered fractional derivatives introduced by Manuel Duarte Ortigueira in 2006 are useful in the description of optical solitons. It is shown that we can construct a fractional extension of the nonlinear Schrödinger (NLS) equation which incorporates Ortigueira’s derivatives and has soliton solutions. It is also shown that this fractional NLS equation has a Lagrangian density and can be derived from a variational principle. Finally, a fractional extension of Noether’s theorem is formulated to determine the conserved quantities associated to the invariances of the action integral under infinitesimal transformations.

Keywords

Fractional Derivatives, Centered Derivatives, Noether’s Theorem, Ortigueira, Optical Solitons

Received 20 May 2016; accepted 23 July 2016; published 26 July 2016

1. Introduction

In 2010, it was found that the famous nonlinear Schrödinger (NLS) equation:

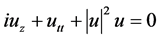, (1)

which occupies a central role in the study of light pulses propagating in optical fibers, has a fractional extension which has soliton-like solutions [1] . The existence of this fractional NLS equation is, to our knowledge, the first contact between fractional calculus and the theory of optical solitons. In Equation (1) z represents the distance along an optical fiber, t is the so-called retarded time, and 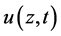is the envelope of the electric field of a laser beam. In this context, the evolution variable is not the time, but the distance z along the fiber, and consequently the initial condition for Equation (1) is defined by the function.

Equation (1) is adequate to describe optical pulses when the power transmitted along the fiber is low (a few milliwatts), and the width of the pulses is in the range of a few picoseconds. However, when the pulses are shorter and the power is higher, the NLS equation has to be modified by adding higher-order dispersive and nonlinear terms, as in the equation:

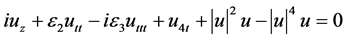(2)

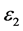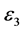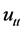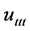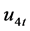where and are real constants whose values depend on the frequency of the laser, and, and are the second, third and fourth partial derivatives of u with respect to time. In 2003 [2] , it was found that this equation had exact solitons of a peculiar type, known as embedded solitons [3] - [7] , and from the results found in [2] it followed that exact soliton solutions also existed in the following equations:

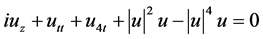(3)

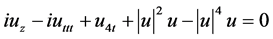(4)

The existence of exact solitons in these two equations suggests that perhaps soliton solutions may also exist in a fractional equation of the form:

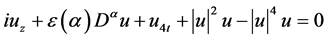(5)

where α is a real number in the interval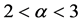, 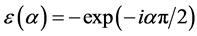is a function satisfying the boundary conditions 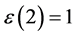and, and is a fractional derivative. The rationale which leads to the form of the function is explained in [1] . As in the context of light pulses propagating in optical fibers, the time is not the evolution variable, there is no reason to privilege left-sided fractional derivatives over right- sided ones, and consequently in [1] it is decided to introduce in Equation (5) the following fractional derivative:

(6)

where and are, respectively, the left and right Grünwald-Letnikov derivatives [8] - [11] :

(7)

(8)

and the coefficient which appears in (6) in front of the right-sided derivative is the extrapolation to the fractional case of the coefficient which appears in the finite difference approximation to the n-th derivative of a function when forward differences are used. Later on, in [1] , it is shown that this factor is indeed necessary in order to maintain the conservation of energy.

Unexpectedly, the results found in [1] show that Equation (5) [with the fractional derivative defined in (6)] does not have soliton solutions. However, it is also found that soliton solutions do exist if we add an additional nonlinear term of the form. More precisely, it is found that the equation:

(9)

does indeed have stable soliton solutions.

We can see that the derivative defined in (6) can be considered as an alternative to define a centered fractional derivative, as it combines left- and right-sided Grünwald-Letnikov derivatives. However, other possibilities exist. One of them is the Riesz derivative [12] :

(10)

where and are the left and right Riemann-Liouville derivatives. This derivative has been used, for example, to construct fractional generalizations of the Fokker-Planck equation in statistical mechanics [13] . Another interesting possibility has been proposed by M. Duarte Ortigueira, who defined the following two centered fractional derivatives [14] [15] :

(11)

(12)

The first of these derivatives is adequate when α is close to an even integer, and it is called “type 1” fractional centered derivative in Ortigueira’s papers. The second one is the “type 2” centered derivative, and it is appropriate when α is closer to an odd integer.

In the present communication we investigate if it is possible to replace the integer-order derivatives and which appear in Equation (2) by the centered fractional derivatives (11) and (12), in such a way that the resulting fractional equation admits the propagation of soliton-like solitary waves. As we shall see in this paper, this fractional generalization of Equation (2) indeed exists. Then, we will show that it is possible to obtain this new fractional equation from a variational principle. To this end we begin by constructing a fractional extension of the least action principle which can be applied to Lagrangian densities which involve Ortigueira’s centered fractional derivatives, and then we determine a suitable Lagrangian for our fractional generalization of Equation (2). Once with this Lagrangian, we will show that it is possible to formulate a generalized Noether’s theorem which applies to this type of Lagrangians (with centered fractional derivatives).

The paper is structured as follows: in Section 2 we show that it is indeed possible to replace the integer-order derivatives which appear in Equation (2) by a linear combination of Ortigueira’s centered derivatives (11) and (12), and the resulting equation admits the propagation of soliton-like solutions. In Section 3 we obtain a generalized version of the least action principle which can be applied to Lagrangian densities which involve Ortigueira’s centered derivatives. We will see the form of the Euler-Lagrange equations corresponding to these fractional Lagrangians, and we will determine a suitable Lagrangian which lead us to the fractional extension of Equation (2) found in Section 2. In Section 4, we formulate a generalized Noether’s theorem that can be applied to Lagrangians that contain Ortigueira’s centered derivatives. Then, by means of this theorem, we show that our fractional extension of Equation (2) conserves the energy, the momentum and the Hamiltonian. Finally, in Section 5, we present our conclusions.

2. Fractional generalization of Equation (2)

To begin this section, we should mention that Equations (11) and (12) were obtained by extrapolating the finite difference approximation for the n-th derivative of f(t) with centered differences. However, a careful derivation of these extrapolations shows that in Equation (11) a factor should appear, and Equation (12) should contain a factor. These two complex factors are not contained in the definitions (11) and (12) of Ortigueira’s fractional derivatives, but it is not evident why these factors have been dropped. An obvious advantage of dropping these factors is that the expressions (11) and (12) have real values if the function is real. However, when we deal with equations whose dependent variable is a complex function, it is not clear if these factors should indeed be discarded. In these cases [when is complex] we might use the complex centered fractional derivatives:

(13)

(14)

which shall be called “even” and “odd” centered fractional derivatives from now on.

Now, let us focus our attention on our first goal: to find out if it is possible to generalize Equation (2) by replacing the integer-order derivatives and by centered fractional ones. Since the variable in Equation (2) is a complex function, it is not evident if we should replace and by Ortigueira’s centered derivatives (11)-(12), or if we should use the expressions (13)-(14). To find out which derivatives [(11)-(12) or (13)-(14)] are more adequate to describe the dispersion of optical pulses, we begin by comparing the solutions of the following linear equations:

(15)

(16)

In Figure 1, we can see the evolution of the solution of Equation (15) with corresponding to an initial condition of the form:

(17)

where and. The behavior of the solution that we see in Figure 1 is reasonable: the pulse is slowly dispersed, as we could have anticipated. On the other hand, if we calculate the solution of Equation (16) for the same initial condition (17), we find that the pulse diverges (i.e. its amplitude grows enormously). Consequently, it is now clear that Ortigueira’s type 1 derivative (11) is more adequate to describe the dispersion of optical pulses than the derivative defined in (13).

In a similar way, we can now compare the solutions of the equations:

(18)

(19)

with and the same initial condition (17). In Figure 2, we can see how the solution of (18) evolves. We

Figure 1. Solution of Equation (15) with α = 2.1 and the initial condition (17). The temporal profile of the solution is shown for z = 0, 8, 16, 24, 36 and 40.

Figure 2. Solution of Equation (18) with α = 2.9 and the initial condition (17). The temporal profile of the solution is shown for z = 0, 8, 16, 24, 36 and 40.

can see that the pulse slowly disperses, and it moves along the t axis, which is a consequence of being near to 3 (we know that the effect of a third derivative would be to move the pulse). On the other hand, the solution of Equation (19) with the initial condition (17) diverges. Consequently, when is closer to 3 Ortigueira’s derivative (12) is a better option to describe the dispersion of optical pulses than the derivative (14).

It is worth observing that the divergence of the solutions of Equations (16) and (19) might be considered as a posteriori proof that Ortigueira’s decision of eliminating the complex factors and from the definitions (11) and (12) was a correct one.

There is, however, a small discrepancy that has to be clarified. In Figure 2 we can see that the pulse advances to the left, while we expected that the movement would be to the right (as this would be the effect of the term). This discrepancy is due to the fact that:

(20)

Therefore, if we want to generalize Equation (2) by including centered fractional derivatives, we should replace by (i.e. we must add a minus sign). In a similar way, it is known that:

(21)

and consequently should be replaced by.

The above results seem to imply that a reasonable fractional generalization of Equation (2) would be:

(22)

But we can improve this equation by introducing weight factors in front of the fractional derivatives. We desire that the influence of the type 1 derivative diminishes as moves from 2 to 3, while, at the same time, the importance of the type 2 derivative should increase. This effect may be accomplished by introducing a factor:

(23)

in front of the type 1 derivative, and a factor:

(24)

in front of the type 2 derivative. Therefore, a good candidate to generalize Equation (2) to fractional orders seems to be:

(25)

To find out if this equation has soliton-like solutions we will solve it numerically with an initial condition that has a chance to be near to a soliton. And a promising initial condition could the exact soliton solution of Equation (2), which has the form [2] :

(26)

where:

(27)

(28)

(29)

(30)

(31)

Therefore, in this solution has the form:

(32)

and this is the initial condition that we will use to solve Equation (25).

In Figure 3, we can see the solution of Equation (25) for and. We can observe that at the beginning the pulse’s amplitude decreases, but then it stabilizes, and the pulse propagates without being dispersed away. And similar solutions are obtained with and. Therefore, Equation (25) is indeed a fractional generalization of Equation (2) which accepts soliton propagation.

We should observe, however, that the evolution of the initial condition (32) is different if. In Figure 4, for example, we can observe the solution (at various values of z) corresponding to. We can see that the pulse tends to split in two pulses, then it returns to a single-hump profile, and then the process repeats again. This behavior is reminiscent of that of the third-order soliton of the standard NLS equation [16] [17] , and therefore the possibility that higher-order fractional solitons might exist in Equation (25) is an issue that might deserve further studies in the future.

It is worth remembering that in the case of Equation (9) it was necessary to include the nonlinear term in order to have soliton solutions [1] . However, in the case of Equation (25) this nonlinear term is not necessary. In fact, the numerical solution of the equation:

(33)

shows that the initial condition (32) is dispersed away quite rapidly if, 2.3, 2.5 and 2.7. In Figure 5, we

Figure 3. Solution of Equation (25) with, and the initial condition defined by the Equations (27), (28) and (32). The temporal profile of the solution is shown for z = 0, 16, 32, 48, 72 and 80.

Figure 4. Solution of Equation (25) with, and the initial condition defined by the Equations (27), (28) and (32). The temporal profile of the solution is shown for z = 0, 16, 32, 48, 72 and 80.

Figure 5. Solution of Equation (33) with, and the initial condition defined by the Equations (27), (28) and (32). The temporal profile of the solution is shown for z = 0, 16, 32, 48, 72 and 80.

can see the evolution of the pulse when. Similar results are obtained with, 2.3 and 2.7.

The fact that Equation (25) does not require the nonlinear term to have soliton solutions seems to imply that the dispersion of optical pulses is better described with Ortigueira’s centered derivatives, than using the sum of left and right Grünwald-Letnikov derivatives used in [2] and shown in Equation (6).

To close this section we would like to observe that in Equations (9) and (25) we put the coefficients of, and equal to one just to simplify the exposition. However, it is known that Equation (25) has exact soliton solutions when these coefficients take values, and different from one (see [2] ). In the same way, it is expected that Equation (9) will also accept soliton solutions when we allow these coefficients to have different values. However, to determine the precise boundaries which define the regions in the space of parameters where Equation (9) has soliton solutions is a task which requires extensive numerical calculations, and it lies outside the scope of the present work.

3. Fractional Euler-Lagrange Equation

Now, let us investigate if it is possible to formulate a generalized least action principle which applies to Lagrangian densities which involve Ortigueira’s centered fractional derivatives. Therefore, let us begin by supposing that we have a functional (that we shall call “action”, as usual) defined as follows:

(34)

where is a complex function, z and t are real variables, and are Ortigueira’s derivatives of types 1 and 2, respectively, and the value of the integrand (the Lagrangian density) is real.

Once with our action integral, we would like to obtain the conditions that the function must satisfy in order that the action integral attains an extremum. For this to occur it is necessary that, and this condition implies that:

(35)

where the variation of the Lagrangian is given by:

(36)

Now, in order to obtain a fractional differential equation from the condition (35), it is necessary to rearrange the integrand in (35) in such a way that each of its terms contains a factor or, and for this to happen it is necessary to integrate by parts the terms containing, , , and (and similar terms with instead of u). The integration by parts of the terms containing, and is a stan-

dard calculation, but the integration of the terms containing and requires the new Parseval relations:

(37)

(38)

which can be obtained directly from the definitions of the derivatives and. These equations are similar to the known equation [18] :

(39)

where and are the left and right Riemann-Liouville fractional derivatives. However, it should be observed that Equation (39) involves both Riemann-Liouville derivatives (left-handed and right-handed), while in Equation (37) only type 1 derivatives appear, and in Equation (38) only type 2 derivatives occur. More- over, there is an unexpected asymmetry between Equations (37) and (38) due to the minus sign that appears in Equation (38).As we shall see in the following, the fact that Ortigueira’s derivatives satisfy the Parseval relations (37) and (38) is absolutely essential in order to obtain the Euler-Lagrange equations that u and must satisfy to guarantee that the action integral (34) attains an extremum.

Using the Parseval relations (37) and (38) we can integrate by parts all the terms in the integrand of Equation (35) and then, collecting the terms which contain (and those containing), it follows that Equation (35) implies that:

(40)

and a similar equation holds with instead of u. These are the Euler-Lagrange equations corresponding to a fractional Lagrangian density which involves Ortigueira’s centered fractional derivatives.

If we now consider the Lagrangian density:

(41)

and we substitute it into Equation (40), we obtain Equation (25). Therefore, the fractional equation (25), in addition of having soliton-like solutions, can be obtained from the least action principle using the Lagrangian density (41).

4. Fractional Noether’s Theorem

Noether’s theorem states that if the action integral is invariant under an infinitesimal transformation, then a conservation law exists. In the following we will investigate if this theorem also holds when we have action integrals which involve Lagrangian densities which depend on integer-order derivatives and also on centered fractional ones.

In this communication, we will only consider infinitesimal transformations of the form:

(42)

(43)

(44)

(45)

where and are just real constants and is the parameter of the transformation. Having these transformations, we can define:

(46)

(47)

and from these equations it follows that:

(48)

(49)

Now, in order to arrive at Noether’s theorem, it is necessary to substitute, , Equations (48) and (49), and the Euler-Lagrange Equation (40) (and its counterpart with instead of u), into the variation of the Lagrangian:

(50)

It should be noticed that Equation (50) differs from (36) because the first two terms on the r.h.s of (50) did not appear in Equation (36). In the derivation of the Euler-Lagrange equations from the least action principle, only the functions and (and their derivatives) are varied, while in the infinitesimal transformation (42)-(45) also the independent variables z and t are varied. This is the reason for including the first two terms on the r.h.s of Equation (50).

Once we have substituted, , Equations (48)-(49) and the Euler-Lagrange equations into, we impose the condition, and a lengthy algebraic exercise shows that this condition can be rewritten in the form:

(51)

where we have defined:

(52)

(53)

(54)

The form of Equation (51) is interesting because it does not have the form of a conservation law due to the presence of the last term (the term P). This term disappears when the Lagrangian density does not contain the fractional derivatives, , and, and in that case Equation (51)reduces to the usual form of a conservation law. It should be noticed that if the parameter (the order of the fractional derivatives) takes an integer value, the fractional derivatives become standard (integer-order) derivatives, and the term P disappears. In that case (when takes an integer value) the Lagrangian changes (as the fractional derivatives are replaced by standard ones), and the problem is reduced to a standard one, with a Lagrangian depending on u, and integer-order derivatives of these functions. Therefore, in this case, the standard Noether’s theorem applies, and the requirement leads to a standard conservation law (without the term P).

We should now observe that even when the term P is present in Equation (51), this equation may imply the existence of a conserved quantity, because when we integrate this equation over t (from to), the integral of P turns out to be zero, due to the Equations (37)-(38), and the integral of Equation (51)reduces to:

(55)

and consequently for any solution which satisfies the boundary condition:

(56)

there is a conserved quantity since Equation (55)reduces to:

(57)

Therefore we have the following fractional extension of Noether’s theorem:

If we have a fractional partial differential equation which can be obtained from a Lagrangian density which depends on two functions, and its derivatives, , , , , , , and:

1), where is defined by Equation (50), and the quantities, , and which enter in can be obtained from the infinitesimal transformation (42)-(45).

2) The condition (56) is satisfied [where is defined by Equation (53)], then Equation (57) holds [where is defined by Equation (52)], and therefore a conserved quantity exists.

It should be observed that other fractional generalizations of Noether’s theorem have been formulated in the past [19] - [24] , but none of them is applicable to Lagrangian densities which involve Ortigueira’s centered derivatives.

Now, we will apply the theorem presented above to determine the conserved quantities associated with three infinitesimal transformations. The first one is a infinitesimal gauge transformation:

(58)

(59)

(60)

(61)

It can be verified that the action integral associated to the Lagrangian density shown in Equation (41) is invariant under this transformation (i.e.). Moreover, any function which tends to zero as will satisfy the condition (56), and consequently Noether’s theorem tells us that:

(62)

In other words: the invariance of the action under a gauge transformation implies that the energy of the pulse is conserved.

As a second example we can consider the following infinitesimal transformation:

(63)

(64)

(65)

(66)

A straightforward calculation shows that also in this case we have, and the condition (56)is satisfied by any solution of Equation (25) which tends to zero as. Consequently Noether’s theorem can be applied, and it implies that there is a conservation law of the following form:

(67)

If we now substitute the Lagrangian (41) in this equation, it reduces to:

(68)

where:

(69)

is the Hamiltonian density corresponding to the Lagrangian given in (41). It is worth mentioning that this Hamiltonian does not contain the term, which appears in the Hamiltonian of the NLS equation, because (69)is the Hamiltonian associated to the Lagrangian (41) [which corresponds to Equation (25)], and this Lagrangian does not contain the first derivatives and.

It may be a surprise that the conservation of the Hamiltonian is a consequence of the invariance of the action integral under translations in z. We are used to think that the Hamiltonian conservation is associated to invariances under time translations. However, we must remember that in the context of soliton propagation in optical fibers, the evolution variable is the spatial coordinate z, and therefore, in this context,z plays the same role that is usually played by the time in mechanical problems. This is the reason for the Hamiltonian conservation to be associated to translations in z.

As a third example we can consider a time translation:

(70)

(71)

(72)

(73)

A direct calculation shows that also in this case the variation of the Lagrangian (41) associated to this transformation vanishes (i.e.). Moreover the condition (56) is also satisfied by any function which tends to zero as. Therefore, Noether’s theorem can be applied, and it implies that the following conservation law holds:

(74)

It is worth observing that this conservation law also holds in the case of the standard NLS equation [17] , where it is frequently referred at as “momentum conservation”.

5. Conclusions and Final Remarks

In this communication, we show that there exists a fractional generalization of the NLS equation [Equation (25)] which admits soliton-like solutions, and employs Ortigueira’s centered fractional derivatives [Equations (11)- (12)] to describe the dispersion of light pulses travelling along an optical fiber. It is found that Ortigueira’s centered derivatives are more adequate to describe the dispersion of optical pulses than the Grünwald-Letnikov derivatives used in [1] , since in Equation (25) it is not necessary to include additional nonlinear terms in order to have soliton solutions. We also show that this fractional NLS equation can be deduced from a variational principle, and in order to do so we show that the least action principle can be applied to Lagrangian densities which contain Ortigueira’s centered derivatives. We show that when we have this type of Lagrangians the Euler- Lagrange equations take the form (40), and to obtain these equations it is essential to prove that Ortigueira’s centered derivatives satisfy the Parseval relations (37) and (38). Then, we show that it is possible to formulate a fractional extension of Noether’s theorem which is applicable to Lagrangians which contain Ortigueira’s centered derivatives. We demonstrate this theorem in the particular case of infinitesimal transformations of the forms (42)-(45), which are the only type of transformations considered in the study of optical solitons. Finally, using this fractional Noether’s theorem, we prove that the action integral associated to Equation (25) [and its Lagrangian (41)] is invariant under gauge transformations, and z and t translations, and as a consequence of these invariances, the solutions of Equation (25) conserve the energy, the Hamiltonian and the momentum.

Therefore, we have seen that Ortigueira’s centered fractional derivatives can be incorporated in a generalized NLS equation [Equation (25)] which describes the propagation of light pulses in optical fibers, and this new fractional equation has the following five characteristics:

a) It is an interesting physical model.

b) It has soliton solutions (fractional optical solitons).

c) It is superior to other models which accept fractional optical solitons because Equation (25) does not require additional nonlinear terms to describe the propagation of solitons.

d) It can be obtained from a Lagrangian density, via the least action principle.

e) Some of its conserved quantities can be obtained by means of a generalized fractional Noether’s theorem.

It is worth mentioning that Ortigueira has recently proposed a new unified centered fractional derivative [25] which combines, in a way, the centered derivatives (11) and (12). An interesting topic for future work might be to study an equation similar to Equation (25), but replacing the terms:

with this unified centered derivative, in order to find out if the resulting equation admits soliton solutions and can be derived from a variational principle.

As a final remark we would like to add that the theory of optical solitons is not only related to the fractional derivatives and the fractional calculus (as we have seen in this paper), but also to the concept of fractional dimensions [26] . It might be a topic for future work to study if the propagation of solitons may be related simultaneously to fractional derivatives and fractional dimensions.

Acknowledgements

We thank DGTIC-UNAM (Dirección General de Cómputo y de Tecnologías de Información y Comunicación de la Universidad Nacional Autónoma de México) for granting us access to the computer Miztli through the Project SC16-1-S-6, in order to carry out this work.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Fujioka, J., Espinosa, A. and Rodriguez, R.F. (2010) Fractional Optical Solitons. Physics Letters A, 374, 1126-1134. http://dx.doi.org/10.1016/j.physleta.2009.12.051
[2]	Espinosa-Cerón, A., Fujioka, J. and Gómez-Rodríguez, A. (2003) Embedded Solitons: Four-Frequency Radiation, Front Propagation and Radiation Inhibition. Physica Scripta, 67, 314-324. http://dx.doi.org/10.1088/0031-8949/67/4/008
[3]	Fujioka, J. and Espinosa, A. (1997) Soliton-Like Solution of an Extended NLS Equation Existing in Resonance with Linear Dispersive Waves. Journal of the Physical Society of Japan, 66, 2601-2607. http://dx.doi.org/10.1143/JPSJ.66.2601
[4]	Yang, J., Malomed, B.A. and Kaup, D.J. (1999) Embedded Solitons in Second-Harmonic Generating Systems. Physical Review Letters, 83, 1958-1961. http://dx.doi.org/10.1103/PhysRevLett.83.1958
[5]	Yang, J., Malomed, B.A., Kaup, D.J. and Champneys, A.R. (2001) Embedded Solitons: A New Type of Solitary Wave. Mathematics and Computers in Simulation, 56, 585-600. http://dx.doi.org/10.1016/S0378-4754(01)00327-5
[6]	Champneys, A.R., Malomed, B.A., Yang, J. and Kaup, D.J. (2001) Embedded Solitons: Solitary Waves in Resonance with the Linear Spectrum. Physica D, 152-153, 340-354. http://dx.doi.org/10.1016/S0167-2789(01)00178-6
[7]	Fujioka, J., Espinosa-Cerón, A. and Rodríguez, R.F. (2006) A Survey of Embedded Solitons. Revista Mexicana de Física, 52, 6-14.
[8]	Samko, S.G., Kilbas, A.A. and Marichev, O.I. (1993) Fractional Integrals and Derivatives: Theory and Applications. Gordon and Breach Science Publishers, Amsterdam.
[9]	Atanackovi?, T.M., Pilipovi?, S., Stankovi?, B. and Zorica, D. (2014) Fractional Calculus with Applications in Mechanics: Wave Propagation, Impact and Variational Principles. Wiley, Hoboken.
[10]	Podlubny, I. (1999) Fractional Differential Equations. Academic Press, San Diego.
[11]	Butzer, P.L. and Westphal, U. (2000) An Introduction to Fractional Calculus. In: Hilfer, R., Ed., Applications of Fractional Calculus in Physics, World Scientific, Singapore City. http://dx.doi.org/10.1142/9789812817747_0001
[12]	Herrman, R. (2014) Fractional Calculus: An Introduction for Physicists. 2nd Edition, World Scientific, Singapore City. http://dx.doi.org/10.1142/8934
[13]	Metzler, R. and Klafter, J. (2004) The Restaurant at the End of the Random Walk: Recent Developments in the Description of Anomalous Transport by Fractional Dynamics. Journal of Physics A, 37, R161-R208. http://dx.doi.org/10.1088/0305-4470/37/31/r01
[14]	Duarte Ortigueira, M. (2006) Riesz Potential Operators and Inverses via Fractional Centred Derivatives. International Journal of Mathematics and MathematicalSciences, 2006, Article ID: 48391, 1-12. http://dx.doi.org/10.1155/ijmms/2006/48391
[15]	Duarte Ortigueira, M. (2008) Fractional Central Differences and Derivatives. Journal of Vibration and Control, 14, 1255-1266. http://dx.doi.org/10.1177/1077546307087453
[16]	Agrawal, G. (2013) Nonlinear Fiber Optics. 5th Edition, Academic Press, Oxford.
[17]	Kivshar, Y.S. and Agrawal, G.P. (2003) Optical Solitons: From Fibers to Photonic Crystals. Academic Press, San Diego.
[18]	Kilbas, A.A., Srivastava, H.M. and Trujillo, J.J. (2006) Theory and Applications of Fractional Differential Equations. Elsevier, Amsterdam.
[19]	Frederico, G.S.F. and Torres, D.F.M. (2008) Fractional Conservation Laws in Optimal Control Theory. Nonlinear Dynamics, 53, 215-222. http://dx.doi.org/10.1007/s11071-007-9309-z
[20]	Frederico, G.S.F. and Torres, D.F.M. (2007) A Formulation of Noether’s Theorem for Fractional Problems of the Calculus of Variations. Journal of Mathematical Analysis and Applications, 334, 834-846. http://dx.doi.org/10.1016/j.jmaa.2007.01.013
[21]	Muslih, S.I. (2010) A Formulation of Noether’s Theorem for Fractional Classical Fields. arXiv:1003.0653v1 [math-ph]
[22]	Frederico, G.S.F. and Torres, D.F.M. (2010) Fractional Noether’s Theorem in the Riesz-Caputo Sense. arXiv:1001.4507v1 [math. OC]
[23]	Fujioka, J. (2010) Lagrangian Structure and Hamiltonian Conservation in Fractional Optical Solitons. Communications in Fractional Calculus, 1, 1-14.
[24]	Fujioka, J., Espinosa, A., Rodríguez, R.F. and Malomed, B.A. (2014) Radiating Subdispersive Fractional Optical Solitons. Chaos, 24, 033121-1/11.
[25]	Ortigueira, M.D. and Trujillo, J.J. (2012) A Unified Approach to Fractional Derivatives. Communications in Nonlinear Science and Numerical Simulation, 17, 5151-5157.
[26]	Borovkova, O.V., Lobanov, V.E. and Malomed, B.A. (2012) Solitons Supported by Singular Spatial Modulation of the Kerr Nonlinearity. Physical Review A, 85, 023845-1/5.