The Property of a Special Type of Exponential Spline Function

Ge Yang^*

North China University of Technology, Beijing, China.

DOI: 10.4236/apm.2015.513074                

Abstract

Approximation theory experienced a long term history. Since 50’ last century, the rise of spline function as well as the advance of calculation promotes the growth of classical approximation theory and makes them develop a profound theory in maths, and application values have shown among the field of scientific calculation and engineering technology and etc. At present, the study of spline function had made a great progress and had a lot of fruits, as for that, the reader could look up the book [1] or [2]. Nevertheless, the research staff pays less attention to exponential spline function, since polynomial spline function is a special case of that, so it is much essential and meaningful for one to explore the nature of exponential spline function.

Keywords

Exponential Spline Function, Interpolation, Error Estimation

1. Introduction

At the beginning, we introduce the definition of exponential spline function. From literature [3] , we could learn

the definition: if function 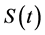satisfies equation, we describe it as exponential

spline function, where L is a differential operator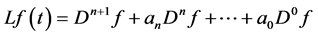. Here,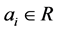 are constant coefficient and represent kth-order derivative. By this definition, we learn that 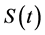exists continuous derivative 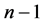and in each interval is linear combination of

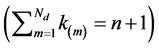
, where the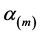’s are the N_d distinct roots of characteristic poly-

nomial and is of order. As exists a single root 0 for characteristic polynomial, 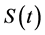is polynomial spline function. Next we will deal with the case of there being unique real root.

2. Main Result

Theorem 1:

If the differential operator’s characteristic polynomial is , where is a root of multiplicity. Then the expression for exponential spline function of this special case is

Proof:

Let be on interval, Suppose

And we have

Since there exists order continuous derivatives for,

Hence

So that

Furthermore, is polynomial of nth degrees.

Therefore

We get,

put

In terms of this idea, we obtain.

Theorem 2: The dimension of the exponential spline function space is.

Proof:

Suppose,

We have

Since

So that is continuous at the knot, hence has order continuous derivatives on interval.

When characteristic polynomial has single real root, the linear space can be written as

Next we prove that is linearly independent

Set On the interval, above equation become, we

have On the interval, we can get, so that, For the interval, By means of the same technique, we can obtain, hence is linearly independent. So that we conclude.

According to theorem 1. 4. 23 of the book [4] , we can prove next conclusion is true.

Corollary: There exists the for every f belonging to, such that

Theorem 3: If condition of interpolation and boundary satisfy:

(1)

then there exist the 3^rd degree exponential spline function satisfied with condition. And we have formula of error evaluation

Proof:

Suppose is 3^rd degree polynomial spline function, let

Hence

Both of them can be denoted by:, , , so that A is invertible matrix.

This lead to (2)

Since, hence, we can get is exponential spline function.

If boundary condition is, , by matrix relation (2), let

and

Since one of 3^rd degree polynomial spline function meet the constraint of interpolation, boundary condition is and.

So that exponential spline function satisfied with condition (1) exists. That is.

Next we prove formula of error evaluation. Suppose, is 3^rd degree exponential spline function satisfied with condition (1).

Let (where is 3^rd degree polynomial spline function)

Since

By formula of error evaluation for 3^rd degree polynomial spline function, we can have

In terms of book [5] , we have

Since

Hence

Furthermore

By above expressions, we can conclude that

.

Fund

Supported partly by National Natural Science Foundation of China (11126140, 11201007) and partly by Beijing Talents Training Program (2011D005002000006) and partly by Science and Technology Development Plan Project of Beijing Education Commission (KM20121000-9013) and partly by Scientific Research Personnel Promotion Plan of North China University of Technology (BJRC201309).

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Li, Y.S. (1983) Spline Function and Interpolation. Shanghai Science and Technology Press, Shanghai.
[2]	Feng, Y.Y., Zeng, F.L. and Deng, J.S. (2013) Spine Function and Approximation Theory. University of Science and Technology of China, Hefei.
[3]	Unser, M. and Blu, T. (2005) Cardinal Exponential Splines: Part I—Theory and Filtering Algorithms. IEEE Transactions on Signal Processing, 53, 1425-1438. http://dx.doi.org/10.1109/TSP.2005.843700
[4]	Zhang, G.Q. and Lin, Y.Q. (1987) Lectures on Functional Analysis. Peking University Press, Beijing.
[5]	Li, Q.Y., Wang, N.C. and Yi, D.Y. (2008) Numerical Analysis. 5th Edition, Tsinghua University Press, Beijing.