On Extensions of Right Symmetric Rings without Identity

Basmah H. Shafee¹, S. Khalid Nauman^2*

¹Department of Mathematics, Umm Al-Qura University, Makkah, KSA.
²Department of Mathematics, King Abdulaziz University, Jeddah, KSA.

DOI: 10.4236/apm.2014.412075                

Abstract

Let us call a ring R (without identity) to be right symmetric if for any triple a,b,c,∈R abc = 0 then acb = 0. Such rings are neither symmetric nor reversible (in general) but are semicommutative. With an idempotent they take care of the sheaf representation as obtained by Lambek. Klein 4-rings and their several generalizations and extensions are proved to be members of such class of rings. An extension obtained is a McCoy ring and its power series ring is also proved to be a McCoy ring.

Keywords

Right (Left) Symmetric Rings, Klein 4-Rings, McCoy Rings

1. Introduction

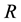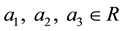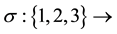
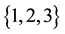
A ring is symmetric if for any triple, then for any permutation 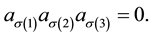J. Lambek in [1] introduced symmetric rings, and got a characterization that a ring 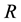with one is symmetric if and only if 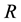contains a subring which is isomorphic to the rings of sections of a sheaf of prime torsion free symmetric rings. Lambek also noticed that the symmetric property is a weaker notion than that of primeness (see [1: p. 362]). The class of symmetric rings lie between the classes of reduced and reversible rings and they have been extensively studied and generalized in various directions, for instance, some references are [2] - [4] , and [5] . Most of the studies on symmetric rings were carried over rings with identity. In this note we assume that rings, in general, are not equipped with the multiplicative identity. Let us say that a ring is right symmetric if for any triple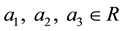, , 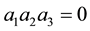then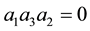. Left symmetric rings are defined analogously. Some concrete examples are given here to show that right (as well as left) symmetric rings are different than symmetric rings. It is observed that, the Lambek criterion about symmetric rings with one, as given in [1] , can be extended to right (or left) symmetric rings with idempotents (Proposition 2.7).

A weaker notion of symmetric is reversible which P.M. Cohn defined in [6] as: a ring 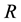is reversible if for any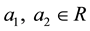, 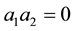implies In [7] , Anderson and Camillo defined that a ring (may not be

with 1) satisfies, if for any, where, the product implies that the product, where, is a permutation. Thus, in their terminology, if a ring satisfies

, it is reversible, and if it satisfies, it is symmetric. They proved that implies, but the converse need not be true in general ([7] ; Example I-4).

For a ring with, clearly, every symmetric ring is reversible, but the converse may not be true, for instance, see ([7] ; Example 1-5). In ([8] : Example 7], Mark proved that the group ring where is the group of quaternions, is reversible but not symmetric. For a ring without one, a symmetric ring may not be reversible. For instance, for any ring A consider the ring of strictly upper triangular matrices Then, , so is symmetric.

On the other hand one sees that if, then, but. Hence is not reversible. Thus by above fairly simple examples we firmly state that, for rings, in general,

In Section 2, after definition, we gave some examples of right symmetric rings which are not symmetric, and developed some interactions with other classes of rings such as von Neuman regular, semicommutative, and Armendariz. In Section 3 we did some extensions of Klein 4-rings and a McCoy ring is constructed in the last section.

2. Right and Left Symmetric Rings

One notices that, in a ring without 1, and with implies that, the commutation only appears on the last two elements. There is no guaranty that, for the support of this claim we provide below some examples. So, let us define that:

Definitions 2.1. A ring is right (respt. left) symmetric if for any triple, , implies that (respt.). is symmetric if is both, left and right symmetric.

Examples 2.2. (1) Klein 4-rings. ([8] : Example 1] Consider the so called Klein-4 ring:, which has two generators a and b, and is a Klein 4-group with respect to addition. Its characteristic is 2 and the relations among its elements are:

Let us consider all possible products of the three non-zero elements of V. There are total products, among them 15 are zero and 12 are non zero. Consider a typical product of three nonzero elements. Then, if either or. This means that. So is right symmetric. If and, then clearly. For instance but. This implies that is not symmetric. (Erroneously it is mentioned in ([8] , Example 1]) that is symmetric). Obviously, there is no question of reversibility as well, as but.

Similarly, the opposite ring, is left symmetric and is neither symmetric nor reversible. Both rings are not reduced also, because is a non-zero nilpotent element.

(2) For any ring define the - -column (respt. row) matrix ring, denoted by (respt., to be a subring, without identity, of the full matrix ring such that it has non-zero elements only in the -column (respt. -row). In fact (respt.) is a left (respt. right) ideal of. Note that, is right symmetric if and only if is right symmetric. Indeed, if we let to be right symmetric and, then

The converse is obvious. Analogously, is left symmetric. Note that, if is symmetric or even a commutative domain or a field, may not be symmetric. For instance, in if

we let, , , then one can easily observe that

but.

Similarly, is left symmetric and is not symmetric.

(3) Let be any domain. Then the direct sum and are right and left symmetric rings, respectively, under component wise addition and multiplication. Similarly, and are right and left symmetric rings, respectively.

(4) In [5] Kwak defined left and right -symmetric rings as follows: Let be an endomorphism on a ring. Then is right (respt. left) -symmetric, if for any triple, , (respt.). Thus right and left symmetric rings are special cases of right and left -symmetric rings, with. It follows immediately from ([5] , Proposition 2.3(2)) that if a reversible ring is left (or right) symmetric, then it is symmetric. Note that in [5] rings are with identity.

There is a symmetry between right and left symmetric rings, because a ring is right symmetric if and only if its opposite ring is left symmetric. So in the following we will only deal with right symmetric rings, left symmetric rings will appear when needed.

A ring R is said to be semicommutative as defined by Bell in [9] , if for any pair, then for all,. There are several names of a semicommutative ring in literature. For historical remarks and other details we refer the reader to [10] . All reduced rings are symmetric and symmetric rings are semicommutative. The ring in Example 2.2. is semicommutative (can be checked easily). A ring R is abelian if every idempotent is central, duo if every right and left ideals are ideals, and reflexive if for any pair, , then. A ring R is von Neumann regular if, there exists an, such that.

A right symmetric ring in general is non abelian, non duo, non reflexive, and not a von Neumann regular ring. We pose quick counter examples for these claims. The Klein 4-ring is right symmetric in which is an idempotent. Because and, so is not central, so V is non abelian. In V, is a right ideal but means that is not an ideal, so V is not right duo. Because but , hence V is not reflexive. Finally, , so V is not von Neumann regular.

It is defined in [11] that a ring with an involution is -reversible, in case for every pair of elements, such that, then.

There are several right symmetric rings without one which are symmetric. For instance, the ring of strictly upper triangular matrices over any ring is without one and is symmetric. Few more cases are given in the following:

Proposition 2.3. (1) Every symmetric ring is right symmetric and every right symmetric ring with one is sym- metric.

(2) Every reduced ring is right symmetric ( [1] : (G); [7] : Theorem I-3). Conversely, a right symmetric ring which is not symmetric cannot be reduced.

(3) Every right symmetric ring is semicommutative.

(4) Every von Neumann regular ring which is right symmetric is symmetric.

(5) Every reversible ring which is right symmetric is symmetric.

(6) Every ring with involution which is right symmetric is symmetric.

(7) Every ring with a reversible involution is right symmetric and hence symmetric.

(8) (1) - (7) all hold if we replace right by left.

Proof: (1) and (5) are obvious.

(2) Let a ring be reduced. Assume that for some, then this means that. Hence is right symmetric.

Conversely, let be right symmetric but not symmetric. Assume that such that and but at least one of cab, cba, bac and bca is not equal to zero. Thus if then. Hence is not reduced. If then, so But then.

(3) Let be a right symmetric ring. Assume that for any pair,. Then for all, abr = 0. Hence, and so is semicommutative.

(4) Assume that is von Neumann regular and is right symmetric. Let be such that. Then for some,. Then or. Thus we conclude that is reduced. Then by (2) is left symmetric, hence symmetric.

(6) Let be a ring with an involution. This means that is an anti-automorphism on of order two. In addition, let be right symmetric. If for some then, because is right symmetric,. Then or that because is right symmetric,. By doubling the involution we get which implies that. Again, gives, and by the doubling of involution we get and so the right symmetry gives.

(7) Let R be a ring with an involution and let be -reversible. Now assume that for some Then which gives or that. By similar techniques we get the remaining permutations equal to zero. So -reversible rings are right and left symmetric, hence symmetric.

(8) holds by left and right symmetry. □

A quick consequence of Proposition 2.3 (6) is the following.

Corollary 2.4. Every right symmetric ring which is not symmetric cannot adhere to an involution.

Examples 2.5. Hence, and, and their generalizations as discussed in Sections 3 & 4 cannot adhere to any involution.

2.6. Some minimalities: (1) and are smallest noncommutative rings (up to isomorphism). These are right and left symmetric, respectively. So the minimal noncommutative right (or left) symmetric rings are V and.

(2) Next higher order noncommutative rings are of order eight. So two minimal noncommutative symmetric rings are strictly upper and lower triangular matrix rings and, respectively. Both are without identity and are not reversible (can be checked easily).

(3) ( [3] ; Example 2.6) A minimal non-commutative symmetric ring with identity is the ring, in which addition and multiplication are defined by the rules:

(see details in [3] ; Example 2.6). This ring has sixteen elements and is also reversible.

Reappearance of the Lambek Criterion: Lambek proved in [1] that a ring with one is symmetric if and only if it is isomorphic to the rings of sections of a sheaf of prime - torsion free symmetric rings. Following is an extension of it.

Proposition 2.7. A ring with an idempotent is right symmetric if and only if contains a subring which is isomorphic to the rings of sections of a sheaf of prime - torsion free symmetric rings.

Proof: “Only if”, is obvious, because a symmetric ring with 1 is a right symmetric ring with an idempotent. For “if”, consider that is right symmetric. Let be an idempotent. Then the corner ring being a subring of is right symmetric and because is the multiplicative identity, so becomes a symmetric ring. Rest follows from ( [1] : Corollary 1]. □

A ring R is called Armendariz as introduced by Rege, S. Chhawchharia in [12] if for any pair of polynomials

and in such that, then ,

. In this section we construct an Armendariz Boolean ring and a polynomial semicommutative ring.

The first part of the following lemma is proved by Nielsen in ( [13] ; Lemma 1]. The remaining relations are just tautologies.

Lemma 2.8. Let R be a right symmetric ring. Let and be two polynomials in

such that. Then the following relations hold:

(1)

(2)

(3)

(4)

Theorem 2.9. Let R be a right symmetric ring. Let and be two polynomials

in such that If the coefficients of (or) are idempotents, then

Proof: Assume that the coefficients of are idempotents. If is idempotent, then by (2) of above lemma

Now the coefficients in are of the form,. The induction step suggests that:, then remove the term from this sum and

multiply the remaining sum by, where consecutively, and removing the zero terms until we get the last term, In this process the relation (4) of Lemma 2.8 is also involved to delete the unwanted terms. Hence we conclude that, , and. If the coefficients of are idempotents, then (1) and (3) of Lemma 2.8 are involved to prove the desired result. □

Corollary 2.10. Let be a right symmetric Boolean ring. Then:

(1) is Armendariz.

(2) For every pair of polynomials

(3) For every pair of polynomials In other words, is semicommutative.

Proof: (1) and (2) are followed from Theorem 2.9.

(3) Let and. Then, Let Then,

. Because all terms in the product of polynomials, and are of the form,we conclude that:. □

3. Some Extensions of Klein 4-Rings

Now we pose few more examples of one sided symmetric rings. These rings are extensions of and First result gives a criterion of all rings of order as symmetric and non symmetric.

Theorem 3.1. For any prime, a ring of order is symmetric if and only if it is reversible. The non-symmetric ring is either left or right symmetric.

Proof: It is known that up to isomorphism there are eleven rings of order. These can be classified as commutative and non-commutative rings. The first statement trivially holds for commutative rings. There are nine commutative rings and the only non-commutative rings are:

and its opposite ring

Both rings are of characteristic and can be verified that these are neither symmetric nor reversible. Note that the non-commutative rings S and of order, for all primes, are right symmetric and left symmetric, respectively. For instance, in case of S, the non-zero elements of S are of the form, and, where, so as in the case of,

Hence is right symmetric. But is not symmetric, because

Clearly is not reversible as well. □

Let be a set of symbols and consider the additive group generated by these symbols. This group has elements. Define the multiplication on by the rule: Then clearly,

These rules clearly imply that is an associative ring without 1 and is of characteristic 2. Let us denote this ring by as its order is. The Klein-4 ring as discussed in Example 2.2 above is and is smallest in the series.

Theorem 3.2. The ring is right symmetric but not left symmetric. Likewise, is left symmetric but not right symmetric.

Proof: Assume that, such that. If any one of, or is zero, then we are done. So consider only non-zero elements.

Assume that, and, where and such that

.

Note that for any and as above,

Same will be the consequences if we replace by, i.e.

and

So if and only if either is even or is even. This means that. Hence is right symmetric.

On the other hand, assume that is even. Because and are odd, then, but. This completes the proof.

The second part can be obtained by symmetry. □

Trivial extension of a ring: Let be any ring, a trivial extension of, is a subring of the upper triangular matrix ring over and is defined as:

Theorem 3.3. The trivial extension ring is a right symmetric ring where is the Klein 4-ring.

Proof: In short we write as an element of but we will follow the rule of matrix multiplication on such ordered pairs. So let, with, where,. Then

(a) and

(b)

We want to prove that. For this we need to establish that

(c) and that

(d)

As in Example 2.2, (a) holds if either or. Assume that and, Then (b) holds if. We substitute in (c) and (d). We see that these are also satisfied.

If and, then (b) holds if. Again we substitute in (c) and (d), we see that these are satisfied. If, then all are satisfied. Hence we conclude that is right symmetric. □

Remarks 3.4. (1) is not symmetric, as one can easily work out that

,

but

(2) It is known that if is reduced then is symmetric ([8] ; Corollary 2.4). Note that is not a reduced ring.

(3) The ring of upper triangular matrices over, is not right symmetric, because for,

and

Thus, in general, or are not right symmetric. Hence, being right symmetric is not Morita invariant.

Theorem 3.5. For a commutative indeterminate, the polynomial ring is right symmetric.

Proof: Because is without 1, so is also without 1 and so. Now let, where a_i, b_j, , , and assume that

where

Also assume that

where

We want to prove that if, then so is. So assume that. Then, , and these terms can be expressed as

where For, we want to establish that, , where

(I) We have five options for. These are, , , , or Any one choice will give us.

(II) Let. From (I) if we choose, then, and so. For, we again have five choices, or, and with the previously chosen, we see that

(III) Let us have as in (I) & (II). Then Here again we have five choices for and For every choice we have which implies and again we have five options here, each gives Thus we find that holds. The choices for or will yield same result.

(IV) Now assume that. This is in continuation of (I), (II), & (III) and the same repetition will give us and simultaneously.

The rest are similar.

Definitely, we need to watch the situation for non-zero values, for instance, if we let and then we see that, Same situation comes if we let and. Hence the required result is obtained. □

4. McCoy Rings without Identity

In [13] , Nielsen defined that a ring is a right McCoy, if, then there exists an, such that. Left McCoy and McCoy rings are defined similarly. It is proved in ( [13] , Theorem 2), that: every reversible ring is left and right McCoy, hence McCoy.

In ( [13] ; Section 3), Nielsen, constructed an example of a right McCoy ring with identity. This example is neither symmetric nor reversible, and there is no question that it is right or left symmetric because it has identity.

In next result we prove that the right symmetric ring is a right McCoy ring without identity.

Theorem 4.1. The ring as constructed in Theorem 2.2. is a McCoy ring.

Proof: Again, let be a set of symbols and consider the additive group generated

by these symbols and define the multiplication on by the rule:

Then is a ring as constructed in Theorem 2.3. The characteristic of this ring is 2. Consider an element of

the form, where, , and is even and let all z_k be distinct so that. Then for any element,.

Assume that and be elements of, with Then

Hence is right McCoy.

On the other hand, note that provided that the coefficients of are the elements of

of the form, where and is even. Hence for any, which shows that

is left McCoy. Hence is McCoy. □

Remarks 4.2. It follows from above that

(i) is McCoy, right symmetric, and semicommutative, but neither symmetric nor reversible.

(ii) is McCoy, left symmetric, and semicommutative, but neither symmetric nor reversible.

Example 4.3. In Section 3 of [14] an example of a McCoy ring is constructed such that its power series ring is not McCoy. Here we prove that the power series ring of Klein 4-ring, which we already have proved that it is

McCoy, is also McCoy. A typical element of is of the form,

where is a coefficient in the power series ring. Clearly, , so. On

the other hand, let and but. Then the coefficients in are in

the set and as previously we got the outcome. Hence we conclude that is McCoy.

We end up at a general statement. The following corollary can be proved by the methods used in Theorem 4.1.

Corollary 4.4. The power series ring is McCoy.

Acknowledgements

This project was funded by the ISR, Umm Al-Qura University, under Grant No: 43305007. The authors, therefore, acknowledge with thanks the financial and technical support provided by ISR.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Lambek, J. (1971) On the Representation of Modules by Sheaves of Factor Modules. Canadian Mathematical Bulletin, 14, 359-368. http://dx.doi.org/10.4153/CMB-1971-065-1
[2]	Ben Yakoub, L. and Louzari, M. (2009) On Extensions of Extended Symmetric and Reversible Rings. International Journal of Algebra, 3, 423-433.
[3]	Huh, C., Kim, H.K., Kim, N.K. and Lee, Y. (2005) Basic Examples and Extensions of Symmetric Rings. Journal of Pure and Applied Algebra, 202, 154-167. http://dx.doi.org/10.1016/j.jpaa.2005.01.009
[4]	Kafkas, G., Ungor, B., Halicioglu, S. and Harmanici, A. (2011) Generalized Symmetric Rings. Algebra and Discrete mathematics, 12, 72-84.
[5]	Kwak, T.K. (2007) Extensions of Extended Symmetric Rings. Bulletin of The Korean Mathematical Society, 4, 777-788. http://dx.doi.org/10.4134/BKMS.2007.44.4.777
[6]	Cohn, P.M. (1999) Reversible Rings. Bulletin of the London Mathematical Society, 31, 641-648. http://dx.doi.org/10.1112/S0024609399006116
[7]	Anderson, D. and Camillo, V. (1999) Semigroups and Rings Whose Zero Product Commutes. Communications in Algebra, 27, 2847-2852.
[8]	Marks, G. (2002) Reversible and Symmetric Rings. Journal of Pure and Applied Algebra, 174, 311-318. http://dx.doi.org/10.1016/S0022-4049(02)00070-1
[9]	Bell, H.E. (1970) Near-rings, in Which Every Element Is a power of Itself. Bulletin of the Australian Mathematical Society, 2, 363-368. http://dx.doi.org/10.1017/S0004972700042052
[10]	Marks, G. (2003) A Taxonomy of 2-Primal Rings. Journal of Algebra, 266, 494-520. http://dx.doi.org/10.1016/S0021-8693(03)00301-6
[11]	Fakieh, W.M. and Nauman, S.K. (2013) Reversible Rings with Involutions and Some Minimalities. The Scientific World Journal, 2013, 8 pages, Article ID: 650702. http://dx.doi.org/10.1155/2013/650702
[12]	Rege, M.B. and Chhawchharia, S. (1997) Armendariz Rings. Proceedings of the Japan Academy, Series. A, Mathematical Sciences, 73, 14-17.
[13]	Nielsen, P.P. (2006) Semi-Commutativity and McCoy Conditions. Journal of Algebra, 298, 134-141. http://dx.doi.org/10.1016/j.jalgebra.2005.10.008
[14]	Camillo, V. and Nielsen, P.P. (2008) McCoy Rings and Zero-Divisors. Journal of Pure and Applied Algebra, 212, 599-615. http://dx.doi.org/10.1016/j.jpaa.2007.06.010