Journal of Modern Physics
Vol.07 No.11(2016), Article ID:69139,8 pages
10.4236/jmp.2016.711121
Biquaternionic Form of Laws of Electro-Gravimagnetic Charges and Currents Interactions
L. A. Alexeyeva
Institute of Mathematics and Mathematical Modeling, Almaty, Kazakhstan

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 8 June 2016; accepted 24 July 2016; published 27 July 2016
ABSTRACT
One the base of differential algebra of biquaternions, the one model of electro-gravimagnetic interactions of electric and gravimagnetic charges and currents has been constructed. For this, three Newton laws analogues are used. The closed system of biquaternionic wave equations is constructed for determination of the charges-currents and electro-gravimagnetic fields and united field of interactions. The equation of charge-current transformation is like the generalization of biquaternionic presentation of Dirac equation. The properties of its solutions are described, depending on properties of external EGM field. The biquaternions of energy-pulse of EGM-field and charges-currents are considered. The energy-pulse of EGM-interactions is calculated.
Keywords:
Electro-Gravimagnetic Field, Electric Charge, Gravimagnetic Charge, Current, Energy, Biquaternion, Bigradient, Dirac Equation, Newton Laws

1. Introduction
In the paper [1] , we described the one biquaternionic model of electro-gravimagnetic (EGM) field, charges and currents. In this model, gravitational field (which is potential) is united with magnetic field (which is torsional) which gives possibility to enter gravimagnetic tension, gravimagnetic charge and gravimagnetic current. There we have shown that in algebra of biquaternions, the charges and currents of EGM-field are physical appearance of bigradient of EGM-intensity. Differential operator bigradient is the generalization of gradient operator on the space of biquaternions which characterizes a direction of more extensive change of biquaternionic functions. If bigradient of EGM-intensity is equal to zero then charges and currents are absent. Also there we constructed the law of inertia for a free system of mass, charges and currents, which described their motion under action only with internal electric and gravimagnetic tensions. This law is the fields analogue of the first Newton law― inertia law for a solid (considered as material point).
Here we consider the motion of electro-gravimagnetic charges and currents under action of external EGM- fields which are created by other charges and currents. The laws of their interaction are the fields analogue of the second and third Newton laws. They have been constructed in the form of biquaternionic wave equations which generalize biquaternionic form of Dirac equations. Some solutions of them are discussed. The energy- pulse of EGM-interactions is calculated.
We used also here the differential algebra of biquaternions in Hamiltonian form which was shortly described in [1] (see [2] for more detail). This form is very convenient for description of physical fields. There are voluminous literature about application of algebras of quaternions and biquaternions in fields theory, in the theory of electromagnetic fields, and quantum mechanics [3] - [17] . Differential algebra of biquaternions gives possibility to simplify mathematical record of systems of Maxwell and Dirac equations to construct their solutions and to study their properties.
The novelty of this work is the construction of laws of electro-gravymagnetic interactions on the base of biquaternions algebra. The properties of this algebra and operation of quaternionic multiplication in fields theory have visual physical interpretation and detect new objective laws which are impossible to determine without this algebra. It’s natural for matter as you’ll see here.
2. Biquaternions of Electro-Gravimagnetic Field, Charges and Currents
Their are the next complex characteristics of EGM-field [1] :
- complex vector of EGM-intensity
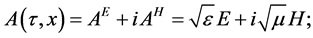
- complex charges field:
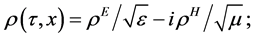
- complex currents field:
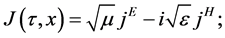
- complex scalar field of attraction-resistance
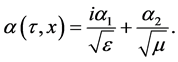
Here real vectors E and H are the tensions of electric and gravimagnetic fields; real scalars 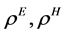 are the densities of electric and gravimagnetic charges; real vectors
are the densities of electric and gravimagnetic charges; real vectors  are the densities of electric and gravi- magnetic currents; values
are the densities of electric and gravi- magnetic currents; values 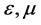 are the constants of electric conductivity and magnetic permeability of the EGM-medium.
are the constants of electric conductivity and magnetic permeability of the EGM-medium.
In biquaternions algebra on Minkowski space 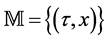 the EGM-field, charges and currents may be presented by use the next biquaternions:
the EGM-field, charges and currents may be presented by use the next biquaternions:
EGM-intensity
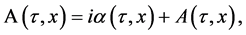
charge-current
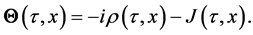
In the paper [1] [2] was shown that connection between EGM-intensity and charge-current has the bigradiental form:
POSTULATE 1
 (1)
(1)
Here and further the bigradients  and
and 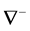 are the next biquaternionic differential operators:
are the next biquaternionic differential operators:
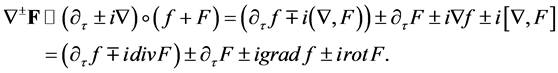
Further we name them mutual bigradients. They define a “directions” of more intensive changing of biquater- nionic field.
3. The Power and Density of Acting Forces
Let’s consider two systems of charges and currents . Every of them generate own EGM-field:
. Every of them generate own EGM-field: 


We name a power-force density the next biquaternion

which is acting from side of A’ -field on the charge and current of A-field. Really, according to definitions, the scalar part is determined as power density of acting forces:

Here 

Selecting the real and imaginary part from the formulae (2) we get the expressions for a density of acting forces


Potentional part of 

- Coulomb’s force
- gravimagnetic force 

- Lorentz force 

- gravielectric force
In real part of the power p (3) we see the powers of Coulomb’s force, gravitational and magnetic forces. The power of gravielectric force in real part of (3) does not enter as it does not work on the mass displacement, because it is perpendicular to its velocity. It’s interesting that Lorentz force also does not enter in real part of (3). It proves that this force is perpendicular to mass velocity, though directly from Maxwell equations this does not follow.
Naturally, by analogy, we assume that formula (5) describes forces, causing a change of electric current. Consequently their power stands in imaginary part p (3).
With entering the scalar field

You see here the additional summands which appear in presentation of the powers (

Vector 

4. CC-Transformations Equation: Second Newton Law
The charge-current field 

POSTULATE 2. The law of a change of a charge-current field under the action of external EGM-field is

Entering the constant of interaction 
If one EGM-field much stronger then second one:
it’s possible to neglect the second field change under influence of charge and current on 


Revealing scalar and vector part from (7), we get the system of two differential equations:


By use (3), (4) we obtain from Equation (9) the two vectorial differential equations:


The Equation (10) is the second Newton law analogue for CC-field. Here the value 

Equation (10) describes the motion of gravimagnetic charges and currents under action of the external EGM- field. Consequently Equation (11) defines the motion of electric charges and currents.
The scalar Equation (8) is the law of conservation of electric and gravimagnetic charges. As you see the external EGM-field can essentially change CC-field.
5. Third Newton Law: The Laws of Charge-Currents Interactions
On the virtue of the third Newton law about acting and counteracting forces, we suppose that must be executed for electro-gravimagnetic forces the equality:
POSTULATE 3
From here we get
fields analogue of third Newton law:

By use it we construct
the law of the charge-current interaction:



Here Equation (13) correspond to the second Newton law which is written for charge-current each of interacting field. Equation (14) is the third Newton law. Together with Maxwell equations for these fields (15) they give closed system of the nonlinear differential equations for determination
It is interesting that in scalar part of Equation (12) it requires the equality of the powers corresponding to forces, acting on charges and currents of the other field, i.e. it is befitted known in mechanics the identity to reciprocity of Betty, which is usually written for the forces works.
6. First Newton Law: Free EGM-Field
Let’s consider A-field, which is generated


which is equivalent to equations:
For initial designations we have following formulas:


Naturally that the well known law of charges conservation in the form (17) must be executed in absence of external EGM-field.
This law (16) was considered in [1] and solutions of this equations were defined in the next form:

Scalar potentials 

where 

We give here also for (16)
the solution of Cauchy problem

were there are the initial conditions:

From postulate 1 to follow that A-field is defined in the form:

where

This formula is a generalization of the famous Kirchhoff formula for solution of Cauchy problem for wave equation [18] .
7. The Solutions of CC-Transformation Equation by Action of Invariable External EGM-Field
Let consider Equation (7) when external EGM-field 


This equation is biquaternionic generalization of Dirac equations. Its presentation by use differential equations coincides with Dirac equations when
There are simple connection between solutions of Equation (7) and Equation (22):

where 
From here to follow that imaginary part of scalar field resistance-absorbtion 








8. Energy-Impulse Biquaternions: First Thermodynamics Law
We introduce the biquaternion of energy-impulse of EGM-field ( EGM-energy-impulse):

where 



P is real vector-function:
By


By analogue we construct the biquaternion of energy-impulse for charge-current field ( CC-energy-impulse):

It contains currents energy :
where first summand includes Joule heat


Only if gravimagnetic and electrical currents are parallel or one from them is equal zero, then
If to take scalar product Equation (9) with

It is easy to see that this law is like to the first thermodynamics law. Here the sum of second and third summands in left part is designated
characterizes the own velocity of the change of current energy of 
If there are not acting external forces,

It’s the first thermodynamics law for a free CC-field.
9. The United Field of Interaction: Energy-Pulse of Interactions
If there are some (N) interacting CC-fields then we have for every of them


United CC-field, as easy to see after summing the first equation over k, is free. It satisfies to the inertia law

because all forces are internal, also as in Newton mechanics of interacting solids.
Let consider the laws of energy transformation at interaction of different charges-currents. Energy-pulse for united charge-current field has the form:
Here the first summand is an amount of energy-pulse of interacting charge-current.
We introduce biquaternion of energy-pulse interaction. Its real part describes energy-pulse interaction for the same name charge and current, but imagine part for different name ones:
or in initial designations:
As result we get the conditions of energy transformation by charges-currents interaction:
energy separation if
energy absorption if
energy conservation if
Vector 
10. Conclusions
We construct here biquaternionic forms of laws of electric and gravimagnetic charges and currents interaction by analogy to Newton laws, which gives us closed hyperbolic system of differential equations for their definition and determination of corresponding EGM-fields. For the free system of charges and currents, Equations (22) and (24) define the behavior of CC-field and EGM-field over time if their initial states are known. After calculating the bigradients from here, the all fields, charges and currents can be defined according to their definitions. It’s the very suitable short form which contains the algorithm for their calculation.
At building of charge-current transformations equation, we get as known gravitational, electric and magnetic forces, so we found the new forces which are needed in experimental motivation. Considered properties (26) of solutions of CC-transformation equation by existence external EGM-field give possibility to test this model on practice.
Note also that the essential at building and studying this model of EGM-field and CC-field is the differential algebra of biquaternions [2] , without which such construction of differential equations, describing interaction of charges and currents in the forms which give the fields analogies of Newton laws and are very convenient for calculations, will be practically impossible.
Cite this paper
L. A. Alexeyeva, (2016) Biquaternionic Form of Laws of Electro-Gravimagnetic Charges and Currents Interactions. Journal of Modern Physics,07,1351-1358. doi: 10.4236/jmp.2016.711121
References
- 1. Alexeyeva, L.A. (2016) Journal of Modern Physics, 7, 435-444.
http://www.scirp.org/journal/jmp
http://dx.doi.org/10.4236/jmp.2016.75045 - 2. Alexeyeva, L.A. (2012) Int. J. Clifford Analysis, Clifford Algebras and Their Applications, 7, 19-39.
- 3. Rastall, R. (1964) Review of Modern Physics, 36, 820-832.
http://dx.doi.org/10.1103/RevModPhys.36.820 - 4. Edmonds Jr., J.D. (1978) American Journal of Physics, 46, 430.
http://dx.doi.org/10.1119/1.11316 - 5. Spilker, G.L. (1983) Report of USSR Academy of Sciences, 272, 1359-1363.
- 6. Rotelli, P. (1989) Modern Physics Letters A, 4, 933-940.
http://dx.doi.org/10.1142/S0217732389001106 - 7. Davies, A.J. (1990) Physical Review D, 41, 2628-2630.
http://dx.doi.org/10.1103/PhysRevD.41.2628 - 8. Finkelstein, D., Jauch, J.M., Schiminovich, S. and Speiser, D. (1992) Journal of Mathematical Physics, 3, 207-220.
http://dx.doi.org/10.1063/1.1703794 - 9. Adler, S.L. (1995) Quaternionic Quantum Mechanics and Quantum Fields. Oxford University Press, New York.
- 10. Kravchenko, V.V. (1995) Doklady Mathematics, 51, 287-289.
- 11. Kassandrov, V.V. (1995) Gravitation and Cosmology, 1, 216-222.
- 12. De Leo, S. and Rodrigues Jr., S. (1998) International Journal of Theoretical Physics, 37, 1707-1720.
http://dx.doi.org/10.1023/A:1026692508708 - 13. Kravchenko, V.V. (2003) Quaternionic Equation for Electromagnetic Fields in Inhomogeneous Media. In: Begehr, H., Gilbert, R. and Wah Wong, M., Eds., Progress in Analysis, Vol. 1, World Scientific, 361-366.
- 14. Efremov, A.P. (2004) Hypercomples Numbers in Geometry and Physics, 1, 111-127.
- 15. Acevedo, M., Lopez-Bonilla, J. and Sanchez-Meraz, M. (2005) Apeiron, 12, 371.
- 16. Alexeyeva, L.A. (2013) Differential Algebra of Biquaternions. Dirac Equation and Its Generalized Solutions. Progress in Analysis. Proceedings of the 8th Congress of the ISAAC, Moscow, 22-27 August 2013, 153-161.
- 17. Alexeyeva, L.A. (2013) Mathematical Journal, 13, 17-35. (In Russian)
http://www.math.kz/images/journal/2013-1/Alexeyeva.pdf - 18. Vladimirov, V.S. (1976) Generalized Functions in Mathematical Physics. Nauka Publisher, Moscow.















