Received 24 September 2015; accepted 20 November 2015; published 23 November 2015

ABSTRACT

This work is a continuation of the earlier article [1] . We establish new numerical methods for solving systems of Volterra integral equations with cardinal splines. The unknown functions are expressed as a linear combination of horizontal translations of certain cardinal spline functions with small compact supports. Then a simple system of equations on the coefficients is acquired for the system of integral equations. It is relatively straight forward to solve the system of unknowns and an approximation of the original solution with high accuracy is achieved. Several cardinal splines are applied in the paper to enhance the accuracy. The sufficient condition for the existence of the inverse matrix is examined and the convergence rate is investigated. We demonstrated the value of the methods using several examples.

Keywords:

Spline Functions, Integral Equations, Numerical Methods

1. Introduction

Integral equations appear in many fields, including dynamic systems, mathematical applications in economics, communication theory, optimization and optimal control systems, biology and population growth, continuum and quantum mechanics, kinetic theory of gases, electricity and magnetism, potential theory, geophysics, etc. Many differential equations with boundary-value can be reformulated as integral equations. One example given in this paper is to use a system of integral equations to solve a third order differential equation. There are also some problems that can be expressed only in terms of integral equations. Scores of papers have appeared on solving integral equations, for examples, cf [2] - [4] .

2. Cardinal Splines with Small Compact Supports

Since the paper [5] by Schoenberg published in 1946, spline functions have been studied by many scholars extensively. Spline functions have excellent features and applications are endless (for examples, cf [6] ). The spline functions on uniform partitions are simple to construct and easy to employ, and are sufficient for a variety of applications.

The starting point is frequently the zero degree polynomial B-spline, an integral iteration formula could be used to construct higher order spline functions with higher degree of smoothness, i.e. let

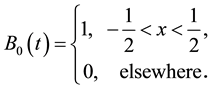
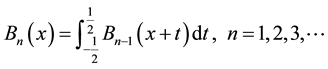(1)

has the global expression 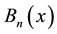are called one dimensional B- splines, which are polynomial splines with small compact supports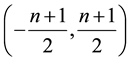, (i.e. for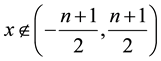), and excellent traits (cf [6] ). In my previous papers (cf [1] [7] - [10] ), low degree orthonormal

spline and cardinal spline functions with small compact supports were applied in solving the second kind of linear Fredholm and Volterra integral equations.

By cardinal conditions (cf [5] ), we mean, let be an interpolation function, 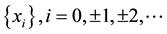be interpolation points, then

The lowest degree continuous cardinal spline is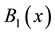. To achieve higher degree of approximation, we need the cardinal spline functions with higher degree of smoothness. We employ splines that were developed in my previous papers.

The cardinal spline that was originally given in [11] is based on from (1) using the similar integral process. Let

Then satisfies the above cardinal condition when Notice that by the

construction, for is a polynomial of degree in each subinterval of its support. Furthermore, we acquired nice approximation properties (cf [11] ).

To achieve higher degree of smoothness, we employ (cf [6] ) and constructed (cf [11] )

It is a simple calculation to check the cardinal condition is satisfied. The has the same support as, which is. However, each polynomial in the sub-intervals of has higher degree than the

ones for, Let The better approximation properties hold for (cf [11] ).

3. Numerical Methods Solving the System of Volterra Integral Equations

In this and next two sections, we are concentrating on the second kind system of linear Volterra integral equations

(I2)

where,

3.1. Method 1-V for Solving the System of Volterra Integral Equations

As for the Volterra system (I2), we solve it in an interval. Again we let Furthermore, plug

(funs 1)

in (I2), we get

Let, we arrive at for

(S4)

which is a simple system of S linear equations on the unknowns. For the convergency rate of solution of the Volterra system (I2), we have

Proposition 1. Given that, , and exist and are bounded in,

and exist and are bounded in. Further

more satisfies the condition:

where. Let satisfy the linear system (S1) and; then

where is the exact solution of the system of Equation (I1).

The proof is very similar to the proof of the following Proposition 3, so we skip it.

3.2. Method 2-V for Solving the Systems of Volterra Integral Equations

To achieve higher approximation rate, we plug

(funs 2)

into (I2), where extra function values still follow the conditions (cond1) and arrive at

Let, By the similar reasons as above, we conclude that

(S5)

which is still a relatively simple system of linear equations.

Remark If the integral equation (I2) has a unique solution, then the linear system (S5) is consistent. Furthermore

(where)

approximates the solution of the system (I2) with a rate of, similar as in the Preposition 6.

Proposition 2. Given that, and exist and are bounded in, exist and are bounded in. Furthermore, satisfies the condition:

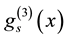
where. Let satisfies the linear system (S2) and, . Let then

where are the exact solution of the Volterra system (I1).

The proof is very similar to the proof of the following Proposition 3, so we skip it.

3.3. Method 3-V for Solving the Systems of Volterra Integral Equations

To achieve higher approximation rate, we let

(funs 3)

again we plug into (I2), where extra function values still follow the conditions (cond2) cf [9] , and we arrive at

Let, by the similar reasons as above, we conclude that,

(S6)

which is still a relatively simple system of linear equations.

Remark If the integral Equation (I2) has a unique solution, then the linear system (S6) is consistent. Furthermore

approximates the solution of the integral Equation (I2) with a rate of as in the following Preposition. Where

Proposition 3. Given that, and exist and are bounded in, exist and are bounded in. Fur-

thermore, satisfies the condition:

where. Let satisfies the linear system (S3) and

Let then

where are the exact solution of the Volterra system (I1).

Proof. Let

,

where the coefficients are the solutions of above linear system (S3), and

, then

(I1)

Plug in

Therefore

4. Numerical Examples

Example 1. Consider,

Let

Apply Method V-1 and solve the linear system, we obtain:

To achieve higher degree of accuracy, we apply Method V-3 and obtain :

Compare with the exact solution:

the error is for.

Example 2. Given a system of linear integral equations: for,

Let We apply the Method V-1, where

are unknown coefficients to be determined. Solve the system of linear equations on unknowns obtain

and

Since the solution is Comparing with the exact solution, the error is for.

Example 3. Consider the third order differential equations:

,

with the initial condition:

Let then we could transfer the equation to a system of integral equations:

,

,

Let

We apply Method V-2 and solve the linear system and arrive at the solution:

Since the exact solution is

Compare with the exact solution, the error.

5. Conclusions

The system of first kind of linear Volterra integral equations has the form

(I4)

where

They can easily be transformed to the system of second kind of linear Fredholm and Volterra integral equations (cf [3] ). We can apply the similar method to solve the first and second kind Fredholm integral systems. So the proposed methods are simple and effective procedures for solving both linear system of Fredholm and Volterra integral equations.

The orthonormal and cardinal splines could also be applied to non-linear integral equations; the resulting system of coefficients will be a non-linear system, which takes more time and effort to solve. The convergence rate could be higher if we apply more complicated orthonormal or cardinal spline functions.

Acknowledgements

The work was partially funded by the National Natural Science Foundation of China under Grant no. 1471093, the Doctoral Program Foundation of the Ministry of Education of China under Grant no. 20110111120026, the Natural Science Foundation of Anhui Province of China under Grant no. 1208085MA15, the Key Project Foundation of Scientific Research, Education Department of Anhui Province under Grant no. KJ2014ZD30.

The authors thank the family members, the colleagues and administrators of the University of LaVerne for their encouragement and support on this research.

Cite this paper

XiaoyanLiu,ZhiLiu,JinXie, (2015) Solving Systems of Volterra Integral Equations with Cardinal Splines. Journal of Applied Mathematics and Physics,03,1422-1430. doi: 10.4236/jamp.2015.311170

References