Applied Mathematics
Vol.06 No.10(2015), Article ID:59871,11 pages
10.4236/am.2015.610157
On Stability of Nonlinear Differential System via Cone-Perturbing Liapunov Function Method
A. A. Soliman, W. F. Seyam
Department Mathematics, Faculty of Sciences, Benha University, Benha, Egypt
Email: a_a_soliman@hotmail.com
Copyright © 2015 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 8 August 2015; accepted 21 September 2015; published 24 September 2015
ABSTRACT
Totally equistable, totally f0-equistable, practically equistable, and practically f0-equistable of system of differential equations are studied. Cone valued perturbing Liapunov functions method and comparison methods are used. Some results of these properties are given.
Keywords:
Totally Equistable, Totally f0-Equistable, Practically Equistable, Practically f0-Equistable

1. Introduction
Consider the non linear system of ordinary differential equations
 (1.1)
(1.1)
and the perturbed system
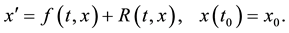 (1.2)
(1.2)
Let Rn be Euclidean n-dimensional real space with any convenient norm , and scalar product
, and scalar product . Let for some
. Let for some
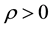

where
 denotes the space of continuous mappings
denotes the space of continuous mappings
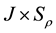 into
into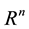 .
.
Consider the scalar differential equations with an initial condition
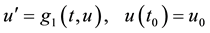 , (1.3)
, (1.3)
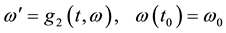 (1.4)
(1.4)
and the perturbing equations
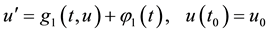 (1.5)
(1.5)
 (1.6)
(1.6)
where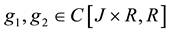 ,
,
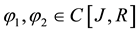 respectively.
respectively.
Other mathematicians have been interested in properties of qualitative theory of nonlinear systems of differential equations. In last decade, in [1] , some different concepts of stability of system of ordinary differential Equations (1.1) are considered namely, say totally stability, practically stability of (1.1), and (1.2); and in [2] , methods of perturbing Liapunov function are used to discuss stability of (1.1). The authors in [3] discussed some stability of system of ordinary differential equations, and in [4] [5] the authors discussed totally and totally φ0-stability of system of ordinary differential Equations (1.1) using Liapunov function method that was played essential role for determine stability of system of differential equations. In [6] the authors discussed practically stability for system of functional differential equations.
In [7] , and [8] , the authors discussed new concept namely, φ0-equitable of the zero solution of system of ordinary differential equations using cone-valued Liapunov function method. In [4] , the author discussed and improved some concepts stability and discussed concept mix between totally stability from one side and φ0- stability on the other side.
In this paper, we will discuss and improve the concept of totally stability, practically stability of the system of ordinary differential Equations (1.1) with Liapunov function method, and comparison technique. Furthermore, we will discuss and improve the concept of totally φ0-stability, and practically φ0-stability of the system of ordinary differential Equations (1.1). These concepts are mix and lie somewhere between totally stability and practically stability from one side and φ0-stability on the other side. Our technique depends on cone-valued Liapunov function method, and comparison technique. Also we give some results of these concepts of the zero solution of differential equations.
The following definitions [8] will be needed in the sequal.
Definition 1.1. A proper subset
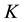 of
of

where



Definition 1.2. The set

Definition 1.3. A function

then there exists

Definition 1.4. A function




2. Totally Equistable
In this section we discuss the concept of totally equistable of the zero solution of (1.1) using perturbing Liapuniv functions method and Comparison principle method.
We define for

The following definition [1] will be needed in the sequal.
Definition 2.1. The zero solution of the system (1.1) is said to be




holds, provided that


Definition 2.2. The zero solution of the Equation (1.3) is said to be




holds, provided that


Theorem 2.1. Suppose that there exist two functions

and there exist two Liapunov functions


where




(H1)

(H2)

where

(H3)
(H4) If the zero solution of (1.3) is equistable, and the zero solution of (1.4) is totally equistable.
Then the zero solution of (1.1) is totally equistable.
Proof. Since the zero solution of the system (1.4) is totally equistable, given




holds, provided that


Since the zero solution of (1.3) is equistable given




holds, provided that
From the condition (H2) we can find


To show that the zero solution of (1.1) is





holds, provided that


Suppose that this is false, then there exists a solution



Let

Since




Then
where

for

Let
Applying the comparison Theorem (1.4.1) of [1] , it yields
where

Define
To prove that
It must be show that


Choose
where

From (2.2) at

From the condition (H2) and (2.4), at

From (2.3), we get
Since
From (2.1), we get

Then from the condition (H2), (2.4) and (2.7) we get
This is a contradiction, then it must be
holds, provided that


Therefore the zero solution of (1.1) is totally equistable.
3. Totally f0-Equistable
In this section we discuss the concept of Totally f0-equistable of the zero solution of (1.1) using cone valued perturbing Liapunov functions method and Comparison principle method.
The following definition [4] will be needed in the sequal.
Definition 3.1. The zero solution of the system (1.1) is said to be totally f0-equistable (f0-equistable with respect to permanent perturbations), if for every




holds, provided that



Let for some
Theorem 3.1. Suppose that there exist two functions

and let there exist two cone valued Liapunov functions



where




(h1)


(h2)


where

(h3)
(h4) If the zero solution of (1.3) is f0-equistable, and the zero solution of (1.4) is totally f0-equistable. Then the zero solution of (1.1) is totally f0-equistable.
Proof. Since the zero solution of (1.4) is totally f0-equistable, given, given




holds, provided that



Since the zero solution of the system (1.3) is f0-equistable, given




holds, provided that


From the condition (h2) we can choose


To show that the zero solution of (1.1) is T1-totally f0-equistable, it must be prove that for every




holds, provided that



Suppose that is false, then there exists a solution



Let

Since




Then
where

for

Let
Define
To prove that
It must be shown that
Choose
From (3.2) at

From the condition (h2) and (3.4), at

From (3.3), we get
Since
From (3.1), we get

Then from the condition (h2), (3.4) and (3.7) we get at
This is a contradiction, then
provided that



4. Practically Equistable
In this section, we discuss the concept of practically equistable of the zero solution of (1.1) using perturbing Liapunov functions method and Comparison principle method.
The following definition [8] will be needed in the sequal.
Definition 4.1. Let



holds, provided that


In case of uniformly practically equistable, the inequality (4.1) holds for any
We define

Theorem 4.1. Suppose that there exist two functions

and there exist two Liapunov functions


where



(I)

(II)

where

(III)
(IV) If the zero solution of (1.3) is equistable, and the zero solution of (1.4) is uniformly practically equistable.
Then the zero solution of (1.1) is practically equistable.
Proof. Since the zero solution of (1.4) is uniformly practically equistable, given


holds provided
Since the zero solution of the system (1.3) is equistable, given


such that for every solution

holds provided that
From the condition (II) we can find

To show that the zero solution of (1.1) practically equistable, it must be exist


holds, provided that
Suppose that this is false, then there exists a solution



Let

From the condition (III) we obtain the differential inequality for
Let
Applying the comparison Theorem [8] , yields
where

To prove that
It must be show that
Choose
where

From (4.3) at

From the condition (II) and (4.5), at

From (4.4), (4.6) and (4.7), we get
From (4.2), we get

Then from the condition (II), (4.5) and (4.8), we get at
This is a contradiction, then
provided that
Therefore the zero solution of (1.1) is practically equistable.
5. Practically f0-Equistable
In this section we discuss the concept of practically f0-equistable of the zero solution of (1.1) using cone valued perturbing Liapunov functions method and comparison principle method.
The following definitions [6] will be needed in the sequal.
Definition 5.1. Let




holds, provided that


In case of uniformly practically f0-equistable, the inequality (5.1) holds for any
We define
Theorem 5.1. Suppose that there exist two functions

and let there exist two cone valued Liapunov functions


where



(i)

(ii)

where

(iii)
(iv) If the zero solution of (1.3) is f0-equistable, and the zero solution of (1.4) is uniformly practically f0- equistable.
Then the zero solution of (1.1) is practically f0-equistable.
Proof. Since the zero solution of the system (1.4) is uniformly practically f0-equistable, given



holds provided

Since the zero solution of the system (1.3) is f0-equistable, given


such that the inequality

From the condition (ii), assume that

also we can choose


To show that the zero solution of (1.1) is practically f0-equistable. It must be show that for


holds, provided that


Suppose that is false, then there exists a solution




Let

From the condition (iii) we obtain the differential inequality
Let
Applying the comparison Theorem of [1] , yields
To prove that
It must be show that
Choose

From (5.3) at

From the condition (ii) and (5.6), at

From (5.5), (5.8) and (5.9), we get
From (5.2), we get

Then from the condition (ii), (5.4), (5.6) and (5.10), we get at
which leads to a contradiction, then it must be
holds, provided that

Acknowledgements
The authors would thank referees the manuscript for a valuable corrections of it.
Cite this paper
A. A.Soliman,W. F.Seyam, (2015) On Stability of Nonlinear Differential System via Cone-Perturbing Liapunov Function Method. Applied Mathematics,06,1769-1780. doi: 10.4236/am.2015.610157
References
- 1. El Sheikh, M.M.A., Soliman, A.A. and Abd Alla, M.H. (2000) On Stability of Non Linear Systems of Ordinary Differential Equations. Applied Mathematics and Computation, 113, 175-198.
http://dx.doi.org/10.1016/S0096-3003(99)00082-X - 2. Soliman, A.A. (2002) On Total Φ0-Stability of Non Linear Systems of Differential Equations. Applied Mathematics and Computation, 130, 29-38.
http://dx.doi.org/10.1016/S0096-3003(01)00087-X - 3. Soliman, A.A. (2003) On Total Stability of Perturbed System of Differential Equations. Applied Mathematics Letters, 16, 1157-1162.
http://dx.doi.org/10.1016/S0893-9659(03)90110-8 - 4. Soliman, A.A. (2005) On Practical Stability of Perturbed Differential Systems. Applied Mathematics and Computation, 163, 1055-1060.
http://dx.doi.org/10.1016/j.amc.2004.06.073 - 5. Akpan, E.P. and Akinyele, O. (1992) On Φ0-Stability of Comparison Differential Systems. Journal of Mathematical Analysis and Applications, 164, 307-324.
http://dx.doi.org/10.1016/0022-247X(92)90116-U - 6. Adeyeye, J.O. (2001) On Cone-Valued Lyapanov Functions and Stability in Two Measures for Integro-Differential Systems. Nonlinear Analysis, 47, 4835-4843.
http://dx.doi.org/10.1016/S0362-546X(01)00595-8 - 7. Lakshmikantham, V. and Leela, S. (1976) On Perturbing Liapunov Functions. Journal of Systems Theory, 10, 85-90.
http://dx.doi.org/10.1007/BF01683265 - 8. Lakshmikantham, V. and Leela, S. (1969) Differential and Integeral Inequalities. Vol. I, Academic Press, New York.









































































































