

1. Introduction
The notion of Courant algebroid was introduced in [1] to study the double of Lie bialgebroids. Equivalent definition was given by Roytenberg [2]. In resent years, with the development and exploration of the theory of categorified Lie algebras, or “Lie 2-algebras”, Courant algebroids have been far and wide studied from several aspects and have been found many applications in the theory of Manin pairs and moment maps [3] [4]; generalized complex structures [5];  -algebras and symplectic supermanifolds [2]; gerbes [6] as well as BV algebras and topological field theories.
-algebras and symplectic supermanifolds [2]; gerbes [6] as well as BV algebras and topological field theories.
But these articles just introduced the Courant algebroids and Dorfman algebroids. And they did not find the relation between the higher-order Courant algebroids and the higher-order Dorfman algebroids.
The standard Courant algebroid is the direct sum bundle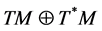 . The standard Courant bracket is given by
. The standard Courant bracket is given by
 (1)
(1)
However, many experts know that on the direct sum bundle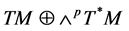 , there is also a similar bracket operation, i.e.
, there is also a similar bracket operation, i.e.
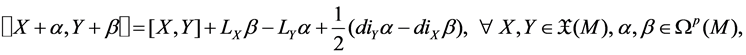
which we call the higher-order Courant bracket. We have proved that the Jacobi identity holds up to an exact term.
So in our paper, we introduce the higher-order Courant algebroids and Dorfman algebroids, and find their relation. In Section 2, we review the higher-order Courant bracket and higher-order Courant Dorfman bracket. Also we review the basic definitions about the Dorfman algebroids and Lie 2-algebras. In Section 3, we intro- duce emphatically the equivalence between the higher-order Courant algebroids and higher-order Dorfman algebroids. In Thm. 3.1, we construct a Lie 2-algebra which is “semistrict”, meaning that the bracket is skew- symmetric, but the Jacobi identity holds only up to isomorphism, where the Lie bracket of observables is given instead by the higher-order Courant bracket. In Thm. 3.2, we construct another Lie 2-algebra with the same objects and morphisms, where the Lie bracket of observables is given instead by the higher-order Dorfman bracket. In Thm. 3.3, we show that these two Lie 2-algebras are isomorphic.
2. Preliminaries
In this section, we introduce the higher-order Courant and Dorfman bracket on the direct sum bundle  and associated properties. Also we review the definition of Lie 2-algebras.
and associated properties. Also we review the definition of Lie 2-algebras.
First, there is a natural 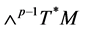 -valued nondegenerate symmetric pairing
-valued nondegenerate symmetric pairing 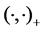 on the direct sum bundle
on the direct sum bundle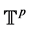 ,
,
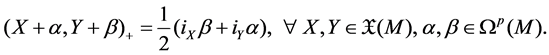 (2)
(2)
The higher-order Courant bracket satisfies some similar properties as the Courant bracket.
Theorem 2.1. [7] For any 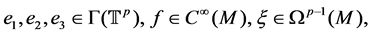 we have
we have
1)  where
where 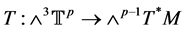 is defined by
is defined by

2) 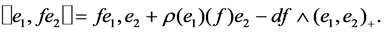
3) ![]()
4) ![]()
Second, we introduce the following higher-order Dorfman bracket,
![]() (3)
(3)
The higher-order Dorfman bracket also satisfies similar properties as the usual Dorfman bracket.
Theorem 2.2. [7] 1) For any ![]() we have
we have
![]()
2) The Dorfman bracket ![]() is a Leibniz bracket, i.e. for any
is a Leibniz bracket, i.e. for any ![]()
![]()
Consequently, ![]() is a Dorfman algebroid.
is a Dorfman algebroid.
3) The pairing (2) and the higher-order Dorfman bracket is compatible in the following sense,
![]() (4)
(4)
Third, we review some definitions about the Lie 2-algebra.
Definition 2.3. [8] A Lie 2-algebra is a 2-term chain complex of vector spaces ![]() equipped with the following structure:
equipped with the following structure:
1) A chain map ![]() which called the bracket;
which called the bracket;
2) A chain homotopy ![]() which called the alternator;
which called the alternator;
3) An antisymmetric chain homotopy ![]() which called the Jacobiator.
which called the Jacobiator.
In addition, the following diagrams are required to commute:
![]()
![]()
Definition 2.4. [8] A Lie 2-algebra for which the Jacobiator is the identity chain homotopy is called hemistrict. One for which the alternator is the identity chain homotopy is called semistrict.
Definition 2.5. [8] Given Lie 2-algebras L and ![]() with bracket, alternator and Jacobiator
with bracket, alternator and Jacobiator ![]()
![]() and
and ![]() respectively, a homomorphism from L to
respectively, a homomorphism from L to ![]() consists of:
consists of:
1) A Chain map ![]() and
and
2) A chain homotopy ![]()
such that the following diagrams commute:
![]()
3. The Equivalence between p-Order Courant Algebroid and p-Order Dorfman Algebroid
In this section, we construct a “semistrict” Lie 2-algebra and a “hemistric” Lie 2-algebra and give the relation between p-order Courant algebroids and p-order Dorfman algebroids. We all know that ![]() is a p- order Courant algebroid, and
is a p- order Courant algebroid, and ![]() is a p-order Dorfman algebroid. We shall construct two Lie 2- algebras associated to
is a p-order Dorfman algebroid. We shall construct two Lie 2- algebras associated to![]() : one hemistrict and one semistrict. Then we shall prove these are isomorphic. Both these Lie 2-algebras have the same underlying 2-term complex, namely:
: one hemistrict and one semistrict. Then we shall prove these are isomorphic. Both these Lie 2-algebras have the same underlying 2-term complex, namely:
![]()
where d is the usual exterior derivative of functions. To see that this chain complex is well-defined.
We make L into a semistrict Lie 2-algebra. For this, we use a chain map called the semi-bracket: ![]() In degree 0, the semi-bracket is given as in Equation (1)
In degree 0, the semi-bracket is given as in Equation (1)
![]()
In degrees 1 and 2, we set it equal to zero: ![]()
Theorem 3.1. ![]() is a p-order Courant algebroid, there is a semistrict Lie 2-algebra
is a p-order Courant algebroid, there is a semistrict Lie 2-algebra ![]() where
where
1) The space of 0-chains is ![]()
2) The space of 1-chains is ![]()
3) The differential is the exterior derivative ![]()
4) The bracket is ![]()
5) The alternator is the bilinear map ![]() defined by
defined by ![]()
6) The Jacobiator is the identity, hence given by the trilinear map ![]()
with ![]()
Proof. We note from Equation (1) that the semi-bracket is antisymmetric. Since both S and the degree 1 chain map are zero, the alternator defined above is a chain homotopy with the right source and target. So again, we just need to check that the Lie 2-algebra axioms hold. The following identities can be checked by simple calculation, and the commutativity of the last diagram follows:
![]()
![]() we have
we have
![]()
Since the Jacobiator is antisymmetric and the alternator is the identity, the first and second diagrams com- mute as well. The third diagram commutes because all the edges are identity morphisms. □
Next, the hemistrict Lie 2-algebra comes with a bracket called the hemi-bracket: ![]() In degree 0, the hemi-bracket is given as Dorfman bracket:
In degree 0, the hemi-bracket is given as Dorfman bracket:
![]()
In degree 1, it is given by: ![]() In degree 2, we necessarily have
In degree 2, we necessarily have![]() , where
, where ![]() while
while ![]()
To see that the hemi-bracket is in fact a chain map, it suffices to check it on hemi-brackets of degree 1:
![]()
Theorem 3.2. ![]() is a p-order Dorfman algebroid, there is a hemistrict Lie 2-algebra
is a p-order Dorfman algebroid, there is a hemistrict Lie 2-algebra ![]() where
where
1) The space of 0-chains is ![]()
2) The space of 1-chains is ![]()
3) The differential is the exterior derivative ![]()
4) The bracket is ![]()
5) The alternator is the bilinear map ![]() defined by
defined by ![]()
6) The Jacobiator is the identity, hence given by the trilinear map ![]() with
with ![]()
Proof That S is a chain homotopy with the right source and target follows from thm. (2.2) and the fact that:
![]()
Thm. (2.2) also says that the Jacobi identity holds. The following equations then imply that J is also a chain homotopy with the right source and target:
![]()
![]()
![]()
So, we just need to check that the Lie 2-algebra axioms hold. The first and the last two diagrams commute since each edge is the identity. The commutativity of the second diagram from thm. (2.2) (3) is shown as follows:
![]()
The third diagram says that ![]() and this follows from the fact that the alternator is symmetric:
and this follows from the fact that the alternator is symmetric:![]() . □
. □
Theorem 3.3. ![]() and
and ![]() are isomorphic as Lie 2-algebras.
are isomorphic as Lie 2-algebras.
Proof. We show that the identity chain maps with appropriate chain homotopies define Lie 2-algebra ho- momorphisms and that their composites are the respective identity homomorphisms. There is a homomorphism ![]() with the identity chain map and the chain homotopy given by
with the identity chain map and the chain homotopy given by ![]() This is a chain homotopy follows from the bracket relation
This is a chain homotopy follows from the bracket relation ![]() noted in Equation (3) together with the equations
noted in Equation (3) together with the equations
![]()
We check that the two diagrams in the definition of a Lie 2-algebra homomorphism commute. Noting that the chain map ![]() is the identity, the commutativity of the first diagram is easily checked by recalling that
is the identity, the commutativity of the first diagram is easily checked by recalling that ![]() and
and ![]() are the identities. Noting that any edge given by the bracket for
are the identities. Noting that any edge given by the bracket for ![]() in degree 1 is the identity and that
in degree 1 is the identity and that ![]() is the identity, we easily check the commutativity of the first diagram:
is the identity, we easily check the commutativity of the first diagram:
![]()
To check the commutativity of the second diagram we only need to perform the following calculation:
![]()
The second diagram meet commutativity if and only if the following equation established:
![]()
Clockwise from the upper left to the lower right corner:
![]()
Counterclockwise from the upper left to the lower right corner:
![]()
Because of the commutativity of the second diagram we must have the the following equation:
![]()
![]()
because of ![]() we get the following calculations:
we get the following calculations:
![]() (5)
(5)
if we choose ![]()
![]()
□
Acknowledgements
We give warmest thanks to Zhangju Liu and Yunhe Sheng for useful comments and discussion. We also thanks the referees for very helpful comments.
Funding
Research was partially supported by NSF of China (11126338, 11461047, 11201218).