Asymptotic Behavior of Stochastic Strongly Damped Wave Equation with Multiplicative Noise

Abstract

In this paper we study the asymptotic dynamics of the stochastic strongly damped wave equation with multiplicative noise under homogeneous Dirichlet boundary condition. We investigate the existence of a compact random attractor for the random dynamical system associated with the equation.

Share and Cite:

Wang, Z. and Zhou, S. (2015) Asymptotic Behavior of Stochastic Strongly Damped Wave Equation with Multiplicative Noise. International Journal of Modern Nonlinear Theory and Application, 4, 204-214. doi: 10.4236/ijmnta.2015.43015.

1. Introduction

Consider the following stochastic strongly damped wave equation with multiplicative noise:

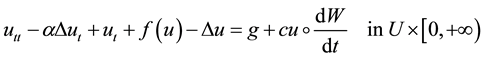(1.1)

with the homogeneous Dirichlet boundary condition

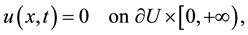(1.2)

and the initial value conditions

(1.3)

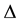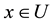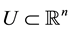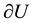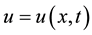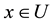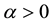
where is the Laplacian with respect to the variable, is a bounded open set with a smooth boundary; is a real function of and; are strong damping coefficients; denotes the Stratonovich sense of the stochastic term; 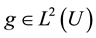is a given external force;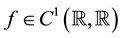, 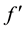are uniformly bounded and there exist such that

(1.4)

(1.5)

where denotes the absolute value of number in. is a one-dimensional two-sided real-valued Wiener process on probability space, where

the Borel -algebra on is generated by the compact open topology, and is the corresponding Wiener measure on. We identify with, i.e., When and Equation (1.1) can be regarded as a stochastic perturbed model of a continuous Josephson junction [1] , which is stochastic damped sine-Gordon equation [2] .

A large amount of studies have been carried out toward the dynamics of a variety of systems related to Equation (1.1). For example, the asymptotical behavior of solutions for deterministic and stochastic wave equations has been studied by many authors, see, e.g. [3] - [27] and the references therein.

In this paper we study the existence of a global random attractor for stochastic strongly damped wave equations with multiplicative noise. The coefficient of the noise term needs to be suitable small,

which is different from that in stochastic strongly damped wave equations with additive noise, this is because the multiplicative noise depends on the state variable but the additive noise term is independent of.

This paper is organized as follows. In the next section, we recall some basic concepts and properties for general random dynamical systems. In Section 3, we provide some basic settings about Equation (1.1) and show that it generates a random dynamical system in proper function space. Section 4 is devoted to proving the existence of a unique random attractor of the random dynamical system.

2. Preliminaries

In this section, we collect some basic knowledge about general random dynamical systems (see [28] [29] for details).

Let be a separable Hilbert space with Borel -algebra. Let be a probability space as in Section 1. Define on via

then is an ergodic metric dynamical system [28] [29] .

In the following, a property holds for -a.e. means that there is with and for.

Definition 2.1 A continuous random dynamical system on X over is a mapping

which is -measurable and satisfies, for -a.e.,

1) is the identity on;

2) for all;

3) is continuous for all.

Definition 2.2 (See [29] ).

1) A set-valued mapping is said to be a random set if the mapping is measurable for any. If is also closed (compact) for each,

is called a random closed (compact) set. A random set is said to be bounded if there exist and a random variable such that

2) A random set is called tempered provided for -a.e.,

3) A random set is said to be a random absorbing set if for any tempered random set, and -a.e., there exists such that

4) A random set is said to be a random attracting set if for any tempered random set, and -a.e., we have

where is the Hausdorff semi-distance given by for any.

5) A random compact set is said to be a random attractor if it is a random attracting set and for -a.e. and all.

Theorem 2.3 (See [29] ). Let be a continuous random dynamical system on over. If there is a tempered random compact absorbing set of, then is a compact random attractor of, where

Moreover, is the unique random attractor of.

3. Stochastic Strongly Damped Wave Equation

In this section, we outline the basic setting of (1.1)-(1.2) and show that it generates a random dynamical system.

Define an unbounded operator

Clearly, is a self-adjoint, positive linear operator with the eigenvalues:

It is well known that generates an analytic semigroup of bounded linear operators on.

Let, endowed with the usual norm

(3.1)

where denotes the usual norm in and stands for the transposition.

It is convenient to reduce (1.1) to an evolution equation of the first order in time

(3.2)

For our purpose, it is convenient to convert the problems (1.1)-(1.2) into a deterministic system with a random parameter, and then show that it generates a random dynamical system.

We now introduce an Ornstein-Uhlenbeck process given by the Brownian motion. Put

(3.3)

which is called Ornstein-Uhlenbeck process and solves the Itô equation

(3.4)

From [30] [31] , it is known that the random variable is tempered, and there is a -invariant set of full measure such that is continuous in for every.

Lemma 3.1 (See [7] ). For the Ornstein-Uhlenbeck process in Equation (3.3), we have the following results

(3.5)

(3.6)

(3.7)

To show that problem (3.2) generates a random dynamical system, we let

which is a given positive number, then problems (1.1)-(1.2) can be rewritten as the equivalent system with random coefficients but without multiplicative noise on,

(3.8)

which has the following vector form

(3.9)

where

We will consider Equation (3.8) or (3.9) for and write as from now on.

By the classical theory concerning the existence and uniqueness of the solutions [17] [32] , one may show that under conditions (1.4)-(1.5), for each, problem (3.9) has a unique solution which is continuous with respect to in for all. Then the solution mapping

(3.10)

generates a continuous random dynamical system over on.

Introduce the homeomorphism, whose inverse homeomorphism is,. Then the transformation

(3.11)

also generates a continuous random dynamical system associated with the problem (3.2) on.

Note that the two random dynamical systems and are equivalent. By transformation (3.11), it is easy to see that has a random attractor provided possesses a random attractor. Thus, we only need to consider the random dynamical system.

4. Random Attractor

In this section, we study the existence of a random attractor. Throughout this section we assume that is the collection of all tempered random subsets of and

(4.1)

For our purpose, we introduce a new norm by

(4.2)

for, where and is chosen such that in which is a small positive number. It is easy to check that is equivalent to the usual norm on in (3.1). For, , let

(4.3)

where denotes the inner product on. By the Poincaré inequality

(4.4)

Equation (4.3) is then positive definite.

Now, we present a property of the operator in that plays an important role in this article.

Lemma 4.1 Let. There exists a small positive constant such that for any,

(4.5)

The proof of Lemma 4.1 is similar to that of Lemma 1 in [24] . We hence omit it here.

Lemma 4.2 Assume that, conditions (1.4), (1.5) and (4.1) hold. Then, there exists a random ball centered at 0 with random radius such that for any, there is a

such that for any satisfies for -a.e. and all,

(4.6)

Proof. Take the inner product of problem (3.9) with. By the Cauchy-Schwartz inequality and the Young inequality, we find that

where is the volume of the set.

By using the Poincaré inequality (4.4), we have that

By all the above inequalities and Lemma 4.1, we have

(4.7)

By the Gronwall lemma, we have that, for all,

(4.8)

By replacing by, we get from problem (4.8) that,

(4.9)

By inequality (4.1), it is easy to see that

(4.10)

It then follows from inequality (4.10), Lemma 3.1, and that

(4.11)

By Lemma 3.1, inequality (4.10) and, we have

(4.12)

We choose

(4.13)

Then, for any tempered random set, there exists a such that for any, satisfies for -a.e., ,

(4.14)

So, the proof is completed.

We now construct a random compact attracting set for RDS. For this purpose, we decompose the solution of Equation (3.9) with the initial value into two parts

, satisfy, respectively

(4.15)

(4.16)

Lemma 4.3 Assume that, conditions (1.4), (1.5) and (4.1) hold. Then, for any, and, we have for -a.e.,

(4.17)

and there exist a tempered random variable and such that for -a.e. and all,

(4.18)

where and satisfy Equations (4.15), (4.16).

Proof. We first take the inner product of Equation (4.15) with. By Lemma 4.1, we obtain

(4.19)

Then by and, we have

(4.20)

Thus, the first assertion is valid.

Next, we take the inner product of Equation (4.16) with. From the positivity of the operator, we easily obtain

(4.21)

By the Cauchy-Schwartz inequality and the Young inequality, we find that

By using inequality (4.4), we have that

Combining all the above inequalities and inequality (4.21), we have

(4.22)

Using the Gronwall lemma, for all, we get

(4.23)

Replacing by we get from the above that,

(4.24)

By Lemma 3.1, inequality (4.10) and, we have

(4.25)

We can choose

(4.26)

then the second assertion is valid.

By Lemma 4.2 and Lemma 4.3, for any, , , and for some constant, we have for -a.e.,

(4.27)

where. Let be a closed ball of:

(4.28)

Then, by the compact embedding of into, is compact in.

Note that

(4.29)

Then by Lemma 4.3 and inequality (4.27), we have for -a.e.

(4.30)

which implies that is a random compact attracting set for. It follows from Equations (4.13) and (4.26) that is tempered. Thus by Theorem 2.3, the main result of this section can now be stated as follows.

Theorem 4.4 Assume that, conditions (1.4), (1.5) and (4.1) hold. Then, the random dynamical system has a unique compact random attractor in, where

in which is a tempered random compact attracting set for.

Supported

The authors are supported by National Natural Science Foundation of China (Nos. 11326114, 11401244, 11071165 and 11471290); Natural Science Research Project of Ordinary Universities in Jiangsu Province (No. 14KJB110003); the Foundation of Zhejiang Normal University (No. ZC304011068).

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1] Lomdahl, P.S., Soerensen, O.H. and Christiansen, P. L. (1982) Soliton Excitations in Josephson Tunnel Junctions. Physical Review B, 25, 5337-5348.
[2] Shen, Z.W., Zhou, S.F. and Shen, W.X. (2010) One-Dimensional Random Attractor and Rotation Number of the Stochastic Damped Sine-Gordon Equation. Journal of Differential Equations, 248, 1432-1457.
http://dx.doi.org/10.1016/j.jde.2009.10.007
[3] Chen, F.X., Guo, B.L. and Wang, P. (1998) Long Time Behavior of Strongly Damped Nonlinear Wave Equations. Journal of Differential Equations, 147, 231-241.
http://dx.doi.org/10.1006/jdeq.1998.3447
[4] Chow, P.-L. (2002) Stochastic Wave Equation with Polynomial Nonlinearity. The Annals of Applied Probability, 12, 361-381.
http://dx.doi.org/10.1214/aoap/1015961168
[5] Ghidaglia, J.M. and Marzocchi, A. (1991) Longtime Behavior of Strongly Damped Nonlinear Wave Equations, Global Attractors and Their Dimension. SIAM Journal on Mathematical Analysis, 22, 879-895.
http://dx.doi.org/10.1137/0522057
[6] Kalantarov, V. and Zelik, S. (2009) Finite-Dimensional Attractors for the Quasi-Linear Strongly-Damped Wave Equation. Journal of Differential Equations, 247, 1120-1155.
http://dx.doi.org/10.1016/j.jde.2009.04.010
[7] Fan, X.M. (2006) Attractors for a Damped Stochastic Wave Equation of Sine-Gordon Type with Sublinear Multiplicative Noise. Stochastic Analysis and Applications, 24, 767-793.
http://dx.doi.org/10.1080/07362990600751860
[8] Fan, X.M. (2004) Random Attractor for a Damped Sine-Gordon Equation with White Noise. Pacific Journal of Mathematics, 216, 63-76.
http://dx.doi.org/10.2140/pjm.2004.216.63
[9] Fan, X.M. and Wang, Y. (2007) Fractal Dimensional of Attractors for a Stochastic Wave Equation with Nonlinear Damping and White Noise. Stochastic Analysis and Applications, 25, 381-396.
http://dx.doi.org/10.1080/07362990601139602
[10] Fan, X.M. (2008) Random Attractors for Damped Stochastic Wave Equations with Multiplicative Noise. International Journal of Mathematics, 19, 421-437.
http://dx.doi.org/10.1142/S0129167X08004741
[11] Li, H.Y. and Zhou, S.F. (2007) One-Dimensional Global Attractor for Strongly Damped Wave Equations. Communications in Nonlinear Science and Numerical Simulation, 12, 784-793.
http://dx.doi.org/10.1016/j.cnsns.2005.06.007
[12] Li, H.Y. and Zhou, S.F. (2008) On Non-Autonomous Strongly Damped Wave Equations with a Uniform Attractor and Some Averaging. Journal of Mathematical Analysis and Applications, 341, 791-802.
http://dx.doi.org/10.1016/j.jmaa.2007.10.051
[13] Lu, K.N. and Schmalfuß, B. (2007) Invariant Manifolds for Stochastic Wave Equations. Journal of Differential Equations, 236, 460-492.
http://dx.doi.org/10.1016/j.jde.2006.09.024
[14] Lv, Y. and Wang, W. (2008) Limiting Dynamics for Stochastic Wave Equations. Journal of Differential Equations, 244, 1-23.
http://dx.doi.org/10.1016/j.jde.2007.10.009
[15] Massatt, P. (1983) Limiting Behavior for Strongly Damped Nonlinear Wave Equations. Journal of Differential Equations, 48, 334-349.
http://dx.doi.org/10.1016/0022-0396(83)90098-0
[16] Pata, V. and Zelik, S. (2006) Smooth Attractors for Strongly Damped Wave Equations. Nonlinearity, 19, 1495-1506.
http://dx.doi.org/10.1088/0951-7715/19/7/001
[17] Temam, R. (1988) Infinite Dimensional Dynamical Systems in Mechanics and Physics. Springer, Berlin.
http://dx.doi.org/10.1007/978-1-4684-0313-8
[18] Wang, B.X. and Gao, X.L. (2009) Random Attractors for Wave Equations on Unbounded Domains. Discrete and Continuous Dynamical Systems, Special, 800-809.
[19] Yang, M.H. and Sun, C.Y. (2009) Attractors for Strongly Damped Wave Equations. Nonlinear Analysis: Real World Applications, 10, 1097-1100.
http://dx.doi.org/10.1016/j.nonrwa.2007.12.001
[20] Yang, M.H. and Sun, C.Y. (2010) Exponential Attractors for the Strongly Damped Wave Equations. Nonlinear Analysis: Real World Applications, 11, 913-919.
http://dx.doi.org/10.1016/j.nonrwa.2009.01.022
[21] Yang, M.H. and Sun, C.Y. (2009) Dynamics of Strongly Damped Wave Equations in Locally Uniform Spaces: Attractors and Asymptotic Regularity. Transactions of the American Mathematical Society, 361, 1069-1101.
http://dx.doi.org/10.1090/S0002-9947-08-04680-1
[22] Yang, M.H., Duan, J.Q. and Kloeden, P. (2011) Asymptotic Behavior of Solutions for Random Wave Equations with Nonlinear Damping and White Noise. Nonlinear Analysis: Real World Applications, 12, 464-478.
http://dx.doi.org/10.1016/j.nonrwa.2010.06.032
[23] Zhou, S.F. (1999) Dimension of the Global Attractor for Strongly Damped Nonlinear Wave Equation. Journal of Mathematical Analysis and Applications, 233, 102-115.
http://dx.doi.org/10.1006/jmaa.1999.6269
[24] Zhou, S.F. (1999) Global Attractor for Strongly Damped Nonlinear Wave Equation. Functional Differential Equations, 6, 451-470.
[25] Zhou, S.F. and Fan, X.M. (2002) Kernel Sections for Non-Autonomous Strongly Damped Wave Equations. Journal of Mathematical Analysis and Applications, 275, 850-869.
http://dx.doi.org/10.1016/S0022-247X(02)00437-7
[26] Zhou, S.F. (2003) Attractors for Strongly Damped Wave Equations with Critical Exponent. Applied Mathematics Letters, 16, 1307-1314.
http://dx.doi.org/10.1016/S0893-9659(03)90134-0
[27] Zhou, S.F., Yin, F.Q. and Ouyang, Z.G. (2005) Random Attractor for Damped Nonlinear Wave Equations with White Noise. SIAM Journal on Applied Dynamical Systems, 4, 883-903.
http://dx.doi.org/10.1137/050623097
[28] Arnold, L. (1998) Random Dynamical Systems. Springer-Verlag, New York and Berlin.
http://dx.doi.org/10.1007/978-3-662-12878-7
[29] Chueshov, I. (2002) Monotone Random Systems Theory and Applications. Springer-Verlag, New York.
http://dx.doi.org/10.1007/b83277
[30] Bates, P.W., Lu, K.N. and Wang, B.X. (2009) Random Attractors for Stochastic Reaction-Diffusion Equations on Unbounded Domains. Journal of Differential Equations, 246, 845-869.
http://dx.doi.org/10.1016/j.jde.2008.05.017
[31] Duan, J.Q., Lu, K.N. and Schmalfuss, B. (2003) Invariant Manifolds for Stochastic Partial Differential Equations. The Annals of Probability, 31, 2109-2135.
http://dx.doi.org/10.1214/aop/1068646380
[32] Pazy, A. (1983) Semigroup of Linear Operators and Applications to Partial Differential Equations. Springer-Verlag, New York.
http://dx.doi.org/10.1007/978-1-4612-5561-1

Journals Menu
Follow SCIRP
Contact us
+1 323-425-8868
customer@scirp.org
WhatsApp +86 18163351462(WhatsApp)
Click here to send a message to me 1655362766
Paper Publishing WeChat

Copyright © 2024 by authors and Scientific Research Publishing Inc.

Creative Commons License

This work and the related PDF file are licensed under a Creative Commons Attribution 4.0 International License.