1. Introduction
Following Ashcroft and Mermin [1] , we regard the conduction electron (“electron” or “hole”) as a wave packet. Dirac showed in his classic book [2] Principles of Quantum Mechanics that, the quantum wave packet moves, following classical mechanical laws. Dirac’s theorem is distinct from Ehrenfest’s theorem [3] that the quantum average of a dynamical variable follows a classical mechanical’s law of motion. Dirac’s theorem requires a unit cell. We shall show in the present work that if we regard the rectangular-box unit cell for the orthorhombic (ORC) crystal as the wave packet, then the “electron” (“hole”) moves, following the classical equations of motion.
In simple cubic (SC), tetragonal (TET) and orthorhombic (ORC) crystals, the lattices have natural orthogonal axes. Their unit cells are different only in having one (1), two (2) and three (3) different sides. Elements Po (Pa) form SC (TET) crystals.
In Section 2 an electron in electric and magnetic fields, a conduction electron in solids, and crystal lattice structures are introduced and summarized. In Section 3 a theory of electron dynamics for an ORC lattice is developed. Choosing the rectangular-box unit cell as the wave packet, we establish that there are 3D k-vectors. The results are summarized, using Bloch theorem [4] . In Section 4 energy bands and gaps are calculated for 1D and 3D Kronig-Penny models [5] . Summary and discussion are given in the final section.
2. Preliminaries
2.1. An Electron in Electromagnetic Fields
Let us take a classical electron in a free space moving in the electric field 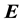 and a magnetic field
and a magnetic field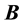 . The Lorentz force
. The Lorentz force 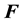 is
is
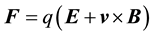 , (1)
, (1)
where 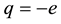 is the charge and
is the charge and
 (2)
(2)
is the electron velocity. We introduce a vector potential 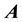 and a scalar potential
and a scalar potential 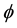 that generate the electric field
that generate the electric field 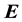 and a magnetic field
and a magnetic field 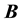 by
by
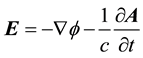 , (3)
, (3)
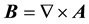 , (4)
, (4)
where
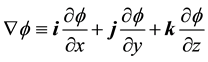 (5)
(5)
 . (6)
. (6)
Note that the Cartesian coordinates 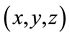 are used here. The del operator
are used here. The del operator 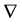 is undefined for non- orthogonal coordinates.
is undefined for non- orthogonal coordinates.
In Hamiltonian formulation a Hamiltonian ![]() is defined:
is defined:
![]() . (7)
. (7)
The equations of motion are derived from
![]() . (8)
. (8)
We may quantize the dynamics by introducing the fundamental commutation rules:
![]() , (9)
, (9)
where j and k indicate components:![]() ,
,![]() .
.
2.2. A Conduction Electron
Wigner and Seitz used a primitive unit cell and lattice periodicity to obtain the ground-state energy of a metal [6] . Let us consider a conduction electron moving in a SC crystal. It is natural to choose the Cartesian coordinates ![]() along the cubic lattice axes. The unit cell is a cube with the side-length
along the cubic lattice axes. The unit cell is a cube with the side-length![]() , called the lattice constant. The ORC crystal has orthogonal axis with different side lengths:
, called the lattice constant. The ORC crystal has orthogonal axis with different side lengths:![]() ,
, ![]() ,
,![]() . The lattice potential
. The lattice potential ![]() is lattice-periodic if
is lattice-periodic if ![]() is translation-invariant:
is translation-invariant:
![]() , (10)
, (10)
where the Bravais vector
![]() , (11)
, (11)
is specified by integers-set![]() ;
;![]() .
.
The cubic cell may be chosen as the wave packet for the conduction electron. The center of mass of the wave packet is expected to move, following Hamilton’s equation of motion. The rigorous proof will be given later. Following Ashcroft and Mermin, we may set up a model of electron dynamics in solids. It is necessary to introduce ![]() -vectors:
-vectors:
![]() , (12)
, (12)
where ![]() are the orthonormal unit vectors, since k-vectors are involved in the equation of motion:
are the orthonormal unit vectors, since k-vectors are involved in the equation of motion:
![]() , (13)
, (13)
where ![]() and
and ![]() are the electric and magnetic fields, respectively. The vector
are the electric and magnetic fields, respectively. The vector
![]() (14)
(14)
is the electron velocity, where ![]() is the energy.
is the energy.
If an electron is in a continuous energy range (energy band), then it will be accelerated by the electric force, following Equation (13), and the material is a conductor. If the electron’s energy is discrete and is in a forbidden band (energy gap), it does not move under a small electric force, and the material is a insulator. If the acceleration occurs only for a mean free time![]() , that is, the inverse of the scattering frequency, the conductivity
, that is, the inverse of the scattering frequency, the conductivity ![]() for a simple metal is given by Drude’s formula [7] :
for a simple metal is given by Drude’s formula [7] :
![]() , (15)
, (15)
where n is the electron density and ![]() the effective mass.
the effective mass.
For some crystals such as simple cubic (SC), face-centered-cubic (FCC), body-centered-cubic (BCC), tetragonal (TET) and ORC crystals, the choice of the orthogonal ![]() -axis and the unit cells are obvious. The 2D crystals can also be treated similarly, only the
-axis and the unit cells are obvious. The 2D crystals can also be treated similarly, only the ![]() -component being dropped.
-component being dropped.
3. Theory
We assume that a wave packet is composed of superposable plane waves characterized by k-vectors. The superposability is the basic property of the Schrödinger wave function in free space. A monoclinic (MCL) crystal can be generated from a TET crystal by distorting the rectangular faces perpendicular to the c-axis into parallelograms. Material plane waves proceeding along the c-axis exist since the x-y planes containing atoms are periodic in the z-direction in equilibrium. It has then one-dimensional (1D) k-vectors. In the x-y plane there is an oblique net whose corners are occuried by V’s for MCL (VO2). The Bravais vector may be defined by
![]() , (16)
, (16)
where ![]() and
and ![]() are non-orthogonal base vectors. In the field theoretical formulation the field point
are non-orthogonal base vectors. In the field theoretical formulation the field point ![]() is given by
is given by
![]() , (17)
, (17)
where ![]() is the point defined within the standard unit cell. Equation (16) describes the 2D lattice periodicity but does not establish k-space as explained below.
is the point defined within the standard unit cell. Equation (16) describes the 2D lattice periodicity but does not establish k-space as explained below.
To see this clearly, we first consider an electron in a simple square (SQ) lattice. The Schrödinger equation is
![]() , (18)
, (18)
where the potential energy is periodic:
![]() , (19)
, (19)
where![]() . If we choose a set of Cartesian coordinates
. If we choose a set of Cartesian coordinates![]() , then the Laplacian term in Equation (18) is given by
, then the Laplacian term in Equation (18) is given by
![]() , (20)
, (20)
a key step for the separation-of-variable method. If we choose a periodic square boundary with the side length ![]() (N: integer), then there are 2D Fourier transforms and (2D) k-vectors.
(N: integer), then there are 2D Fourier transforms and (2D) k-vectors.
We now go back to the original rhombic system. If we choose the x-axis along either ![]() or
or![]() , then the potential energy field
, then the potential energy field ![]() is periodic in the x-direction but it is aperiodic in the y-direction. For a infinite system the only acceptable boundary for the Fourier transformation is the periodic boundary condition. Hence there is no 2D k-space.
is periodic in the x-direction but it is aperiodic in the y-direction. For a infinite system the only acceptable boundary for the Fourier transformation is the periodic boundary condition. Hence there is no 2D k-space.
In SC, TET, and ORC crystals the lattices have natural orthogonal axes. We first take a rectangular 2D lattice. If the potential energy ![]() is the sum of x- and y-dependent terms such that
is the sum of x- and y-dependent terms such that
![]() , (21)
, (21)
then the energy-eigenvalue Schrödinger equation:
![]() , (22)
, (22)
where E is the energy, is separable:
![]() . (23)
. (23)
The 1D Schrödinger equation in x is
![]() , (24)
, (24)
where ![]() is lattice-periodic:
is lattice-periodic:
![]() . (25)
. (25)
Clearly the wave function ![]() also satisfies the same equation. Therefore,
also satisfies the same equation. Therefore, ![]() is likely to be different from
is likely to be different from ![]() only by an x-independent phase:
only by an x-independent phase:
![]() , (26)
, (26)
where k is a real number, see below. Equation (26) represents a form of the Bloch theorem [4] . It generates far-teaching consequences in the theory of conduction electrons.
Let us discuss a few physical properties of the Bloch wave function![]() . By taking the absolute square of Equation (26), we obtain
. By taking the absolute square of Equation (26), we obtain
![]() . (27)
. (27)
The following three main properties are observed.
The probability distribution function ![]() is lattice-periodic:
is lattice-periodic:
![]() . (28)
. (28)
The exponential function of a complex number ![]() (y real) is periodic:
(y real) is periodic:
![]() .
.
where m is an integer. We may choose the real number k in Equation (26), called the k-number (![]() times the wave number), to have a fundamental range:
times the wave number), to have a fundamental range:
![]() ; (29)
; (29)
the two end points are called the Brillouin boundary (points).
There are a number of energy gaps (forbidden regions of energy) in which no solutions of Equation (24) exist. The energy eigenvalues E are characterized by the k-number and the zone number (band index) j, which enumerates the energy bands:
![]() . (30)
. (30)
This property (c) is not obvious, and it will be illustrated by examples later.
To further explore the nature of the Bloch wave function![]() , let us write,
, let us write,
![]() , (31)
, (31)
and substitute it into Equation (26). If the function ![]() is lattice-periodic,
is lattice-periodic,
![]() , (32)
, (32)
then Equation (26) is satisfied. Equation (31) represents a second form of the Bloch theorem. The Bloch wave function ![]() has great similarity with the free-particle wave function:
has great similarity with the free-particle wave function:
![]() , (33)
, (33)
where ![]() is a constant. The connection may be illustrated as follows.
is a constant. The connection may be illustrated as follows.
For a free particle, the k-number can range from ![]() to
to![]() , and the energy is
, and the energy is
![]() (34)
(34)
with no gaps. These features are different from the properties (b) and (c).
An important similarity arises when we write the time-dependent wave function ![]() in the running wave form:
in the running wave form:
![]() (35)
(35)
where the frequency ![]() is defined by
is defined by
![]() (36)
(36)
and the amplitude ![]() is defined by
is defined by
![]() (37)
(37)
Equation (35) shows that the Bloch wave function ![]() represents a running wave characterized by k- number k, angular frequency
represents a running wave characterized by k- number k, angular frequency![]() , and wave train
, and wave train![]() .
.
The group velocity ![]() of the Bloch wave packet is given by
of the Bloch wave packet is given by
![]() . (38)
. (38)
By applying the (quantum) principle of wave-particle duality, we say that the Bloch electron moves with the dispersion (energy-momentum) relation:
![]() , (39)
, (39)
The velocity ![]() is given by Equation (38). This gives a picture of great familiarity.
is given by Equation (38). This gives a picture of great familiarity.
The lattice force ![]() averaged over the unit cell is defined and calculated as
averaged over the unit cell is defined and calculated as
![]() (40)
(40)
where the last line is obtained by partial integration. The first term on the right-hand side (r.h.s.) vanishes because both potential ![]() and density
and density ![]() are periodic. When the unit cell is chosen as a wave packet, the minimum definition length of the density
are periodic. When the unit cell is chosen as a wave packet, the minimum definition length of the density ![]() is
is![]() . We may then assume that
. We may then assume that
![]() . (41)
. (41)
Using this, we obtain from Equation (40)
![]() . (42)
. (42)
This property also holds for the y-motion. Extending these results to a 3D motion, we obtain the desired result:
![]() , (43)
, (43)
indicating that the conduction electron moves free from the lattice force. Only the external forces such as the electric and magnetic forces act on the conduction electrons.
Consider an infinite ORC lattice of lattice constants![]() . We choose a Cartesian frame of coordinates
. We choose a Cartesian frame of coordinates ![]() along the lattice axes. The potential
along the lattice axes. The potential ![]() is lattice-periodic:
is lattice-periodic:
![]() (44)
(44)
where the Bravais lattice vector ![]() is defined by
is defined by
![]() . (45)
. (45)
The Schródinger equation is
![]() , (46)
, (46)
The Bloch wave function ![]() satisfies
satisfies
![]() . (47)
. (47)
where ![]() are k-vectors.
are k-vectors.
The three principal properties of the Bloch wave function are:
The probability distribution ![]() is lattice-periodic:
is lattice-periodic:
![]() . (48)
. (48)
The k-vector ![]() has the fundamental ranges:
has the fundamental ranges:
![]() , (49)
, (49)
the end points, which form a rectangular box, are called the Brillouin boundary.
The energy eigenvalues E have energy gaps, and the allowed energies E are characterized by the zone number j and the k-vectors:
![]() , (50)
, (50)
Using Equation (47), we can express the Bloch wave function ![]() in the form:
in the form:
![]() . (51)
. (51)
4. Energy Bands and Gaps
The Bloch energy-eigenvalues in general have bands and gaps. We show this by taking the Kronig-Penny (K-P) model [5] . Let us consider a periodic 1D square-well potential ![]() with depth
with depth ![]() and well width
and well width ![]() as shown in Figure 1:
as shown in Figure 1:
![]() (52)
(52)
The Schrödinger equation can be written as in Equation (24). Since this is a linear homogeneous differential equation with constant coefficients, the wave function ![]() should have the form:
should have the form:
![]() . (53)
. (53)
According to the Bloch theorem, this function ![]() can be written as
can be written as
![]() , (54)
, (54)
![]() , (55)
, (55)
The condition that the function ![]() be continuous and analytic at the well boundary yields the following relationships: [8]
be continuous and analytic at the well boundary yields the following relationships: [8]
![]() , (56)
, (56)
![]() (57)
(57)
By solving Equation (56) with Equation (57), we obtain the eigenvalue E as a function of k. The band edges are obtained from
![]() , (58)
, (58)
which corresponds to the limits of![]() . Numerical studies of Equations (56) and (57) indicate that (1) there are, in general, a number of negative- and positive-energy bands; (2) at each band edge, an effective mass
. Numerical studies of Equations (56) and (57) indicate that (1) there are, in general, a number of negative- and positive-energy bands; (2) at each band edge, an effective mass ![]() can be defined, whose value can be positive or negative and whose absolute value can be greater or less than the electron mass m; and (3) the effective mass is positive at the lower edge of each band, and it is negative at the upper edge. A typical dispersion relation for the model, showing energy bands and gaps, are shown in Figure 2.
can be defined, whose value can be positive or negative and whose absolute value can be greater or less than the electron mass m; and (3) the effective mass is positive at the lower edge of each band, and it is negative at the upper edge. A typical dispersion relation for the model, showing energy bands and gaps, are shown in Figure 2.
At the lowest band edge ![]() we have
we have
![]() . (59)
. (59)
Near this edge the dispersion (energy-k) relation calculated from Equation (56) is
![]() (60)
(60)
![]() . (61)
. (61)
This one-dimensional K-P model can be used to study a simple 3D system. Let us take an ORC lattice of unit lengths![]() , with each lattice point representing a short-range attractive potential center (ion). The Schrödinger equation is hard to solve.
, with each lattice point representing a short-range attractive potential center (ion). The Schrödinger equation is hard to solve.
![]()
Figure 2. ![]() -
-![]() diagram showing energy bands and forbidden gaps.
diagram showing energy bands and forbidden gaps.
Let us now construct a model potential ![]() defined by
defined by
![]() (62)
(62)
![]() (63)
(63)
Here the n are integers. A similar two-dimensional model is shown in Figure 3. In 3D the domains in which ![]() are parallel plates of thickness
are parallel plates of thickness ![]()
![]() separated by
separated by ![]() in the direction
in the direction![]() ,
,![]() . The intersection of any two plates are straight beams of cross section
. The intersection of any two plates are straight beams of cross section![]() , where the potential
, where the potential ![]() has the value
has the value![]() . The intersections of three plates, where the potential
. The intersections of three plates, where the potential ![]() has the value
has the value![]() , are cubes of side length
, are cubes of side length![]() . The set of these cubes form an ORC lattice, a configuration similar to that of the commercially available molecular lattice model made up of balls and sticks. Note: Each square-well potential
. The set of these cubes form an ORC lattice, a configuration similar to that of the commercially available molecular lattice model made up of balls and sticks. Note: Each square-well potential ![]() has three parameters
has three parameters![]() , and this model represents the true potential fairly well [9] . The Schrödinger equation for the 3D model Hamiltonian
, and this model represents the true potential fairly well [9] . The Schrödinger equation for the 3D model Hamiltonian
![]() (64)
(64)
can now be reduced to three 1D K-P equations. We can then write an expression for the energy of our model system near the lowest band edge as
![]() , (65)
, (65)
where ![]() are effective masses.
are effective masses.
Equation (65) is what is intuitively expected of the energy-k relation for the electron in the ORC lattice. It is stressed that we derived it from first principles, assuming a 3D model Hamiltonian ![]() in Equations (62) and (63).
in Equations (62) and (63).
5. Discussion
5.1. The Coulomb Interaction
The Coulomb potential ![]() between two charges
between two charges![]() , located at
, located at ![]() and
and ![]() in vacuum is given by
in vacuum is given by
![]()
Figure 3. A 2D model potential. Each singly shaded stripe has a potential energy (depth)![]() . Each cross-shaded square has a potential energy
. Each cross-shaded square has a potential energy![]() .
.
![]() , (66)
, (66)
where ![]() Coulomb’s constant,
Coulomb’s constant, ![]() vacuum permittivity. Special relativity requires that the photon travels with the light speed c and no mass carrier can travel faster than c. Hence the instantaneous pair potential in Equation (66) is not allowed in relativistic quantum theory. In quantum electrodynamics the Coulomb interaction is discussed by means of the (longitudinal) photon exchange between two electrons and the zero-mass photon travels with the light speed c. Dirac’s theorem [2] about particles as wave packets may be applied to this relativistic theory.
vacuum permittivity. Special relativity requires that the photon travels with the light speed c and no mass carrier can travel faster than c. Hence the instantaneous pair potential in Equation (66) is not allowed in relativistic quantum theory. In quantum electrodynamics the Coulomb interaction is discussed by means of the (longitudinal) photon exchange between two electrons and the zero-mass photon travels with the light speed c. Dirac’s theorem [2] about particles as wave packets may be applied to this relativistic theory.
In classical statistical mechanics, a one-body distribution function ![]() defined through
defined through
![]() . (67)
. (67)
is introduced. Integrating over the phase space we obtain
![]() . (68)
. (68)
An electron gas system is characterized by the Hamiltonian
![]() . (69)
. (69)
The evolution equation for f is
![]() , (70)
, (70)
where ![]() is a two-body distribution function, defined as an extension of Equation (67). This equation contains two unknown
is a two-body distribution function, defined as an extension of Equation (67). This equation contains two unknown ![]() and
and ![]() and hence cannot be solved as it stands.
and hence cannot be solved as it stands.
If the system Hamiltonian ![]() contains an interparticle interaction
contains an interparticle interaction
![]() (71)
(71)
then the evolution equation for the field operator ![]() is nonlinear:
is nonlinear:
![]() . (72)
. (72)
In quantum field theory the basic dynamical variables are particle-field operators![]() . The quantum statistics of the particles are given by the Bose commutation or the Fermi anticommutation rules satisfied by the field operators. The evolution equations of the field operators are intrinsically nonlinear when the interparticle interaction is present [10] .
. The quantum statistics of the particles are given by the Bose commutation or the Fermi anticommutation rules satisfied by the field operators. The evolution equations of the field operators are intrinsically nonlinear when the interparticle interaction is present [10] .
5.2. Phonons
Let us consider small oscillations for a system of atoms forming a SC lattice. Assume a longitudinal traveling wave along the x-axis. Imagine first hypothetical planes perpendicular to the x-axis containing atoms forming a square lattice. This plane has a mass per unit square of side length a (the lattice constant), equal to the atomic mass m. The plane is subjected to a restoring force per cm2 equal to Young modulus Y. The dynamics of a set of the parallel planes is similar to that of coupled harmonic oscillators.
Assume next a transverse wave traveling along the x-axis. The hypothetical planes containing many atoms are subjected to a restoring stress equal to the shear modulus S. The dynamics is also similar to the coupled harmonic oscillators in 1D.
Low-frequency phonons are those to which Debye’s continuum solid model [11] can be applied. The wave equations for the displacements ![]() are
are
![]() , (73)
, (73)
where ![]() (longitudinal) or t (transverse). The longitudinal-wave phase velocity
(longitudinal) or t (transverse). The longitudinal-wave phase velocity ![]() is
is
![]() , (74)
, (74)
where ![]() is the mass density. The transverse-wave phase velocity
is the mass density. The transverse-wave phase velocity ![]() is
is
![]() , (75)
, (75)
The waves are superposable. Hence, phonons’ travels are not restricted to the crystal’s cubic directions. In short, there is a 3D k-vector,![]() . The wave propagation is isotropic for each mode i.
. The wave propagation is isotropic for each mode i.
Consider now the case of an ORC crystal. We may choose Cartesian coordinates ![]() passing through the center of the unit cell. The small oscillations are similar to the case of a SC lattice. The dynamics of the parallel plates are the same but the restoring forces are different in x-, y- and z-directions. The plane waves have different phase velocities, depending on the directions. They are superposable since these waves are still solutions of the wave Equations (73).
passing through the center of the unit cell. The small oscillations are similar to the case of a SC lattice. The dynamics of the parallel plates are the same but the restoring forces are different in x-, y- and z-directions. The plane waves have different phase velocities, depending on the directions. They are superposable since these waves are still solutions of the wave Equations (73).
Phonons are quanta corresponding to the running plane-wave modes of lattice vibrations. Phonons are bosons, and the energies are distributed, following the Planck distribution function:
![]() . (76)
. (76)
There is no activation energy unlike the case of the “electrons”. This arises from the boson nature of phonons. The temperature T alone determines the average number and energy.
Phonons and conduction electrons are generated based on the same lattice and k-space. This is important when describing the electron-phonon interaction.
The “electrons” and “holes” have the same orthogonal unit cell size. The average phonon size is much greater than the electron size. The low-energy phonons have small k and great wavelengths. The average energy of a fermionic electron is greater than a bosonic phonon by two or more orders of magnitude. This establishes a usual physical picture that a point-like electron runs, and is occasionally scattered by a cloud-like phonon in the crystal.
We saw earlier that a MCL crystal has 1D k-vectors pointing along the c-axis for the electrons. There are similar 1D k-vectors for phonons. Besides, there are two other sets of 1D k-vectors. Plane waves running in the z-direction can be visualized by imagining the parallel plates, each containing a great number of atoms executing longitudinal and transverse small oscillations. Consider an oblique net of points (atoms) viewed from
![]()
Figure 4. An oblique net with base vectors![]() .
.
the top, shown in Figure 4. Planes defined by the vector ![]() and the c-axis are parallel and each plane contains a great number of atoms. Planes defined by the vector
and the c-axis are parallel and each plane contains a great number of atoms. Planes defined by the vector ![]() and the c-axis are also parallel, and each contains a number of atoms also executing small oscillations. These three sets of 1D phonons stabilize the lattice. The phonons run anisotropically since the restoring stresses and phase velocities are direction-dependent.
and the c-axis are also parallel, and each contains a number of atoms also executing small oscillations. These three sets of 1D phonons stabilize the lattice. The phonons run anisotropically since the restoring stresses and phase velocities are direction-dependent.
We next consider a TCL crystal, which has no k-vectors for the electrons. There are, however, a set of 1D k-vectors for phonons. Take a primitive TCL unit cell. The opposing faces are parallel to each other. There are restoring forces characterized by Young modulus Y and shear modulus S. Then, there are 1D k-vectors perpendicular to the faces. The set of 1D phonons can stabilize the lattice. These phonons in TCL are highly directional. There is no spherical wave formed.
We used the lattice property that the facing planes are parallel. This parallel-plane configuration is common to all seven crystal systems [1] [12] . A typical HEX system, graphite, clearly has three sets of parallel material planes containing many atoms. The RHL system has parallel planes, too. The parallel material planes configuration is the basic condition for the phonon generation and the lattice stability.
5.3. Metal-Insulater Transition
In 1959 Morin reported his discovery of a metal-insulator transition (MIT) in vanadium dioxide (VO2) [13] . Compound VO2 forms a monoclinic (MCL) crystal on the low temperature side and a tetragonal (TET) crystal on the high temperature side. When heated, VO2 undergoes an insulator-to-metal transition around 340 K, with the resistance drop by four orders of magnitude. The origin of the phase transition has been attributed to Peierls instability driven by strong electron-phonon interaction [14] , or to Coulomb repulsion and electron localization due to the electron-electron interaction on a Mott-Hubbard picture by other authors [15] - [17] .
A simpler view on the MIT we propose is as follows [18] . The existence of k-vectors is prerequisite for the electrical conduction. The TET (VO2)3 unit cells are periodic along the crystal’s x-, y-, and z-axes, and hence there are three-dimensional (3D) k-vectors. There are 1D k-vectors along the c-axis for a MCL crystal. The MIT occurs since the dimensionality of the k-vectors is reduced from three (3) to one (1) is going from the TET to the MCL crystals.
Whittaker et al. [19] measured the resistance R in K-doped V2O5 nanowires. They observed that (a) the resistance R for the low-temperature (MCL) phase shows an Arrhenius-type T-dependence:
![]() (77)
(77)
![]() activation energy
activation energy![]() ,
, ![]() Boltzmann constant, and that (b) the resistance R for the high- temperature (TET) phase is T-independent. These different behaviors may arise as follows: the currents in (a) run along the nanowire axis, which is also the easy c-axis of the MCL crystal. Hence the Arrhenius behavior (for the conductivity) is observed for the case (a). For the case (b) the currents run in 3D. Only those electrons near the Fermi surface are excited and participate in the transport. Then, the density of excited electrons,
Boltzmann constant, and that (b) the resistance R for the high- temperature (TET) phase is T-independent. These different behaviors may arise as follows: the currents in (a) run along the nanowire axis, which is also the easy c-axis of the MCL crystal. Hence the Arrhenius behavior (for the conductivity) is observed for the case (a). For the case (b) the currents run in 3D. Only those electrons near the Fermi surface are excited and participate in the transport. Then, the density of excited electrons, ![]() , is related to the total electron density
, is related to the total electron density ![]() by
by
![]() , (78)
, (78)
where ![]() is a number close to unity. The factor T drops out with the T-linear phonon scattering rate (for the conductivity). The T-dependence of the exponential factor is small since the activation energy
is a number close to unity. The factor T drops out with the T-linear phonon scattering rate (for the conductivity). The T-dependence of the exponential factor is small since the activation energy ![]() is much greater than the observation temperature around 10˚C. Thus, the resistance is nearly constant. There is a sudden drop of resistance around 300 K, where the two phases separate.
is much greater than the observation temperature around 10˚C. Thus, the resistance is nearly constant. There is a sudden drop of resistance around 300 K, where the two phases separate.
The MIT designation is a misnomer. The semiconductor-microconductor transition correctly describes the phenomenon since the transition is between the 3D-k semiconductor with an activation energy and the 1D-k semiconductor called here the microconductor. In the low temperature phase the resistance R decreases with the temperature T, indicating the semiconductor character. In the normal metal the resistance R increases with T, arising from the phonon population change. In the high temperature phase the resistance R is finite, and therefore the material is not insulator. There are sharp drop and rise in the resistance, and the phase change depends on the heating and cooling directions, arising from the domain-by-domain transitions.
5.4. Graphene
Graphene forms a 2D honeycomb lattice. The WS unit cell is a rhombus (darkened area) shown in Figure 5(a). We showed in our earlier work [18] that the graphene has “electrons” and “holes” based on the rectangular unit cell (dotted black-lines) shown in Figure 5(b). We briefly review our calculations.
The prevalent theory based on the WS rhombus unit cell model predicts a gapless semiconductor with an “electron”-“hole” symmetry. In our earlier work [18] we showed that (a) the “electron” and the “hole” have different charge distributions and different effective masses, (b) that the “electrons” and “holes” move in different easy channels, (c) that the “electrons” and “holes” are thermally excited with different activation energies, and (d) that the “electron” activation energy ![]() is smaller than the “hole” activation energy
is smaller than the “hole” activation energy![]() :
:
![]() . (79)
. (79)
Thus, “electrons” are the majority carriers in graphene. The thermally activated electron densities are then given by
![]() , (80)
, (80)
where ![]() and 2 represent the “electron” and “hole”, respectively. Magnetotransport experiments by Zhang et al. [20] indicate that the “electrons” are majority carriers in graphene. Thus, our theory is in agreement with experiments.
and 2 represent the “electron” and “hole”, respectively. Magnetotransport experiments by Zhang et al. [20] indicate that the “electrons” are majority carriers in graphene. Thus, our theory is in agreement with experiments.
5.5. Graphite
Graphite is composed of graphene layers stacked in the manner ABAB∙∙∙ along the c-axis. We may choose an orthogonal (Cartesian) unit cell shown in Figure 6. The unit cell contains 16 C’s. The two rectangles (white solid lines) are stacked vertically with the interlayer separation, ![]() Å much greater than the nearest neighbor distance between two C’s,
Å much greater than the nearest neighbor distance between two C’s, ![]() Å:
Å:
![]() . (81)
. (81)
The unit cell has three side-lengths:
![]() . (82)
. (82)
Clearly, the system is periodic along the orthogonal directions with the three periods ![]() given in
given in
![]()
Figure 5. (a) WS unit cell, rhombus (darkened area) for graphene; (b) The orthogonal unit cell, rectangle (dotted lines).
![]()
Figure 6. An orthogonal (Cartesian) unit cell (white solid lines) viewed from the top for graphite. The unit cell of graphite has two layers of graphene. The carbons (circles) in the A (B) planes are shown in black (gray).
Equation (82). Both “electron” and “hole” have the same unit cell size. The system is orthorhombic with the sides![]() ,
, ![]() ,
, ![]() ,
,![]() .
.
The negatively charged “electron” (with the charge![]() ) in graphite is welcomed by the positively charged C+ when moving vertically up or downwards on the paper. That is, the easy directions for the “electrons” are vertical. The easy directions for the “holes” are horizontal. There are no hindering hills for “holes” moving horizontally. Hence, the “electron” in graphite has the lower activation energy than the “hole”:
) in graphite is welcomed by the positively charged C+ when moving vertically up or downwards on the paper. That is, the easy directions for the “electrons” are vertical. The easy directions for the “holes” are horizontal. There are no hindering hills for “holes” moving horizontally. Hence, the “electron” in graphite has the lower activation energy than the “hole”:![]() . Then, “electrons” are the majority carriers in graphite. The thermoelectric power (Seebeck coefficient) measurements by Kang et al. [21] show that the majority carriers in graphite are “electrons”, in agreement with our theory.
. Then, “electrons” are the majority carriers in graphite. The thermoelectric power (Seebeck coefficient) measurements by Kang et al. [21] show that the majority carriers in graphite are “electrons”, in agreement with our theory.
It is sometimes said [22] that since the separation distance ![]() is much greater than the nearest neighbor distance
is much greater than the nearest neighbor distance![]() , the conduction in graphite is two-dimensional, and can be discussed in terms of the motion in the graphene as a first approximation. We take a different point of view. The conduction electrons move as wave packets having the 3D orthogonal unit cell sizes. The conduction is two-dimensional because of the inequality (81). But the transport behaviors in graphite and graphene are very different because of the different unit cells. A room temperature quantum Hall effect (QHE) was observed in graphene [23] [24] . Are there quantum Hall and superconductivity states for graphite, too? These are important questions.
, the conduction in graphite is two-dimensional, and can be discussed in terms of the motion in the graphene as a first approximation. We take a different point of view. The conduction electrons move as wave packets having the 3D orthogonal unit cell sizes. The conduction is two-dimensional because of the inequality (81). But the transport behaviors in graphite and graphene are very different because of the different unit cells. A room temperature quantum Hall effect (QHE) was observed in graphene [23] [24] . Are there quantum Hall and superconductivity states for graphite, too? These are important questions.
The construction of the orthogonal unit cell developed here can be followed in other materials forming HEX crystals: Zinc (Zn) and Beryllium (Be) form HEX crystals. The closed orbits on the coronet-like Fermi surface generate cyclotron resonance, which may be discussed using the orthogonal unit cells.
6. Summary
In summary, we established that
The conduction electron (“electron”, “hole”) in an ORC crystal moves when a primitive orthogonal unit cell is chosen as the quantum wave packet.
CUB, TET, ORC, RHL, HEX crystal systems have 3D k-spaces for electrons. The MCL system has a 1D k-space. The TCL has no k-vectors.
The MCL and TCL have 1D phonons, which are highly directional. No spherical phonon distributions are generated.
For RHL and HEX crystals the orthogonal unit cells different from the WS unit cells must be chosen for electron and phonon dynamics.
“Electrons” and “holes” have the same unit cell size, and they move with different effective masses. “Electrons” and “holes” in semiconductors are excited with different activation energies. Phonons are excited with no activation energies.
Both phonons and electrons are generated based on the same orthogonal unit cells. This fact is important when dealing with the electron-phonon interaction.
The electron size is the primitive unit cell size. The average phonon size is greater by two or more orders of magnitude at the room temperature.
Instantaneous interparticle Coulomb potential ![]() violates the special relativity principles. Dirac’s theorem [2] about interparticles (electrons) as wave packets is valid if the Coulomb interaction is described in relativistic quantum field theory, and it is regarded as a result of a longitudinal photon exchange between the electrons.
violates the special relativity principles. Dirac’s theorem [2] about interparticles (electrons) as wave packets is valid if the Coulomb interaction is described in relativistic quantum field theory, and it is regarded as a result of a longitudinal photon exchange between the electrons.
The MIT in VO2 is in reality a transition between two semiconductors having 1D and 3D k-vectors. The 1D semiconductor (low temperature phase) is highly anisotropic.
The majority carriers in graphene and graphite are “electrons”.
Graphite has “electrons” and “holes”. Phonon exchange may generate Cooper pairs. Then graphite can be a superconductor.