A New Application of the Flux Approximation Method on Hyperbolic Conservation Systems ()
1. Introduction
It is well known that no classical solution exists for the following initial value problem
 (1.1)
(1.1)
with bounded measurable initial data
 (1.2)
(1.2)
where  is the unknown vector function standing for the density of physical quantities and
is the unknown vector function standing for the density of physical quantities and  is a given vector function denoting the conservative term. These equations are commonly called conservation laws.
is a given vector function denoting the conservative term. These equations are commonly called conservation laws.
Since, in general, the discontinuity or the shock waves will appear in the solution to the Cauchy problem (1.1)- (1.2), there are two standard methods to obtain a weak solution or a generalized solution  for given hyperbolic conservation laws. One is to construct a sequence of smooth functions to approximate
for given hyperbolic conservation laws. One is to construct a sequence of smooth functions to approximate . For example, to add a small parabolic perturbation term to the right-hand side of (1.1):
. For example, to add a small parabolic perturbation term to the right-hand side of (1.1):
 (1.3)
(1.3)
where  is a constant. For each fixed
is a constant. For each fixed , we have a classical solution
, we have a classical solution  of (1.3)-(1.2), then we try to prove that the limit
of (1.3)-(1.2), then we try to prove that the limit  of
of  as
as  goes to zero is the solution of (1.1)-(1.2), where the compactness could be obtained by the compensated compactness arguments [1,2] when the functions have only the uniform boundedness in a suitable Banach space or the technique given in [3] when the functions are of total bounded variation estimates; another is the finite difference method [4]. We construct a sequence of simple functions by choosing a suitable difference scheme which is based on the given hyperbolic conservation laws and then prove the compactness of the sequence of functions. Normally, in the second method, we know that the sequence of simple functions is of total bounded variation estimates.
goes to zero is the solution of (1.1)-(1.2), where the compactness could be obtained by the compensated compactness arguments [1,2] when the functions have only the uniform boundedness in a suitable Banach space or the technique given in [3] when the functions are of total bounded variation estimates; another is the finite difference method [4]. We construct a sequence of simple functions by choosing a suitable difference scheme which is based on the given hyperbolic conservation laws and then prove the compactness of the sequence of functions. Normally, in the second method, we know that the sequence of simple functions is of total bounded variation estimates.
However, the third front tracking method [5], here we just call it the flux approximation method, is also used in many different cases.
In [6], Dafermos first introduced this method to the scalar conservation law
 (1.4)
(1.4)
where  is a scalar function, and
is a scalar function, and  is a locally Lipschitz continuous function. He constructed a sequence of piecewise linear functions
is a locally Lipschitz continuous function. He constructed a sequence of piecewise linear functions  and a sequence of step functions
and a sequence of step functions  to approximate
to approximate  and the initial date
and the initial date  respectively. Let the solutions of the following Cauchy problem be
respectively. Let the solutions of the following Cauchy problem be :
:
 (1.5)
(1.5)
with the initial data
 (1.6)
(1.6)
For each fixed , since the simplicity of the flux function
, since the simplicity of the flux function  and the initial date
and the initial date , the sequence of solutions
, the sequence of solutions  can be easily obtained first. Then by using the standard compactness argument by Oleinik, the convergence of
can be easily obtained first. Then by using the standard compactness argument by Oleinik, the convergence of  can be proved as
can be proved as  goes to zero.
goes to zero.
Later, the above idea was used to study the existence of Riemann solutions for some special systems of two equations. For example, in [7], the author first studied the Riemann solution for the Cauchy problem of the following system
 (1.7)
(1.7)
with initial data
 (1.8)
(1.8)
The more details about the Front Tracking method for systems of hyperbolic conservation laws can be found in the books [5,8] and the references cited therein.
In [9], Keyfitz introduced a different way to approximate the nonlinear flux function . Consider the Cauchy problem
. Consider the Cauchy problem
 (1.9)
(1.9)
with the Riemann initial data, where  since the system is hyperbolic or
since the system is hyperbolic or  as required in
as required in
[9]. For each fixed , System (1.9) is strictly hyperbolic and Riemann solution
, System (1.9) is strictly hyperbolic and Riemann solution  could be easily obtained. Then a Riemann solution of system (1.7) follows since it is the limit of
could be easily obtained. Then a Riemann solution of system (1.7) follows since it is the limit of  as
as  goes to zero.
goes to zero.
The method of flux approximation was applied by the first author of this paper to study the existence of weak solutions [10,11], the existence of global Lipschitz solutions [12],  compactness for weak entropy-entropy flux pairs of the isentropic gas dynamics [11],
compactness for weak entropy-entropy flux pairs of the isentropic gas dynamics [11],  estimate for isentropic gas dynamics with a superline source [13], the global
estimate for isentropic gas dynamics with a superline source [13], the global  solutions of Aw-Rascle traffic flow model [14] (or the nonsymmetric systems of Keyfitz-Kranzer type) with negative adiabatic exponent and so on, which we shall introduce below. A new application of this method related to the LeRoux system is introduced in Theorem 1, Section 2.
solutions of Aw-Rascle traffic flow model [14] (or the nonsymmetric systems of Keyfitz-Kranzer type) with negative adiabatic exponent and so on, which we shall introduce below. A new application of this method related to the LeRoux system is introduced in Theorem 1, Section 2.
2. A New Application of Flux Approximation Method
In this section, we introduce a new application of the flux approximation method. We found two hyperbolic conservation systems of Temple’s type [15], and the global weak solution of each system could be obtained by the limit of the linear combination of two systems.
Consider the hyperbolic systems
 (2.1)
(2.1)
and
 (2.2)
(2.2)
By simple calculations, two eigenvalues of system (2.1) are
 (2.3)
(2.3)
where , with corresponding right eigenvectors
, with corresponding right eigenvectors
 (2.4)
(2.4)
and
 (2.5)
(2.5)
The Riemann invariants of (2.1) are
 (2.6)
(2.6)
Thus, the curves  are straight lines on the
are straight lines on the  -plane.
-plane.
Similarly, two eigenvalues of system (2.2) are
 (2.7)
(2.7)
with the corresponding right eigenvectors (2.4) and
 (2.8)
(2.8)
The Riemann invariants of (2.2) are also given by (2.6)
Therefore if we consider the bounded solution in the region: , it follows from (2.5) (or (2.8)) that both characteristic fields of system (2.1) (or system (2.2)) are genuinely nonlinear in the sense of Lax [16].
, it follows from (2.5) (or (2.8)) that both characteristic fields of system (2.1) (or system (2.2)) are genuinely nonlinear in the sense of Lax [16].
Now we prove that both systems (2.1) and (2.2) have the same entropies.
Let . Then for smooth solutions, (2.2) is equivalent to the following system:
. Then for smooth solutions, (2.2) is equivalent to the following system:
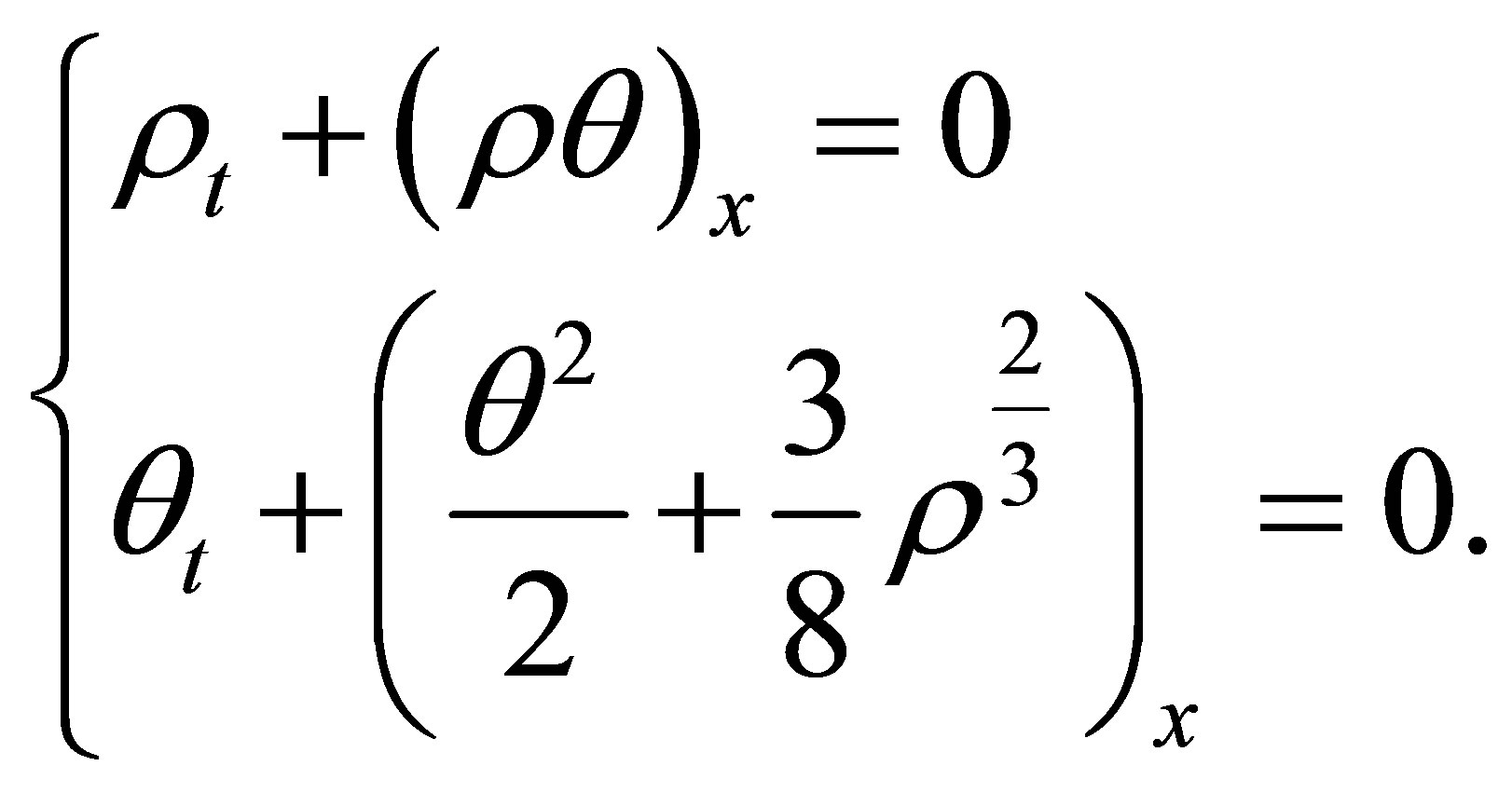 (2.9)
(2.9)
Considering the entropy-entropy flux pair  of system (2.2) as functions of variables
of system (2.2) as functions of variables , we have
, we have
 (2.10)
(2.10)
Eliminating the  from (2.10), we have
from (2.10), we have
 (2.11)
(2.11)
Similarly, for smooth solutions, (2.1) is equivalent to the following system:
 (2.12)
(2.12)
For the entropy-entropy flux pair  of system (2.1), we have
of system (2.1), we have
 (2.13)
(2.13)
Eliminating the  from (2.13), we have also the same entropy Equation (2.11).
from (2.13), we have also the same entropy Equation (2.11).
Using the compensated compactness arguments, we may easily obtain the global existence of weak solutions for the Cauchy problem of system (2.2) in the upper  -plane
-plane  or system (2.1) in the region
or system (2.1) in the region  for a suitable constant
for a suitable constant , which could be guaranteed since the curves
, which could be guaranteed since the curves  are straight lines, where
are straight lines, where  are four suitable constants. The details could be found in Chapter 7 of [17] or the original paper by Diperna [18].
are four suitable constants. The details could be found in Chapter 7 of [17] or the original paper by Diperna [18].
Now we consider the linear combination of systems (2.1) and (2.2):
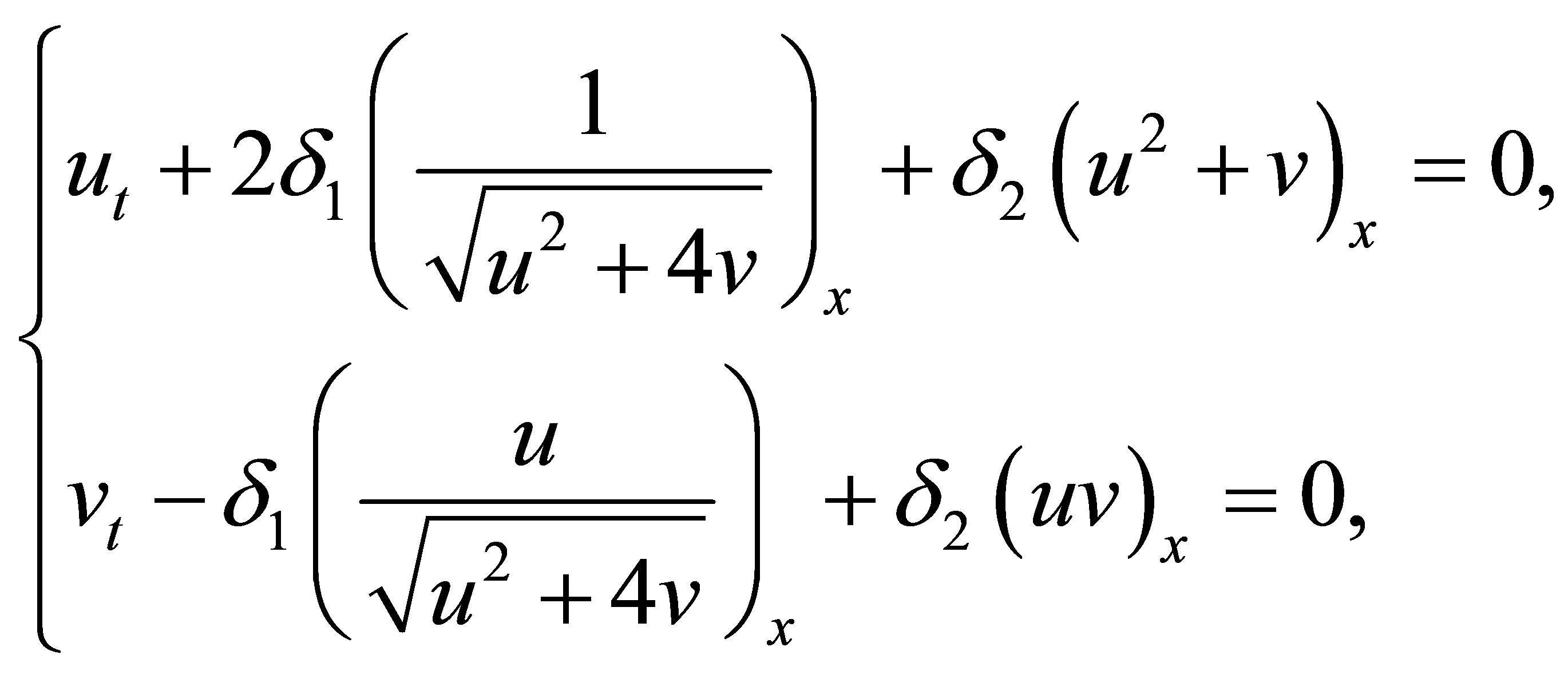 (2.14)
(2.14)
where  are two positive flux approximation perturbations.
are two positive flux approximation perturbations.
The eigenvalues of system (2.14) are solutions of the following characteristic equation:
 (2.15)
(2.15)
Two roots of Equation (2.15) are
 (2.16)
(2.16)
with the corresponding right eigenvectors (2.4) and the Riemann invariants (2.6). Moreover,
 (2.17)
(2.17)
Therefore both characteristic fields of system (2.14) are genuinely nonlinear in the region: .
.
Now we consider the Cauchy problem of system (2.14) with initial data
 (2.18)
(2.18)
and have the main results in the following theorem
Theorem 1. Suppose the initial data  be bounded measurable and
be bounded measurable and  for a suitable constant
for a suitable constant . Then for any fixed
. Then for any fixed , the global weak solution
, the global weak solution  of the Cauchy problem (2.14) and (2.18) exists. Moreover, for fixed
of the Cauchy problem (2.14) and (2.18) exists. Moreover, for fixed  (or
(or ), there exists a subsequence
), there exists a subsequence  (or
(or ) of
) of , which piontwisely converges, as
, which piontwisely converges, as  (or
(or ) goes to zero, to the solution of the Cauchy problem of system (2.1) (or (2.2)) with the initial data (2.18).
) goes to zero, to the solution of the Cauchy problem of system (2.1) (or (2.2)) with the initial data (2.18).
The proof of Theorem 1: The proof of Theorem 1 can be obtained by the standard vanishing artificial viscosity method coupled with the compensated compactness argument and the famous framework of DiPerna [18] for strictly hyperbolic, genuinely nonlinear systems of two equations. We add the viscosity terms to the right hand side of (2.14) and consider the following parabolic system
 (2.19)
(2.19)
with the initial data (2.18). According to the calculations given in (2.3) and (2.7), we know that the two eigenvalues of system (2.14) are
 (2.20)
(2.20)
with the corresponding right eigenvectors (2.4) and the Riemann invariants (2.6).
For any constant , the curves
, the curves  or
or  is a straight line on the
is a straight line on the  -plane, then we may choose suitable constants
-plane, then we may choose suitable constants  such that
such that  forms a bounded invariant region. Moreover, in this region,
forms a bounded invariant region. Moreover, in this region,  for a suitable constant
for a suitable constant . Since system (2.14) is strictly hyperbolic and genuinely nonlinear, and the viscosity solutions
. Since system (2.14) is strictly hyperbolic and genuinely nonlinear, and the viscosity solutions  of system (2.19) are uniformly bounded, then the famous compactness framework of DiPerna [18] gives us the convergence of
of system (2.19) are uniformly bounded, then the famous compactness framework of DiPerna [18] gives us the convergence of
 (2.21)
(2.21)
where the limit 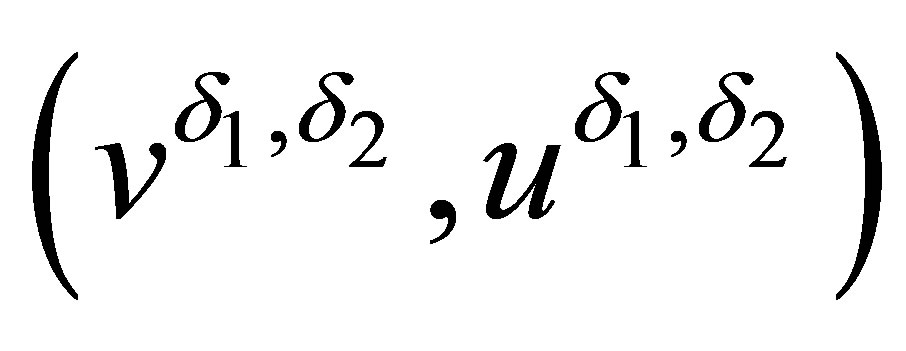 is a weak solution of system (2.14) or satisfies (2.14) in the sense of distributions. For fixed
is a weak solution of system (2.14) or satisfies (2.14) in the sense of distributions. For fixed  (or
(or ), and for the generalized functions
), and for the generalized functions , we may rewrite system (2.14) as
, we may rewrite system (2.14) as
 (2.22)
(2.22)
Since the left hand side of (2.22) or system (2.1) is also strictly hyperbolic and genuinely nonlinear, and the functions  are uniformly bounded, independent of
are uniformly bounded, independent of , so the DiPerna’s result [18] reduces the following convergence
, so the DiPerna’s result [18] reduces the following convergence
 (2.23)
(2.23)
where the limit  is a weak solution of system (2.1) or satisfies (2.1) in the sense of distributions, which ends the proof of Theorem 1.
is a weak solution of system (2.1) or satisfies (2.1) in the sense of distributions, which ends the proof of Theorem 1.
3. Acknowledgements
This work was partially supported by the Natural Science Foundation of Zhejiang Province of China (Grant No. LY12A01030 and Grant No. LZ13A010002) and the National Natural Science Foundation of China (Grant No. 11271105).
NOTES