Matrix Inequalities for the Fan Product and the Hadamard Product of Matrices ()
1. Introduction
Let , and
, and . We write
. We write 
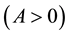 if
if 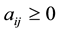
 for any
for any . If
. If , A is called a nonnegative matrix, and if A > 0, A is called a positive matrix. The spectral radius of a nonnegative matrix A is denoted by
, A is called a nonnegative matrix, and if A > 0, A is called a positive matrix. The spectral radius of a nonnegative matrix A is denoted by .
.
We denote by Zn the class of all n × n real matrices, all of whose off-diagonal entries are nonpositive. A matrix 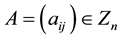 is called an M-matrix if there exists a nonnegative matrix B and a nonnegative real number s, such that
is called an M-matrix if there exists a nonnegative matrix B and a nonnegative real number s, such that 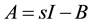 with
with , where I is the identity matrix. If
, where I is the identity matrix. If 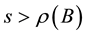 (resp.,
(resp., ), then the M-matrix A is nonsingular (resp., singular) (see [1] [2] ). Denote by Mn the set of nonsingular M-matrices. We define
), then the M-matrix A is nonsingular (resp., singular) (see [1] [2] ). Denote by Mn the set of nonsingular M-matrices. We define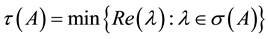 , where
, where 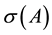 denotes the spectrum of A.
denotes the spectrum of A.
The Fan product of two matrices ![]() and
and ![]() is the matrix
is the matrix![]() , where
, where
![]()
If![]() , then so is
, then so is![]() . In ([2] , p. 359), a lower bound for
. In ([2] , p. 359), a lower bound for ![]() was given: if
was given: if![]() , then
, then![]() .
.
If![]() , and
, and![]() , we write
, we write![]() , where
, where![]() . Thus we define
. Thus we define![]() . Obviously, JA is nonnegative. Recently, some authors gave some lower bounds of
. Obviously, JA is nonnegative. Recently, some authors gave some lower bounds of ![]() (see [3] -[8] ). In [4] , Huang obtained the following result for
(see [3] -[8] ). In [4] , Huang obtained the following result for![]() ,
,
![]() (1)
(1)
The bound of (1) is better than the bound ![]() in ([2] , p. 359).
in ([2] , p. 359).
In [7] , Liu gave a lower bound of![]() ,
,
![]() (2)
(2)
where![]() . The bound of (2) is better than the one of (1).
. The bound of (2) is better than the one of (1).
For a nonnegative matrix![]() , let
, let![]() , where
, where![]() . We denote
. We denote![]() , where
, where![]() ,
,
![]()
The Hadamard product of two matrices ![]() and
and ![]() is the matrix
is the matrix ![]() . For two nonnegative matrices A and B, recently, some authors gave several new upper bounds of
. For two nonnegative matrices A and B, recently, some authors gave several new upper bounds of ![]() (see [3] -[7] [9] ). In [4] , Huang obtained the following result for
(see [3] -[7] [9] ). In [4] , Huang obtained the following result for![]() ,
,
1) If![]() , then
, then
![]() (3)
(3)
2) If ![]() or
or ![]() for some i0, but
for some i0, but![]() , then
, then
![]() (4)
(4)
3) If ![]() and
and![]() , then
, then
![]() (5)
(5)
4) If ![]() and
and ![]() for some i0, j0, then the upper bound of
for some i0, j0, then the upper bound of ![]() is the maximum value of the upper bounds of the inequalities in (3)-(5).
is the maximum value of the upper bounds of the inequalities in (3)-(5).
The bound of ![]() in [4] is better than that in ([2] , p. 358).
in [4] is better than that in ([2] , p. 358).
In [7] , Liu gave a new upper bound of![]() ,
,
1) If![]() , then
, then
![]() (6)
(6)
where![]() .
.
2) If ![]() and
and ![]() or
or ![]() and
and ![]() for some
for some![]() , but
, but![]() , then
, then
![]() (7)
(7)
3) If ![]() and
and![]() , then
, then
![]() (8)
(8)
4) If ![]() and
and ![]() for some i0, j0, then the upper bound of
for some i0, j0, then the upper bound of ![]() is the maximum value of the upper bounds of the inequalities in (6)-(8).
is the maximum value of the upper bounds of the inequalities in (6)-(8).
The bound of ![]() in [7] is better than that in [4] .
in [7] is better than that in [4] .
The paper is organized as follows. In Section 2, we give a new lower bound of![]() . In Section 3, we present a new upper bound of
. In Section 3, we present a new upper bound of![]() .
.
2. Inequalities for the Fan Product of Two M-Matrices
In this section, we will give a new lower bound of![]() .
.
If ![]() and
and![]() , we write
, we write ![]() for the k-th Hadamard power of A. If
for the k-th Hadamard power of A. If ![]() and
and![]() , we write
, we write![]() .
.
Lemma 1. [7] Let![]() , and let
, and let ![]() be two positive diagonal matrices. Then
be two positive diagonal matrices. Then
![]()
Lemma 2. [2] If ![]() is a nonnegative matrix and
is a nonnegative matrix and![]() , then
, then
![]()
Theorem 1. Let ![]() and
and![]() . Then
. Then
![]()
where![]() .
.
It is evident that the Theorem holds with equality for n = 1. Next, we assume that![]() .
.
(1) First, we assume that ![]() is irreducible matrix, then A and B are irreducible. Obviously JA and JB are also irreducible and nonnegative, so
is irreducible matrix, then A and B are irreducible. Obviously JA and JB are also irreducible and nonnegative, so ![]() and
and ![]() are nonnegative irreducible matrices. Then there exist two
are nonnegative irreducible matrices. Then there exist two
positive vectors ![]() and
and ![]() such that
such that ![]() and
and![]() . Let
. Let
![]()
Then we have ![]() and
and![]() , that is
, that is
![]()
Let ![]() and
and ![]() in which U and V are the nonsingular diagonal matrices
in which U and V are the nonsingular diagonal matrices
![]() and
and![]() . Then, we have
. Then, we have
![]()
![]()
It is easy to see that![]() ,
, ![]() , and VU are nonsingular since V and U are. From Lemma 1, we have
, and VU are nonsingular since V and U are. From Lemma 1, we have
![]()
Thus, we obtain![]() , and
, and
![]()
We next consider the minimum eigenvalue ![]() of
of![]() . Let
. Let![]() . Then we have that
. Then we have that![]() . By Theorem 1.23 of [10] , there exist
. By Theorem 1.23 of [10] , there exist![]() ,
, ![]() , such that
, such that
![]()
By Hölder’s inequality, we have
![]()
Then, we have
![]()
Since![]() , then
, then
![]()
Hence,
![]()
i.e.,
![]()
(2) Now, assume that ![]() is reducible. It is well known that a matrix in Zn is a nonsingular M-matrix if and only if all its leading principal minors are positive (see [11] ). If we denote by
is reducible. It is well known that a matrix in Zn is a nonsingular M-matrix if and only if all its leading principal minors are positive (see [11] ). If we denote by ![]() the n × n permutation matrix with
the n × n permutation matrix with![]() , the remaining tij zero, then both
, the remaining tij zero, then both ![]() and
and ![]() are irreducible nonsingular M-matrix for any chosen positive real number
are irreducible nonsingular M-matrix for any chosen positive real number![]() , sufficiently small such that all the leading principal minors of both
, sufficiently small such that all the leading principal minors of both ![]() and
and ![]() are positive. Now, we substitute
are positive. Now, we substitute ![]() and
and ![]() for A and B, respectively, in the previous case, and then letting
for A and B, respectively, in the previous case, and then letting![]() , the result follows by continuity.
, the result follows by continuity.
Remark 1. By Lemma 2, the bound in Theorem 1 is better than that in Theorem 4 of [8] and Theorem 2 of [7] .
Example 1. Let
![]()
By calculating with Matlab 7.1, it is easy to show that![]() .
.
Applying Theorem 4 of [4] , Theorem 3.1 of [5] , Theorem 2 of [7] , and Theorem 3.1 of [8] , we have![]() ,
, ![]() ,
, ![]() , and
, and![]() , respectively. But, if we apply Theorem 1, we have
, respectively. But, if we apply Theorem 1, we have
![]()
The numerical example shows that the bound in Theorem 1 is better than that in Theorem 4 of [4] , Theorem 3.1 of [5] , Theorem 2 of [7] , and Theorem 3.1 of [8] .
3. Inequalities for the Hadamard Product of Two Nonnegative Matrices
In this section, we will give a new upper bound of ![]() for nonnegative matrices A and B. Similar to [7] , for
for nonnegative matrices A and B. Similar to [7] , for![]() , write Q = A − D, where
, write Q = A − D, where![]() . We denote
. We denote ![]() with
with![]() , where
, where
![]()
Note that ![]() is nonnegative, and
is nonnegative, and ![]() if
if![]() ,
,![]() . For
. For![]() , let
, let![]() , where
, where
![]()
Similarly, the nonnegative matrix ![]() is defined.
is defined.
Lemma 3. [2] Let![]() , and let
, and let ![]() be diagonal matrices. Then
be diagonal matrices. Then
![]()
Lemma 4. [12] Let ![]() be a nonnegative matrix. Then
be a nonnegative matrix. Then
![]()
Theorem 2. Let![]() ,
, ![]() and
and![]() . Then
. Then
1) If![]() , then
, then
![]() (9)
(9)
where![]() .
.
2) If ![]() and
and ![]() or
or ![]() and
and ![]() for some
for some![]() , but
, but![]() , then
, then
![]() (10)
(10)
3) If ![]() and
and![]() , then
, then
![]() (11)
(11)
4) If ![]() and
and ![]() for some i0, j0, then the upper bound of
for some i0, j0, then the upper bound of ![]() is the maximum value of the upper bounds of the inequalities in (9)-(11).
is the maximum value of the upper bounds of the inequalities in (9)-(11).
Proof. It is evident that 4) holds with equality for n = 1. Next, we assume that![]() .
.
(1) First, we assume that ![]() is irreducible matrix, then A and B are irreducible. Obviously
is irreducible matrix, then A and B are irreducible. Obviously ![]() and
and ![]() are also irreducible and nonnegative, so
are also irreducible and nonnegative, so ![]() and
and ![]() are nonnegative irreducible matrices. Then there exist two positive vectors
are nonnegative irreducible matrices. Then there exist two positive vectors ![]() and
and ![]() such that
such that ![]() and
and![]() . Let
. Let
![]()
Then we have ![]() and
and![]() , that is
, that is
![]()
Let ![]() and
and ![]() in which U and V are the nonsingular diagonal matrices
in which U and V are the nonsingular diagonal matrices
![]() and
and![]() . Then we have
. Then we have
![]()
![]()
It is easy to see that![]() ,
, ![]() , and VU are nonsingular since V and U are. From Lemma 4, we have
, and VU are nonsingular since V and U are. From Lemma 4, we have
![]()
Thus, we obtain![]() , and
, and
![]()
We next consider the minimum eigenvalue ![]() of
of![]() . For nonnegative irreducible matrices
. For nonnegative irreducible matrices ![]()
and![]() , by definition of the Hadamard product of
, by definition of the Hadamard product of ![]() and
and![]() , Hölder’s inequality, and Lemma 5, we have
, Hölder’s inequality, and Lemma 5, we have
![]()
Thus, we obtain
1) If![]() , then
, then
![]()
2) If ![]() and
and ![]() or
or ![]() and
and ![]() for some i0, j0, but
for some i0, j0, but![]() , then
, then
![]()
3) If ![]() and
and![]() , then
, then
![]()
4) If ![]() and
and ![]() for some i0, j0, then the upper bound of
for some i0, j0, then the upper bound of ![]() is the maximum value of the upper bounds of the inequalities in (9)-(11).
is the maximum value of the upper bounds of the inequalities in (9)-(11).
(2) Now, we assume that ![]() is reducible. If we denote by
is reducible. If we denote by ![]() the n × n permutation matrix with
the n × n permutation matrix with![]() , the remaining tij = 0, then both
, the remaining tij = 0, then both ![]() and
and ![]() are irreducible nonsingular matrices for any chosen positive real number
are irreducible nonsingular matrices for any chosen positive real number![]() . Now, we substitute
. Now, we substitute ![]() and
and ![]() for A and B, respectively, in the previous case, and then letting
for A and B, respectively, in the previous case, and then letting![]() , the result follows by continuity.
, the result follows by continuity.
Remark 2. By Lemma 2, the bound in Theorem 2 is better than that in Theorem 6 of [6] and Theorem 3 of [9] .
Example 2. Let
![]()
By calculation with Matlab 7.1, we have![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
If we apply Theorem 6 of [4] , Theorem 3 of [7] , and Theorem 2.2 of [9] , we have![]() ,
, ![]() , and
, and![]() , respectively. But, if we apply Theorem 2, we have
, respectively. But, if we apply Theorem 2, we have
![]()
The numerical example shows that the bound in Theorem 2 is better than that in Theorem 6 of [4] , Theorem 3 of [7] , and Theorem 2.2 of [9] .