1. Introduction
Interest rate derivatives are contracts whose value depends in some way on the level of interest rates. Swap contracts have existed since the early 1980s and since then there has been significant growth in terms of volume and diversity of contracts. In general an interest rate swap is an agreement between two companies, whereupon one company agrees to pay cash flows equal to the interest on a predetermined fixed rate on a notional principal, X, at regular set times  (e.g. every 6 months) over the length of the contract time and in return receives interest at a floating rate (usually the LIBOR rate) on the same notional amount on the same set payment periods within the contract time. In the “plain vanilla” interest rate swap, the floating rate is the rate that prevails at the previous payment date, or in the case of the first payment, the rate at the opening of the contract. With LIBOR- in-arrears swaps, the floating rate paid on a payment date equals the rate observed on the payment date itself. Hence the floating leg cannot be valued as the sum of forward LIBORs.
(e.g. every 6 months) over the length of the contract time and in return receives interest at a floating rate (usually the LIBOR rate) on the same notional amount on the same set payment periods within the contract time. In the “plain vanilla” interest rate swap, the floating rate is the rate that prevails at the previous payment date, or in the case of the first payment, the rate at the opening of the contract. With LIBOR- in-arrears swaps, the floating rate paid on a payment date equals the rate observed on the payment date itself. Hence the floating leg cannot be valued as the sum of forward LIBORs.
Caplets and floorlets are the interest rate counterparts of European call and put options. Similar to vanilla swaps, the payoff of vanilla caplets and floorlets is based on the interest rate at the previous payment date whereas the payoff of in-arrears caplets and floorlets is based on the LIBOR rates at the actual time of the payment. Hence in-arrears derivatives are not as straightforward to price as their vanilla counterparts.
As stated by Chen and Sandmann [1] , typically when no assumptions are made about the term structure of interest rates, it is not possible to price these in-arrears products; and even when term structures are assumed, it is often not possible to find closed form solutions for the products. For this reason convexity adjustments (or convexity corrections) are often used by practitioners as a rule of thumb in the valuation of in-arrears term structure products. In [2] , Mallier and Alobaidi assume that risk-neutral interest rates follow the Cox-Ingersoll-Ross (CIR) model of the form
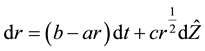
where a, b and c are constants and 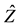 is a Wiener process under a risk-neutral probability measure. By using a Green’s function approach they manage to derive an analytical expression for in-arrears swaps.
is a Wiener process under a risk-neutral probability measure. By using a Green’s function approach they manage to derive an analytical expression for in-arrears swaps.
It has been shown (see e.g. [3] ) that when the short-term interest rate, r, follows a stochastic differential equation of the form

where a, b, γ and c are constants and dZ is an increment in a Wiener process under a real probability measure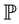 ; the value of γ is very important in differentiating between the different models’ abilities to adequately capture the dynamics of interest rates. In particular the unconstrained estimate of γ by Chan et al. [3] was 1.5. This was agreed upon by Campbell et al. [4] who showed that the heteroskedasticity of the short rate was markedly reduced as γ increased from 1 to 1.5. In [5] , Ahn and Gao showed that the interest rate model
; the value of γ is very important in differentiating between the different models’ abilities to adequately capture the dynamics of interest rates. In particular the unconstrained estimate of γ by Chan et al. [3] was 1.5. This was agreed upon by Campbell et al. [4] who showed that the heteroskedasticity of the short rate was markedly reduced as γ increased from 1 to 1.5. In [5] , Ahn and Gao showed that the interest rate model
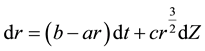 (1)
(1)
outperformed many of the popular interest rate models including the Vasicek and CIR models. The nonlinear drift in (1) implies a substantial nonlinear mean-reverting behaviour when the interest rate is above its long-run mean. Hence after a large interest rate rise, the interest rate can potentially quickly decrease, while after a low interest rate period, it can be slow to increase. It has also been shown that with a > 0, r will always remain positive. This model was further improved (see e.g. [6] [7] )
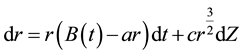 (2)
(2)
to include a time-dependent long-run target allowing yield-curves to be fitted.
In [6] , a solution was found for the price of bonds with maturity T, under the assumption that the risk-neutral process for r has a similar form to (2), namely
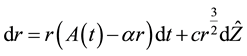 (3)
(3)
where 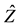 is a Wiener process under an equivalent risk-neutral measure
is a Wiener process under an equivalent risk-neutral measure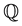 . The solution given is
. The solution given is
 (4a)
(4a)
where
 (4b)
(4b)
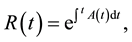 (4c)
(4c)
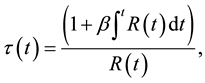 (4d)
(4d)
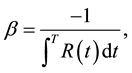 (4e)
(4e)
where ![]() is the Kummer-M function (see e.g. [8] ). This was found by solving the governing partial differential Equation (PDE) for
is the Kummer-M function (see e.g. [8] ). This was found by solving the governing partial differential Equation (PDE) for ![]() namely
namely
![]() (5)
(5)
subject to the final condition ![]() and boundary conditions
and boundary conditions![]() ,
,![]() .
.
In Section 3 of this paper, we extend the results in [6] by finding an exact solution for in-arrears swaps under the assumption that risk-neutral interest rates follow the time-dependent 3/2 model (3). As is typical with swap pricing, we divide the swap into a series of forward rate agreements (FRAs) and price each of these individually. The value of the swap is the sum of the individual FRAs. This was also the approach of Mallier and Alobaidi [2] . Then in Section 4 we derive analytic approximations for caplets and floorlets based on the time-dependent interest rate model (3) and compare their values with those obtained using an accurate (but computationally intensive) numerical scheme. Firstly however, we briefly summarise Lie’s classical symmetries method which is used to solve the PDEs in this paper.
2. Lie’s Classical Symmetries Method
In essence, the classical method for finding symmetry reductions of a second-order PDE in one dependent variable V and two independent variables (r, t)
![]() (6)
(6)
is to find a one-parameter Lie group of transformations in infinitesimal form
![]() (7a)
(7a)
![]() (7b)
(7b)
![]() (7c)
(7c)
which leaves (6) invariant. The coefficients ![]() and
and ![]() of the infinitesimal symmetry are often referred to as the “infinitesimals”. This invariance requirement is determined by
of the infinitesimal symmetry are often referred to as the “infinitesimals”. This invariance requirement is determined by
![]() (8)
(8)
where
![]() (9)
(9)
are vector fields that span the associated Lie algebra, and are called the infinitesimal generators of the transformation (7a-c), and ![]() is the second extension (or second prolongation) of
is the second extension (or second prolongation) of![]() , extended to the second jet space, co-ordinatised by
, extended to the second jet space, co-ordinatised by ![]() (see Chapter 2 in the book of Bluman and Kumei [9] ).
(see Chapter 2 in the book of Bluman and Kumei [9] ).
Then for known functions![]() , invariant solutions V corresponding to (7a-c) satisfy the invariant surface condition (ISC)
, invariant solutions V corresponding to (7a-c) satisfy the invariant surface condition (ISC)
![]() (10)
(10)
which when solved as a first-order PDE by the method of characteristics, yields the functional form of the similarity solution in terms of an arbitrary function, i.e.
![]()
where
![]()
and where ![]() is an arbitrary function of invariant z for the symmetry. Substituting this functional form into (6) produces an ordinary differential Equation which one solves for the function
is an arbitrary function of invariant z for the symmetry. Substituting this functional form into (6) produces an ordinary differential Equation which one solves for the function![]() .
.
Further, for a final-value problem with the final condition ![]() then we need a linear combination of generators such that condition (10) is satisfied at
then we need a linear combination of generators such that condition (10) is satisfied at![]() ,
, ![]() i.e.
i.e.
![]() (11)
(11)
![]() can be found from the final condition. As well, for evolution equations
can be found from the final condition. As well, for evolution equations ![]() can be found from the governing PDE (see [10] for details).
can be found from the governing PDE (see [10] for details).
3. LIBOR-in-Arrears Swaps
In this section we derive the analytic solution for the price of in-arrears swaps based on the risk-neutral interest rate model (3). The value given here is from the perspective of the receiver i.e. the investor who receives the fixed rate ![]() and pays the floating rate. As in [11] , we assume that the actual floating rate is the spot rate
and pays the floating rate. As in [11] , we assume that the actual floating rate is the spot rate![]() . The value to the payer i.e. the investor who pays the fixed and receives the floating, is simply the negative of the value given here.
. The value to the payer i.e. the investor who pays the fixed and receives the floating, is simply the negative of the value given here.
Theorem 1. The value of an in-arrears swap with notional value 1 and fixed rate r0 to a receiver with payment times Ti every half year, when the interest rate follows the risk-neutral process (3) is given by
![]() (12)
(12)
![]() (13)
(13)
and ![]() are given in (4b-e).
are given in (4b-e).
Proof. The value of an FRA to the investor who receives the fixed rate ![]() at
at ![]() satisfies (5) subject to
satisfies (5) subject to
![]() ,
, ![]() ,
,![]() . As (5) is linear and homogeneous, and the solution to the
. As (5) is linear and homogeneous, and the solution to the
bond under (3) is already known (given by Equation (4a-e)), we need only find the solution to (5) subject to![]() ,
, ![]() ,
,![]() . We denote this solution as
. We denote this solution as![]() .
.
With the help of the package Dimsym [12] , we find that PDE (5) has the symmetry with generator
![]() (14)
(14)
where
![]()
![]()
and where ![]() are arbitrary constants and
are arbitrary constants and![]() .
.
In order for the final condition to satisfy (11) and the boundary conditions to be invariant we choose
![]()
noting that![]() . Solving the ISC (10) corresponding to (14), then upon simplification, the functional form of the solution can be written as
. Solving the ISC (10) corresponding to (14), then upon simplification, the functional form of the solution can be written as
![]() (15)
(15)
Substitution of this functional form into (5) gives that ![]() needs to satisfy
needs to satisfy
![]() (16)
(16)
subject to![]() , and
, and ![]() and where
and where ![]()
Solving (16) for ![]() we get
we get
![]()
where![]() , and A and B are constants. Taking into consideration the boundary conditions we take B = 0 and
, and A and B are constants. Taking into consideration the boundary conditions we take B = 0 and![]() .
.
Undoing the change of variables we get the solution to ![]() as given in (13).
as given in (13).
4. Asymptotic Solution for Caplets
Again assuming equidistant payment times![]() , in a vanilla cap the contract holder receives
, in a vanilla cap the contract holder receives ![]() at time
at time![]() ,
, ![]() where
where ![]() X is the notional amount,
X is the notional amount, ![]() is the LIBOR rate at time
is the LIBOR rate at time ![]() and
and ![]() is the fixed cap rate. In a vanilla floor with fixed floor rate
is the fixed cap rate. In a vanilla floor with fixed floor rate ![]() the payment size is
the payment size is ![]() at
at![]() ,
, ![]() with the first payment time at
with the first payment time at ![]() and last one at
and last one at![]() . Hence the payment size at time
. Hence the payment size at time ![]() is based on the LIBOR rate at time
is based on the LIBOR rate at time![]() . In contrast with in-arrears caps and floors the contract holder receives
. In contrast with in-arrears caps and floors the contract holder receives ![]() and
and ![]() respectively at times
respectively at times![]() ,
, ![]() so that the payment size at time
so that the payment size at time ![]() is actually based on the LIBOR rate at time
is actually based on the LIBOR rate at time ![]() Each of the individual cashflows in a cap are called caplets and the individual cashflows in a floor are called floorlets. Hence caps and floors are sums of the individual caplets and floorlets respectively. Further, the value of a floor can be found from the cap-floor parity, namely “floor = cap − swap” (see e.g. [11] ). The most common valuation of interest rate caplets is via the Black-76 model [13] . Under this model the underlying interest rate is assumed to follow a log-normal distribution, which is not in agreement with empirical findings.
Each of the individual cashflows in a cap are called caplets and the individual cashflows in a floor are called floorlets. Hence caps and floors are sums of the individual caplets and floorlets respectively. Further, the value of a floor can be found from the cap-floor parity, namely “floor = cap − swap” (see e.g. [11] ). The most common valuation of interest rate caplets is via the Black-76 model [13] . Under this model the underlying interest rate is assumed to follow a log-normal distribution, which is not in agreement with empirical findings.
In this section we look at approximating the value of caplets and floorlets for short times to expiry, based on the risk-neutral interest rate model (3). We note that caplets and floorlets characteristically have short tenor, especially when the associated caps and floors have maturities of about one year. For simplicity we let ![]() but the solutions derived can simply be multiplied by
but the solutions derived can simply be multiplied by ![]() We start with the caplet.
We start with the caplet.
With![]() , from Equation (5) the value of an in-arrears caplet
, from Equation (5) the value of an in-arrears caplet ![]() with fixed cap rate K and expiry T satisfies
with fixed cap rate K and expiry T satisfies
![]() (17)
(17)
subject to ![]() where
where![]() . To find an approximation to (17) for small
. To find an approximation to (17) for small ![]() we follow the method outlined by Howison [14] . We let
we follow the method outlined by Howison [14] . We let ![]() where
where ![]() and assume the solution can be expanded as a series
and assume the solution can be expanded as a series
![]() (18)
(18)
Substituting (18) into (17) we get
![]() (19)
(19)
Upon equating coefficients of ![]() and
and![]() , and with consideration of corresponding boundary and initial con- ditions, we get that
, and with consideration of corresponding boundary and initial con- ditions, we get that
![]() (20)
(20)
where![]() . However, the above solution is not differentiable at
. However, the above solution is not differentiable at ![]() and as we expect large Gamma
and as we expect large Gamma
i..e ![]() near
near![]() , this “outer” solution is not valid in the vicinity of
, this “outer” solution is not valid in the vicinity of![]() . For the “inner” solution where
. For the “inner” solution where
r is near K, the second-order derivative with respect to r needs to be included in the differential system. We in-
troduce the inner variable ![]() and rescale V to
and rescale V to![]() .
.
This leads to the equation
![]()
i.e
![]() (21)
(21)
where![]() ,
, ![]() , to be solved subject to
, to be solved subject to![]() ,
, ![]() ,
,
![]() .
.
We now expand
![]() (22)
(22)
and substitute this form into (21). Equating terms of ![]() we get
we get
![]() (23)
(23)
subject to![]() ,
, ![]() ,
,![]() .
.
PDE (23) admits a six-dimensional finite Lie group of transformations (see e.g. [9] ). With consideration of the
initial and boundary conditions, we use the symmetry with generator![]() . This leads to
. This leads to
an invariant solution of the form ![]() where
where![]() . Substitution of this invariant form into (23) yields the reduced equation
. Substitution of this invariant form into (23) yields the reduced equation
![]() (24)
(24)
![]()
1We use the results [14] that (i) if ![]() and
and ![]() then a particular solution is
then a particular solution is ![]() and (ii) if
and (ii) if ![]() and
and ![]() then a particular solution is
then a particular solution is ![]()
which needs to be solved subject to![]() ,
, ![]()
Hence we get that ![]() and so
and so
![]() (25)
(25)
Now collecting terms of ![]() we get that
we get that ![]() satisfies
satisfies
![]() (26)
(26)
subject to![]() ,
, ![]() ,
,![]() . The solution to this problem is1
. The solution to this problem is1
![]() (27)
(27)
The two-term inner expansion can then be found by![]() .
.
We then match the inner and outer solutions to get a solution that is uniformly valid by calculating “outer + inner − common” where “common” is that part of the solution that is common to both. In this case as ![]() the inner solution is the same as the outer solution and so the outer expansion is in fact the common expansion. This means that the inner expansion is uniformly valid. In terms of the original variables our approximate solution for a caplet with fixed cap rate K and short time to expiry T based on the risk-neutral interest rate (3) is then
the inner solution is the same as the outer solution and so the outer expansion is in fact the common expansion. This means that the inner expansion is uniformly valid. In terms of the original variables our approximate solution for a caplet with fixed cap rate K and short time to expiry T based on the risk-neutral interest rate (3) is then
![]() (28)
(28)
In a similar way we get an approximate solution for the value of a floorlet with expiry T and fixed floor rate K as
![]() (29)
(29)
To test our approximate solutions we numerically solve PDE (17) using the mathematics software package MAPLE [15] (which uses a centered implicit finite-difference scheme) with step sizes of 10−4 and use this as a proxy for the true solution. We note that obtaining such numerical values is very labour-intensive and computationally-intensive whereas our approximate values are fast and easy-to-implement. Firstly, to test the accuracy of the finite-difference scheme, we used the method to numerically solve for in-arrears swap values i.e. Equation (5) subject to the conditions for the swap as given in Section 3, and compared these values to the exact solution (12). Using the parameter values![]() ,
, ![]() ,
, ![]() and with
and with ![]() we found that for r values from 0.045 to 0.065, with
we found that for r values from 0.045 to 0.065, with![]() , absolute errors were of the order of 10−10; for
, absolute errors were of the order of 10−10; for ![]() absolute errors were of the order of 10−6 and for
absolute errors were of the order of 10−6 and for ![]() absolute errors did not exceed 10−5.
absolute errors did not exceed 10−5.
Using the same parameter values as our testing procedure, i.e.![]() ,
, ![]() ,
, ![]() and with c = 1,
and with c = 1,
we computed signed percentage errors for caplet values i.e.![]() , using the approximate solution
, using the approximate solution
(28) and the “parity” value i.e “floorlet + swap” value using the exact value of the swap given by Equation (12) multiplied by −2, and the approximate floorlet value given in Equation (29). The results are listed in Table 1. Similarly in Table 2 are the percentage errors of the approximate floorlet solutions given by (29) and the parity values found from “caplet-swap”.
From Table 1 and Table 2 it can be seen that as expected, in general, the shorter times to expiry yield the more accurate results. In particular we note
Equation (28) yields values for caplets that are at-the-money (ATM) or in-the-money (ITM) that are above our exact values, with relative errors <1.1% for![]() , <2.15% for
, <2.15% for ![]() and <3.2% for
and <3.2% for![]() .
.
For caplets that are ATM or ITM, the parity formula yields the more accurate results where the value is dominated by the exact swap value. ATM options are slightly overpriced and mostly ITM options are underpriced slightly.
For the values of ![]() where caplets are out-of-the money (OTM), the floorlet values have small percentage errors but these errors are large in comparison to the corresponding caplet values thus producing very large parity percentage errors.
where caplets are out-of-the money (OTM), the floorlet values have small percentage errors but these errors are large in comparison to the corresponding caplet values thus producing very large parity percentage errors.
Equation (29) yields values for ATM and ITM floorlets with relative errors <0.65% for![]() , <1.3% for
, <1.3% for ![]() and <2% for
and <2% for![]() .
.
For floorlets that are ITM, the parity formula yields the more accurate results where the value is dominated by the exact swap value.
For the values of r where floorlets are out-of-the money (OTM), the caplet values have small percentage errors but these are large in comparison to corresponding floorlet values producing very large parity percentage errors.
The results suggest that parity values would be best to price ATM and ITM caplets and ITM floorlets; their values mostly slightly underpricing compared to the exact solution, while Equation (28) could be used to price OTM caplets and Equation (29) used to price ATM and OTM floorlets; their values producing small percentage errors especially (and surprisingly) for the larger values of ![]() and
and![]() .
.
It should also be noted that similar percentage error results were found using other volatility coefficient values c.
5. Discussion
The form of an interest rate model is crucial in the subsequent modelling of interest rate products and the accuracy of their valuations. It has been shown empirically by a number of authors that the 3/2 model (3) outperforms many of the popular interest rate models, such as the Vasicek and CIR models, in its ability to capture the actual behaviour of the interest rate. Including a free function of time in the drift further enhances the model’s ability to capture the interest rate dynamics. In this paper we have assumed the risk-neutral interest rate model (3) and extended the results in [6] by finding an exact solution for the value of in-arrears swaps and approximate values for caplets and floorlets with short times to expiry. As noted previously, caplets and floorlets have characteristically short tenors especially when the maturity of the cap/floor to which they belong, is about one year. The approximate option values have been shown to produce small percentage errors and in particular the parity values “caplet = floorlet + swap” and “floorlet = caplet − swap” produce best results for ATM and ITM caplets and ITM floorlets.