Sums of Involving the Harmonic Numbers and the Binomial Coefficients ()
1. Introduction and Preliminaries
Let 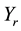 be the exponential complete Bell polynomials and
be the exponential complete Bell polynomials and
In [1] , Zave established the following series expansion:

 (1)
(1)
where  for
for ,
,  and
and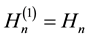 .
.
Spiess [2] introduced the numbers 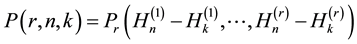 and
and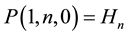 ,
,  for
for![]() ; then Equation (1.1) is equivalent to
; then Equation (1.1) is equivalent to
![]()
![]()
where![]() ,
, ![]() ,
, ![]() ,
,
![]()
The paper is organized as follows. In Section 2, we obtain some for ![]() and binomial coefficients by means of the Riordan arrays. In Section 3, we establish some identities involving the numbers
and binomial coefficients by means of the Riordan arrays. In Section 3, we establish some identities involving the numbers ![]() and inverse of binomial coefficients. Finally, in Section 4, we give the asymptotic expansions of some summations
and inverse of binomial coefficients. Finally, in Section 4, we give the asymptotic expansions of some summations
involving the numbers ![]() by Darboux’s method. Due to [3] [4] , a Riordan array is a pair
by Darboux’s method. Due to [3] [4] , a Riordan array is a pair ![]() of formal power series with
of formal power series with![]() . It defines an infinite lower triangular array
. It defines an infinite lower triangular array ![]() according to the rule
according to the rule
![]()
Hence we write![]() . If
. If ![]() is an Riordan array and
is an Riordan array and ![]() is the generating function of the sequence
is the generating function of the sequence![]() , i.e.,
, i.e.,![]() . Then we have
. Then we have
![]() (2)
(2)
Based on the generating function (1), we obtain the next Riordan arrays, to which we pay particular attention in the present paper:
![]() (3)
(3)
Lemma 1 (see [5] ) Let ![]() be a real number and
be a real number and![]() . When
. When![]() ,
,
![]()
![]()
2. Identities Involving the Numbers ![]() and Binomial Coefficients
and Binomial Coefficients
Theorem 1. Let![]() ,
, ![]() ,
, ![]() , then
, then
![]() (4)
(4)
Proof. By (1), we have
![]() (5)
(5)
Comparing the coefficients of ![]() on both sides of (5), we completes the proof of Theorem 1.
on both sides of (5), we completes the proof of Theorem 1.
Recall that ![]() Thus, setting
Thus, setting ![]() in Theorem 1 gives the next three identities, respectively.
in Theorem 1 gives the next three identities, respectively.
Corollary 1. Let![]() ,
, ![]() , the following relations hold
, the following relations hold
![]()
Theorem 2. Let![]() ,
, ![]() , then
, then
![]() (6)
(6)
Proof. To obtain the result, make use of the Theorem 1.
Theorem 3. Let![]() ,
, ![]() , then
, then
![]() (7)
(7)
Proof. Applying the summation property (2) to the Riordan arrays (3), we have
![]()
which is just the desired result.
Setting ![]() in Theorem 3 gives the next Corollary.
in Theorem 3 gives the next Corollary.
Corollary 2 Let![]() , then
, then
![]()
Corollary 3 Let![]() ,
, ![]() , then
, then
![]()
![]()
Proof. Setting ![]() in Theorem 3 gives Corollary 3.
in Theorem 3 gives Corollary 3.
Corollary 4. Let![]() , then
, then
![]()
Proof. Setting ![]() in Corollary 2 yields Corollary 4.
in Corollary 2 yields Corollary 4.
Theorem 4. Let![]() ,
, ![]() , then
, then
![]() (8)
(8)
Proof. which is just the desired result.
Setting ![]() in Theorem 4 gives the next Corollary.
in Theorem 4 gives the next Corollary.
Corollary 5. Let![]() , then
, then
![]()
Corollary 6. The substitutions ![]() in Theorem 4 gives the next four identities, respectively.
in Theorem 4 gives the next four identities, respectively.
![]()
Setting ![]() in Corollary 5 gives the next four identities, respectively.
in Corollary 5 gives the next four identities, respectively.
Corollary 7. Let![]() , then
, then
![]()
Theorem 5. Let![]() ,
, ![]() , then
, then
![]() (9)
(9)
where ![]() are the Stirling numbers of the first kind.
are the Stirling numbers of the first kind.
Proof. By (1) and (2), we have
![]()
which is just the desired result.
Setting ![]() in Theorem 5 gives the next Corollary.
in Theorem 5 gives the next Corollary.
Corollary 8. Let![]() , then
, then
![]()
Setting ![]() in Theorem 6 gives the next Corollary.
in Theorem 6 gives the next Corollary.
Corollary 9. Let![]() ,
, ![]() , then
, then
![]()
We give four applications of Corollary 9:
Corollary 10. Let![]() , then
, then
![]()
3. Identities Involving ![]() and Inverse of Binomial Coefficients
and Inverse of Binomial Coefficients
For identities involving Harmonic numbers and inverse of binomial coefficients ![]() in given in [6] .
in given in [6] .
In Section, we obtain some for ![]() and binomial coefficients by means of the Riordan arrays. From these identities, we deduce some identities involving binomial coefficients, Harmonic numbers and identities related to
and binomial coefficients by means of the Riordan arrays. From these identities, we deduce some identities involving binomial coefficients, Harmonic numbers and identities related to![]() ,
, ![]()
In [7] , the inverse of a binomial coefficient is related to an integral, as follows
![]() (10)
(10)
From the generating function of ![]() and (10), we have
and (10), we have
Theorem 6. For ![]() be any integer, then
be any integer, then
![]() (11)
(11)
Proof. From (1) and (10), we obtain
![]()
This gives (11).
Corollary 11 Setting ![]() in Theorem 6, The following relation holds:
in Theorem 6, The following relation holds:
![]() (12)
(12)
![]() (13)
(13)
![]() (14)
(14)
![]() (15)
(15)
Setting ![]() in Corollary 11, gives the next identities.
in Corollary 11, gives the next identities.
Corollary 12 The following relation holds
![]() (16)
(16)
![]() (17)
(17)
![]() (18)
(18)
![]() (19)
(19)
![]() (20)
(20)
![]() (21)
(21)
![]() (22)
(22)
![]() (23)
(23)
Corollary 13. The following relation holds
![]() (24)
(24)
![]() (25)
(25)
![]() (26)
(26)
![]() (27)
(27)
Proof. (16) minus(20) give (24); (17) minus (21), (18) minus (22) and (19) minus (23), yields (25), (26) and (27), respectively.
Leonhard Euler (1707-1783) had already stated the equation
![]()
Recall the Euler sum identities [8] [9] .
![]()
The next, we gives identities related to![]() ,
, ![]()
For completeness we supply proofs:
![]() (28)
(28)
![]() (29)
(29)
Similarly, we obtain summation formulas related![]() ,
, ![]()
![]() (30)
(30)
![]() (31)
(31)
By (18) and (28), (19) and (31), we have
![]() (32)
(32)
![]() (33)
(33)
Similarly, for completeness we supply a proof:
![]() (34)
(34)
By (28) minus (30), we get
![]() (35)
(35)
Applying (25) and (34), (26) and (32), we have
![]()
4. Asymptotics
Theorem 7 For ![]() be any integer, as
be any integer, as![]() , we have
, we have
![]() (36)
(36)
Proof. By Lemma 1, we have
![]()
and this complete the proof.
Similarly, we can obtain the next Theorem.
Theorem 8. Let ![]() be any integer, as
be any integer, as![]() , we have
, we have
![]() (37)
(37)
Theorem 9. For ![]() be any integer, as
be any integer, as![]() , we have
, we have
![]() (38)
(38)
Proof. By Lemma 1, we have
![]()
this give (38).
Theorem 10. For ![]() be any integer, as
be any integer, as![]() , we have
, we have
![]() (39)
(39)
Proof. By Corollary 3 of [10] , immediately complete the proof of Theorem 10.
Acknowledgements
The author would like to thank an anonymous referee whose helpful suggestions and comments have led to much improvement of the paper. The research is supported by the Natural Science Foundation of China under Grant 11461050 and Natural Science Foundation of Inner Mongolia under Grant 2012MS0118.