1. Introduction
The theory of quantum games is an evolving discipline that, similar to quantum information [1] [2] , explores the implications of quantum mechanics to fields outside physics proper, such as economics, finance, auctions, gambling, etc. [3] - [9] . One way of constructing a quantum game is to start from a standard (classical) game and to “quantize” it by formulating appropriate rules and letting the players employ quantum information tools such as qubits and quantum gates (or strategies in the quantum game nomenclature). This procedure has been applied on classical strategic games that describe an interactive decision-making in which each player chooses his strategy only once, and all choices are taken simultaneously. A simple example is a quantum game based on 2-player-2- strategies classical game usually defined by a game table (for example, the prisoner dilemma). We refer to it briefly as a 2-2 game.
2. Quantum Games: The Role of Entanglement
There is an extensive work on the quantized version of classical strategic 2-2 games, most of them are based on the protocol specified in [5] . It requires application of an entanglement (unitary) operator 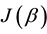 (where β is a real parameter), which acts on a non-entangled 2-qubit (pure) state resulting in an entangled state whose degree of entanglement is measured by its von-Neumann entropy
(where β is a real parameter), which acts on a non-entangled 2-qubit (pure) state resulting in an entangled state whose degree of entanglement is measured by its von-Neumann entropy![]() . A desired property of
. A desired property of ![]() is that
is that ![]() is a continuous function of β that varies (preferably monotonically) between 0 (no entanglement) and
is a continuous function of β that varies (preferably monotonically) between 0 (no entanglement) and ![]() (maximal entanglement). The reason for exploring partially entangled 2-qubit states is that the existence of pure strategy Nash equilibrium in the 2-2 quantum game crucially depends on the degree of entanglement (see below).
(maximal entanglement). The reason for exploring partially entangled 2-qubit states is that the existence of pure strategy Nash equilibrium in the 2-2 quantum game crucially depends on the degree of entanglement (see below).
Controlling the entropy by a single parameter that all values between 0 and ![]() are obtained is referred to here as single parameter completeness. The relevance of this problem to quantum information in general is quite obvious. An important procedure in quantum information is to design a quantum gate that generates Bell states [1] [2] . The gate, operating on a non-entangled two qubit state, results in a Bell state that is maximally entangled. Therefore, designing the gate
are obtained is referred to here as single parameter completeness. The relevance of this problem to quantum information in general is quite obvious. An important procedure in quantum information is to design a quantum gate that generates Bell states [1] [2] . The gate, operating on a non-entangled two qubit state, results in a Bell state that is maximally entangled. Therefore, designing the gate ![]() that does the job for an arbitrary two quNit state resulting in an entangled state whose degree of entanglement is controlled by a single parameter should be very useful.
that does the job for an arbitrary two quNit state resulting in an entangled state whose degree of entanglement is controlled by a single parameter should be very useful.
3. Classical Commensurability
Another practical property required from ![]() is that it can easily be constructed from the classical strategies. In a 2-2 game, the classical strategy of a player is
is that it can easily be constructed from the classical strategies. In a 2-2 game, the classical strategy of a player is![]() , and an appropriate construction is then
, and an appropriate construction is then
![]() . Its action on an unentangled 2-qubit state (e.g
. Its action on an unentangled 2-qubit state (e.g![]() ) yields,
) yields,
![]() , (1)
, (1)
where the left (right) factor in the Kronecker product refers to player 1 (2). In this way, ![]() appears in a Schmidt decomposed form, enabling an easy computation of the corresponding entanglement entropy of the 2-qubit state on the RHS as
appears in a Schmidt decomposed form, enabling an easy computation of the corresponding entanglement entropy of the 2-qubit state on the RHS as
![]() . (2)
. (2)
Thus, ![]() is a continuous function of
is a continuous function of ![]() and gets all values in
and gets all values in![]() , namely
, namely ![]() as defined in Equation (1) satisfies single parameter completeness. Other properties (of less significance) are that
as defined in Equation (1) satisfies single parameter completeness. Other properties (of less significance) are that ![]() is
is
periodic with period![]() , symmetric about the mid-point
, symmetric about the mid-point![]() , with
, with ![]() and
and![]() .
.
The entangler defined in Equation (1) has a property referred to as classical commensurability,
![]() . Following the rules of the game specified in [5] , it means that players in a 2-2 quantum game can, if they wish, use their classical strategies as a special case and if they do so, they collect the corresponding classical payoffs. In most cases, however, the classical strategies do not constitute a pure-strategy Nash Equilibrium (NE) (defined below). Generically, as we explain below, classical commensurability does not hold for
. Following the rules of the game specified in [5] , it means that players in a 2-2 quantum game can, if they wish, use their classical strategies as a special case and if they do so, they collect the corresponding classical payoffs. In most cases, however, the classical strategies do not constitute a pure-strategy Nash Equilibrium (NE) (defined below). Generically, as we explain below, classical commensurability does not hold for ![]() [10] .
[10] .
4. The Present Work
In the present work we examine the issue of constructing ![]() for a
for a ![]() quantum game based on a 2- players-N-strategies classical game. We suggest a natural extension of Equation (1) for constructing an operator
quantum game based on a 2- players-N-strategies classical game. We suggest a natural extension of Equation (1) for constructing an operator ![]() that turns a non-entangled 2-quNit state into an entangled one. For
that turns a non-entangled 2-quNit state into an entangled one. For ![]() the corresponding von- Neumann entropy
the corresponding von- Neumann entropy ![]() varies continuously between 0 and
varies continuously between 0 and ![]() so that single parameter completeness is satisfied. Unfortunately, this method does not work for
so that single parameter completeness is satisfied. Unfortunately, this method does not work for ![]() because in that case
because in that case![]() . To alleviate this deficiency we suggest another method (albeit less intuitive) to design
. To alleviate this deficiency we suggest another method (albeit less intuitive) to design ![]() that satisfies single parameter completeness for any
that satisfies single parameter completeness for any![]() . In what follows we will first introduce the classical 2-3 game using quantum information language and formulate its quantum version (extension to
. In what follows we will first introduce the classical 2-3 game using quantum information language and formulate its quantum version (extension to ![]() is straightforward). Most of this introductory exposition is well established and is included here merely for self consistence. Then, in the second step, we shall address the issue of constructing
is straightforward). Most of this introductory exposition is well established and is included here merely for self consistence. Then, in the second step, we shall address the issue of constructing![]() .
.
5. Two-Players-Three-Strategies Classical Games: Trits
Consider a two-players classical game with three strategies for each player. For example, two prisoners may have three options, marked as three values of a trit![]() ,
, ![]() and
and ![]() for Confess, Stay quiet or Don’t confess. The two prisoner “system” can be found in nine two-trit states
for Confess, Stay quiet or Don’t confess. The two prisoner “system” can be found in nine two-trit states![]() ,
, ![]() ,
, ![]() , corresponding to the nine entries of the game table. The protocol of the classical game with 2-players and 3-strategies is as follows: The referee (judge) calls the players (prisoners) and tells them he assumes that they are in an initial two-trit state
, corresponding to the nine entries of the game table. The protocol of the classical game with 2-players and 3-strategies is as follows: The referee (judge) calls the players (prisoners) and tells them he assumes that they are in an initial two-trit state ![]() meaning (C,C) namely both confess. He then asks them to decide whether to leave their respective trit state as it is on
meaning (C,C) namely both confess. He then asks them to decide whether to leave their respective trit state as it is on ![]() or to change it either to
or to change it either to ![]() (meaning S) or to
(meaning S) or to ![]() (meaning D). These replacement operations (specified explicitly below) are the players’ classical strategies. If the initial state suggested by the referee is
(meaning D). These replacement operations (specified explicitly below) are the players’ classical strategies. If the initial state suggested by the referee is ![]() the strategies of the two players include
the strategies of the two players include ![]() (leaving the trit at
(leaving the trit at ![]() as it is),
as it is), ![]() (swapping of
(swapping of ![]() and
and ![]() namely, replacing C by S) and
namely, replacing C by S) and ![]() (swapping
(swapping ![]() and
and ![]() namely replacing C
namely replacing C
by D). These three operations generate the group ![]() of permutations of three elements
of permutations of three elements![]() . Explicitly,
. Explicitly,
![]()
We shall indicate below that a quantum strategy is a gate represented by an ![]() matrix. A reasonable requirement for the procedure of “quantizing” a classical game is that the classical strategies obtain as special case of the quantum ones. For that purpose we need to construct a representation of the permutation group
matrix. A reasonable requirement for the procedure of “quantizing” a classical game is that the classical strategies obtain as special case of the quantum ones. For that purpose we need to construct a representation of the permutation group ![]() in terms of unitary matrices with unit determinant. This can be achieved by choosing
in terms of unitary matrices with unit determinant. This can be achieved by choosing
![]() . (3)
. (3)
For example, suppose player 1 and 2 choose respective strategies ![]() and
and![]() . This brings the system into a state
. This brings the system into a state![]() . Then the respective payoff of player
. Then the respective payoff of player![]() ,
, ![]() will be
will be![]() , where
, where ![]() is the payoff of player
is the payoff of player ![]() at entry
at entry ![]() of the game table.
of the game table.
6. The Analogous Quantum Game: 1 and 2 Quirt States
We now briefly explain the structure of the corresponding quantum game. Its main ingredients are qutrits, quantum strategies, and entanglement operations. Both versions use the same game table but the payoff rules are somewhat different.
Consider the three dimensional Hilbert space ![]() with orthonormal basis vectors
with orthonormal basis vectors![]() ,
, ![]() and
and![]() . A qutrit is a vector
. A qutrit is a vector ![]() of unit norm,
of unit norm,![]() . A general 2-qutrit state is a normalized vector in
. A general 2-qutrit state is a normalized vector in![]() ,
,
![]() (4)
(4)
A maximally entangled two-qutrit state is written as
![]() , (5)
, (5)
given in a Schmidt decomposed form. Its entanglement degree is measured by the von Neumann entropy
![]() .
.
7. 2-3 Quantum Game Strategies
A strategy of a player in a 2-3 quantum game is an ![]() matrix by which he operates on his qutrit (that is a quantum gate). A strategy
matrix by which he operates on his qutrit (that is a quantum gate). A strategy ![]() depends on eight Euler angles
depends on eight Euler angles![]() . The explicit expression of
. The explicit expression of ![]() in terms of Gellman matrices
in terms of Gellman matrices![]() ,
, ![]() is well known. For quantum game theory, a practical parametrization of
is well known. For quantum game theory, a practical parametrization of ![]() is suggested in [10] .
is suggested in [10] .
8. 2-3 Quantum Game Procedure
The referee suggests an initial non-entangled two-qutrit state ![]() (e.g, the analog of the classical two trit state
(e.g, the analog of the classical two trit state![]() ). Before letting the players apply their quantum strategies, the referee operates on
). Before letting the players apply their quantum strategies, the referee operates on ![]() with a unitary operator
with a unitary operator ![]() such that
such that ![]() is entangled (otherwise the game remains classical). Construction of the operator
is entangled (otherwise the game remains classical). Construction of the operator ![]() (our central goal) is detailed below. At this stage of the game, the players apply their respective strategies
(our central goal) is detailed below. At this stage of the game, the players apply their respective strategies![]() . Finally, the referee applies the operator
. Finally, the referee applies the operator![]() , leading to the final state
, leading to the final state
![]() , (6)
, (6)
where ![]() is the octet of 8 Euler angles defining the
is the octet of 8 Euler angles defining the ![]() matrix
matrix ![]() (that is the strategy of player
(that is the strategy of player![]() ). The payoff
). The payoff ![]() of player
of player ![]() is given by
is given by
![]() , (7)
, (7)
where ![]() are the payoffs at entry
are the payoffs at entry ![]() of the classical game table. Like in the classical game, each player choses a strategy with the goal of maximizing his payoff.
of the classical game table. Like in the classical game, each player choses a strategy with the goal of maximizing his payoff.
9. Pure Strategy Nash Equilibrium (NE)
Because the set of 8 Euler angles ![]() uniquely determines the player’s strategy
uniquely determines the player’s strategy![]() , a pure strategy NE in the 2-3 quantum game is a pair of strategies
, a pure strategy NE in the 2-3 quantum game is a pair of strategies ![]() (each represents 8 angles) such that
(each represents 8 angles) such that
![]() (8)
(8)
The question of whether pure strategy NE exists in a 2-2 quantum game and its relation to the degree of entanglement (controlled by![]() ) has been discussed in numerous works [11] - [17] . In brief, if there is NE in the classical game that is not Pareto efficient [18] , then there is a critical value
) has been discussed in numerous works [11] - [17] . In brief, if there is NE in the classical game that is not Pareto efficient [18] , then there is a critical value ![]() above which there is no pure strategy NE in the quantum game. As
above which there is no pure strategy NE in the quantum game. As ![]() approaches
approaches ![]() from below, the respective payoffs in the quantum game at NE approach the Pareto point of cooperation [13] [17] . This is the main reason why, right from the onset, we stress the relevance of partially entangled 2-quNit states where
from below, the respective payoffs in the quantum game at NE approach the Pareto point of cooperation [13] [17] . This is the main reason why, right from the onset, we stress the relevance of partially entangled 2-quNit states where![]() .
.
10. Absence of Classical Commensurability
We now explain why in a 2-3 quantum game there is no classical commensurability [10] . Recall that classical commensurability means that if the players use classical strategies they respectively get their classical payoffs. For a classical strategy ![]() we have
we have![]() . From Equation (6) it means that
. From Equation (6) it means that ![]() should commute with all outer products of the classical strategies. If the initial state is
should commute with all outer products of the classical strategies. If the initial state is ![]() the 9 outer products of classical strategies are
the 9 outer products of classical strategies are![]() , where
, where ![]() (see Equation (3)). Classical commensurability then requires
(see Equation (3)). Classical commensurability then requires
![]() . (9)
. (9)
This is possible only if J is a function of ![]() where A is a
where A is a ![]() matrix satisfying
matrix satisfying ![]()
![]() , and
, and ![]() is not just a multiple of
is not just a multiple of![]() . But this is impossible because
. But this is impossible because ![]() and
and ![]() generate an irreducible representation of the permutation group
generate an irreducible representation of the permutation group ![]() and hence, according to Schure’s lemma
and hence, according to Schure’s lemma ![]() is just a multiple of
is just a multiple of![]() . These arguments naturally hold for any
. These arguments naturally hold for any![]() .
.
11. Designing the Entangler ![]()
The main result of the present study concerns the analysis and construction of an entanglement operator ![]() for
for![]() . We carry it out for
. We carry it out for ![]() and then extend it straightforwardly to any
and then extend it straightforwardly to any![]() . For
. For ![]() we require that
we require that ![]() yields an entangled 2-qutrit state with
yields an entangled 2-qutrit state with ![]() specifying the degree of entanglement that achieves any value between 0 and
specifying the degree of entanglement that achieves any value between 0 and![]() . Following the 2-2 game framework specified in Equation (1), we try to construct J by exponentiating a combination of classical strategies. In order to get the “diagonal” 2-qutrit states
. Following the 2-2 game framework specified in Equation (1), we try to construct J by exponentiating a combination of classical strategies. In order to get the “diagonal” 2-qutrit states ![]() and
and ![]() from the qutrit state
from the qutrit state![]() , we have to operate on
, we have to operate on ![]() with
with![]() . Therefore, we define
. Therefore, we define
![]() . (10)
. (10)
Calculation of the exponent yields
![]() . (11)
. (11)
Maximal entanglement obtains when the absolute values of all three coefficients are equal, namely
![]() . (12)
. (12)
Here ![]() raises monotonically from 0 to its first maximum
raises monotonically from 0 to its first maximum![]() , hence we have found the desired entanglement operator that satisfies single parameter completeness. Figure 1(a) displays the von Neumann entropy
, hence we have found the desired entanglement operator that satisfies single parameter completeness. Figure 1(a) displays the von Neumann entropy ![]() of the entangled 2-qutrit state (11) as function of
of the entangled 2-qutrit state (11) as function of![]() . Here again it possesses other properties, namely
. Here again it possesses other properties, namely
![]() is a periodic function of
is a periodic function of ![]() with period
with period ![]() and it is symmetric about the mid-point
and it is symmetric about the mid-point ![]() where it has a local minimum. It has two maxima for
where it has a local minimum. It has two maxima for ![]() where it equals
where it equals![]() . Inspecting Equation (10),
. Inspecting Equation (10),
we see that, in quantum games, the entanglement is not obtained in terms of spin rotations but, rather, in terms of permutation exponentials that are SU(3) matrices (for ![]() these are the same).
these are the same).
12. Extension to Arbitrary N
Let ![]() denote the
denote the ![]() matrix representing the permutation
matrix representing the permutation ![]() and let
and let![]() ,
, ![]() be an unentangled 2-quNit state. To get an entangled state from
be an unentangled 2-quNit state. To get an entangled state from ![]() we define and assert that
we define and assert that
![]() . (13)
. (13)
To proceed, let us define the absolute value squared of the coefficients
![]() . (14)
. (14)
It is easy to verify that: 1)![]() ; 2)
; 2)![]() ; 3)
; 3) ![]() is periodic with pe-
is periodic with pe-
riod ![]() and symmetric about the mid-point
and symmetric about the mid-point![]() . The entanglement entropy is
. The entanglement entropy is
![]() . (15)
. (15)
Maximal entanglement ![]() obtains for
obtains for ![]() that is the solution of the equality
that is the solution of the equality
![]() . (16)
. (16)
For ![]() the two solutions are specified in Equation (12). For
the two solutions are specified in Equation (12). For![]() , there is a single solution at
, there is a single solution at![]() , as shown in Figure 1(b). Thus, for
, as shown in Figure 1(b). Thus, for ![]() we have achieved our goal of constructing an entanglement operator
we have achieved our goal of constructing an entanglement operator ![]() such that the degree of entanglement
such that the degree of entanglement ![]() of the 2-quNit state
of the 2-quNit state ![]() varies continuously reaching all values in the interval
varies continuously reaching all values in the interval![]() , so that single parameter completeness is satisfied.
, so that single parameter completeness is satisfied.
For ![]() there is no solution
there is no solution ![]() of Equation (16), and maximal entanglement is not achieved. It might be argued that 2-N quantum games with
of Equation (16), and maximal entanglement is not achieved. It might be argued that 2-N quantum games with ![]() are much rarer than those with smaller N but we believe that the construction of
are much rarer than those with smaller N but we believe that the construction of ![]() that satisfies single parameter completeness also for
that satisfies single parameter completeness also for ![]() is useful in other areas (outside the ballpark of quantum game theory), so we carry it out for the sake of completeness.
is useful in other areas (outside the ballpark of quantum game theory), so we carry it out for the sake of completeness.
The method suggested here is not based on permutation exponentials as in Equation (10). It consists of the following steps.
1) Assume a lexicographic order of the ![]() basis states
basis states ![]() such that the diagonal states
such that the diagonal states ![]() appear in
appear in
the first N places. Choose a unitary ![]() matrix of the form
matrix of the form ![]() where R is an
where R is an ![]() uniray matrix with equal first column elements
uniray matrix with equal first column elements ![]() and
and ![]() is the
is the ![]() unit matrix. The problem is
unit matrix. The problem is
then reduced to the ![]() dimensional subspace spanned by
dimensional subspace spanned by![]() . By construction,
. By construction,
![]()
that is a maximally entangled state.
2) Diagonalize ![]() as
as ![]() where
where ![]() is the matrix of eigenvectors of
is the matrix of eigenvectors of![]() , and
, and
![]()
is the diagonal matrix of (unimodular) eigenvalues of ![]() with eigenphases
with eigenphases![]() .
.
3) Now consider the matrix
![]() . (17)
. (17)
By construction, ![]() and
and![]() . Hence, the state
. Hence, the state ![]() is partially entangled. Since it is given in a Schmidt decomposed form, the corresponding von Neumann entropy
is partially entangled. Since it is given in a Schmidt decomposed form, the corresponding von Neumann entropy ![]() is easily calculable.
is easily calculable. ![]() is continuous in
is continuous in ![]() with
with ![]() and
and![]() , namely single parameter completeness is satisfied. Generically, the eigen phases are not rational multiples of
, namely single parameter completeness is satisfied. Generically, the eigen phases are not rational multiples of ![]() so that
so that ![]() is not periodic, but this lack of periodicity is of no special significance.
is not periodic, but this lack of periodicity is of no special significance.
13. Illustration for ![]()
A convenient way to build an appropriate unitary ![]() matrix
matrix ![]() is to start from a simple non-singular matrix
is to start from a simple non-singular matrix ![]() and then orthogonalize it within the Grahm-Schmidt procedure. For example,
and then orthogonalize it within the Grahm-Schmidt procedure. For example,
![]()
Proceeding with the list of steps prescribed above we can easily construct ![]() and compute the von Neumann entropy of the state
and compute the von Neumann entropy of the state ![]() upon which the players apply their strategies according to the game protocol specified in Equation (6). The result is given in Figure 2.
upon which the players apply their strategies according to the game protocol specified in Equation (6). The result is given in Figure 2.
As explained in the figure’s caption, the degree of entanglement is controlled by a single parameter and ![]() is a continuous function of
is a continuous function of ![]() reaching any value in the interval
reaching any value in the interval![]() . Thus we have achieved our goal of constructing an entangler
. Thus we have achieved our goal of constructing an entangler ![]() that turns a non-entangled 2-quNit state into an entangled one given in a Schmidt decomposed form with single parameter completeness satisfied.
that turns a non-entangled 2-quNit state into an entangled one given in a Schmidt decomposed form with single parameter completeness satisfied.
14. Summary
In conclusion, we suggest two methods to design an entanglement operator ![]() that turns a non-entangled
that turns a non-entangled
2-quNit state to a partially entangled state whose von Neumann entropy is fully controlled by a single real parameter. The first method is intuitively clear and simple, based on exponential of classical strategies, Equation (10), and results in the von Neumann entropy, as displayed in Figure 1. This method does not work for ![]() because the resulting entropy does not reach the maximally entangled value
because the resulting entropy does not reach the maximally entangled value![]() . For that reason we suggest another method that is somewhat less transparent but works for any N. The resulting entropy as function of
. For that reason we suggest another method that is somewhat less transparent but works for any N. The resulting entropy as function of ![]() is displayed in Figure 2.
is displayed in Figure 2.
The entangler ![]() constructed here generalizes, in two directions, the familiar quantum gate used in quantum information science to create Bell states from non-entangled two-qubit state. First, it is applicable to any two-quNit state, and second, it contains a single continuous parameter that controls the degree of entanglement of the resultant state.
constructed here generalizes, in two directions, the familiar quantum gate used in quantum information science to create Bell states from non-entangled two-qubit state. First, it is applicable to any two-quNit state, and second, it contains a single continuous parameter that controls the degree of entanglement of the resultant state.
Acknowledgements
I would like to thank Oscar Vollij for his excellent course in (classical) game theory. Discussions with Eytan Bachmat, Hosho Katsura, Rioichi Shindou, Doron Cohen and Yehuda Band are highly appreciated. This work is partially supported by grant 400/2012 of the Israeli Science Foundation (ISF).