Keywords:
The inverse problems generally are ill-posed in Hadamard sense. These words lead us to think that there exist inverse problems that are well-posed and which are possible to be solved analytically [1].
The ill-posed problems do not fulfill with at least one of the conditions of existence, uniqueness or stability of the solution. Nevertheless, if a problem does not have a solution, or the solution is not unique, it is possible to correct in spite of doing considerations on the domain and the co-domain of the operator who represents the problem.
Unlike a complex number different from zero for which, it is always possible to find his logarithm. The functions need certain conditions on his domain to guarantee the existence of his logarithm [2]. It appears that one of these conditions is that the domain is simply connected. To assure the uniqueness of the logarithm of a function, it is es- sential to take a branch of the logarithm.
In this paper, there appears the problem of finding the logarithm of a given function, by different techniques. It is demonstrated that the above-mentioned problem is an inverse stable problem in Hadamard sense [3]. Therefore, the problem of existence and uniqueness is solved. In addition, it is demonstrated that it is stable, which transforms it into a well-posed inverse problem. It is realized the analysis of the solution of the inverse problem for when the do- main of the inverse operator, it corresponds to the functions that are not annulled and in addition they are holomor- phic in some region [4]. It is demonstrated that when 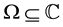 is a simply connected domain, the solution of the in- verse problem exists and is unique. In a similar way, when the space
is a simply connected domain, the solution of the in- verse problem exists and is unique. In a similar way, when the space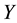 , it corresponds to the space of the conti- nuous functions that are not annulled on a region
, it corresponds to the space of the conti- nuous functions that are not annulled on a region 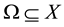 simply connected and where Z is a Hausdorff’s space and connected for paths, the solution of the inverse problem exists, is unique and is stable [5].
simply connected and where Z is a Hausdorff’s space and connected for paths, the solution of the inverse problem exists, is unique and is stable [5].
2. Exposition of the problem
It’s known that not all the real numbers have logarithm, nevertheless, all complex numbers have it.
The natural question that arises is under what conditions a function has logarithm. To answer to the previous question, an operator 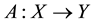 is considered, if
is considered, if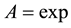 , and
, and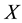 ,
,  are spaces of functions, it is possible to pose the direct problem: From a function on
are spaces of functions, it is possible to pose the direct problem: From a function on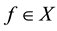 , find to a function
, find to a function  such that
such that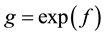 . The corresponding inverse problem is given a function
. The corresponding inverse problem is given a function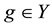 , to find to a function
, to find to a function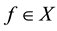 , such that
, such that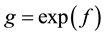 . It’s known that the inverse of the operator
. It’s known that the inverse of the operator 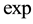 is the operator
is the operator , so the inverse problem can be seen as the search of the logarithm of a given function. Notice that as is considered the operator
, so the inverse problem can be seen as the search of the logarithm of a given function. Notice that as is considered the operator , it has felt to take as space
, it has felt to take as space 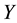 to the functions that are not annulled and in addition be continuous or holomorphic on some region; nevertheless they must determine the conditions that the region must fulfill to demonstrate the existence, uni- queness and stability of the inverse problem.
to the functions that are not annulled and in addition be continuous or holomorphic on some region; nevertheless they must determine the conditions that the region must fulfill to demonstrate the existence, uni- queness and stability of the inverse problem.
3. Holomorphic logarithm
In this section, it is considered that  corresponds to the holomorphic functions and
corresponds to the holomorphic functions and  to the holomorphic functions that are not annulled on any region. There’s demonstrated that when Ω is a simply connected domain, the solution of the inverse problem exists and is unique when a branch of the logarithm is fixed. The problem consists of knowing if given a function holomorphic f can be a function
to the holomorphic functions that are not annulled on any region. There’s demonstrated that when Ω is a simply connected domain, the solution of the inverse problem exists and is unique when a branch of the logarithm is fixed. The problem consists of knowing if given a function holomorphic f can be a function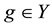 , such that
, such that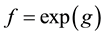 .
.
Definition: Given a domain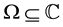 , and be
, and be 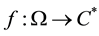 and
and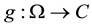 . It is said that
. It is said that  is a loga- rithm of
is a loga- rithm of 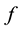 if
if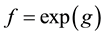 . If the functions
. If the functions 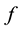 and
and 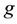 are holomorphic in Ω, it is said that
are holomorphic in Ω, it is said that 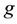 is a ho- lomorphic logarithm of
is a ho- lomorphic logarithm of  [6].
[6].
Theorem 1: Let 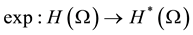 be, where Ω is a simply connected set of the plane, then the so- lution of the inverse problem exists and is unique.
be, where Ω is a simply connected set of the plane, then the so- lution of the inverse problem exists and is unique.
Proof: If it thinks that Ω it is a simply connected domain, by theorem of the Riemann application, the simply connected domains of the flat sound of two types: the conformal equivalent to the plane 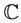 and the conformal equivalent to the unitary disc D. For such a motive, the existence of the solution of the inverse problem, are obtained as a consequence of the propositions 1, 2 and 3. As for the uniqueness, it is essential to take a branch of the logarithm.
and the conformal equivalent to the unitary disc D. For such a motive, the existence of the solution of the inverse problem, are obtained as a consequence of the propositions 1, 2 and 3. As for the uniqueness, it is essential to take a branch of the logarithm.
Proposition 1: Every function 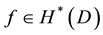 admits a holomorphic logarithm.
admits a holomorphic logarithm.
Proof: It is known that 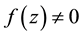 for every
for every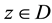 . Let
. Let 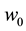 be a point such that
be a point such that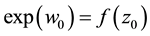 . It’s de- fined by:
. It’s de- fined by:
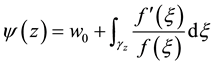 , (1)
, (1)
where 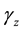 is the curve that joins to
is the curve that joins to 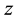 with
with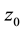 .
. 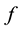 it’s holomorphic and is not annulled on
it’s holomorphic and is not annulled on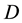 , then
, then  is holomorphic on
is holomorphic on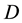 . Then,
. Then, 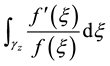 it´s definite as well. This way,
it´s definite as well. This way, . Consider
. Consider
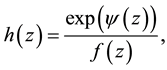 then
then 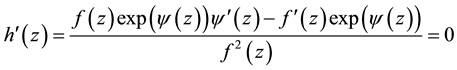
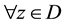 . Then,
. Then, 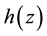 it is constant on
it is constant on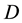 . So,
. So, 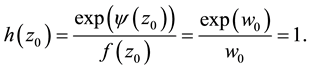
Then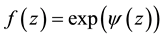 .
.
Let 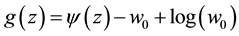 be, then
be, then  and the proof it is complete.
and the proof it is complete.
Proposition 2: Let Ω be a simply connected domain own of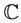 , admits a holomorphic logarithm.
, admits a holomorphic logarithm.
Proof: Consider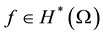 , since Ω be a simply connected domain own of
, since Ω be a simply connected domain own of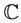 , there is an conformal function
, there is an conformal function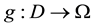 , such that
, such that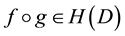 . Since
. Since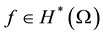 , follows that
, follows that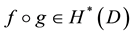 . By the propo- sition 1, the function
. By the propo- sition 1, the function 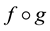 admits a holomorphic logarithm in
admits a holomorphic logarithm in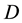 . That is, there exists
. That is, there exists 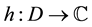 such that
such that 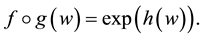
Hereby 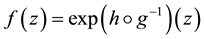 and therefore
and therefore 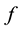 admits a holomorphic logarithm in Ω.
admits a holomorphic logarithm in Ω.
Proposition 3: Every function 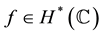 admits a holomorphic logarithm.
admits a holomorphic logarithm.
Proof: Since 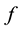 it is not annulled in
it is not annulled in  and
and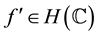 . Then
. Then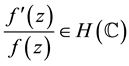 , that is, there exist a function
, that is, there exist a function 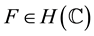 such that is the primitive of
such that is the primitive of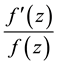 , that is to say,
, that is to say, 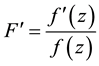 for everything
for everything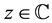 . Notice that
. Notice that
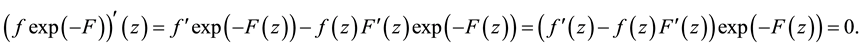
Then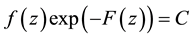 , if
, if 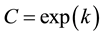 it follows that
it follows that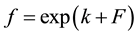 .
.
4. Continuous logarithm
In this section, the inverse problem it’s studied, when the condition weakens of being a holomorphic function to being a continuous function. Nevertheless, on having asked him only continuity to the functions and hav- ing tried to solve the inverse problem, it is needed to do more restrictions on the domain of the above men- tioned functions.
It is considered to be that 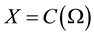 and the space
and the space 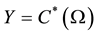 that correspond to the spaces of conti- nuous and continuous that are not annulled function respectively. There is demonstrated that when
that correspond to the spaces of conti- nuous and continuous that are not annulled function respectively. There is demonstrated that when 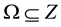 it’s a simply connected domain and
it’s a simply connected domain and 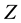 is Hausdorff space and connected by paths, the solution of the inverse problem exists, is unique if a branch of the logarithm is fixed adapted and is stable.
is Hausdorff space and connected by paths, the solution of the inverse problem exists, is unique if a branch of the logarithm is fixed adapted and is stable.
Theorem 2 Let 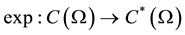 be, where
be, where 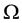 a simply connected set is (not necessarily it is a subset of the plane) content in Hausdorff space and connected by paths; then the solution of the inverse problem exists, is unique and is stable.
a simply connected set is (not necessarily it is a subset of the plane) content in Hausdorff space and connected by paths; then the solution of the inverse problem exists, is unique and is stable.
Proof: The existence of the solution of the inverse problem, it’s demonstrated in the proposition 3 and the ex- istence in the proposition 4.
Since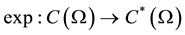 , where
, where 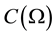 and
and 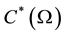 are Banach spaces, in addition, the operator is continuous; then, by the open mapping theorem, the inverse operator is continuous and therefore, the solution of the inverse problem is stable.
are Banach spaces, in addition, the operator is continuous; then, by the open mapping theorem, the inverse operator is continuous and therefore, the solution of the inverse problem is stable.
Definition: Let 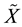 and
and 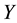 be topological spaces. Consider to
be topological spaces. Consider to 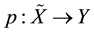 a continuous function. Is say that
a continuous function. Is say that 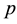 is a covering map if
is a covering map if 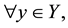 it exists a neighborhood
it exists a neighborhood 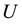 for
for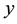 , with following properties:
, with following properties:
1) 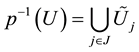 with
with 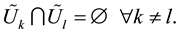
2) 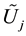 is a neighborhood for
is a neighborhood for 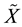 such that
such that 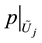 is a homeomorphism on
is a homeomorphism on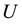 ,
,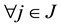 .
.
Proposition 4: The 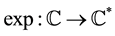 function is a covering map.
function is a covering map.
Definition: Let  be a continuous function and let
be a continuous function and let  be a covering map. The
be a covering map. The 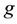 ap- plication is a lifting for
ap- plication is a lifting for 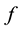 (in respect of
(in respect of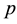 ) if
) if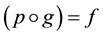 .
.
Note that if it is known that the exp function is a covering map, then to define the lifting, and under the condi- tions for the existence and uniqueness of the lifting, it will be had that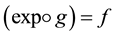 ; and therefore on having demonstrated the existence and uniqueness of the lifting, there will be demonstrated the existence and unique- ness of the logarithm of a continuous function .
; and therefore on having demonstrated the existence and uniqueness of the lifting, there will be demonstrated the existence and unique- ness of the logarithm of a continuous function .
4.1. Uniqueness of the lifting
For the uniqueness of the lifting it is necessary that the 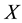 topological space be connected and Hausdorff.
topological space be connected and Hausdorff.
Theorem 3 Let 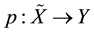 be a local homeomorphism, let
be a local homeomorphism, let 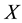 be a connected and Hausdorff space and let
be a connected and Hausdorff space and let 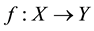 be a continuous application. Suppose that
be a continuous application. Suppose that 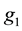 and
and 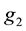 are lifting of
are lifting of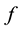 . Then, if
. Then, if  exists, such that
exists, such that , it follows that
, it follows that 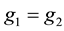 in
in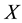 .
.
Proof: The set 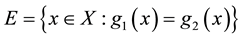 is defined. Note that
is defined. Note that 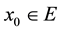 and therefore
and therefore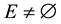 . If it is shown that
. If it is shown that 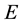 is open and closed it follow that
is open and closed it follow that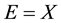 . To show that
. To show that 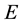 is a closed set, enough to prove that
is a closed set, enough to prove that 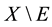 is an open set. Let
is an open set. Let 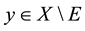 be, clearly
be, clearly 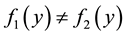 and since
and since  is a Hausdorff space, exist
is a Hausdorff space, exist
neighborhoods 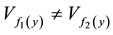 for
for 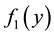 and
and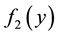 , respectively such that
, respectively such that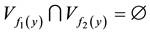 . Consider that
. Consider that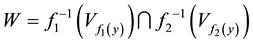 , note that
, note that 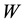 isn’t an empty set, then
isn’t an empty set, then 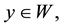 so,
so, 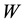 is open set since is a
is open set since is a
finite intersection of open sets. Let 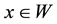 be, then
be, then 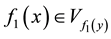 and
and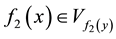 . What implies that
. What implies that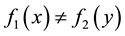 . Therefore
. Therefore 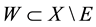 and so, the
and so, the 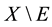 open set and then the
open set and then the 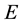 set is closed. To show that
set is closed. To show that 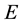 is an open set. Let
is an open set. Let 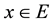 be and
be and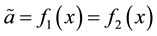 . By hypothesis, there is a neighborhood
. By hypothesis, there is a neighborhood 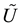 for
for 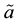 such that
such that 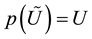 is open set and
is open set and 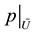 is an homeomorphism. Since
is an homeomorphism. Since 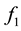 and
and 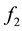 are continuous func- tion, there is
are continuous func- tion, there is 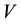 an neighborhood for
an neighborhood for  such that
such that 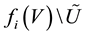 and
and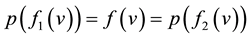 . Since
. Since 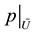 is an injective function, it follows that
is an injective function, it follows that 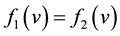 so,
so, 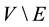 it’s a closed set and then the
it’s a closed set and then the 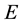 set is open. Since
set is open. Since 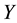 is connected space and
is connected space and 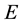 is a non-empty, open and closed set, it follows that
is a non-empty, open and closed set, it follows that .
.
4.2. Existence of the lifting
The study of the existence of the lifting needs of the study of the fundamental group.
Let 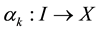 be, where
be, where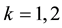 , curves that begin and end in
, curves that begin and end in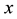 , it is to say, they are closed curves. Is said that
, it is to say, they are closed curves. Is said that 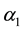 it is related with
it is related with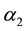 ,
, 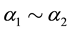 , if it exists
, if it exists 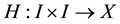 a continuous function such that
a continuous function such that
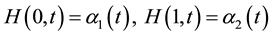 ,
, 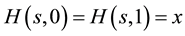
The 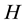 function is called continuous homotopy.
function is called continuous homotopy.
It is easy to see that the relation 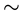 is an equivalence relation. Since it is known well, everything equivalence relation induces a partition. In this case the classes
is an equivalence relation. Since it is known well, everything equivalence relation induces a partition. In this case the classes  are formed by the set of curves
are formed by the set of curves 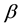 that are homotopic to
that are homotopic to . Intuitively it is possible to define the operation join curved and the above mentioned operation gives a structure of group.
. Intuitively it is possible to define the operation join curved and the above mentioned operation gives a structure of group.
Definition: The first group of homotopy of 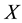 is defined, with basis
is defined, with basis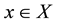 :
:
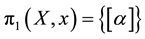 ,
,
where 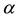 is a closed curve.
is a closed curve.
We noted that 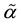 is the curve
is the curve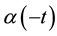 .
.
The following result gives necessary and sufficient conditions for the existence of the lifting.
Theorem 4: Let 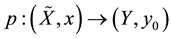 be a covering map and let
be a covering map and let 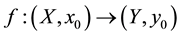 be a continuous function, with
be a continuous function, with 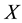 a connected by paths set. Then they are equivalent:
a connected by paths set. Then they are equivalent:
1) A lifting 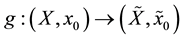 there exists for
there exists for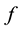 ;
;
2)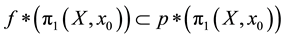 .
.
Where 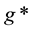 is the induced mapping of fundamental groups.
is the induced mapping of fundamental groups.
Proof: The demonstration  obviously, because
obviously, because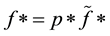 .
.
There will be demonstrated that . Let
. Let  be and let
be and let  be a curve in
be a curve in 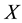 from
from  to
to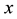 . The curve
. The curve 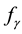 in
in  that it begins in
that it begins in 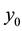 it has a unique lifting
it has a unique lifting  that it begins
that it begins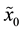 .
. 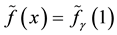 is defined. It is demonstrated that it is definite as well, independently of the choice of
is defined. It is demonstrated that it is definite as well, independently of the choice of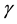 , let
, let 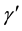 be another curve from
be another curve from 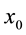 to
to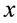 . Then
. Then  is a closed curve of
is a closed curve of 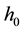 to
to 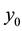 with:
with:
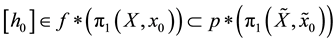 .
.
This means that there is a homotopy 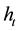 from
from 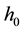 to a closed curve
to a closed curve 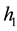 that gets up to a closed curve
that gets up to a closed curve 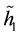 in
in 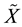 based in
based in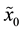 . Property homotopy covering is applied to
. Property homotopy covering is applied to 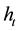 to obtain a lifting
to obtain a lifting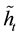 . Since
. Since 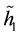 is a closed curve to
is a closed curve to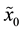 ,
, 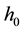 it’s too. By the uniqueness of the lift curve, the first half of
it’s too. By the uniqueness of the lift curve, the first half of 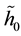 is
is 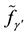 and the second half is
and the second half is 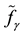 route the other way around, with the common midpoint
route the other way around, with the common midpoint . This shows that
. This shows that 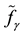 it´s definite as well. Need to show that
it´s definite as well. Need to show that 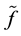 is a continuous function. Let
is a continuous function. Let  be a neighborhood of
be a neighborhood of 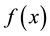 that it has a lifting
that it has a lifting 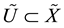 containing to
containing to 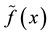 such that
such that 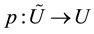 is an homeomorphism. A connected by path neighborhood
is an homeomorphism. A connected by path neighborhood 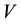 is chosen for
is chosen for 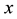 with
with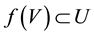 . The curves from
. The curves from 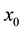 to
to 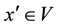 points, it is possible to take a given fixed curve
points, it is possible to take a given fixed curve 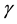 from
from 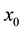 to
to 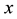 follow by curves
follow by curves 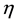 in
in 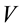 from y to the points
from y to the points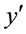 . Then, the curves
. Then, the curves 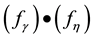 in
in 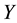 it has lifting
it has lifting 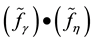 where
where 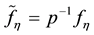 and
and 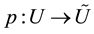 is the inverse of
is the inverse of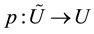 . So,
. So, 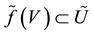 and
and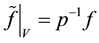 , therefore
, therefore 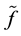 is continuous in
is continuous in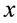 .
.
References